Complete Solutions to Level-VI Passage answer of chapter MOTION IN A PLANE of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | MOTION IN A PLANE |
| NARAYANA | Level-VI Passage answer |
| Number Of Questions | 18 |
| Academic Year | 2023-24 |
A river of width `w` is flowing such that the stream velocity varies with `y` as `v_(R)=v_(0)[1+(sqrt3-1)/wy]`, where `y` is the perpendicular distance from one bank.A boat starts rowing from the bank with constant velocity `v=2v_(0)` in such a way that it always moves along a straight line perpendicular to the banks.
At what time will he reach the other bank
View SolutionA river of width `w` is flowing such that the stream velocity varies with `y` as `v_(R)=v_(0)[1+(sqrt3-1)/wy]`, where `y` is the perpendicular distance from one bank.A boat starts rowing from the bank with constant velocity `v=2v_(0)` in such a way that it always moves along a straight line perpendicular to the banks.
What will be the velocity of the boat along the straight line when he reaches the other bank
View SolutionA man is riding on a flat car travelling with a constant speed of `10m//s`.He wishes to throw a ball through a stationary hoop `15 m` above the height of his hands in such a manner that the ball will move horizontally as it passes through the hoop. He throws the ball with a speed of `12.5 m//s` w.r.t himself.
How many seconds after he release the ball will it pass through the hoop?
View SolutionA man is riding on a flat car travelling with a constant speed of `10m//s`.He wishes to throw a ball through a stationary hoop `15 m` above the height of his hands in such a manner that the ball will move horizontally as it passes through the hoop. He throws the ball with a speed of `12.5 m//s` w.r.t himself.
At what horizontal distance in front of the hoop must he release the ball?
View SolutionA cannon is fixed with a smooth massive trolley car at an angle `theta` as shown in the figure.The trolley car slides from rest down the inclined plane of angle of inclination `beta`.
The muzzle velocity of the shell fired at `t=y_(0)` from the cannon is `u`,such that the shell moves perpendicular to the inclined just after the firing.
The value of `t_(0)` is:
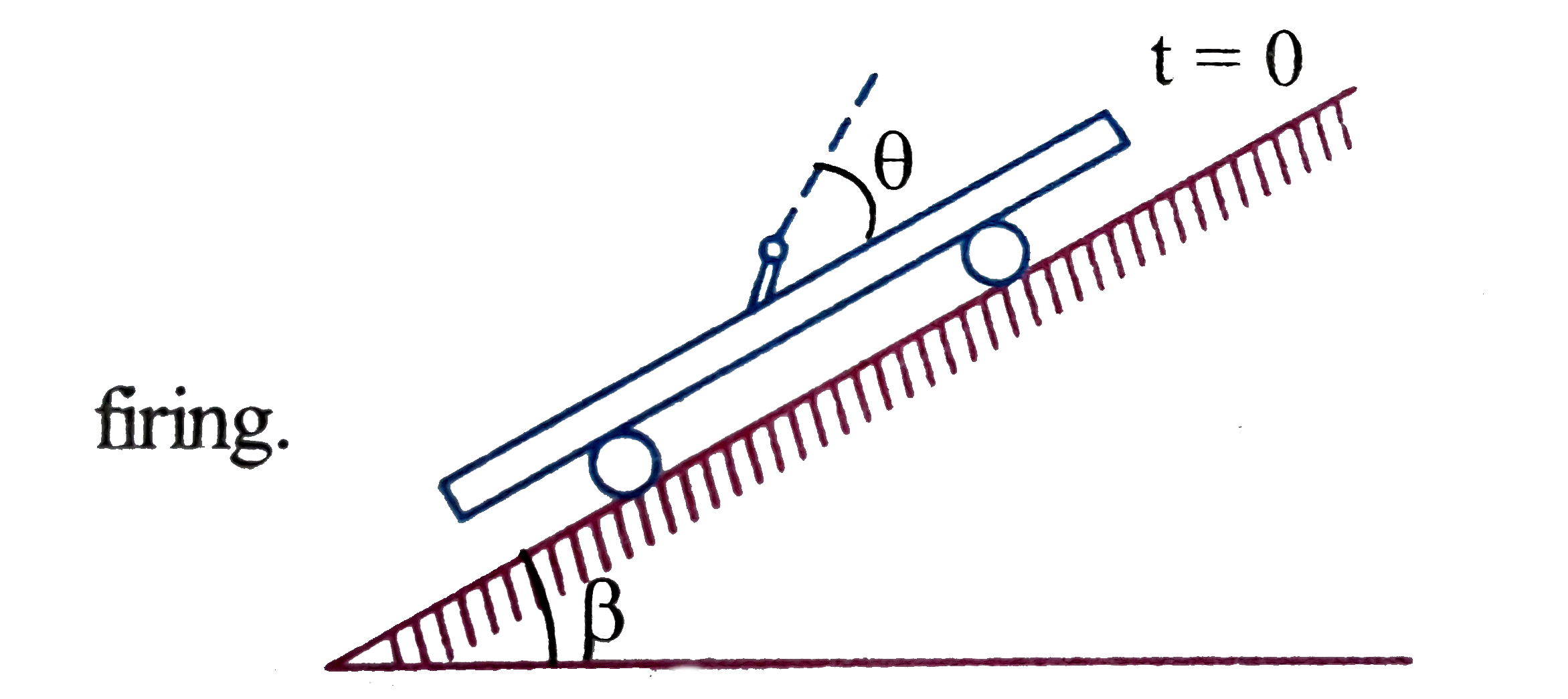
View SolutionA cannon is fixed with a smooth massive trolley car at an angle `theta` as shown in the figure.The trolley car slides from rest down the inclined plane of angle of inclination `beta`.
The muzzle velocity of the shell fired at `t=y_(0)` from the cannon is `u`,such that the shell moves perpendicular to the inclined just after the firing.
The time of flight of the shell is:
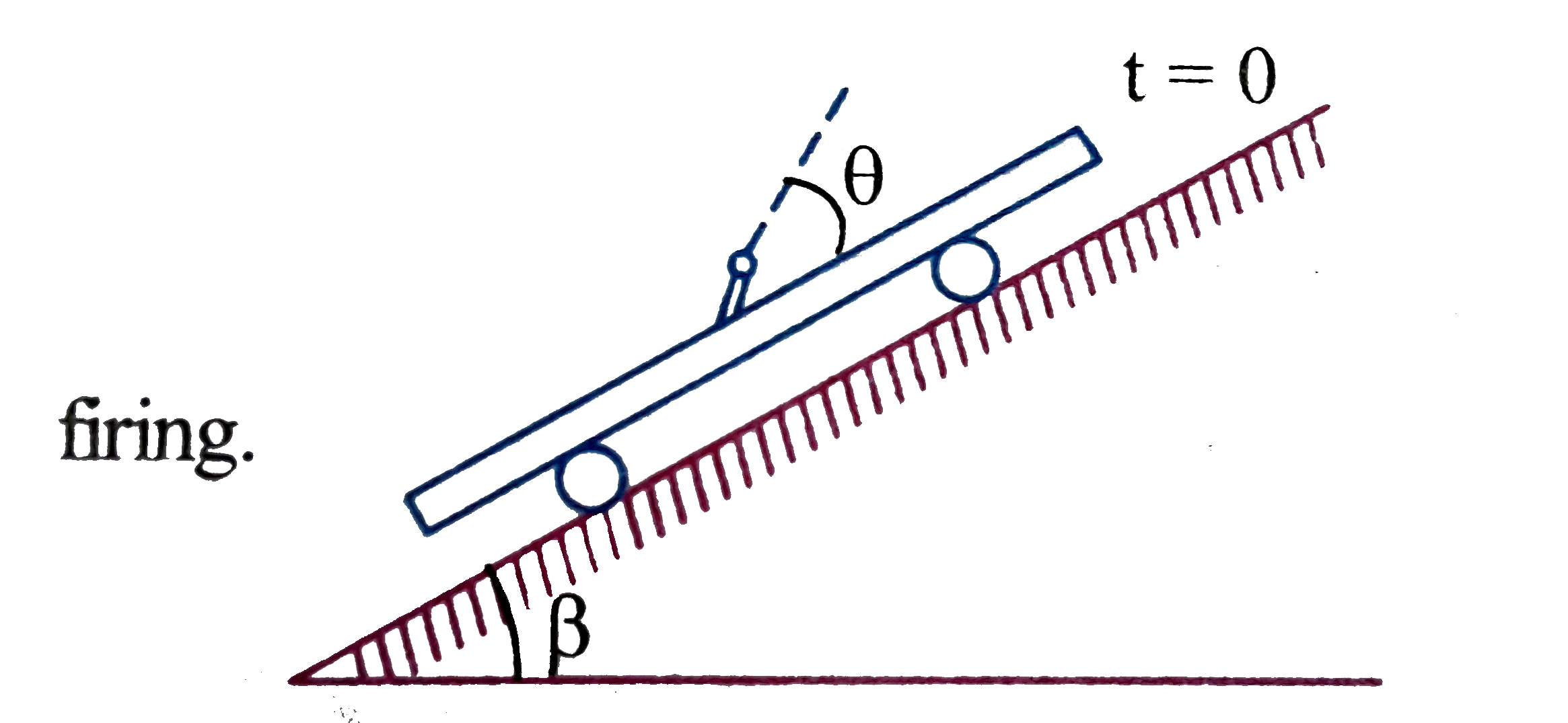
View SolutionA cannon is fixed with a smooth massive trolley car at an angle `theta` as shown in the figure.The trolley car slides from rest down the inclined plane of angle of inclination `beta`.
The muzzle velocity of the shell fired at `t=y_(0)` from the cannon is `u`,such that the shell moves perpendicular to the inclined just after the firing.
the difference in range of the shell relative to the trolley car and ground is:
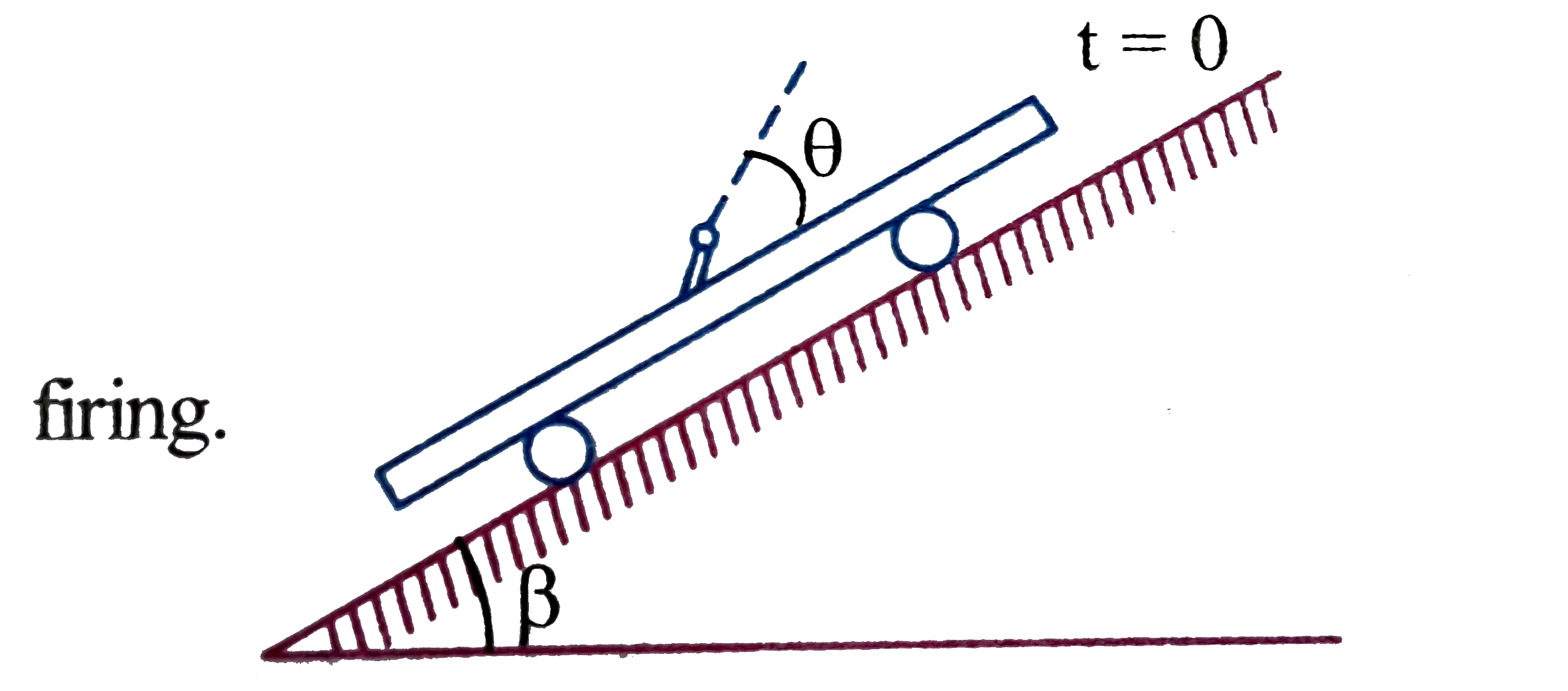
View SolutionA cannon is fixed with a smooth massive trolley car at an angle `theta` as shown in the figure.The trolley car slides from rest down the inclined plane of angle of inclination `beta`.
The muzzle velocity of the shell fired at `t=y_(0)` from the cannon is `u`,such that the shell moves perpendicular to the inclined just after the firing.
after what time should the shell be fired such that it will go vertically up?
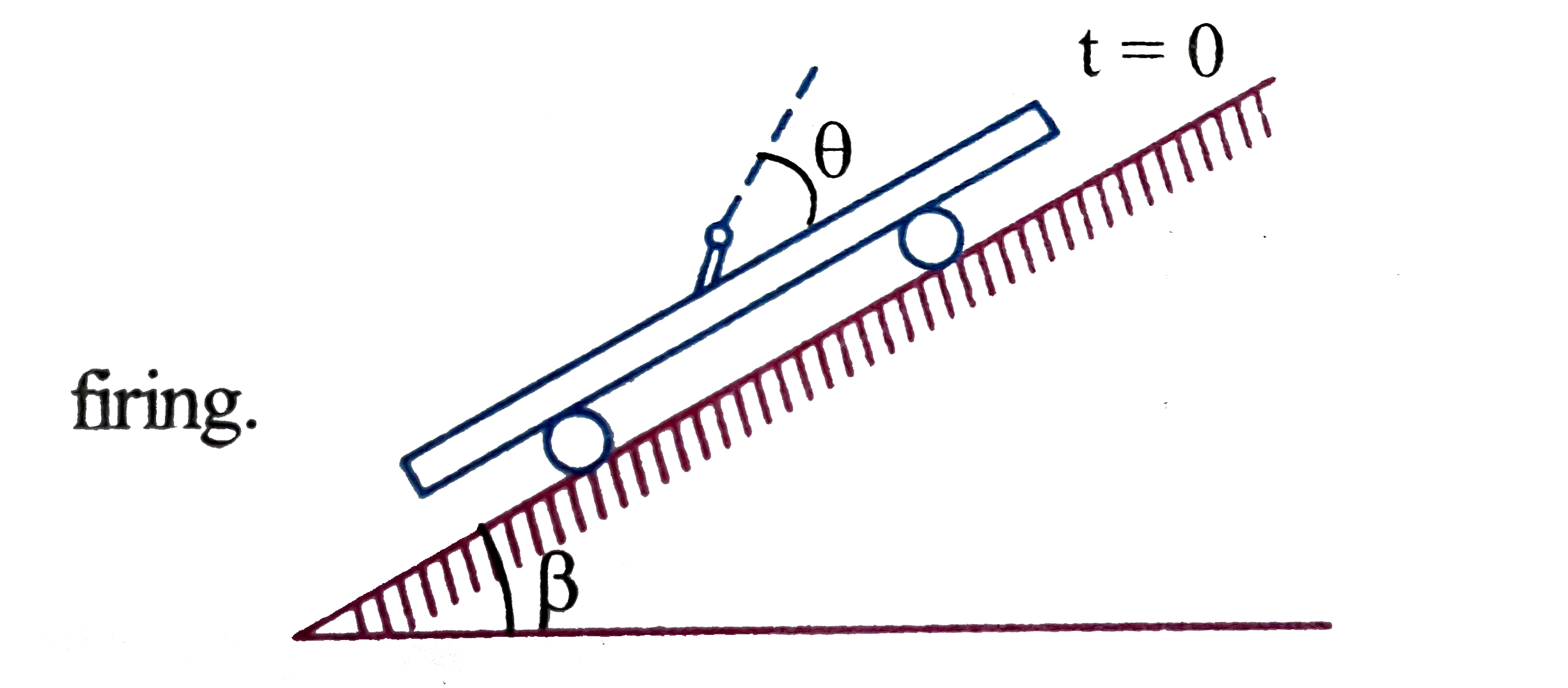
View SolutionWhen we analyse the projectile motion from any accelerated frame `O` as `vecr_(o),vecu_(o)` and `veca_(o)` respectively, express the following terms, `vecr_(pO)+veca_(p)-vecr_(O),vecu_(pO)=vecu_(p)-vecu_(O)` and `veca_(pO)=veca_(p)-veca_(O)`
where `P` stands for projectile. Then using the following kinematical equations of the projectile (For constant acceleration) relative to the accelerating frame ,we have `vecS_(pO)=vecu_(pO)t+1/2veca_(pO)t^(2),vecv_(pO)`
`=vecu_(pO)+veca_(pO)t` and `v_(pO)^(2)=u_(pO)^(2)+2veca.vecs_pO`
Using the above expressions, anwer the following
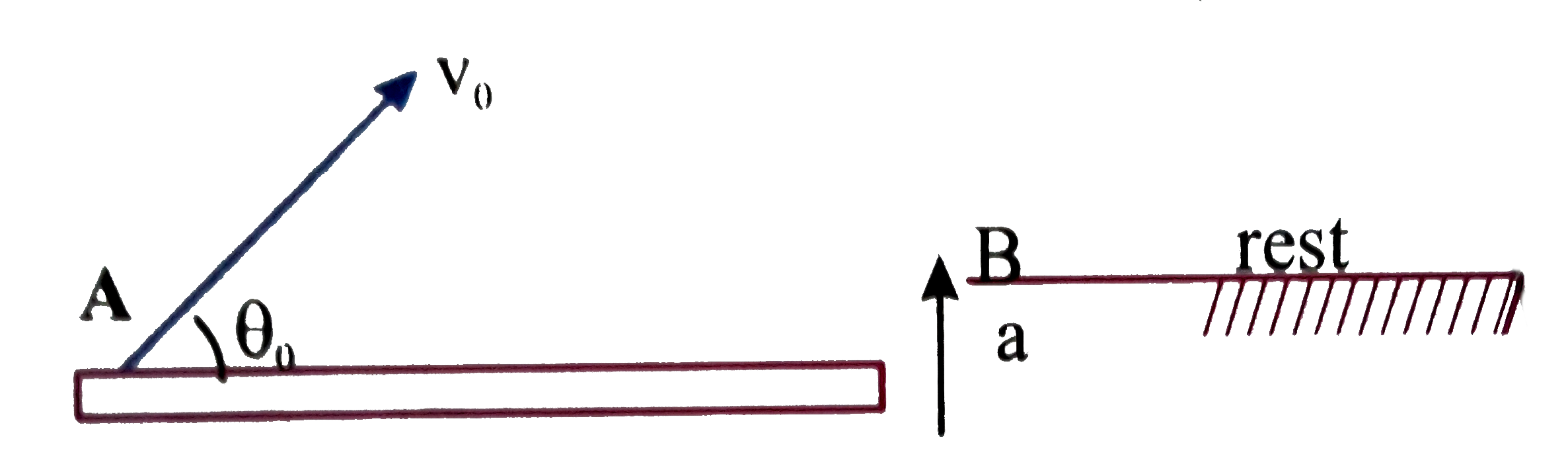
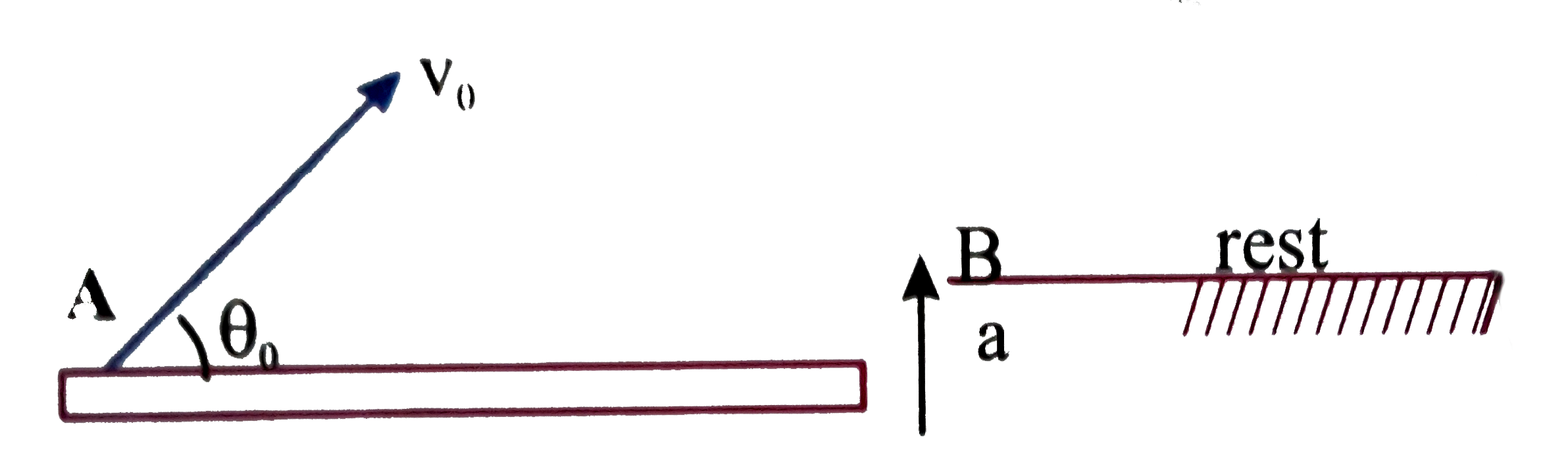
question:A projectile has initial velocity `v_(0)` realtive to the large plate which is moving with a constant upward acceleration a.
Which of the following remains equal for the observers `A` and `B`?
View SolutionWhen we analyse the projectile motion from any accelerated frame `O` as `vecr_(o),vecu_(o)` and `veca_(o)` respectively, express the following terms, `vecr_(pO)+veca_(p)-vecr_(O),vecu_(pO)=vecu_(p)-vecu_(O)` and `veca_(pO)=veca_(p)-veca_(O)`
where `P` stands for projectile. Then using the following kinematical equations of the projectile (For constant acceleration) relative to the accelerating frame ,we have `vecS_(pO)=vecu_(pO)t+1/2veca_(pO)t^(2),vecv_(pO)`
`=vecu_(pO)+veca_(pO)t` and `v_(pO)^(2)=u_(pO)^(2)+2veca.vecs_pO`
Using the above expressions, anwer the following
question:A projectile has initial velocity `v_(0)` realtive to the large plate which is moving with a constant upward acceleration a.
Refering to Q.1, velocity of the projectile relative to `B` ofter some time
View Solution`A` point moves in the plane `xy` according to the law, `x=a sin omegat,y=a(1-cosomegat)` Answer the following question taking `a` and `omega` as positive constant
The distance travelled by the point during the time `T` is
View Solution`A` point moves in the plane `xy` according to the law, `x=a sin omegat,y=a(1-cosomegat)` Answer the following question taking `a` and `omega` as positive constant
The equation of the trajectory of the particle is
View Solution`A` point moves in the plane `xy` according to the law, `x=a sin omegat,y=a(1-cosomegat)` Answer the following question taking `a` and `omega` as positive constant
The magnitude of the velocity of the point as a function of time is
View SolutionAt time `t=0`, the position vector of a particle moving in the `x-y` plane is `5hatj m`.By time `t=0`.`62 sec`,its position vector has become `(5.1hati+0.4 hatj)m`.with the data answer the following questions.
The magnitude of the average velocity during the above time interval.
View SolutionAt time `t=0`, the position vector of a particle moving in the `x-y` plane is `5hatj m`.By time `t=0`.`62 sec`,its position vector has become `(5.1hati+0.4 hatj)m`.with the data answer the following questions.
The angle `theta` made by the average velocity with the positive `x` axis
View SolutionThe position vector of a particle at time `t` is given by `vecr=2t hati+5t hatj+4sin omegat hatk` where `omega` is a constant.Answer the following questions
Velocity vector of the particle is
View SolutionThe position vector of a particle at time `t` is given by `vecr=2t hati+5t hatj+4sin omegat hatk` where `omega` is a constant.Answer the following questions
Velocity vector is perpendicular to …….vector
View SolutionThe position vector of a particle at time `t` is given by `vecr=2t hati+5t hatj+4sin omegat hatk` where `omega` is a constant.Answer the following questions
Acceleration of the particle is
View Solution
Level-VI Passage answer questions and complete solutions for chapter MOTION IN A PLANE of NARAYANA PHYSICS (HINGLISH) of Class 11
All Exercises from NARAYANA Class 11 PHYSICS MOTION IN A PLANE
- C.U.Q
- Example
- Integer
- Level -V Multi answer
- Level -V Passage
- Level -V Single answer
- Level-I (H.W)
- Level-I(C.W)
- Level-II(C.W)
- Level-II(H.W)
- Level-III(C.W)
- Level-Vi Integer
- Level-Vi multiple answer
- Level-VI Passage answer
- Level-Vi single answer
- NCERT BASED QUES. MORE THAN ONE OPTION
- NCERT BASED QUES. PASSAGE
- NCERT BASED QUES. SINGLE ANS.