Complete Solutions to Level-Vi single answer of chapter MOTION IN A PLANE of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | MOTION IN A PLANE |
| NARAYANA | Level-Vi single answer |
| Number Of Questions | 30 |
| Academic Year | 2023-24 |
An open merry go round rotates at an angular velocity `omega`.A person stands in it at a distance `r` from the rotational axis.It is raining and the rain drops falls vertically at a velocity `v_(0)`.How should the person hold an umbrella to prorect himself from the rain in the best way.Angle made by umbrealla with the vertical is
View SolutionA standing man observes rain falling with the velocity of `20 m s^-1` at an angle of `30^@` with the vertical.
(a) Find the velocity with which the man should move so that rain appears to fall vertically to him.
Now if he futher increases his speed, rain again appears to fall at `30^@` with the vertical. Find his new velocity.
View SolutionA person standing on a road has to hold his umbrella at `60^(0)` with the verticcal to keep the rain away. He throws the umbrella an starts running at `20 ms^(-1)`. He finds that rain drops are hitting his head vertically. Find the speed of the rain drops wigh respect to (a) the road (b) the moving person.
View SolutionTwo swimmers leave point A on the bank of the river to reach point B lying right across on the other bank. One of them crosses the river along the straight line AB while the other swims at right angles to the stream and then walks the distance that he has been carried away by the stream to get to point B. What was the velocity u of his walking if both swimmers reached the destination simultaneously? The stream velocity `v_0=2.0 km//hour` and the velocity `v^'` of each swimmer with respect to water equals to `2.5km` per hour.
View SolutionA ball is thrown vertically upward from the 12 m level with an initial velocity of `18 m//s.` At the same instant an open platform elevator passes the 5 m level, moving upward with a constant velocity of `2 m//s.` Determine (`g = 9.8 m//s^2` )
(a) when and where the ball will meet the elevator,
(b) the relative velocity of the ball with respect to the elevator when the ball hits the elevator.
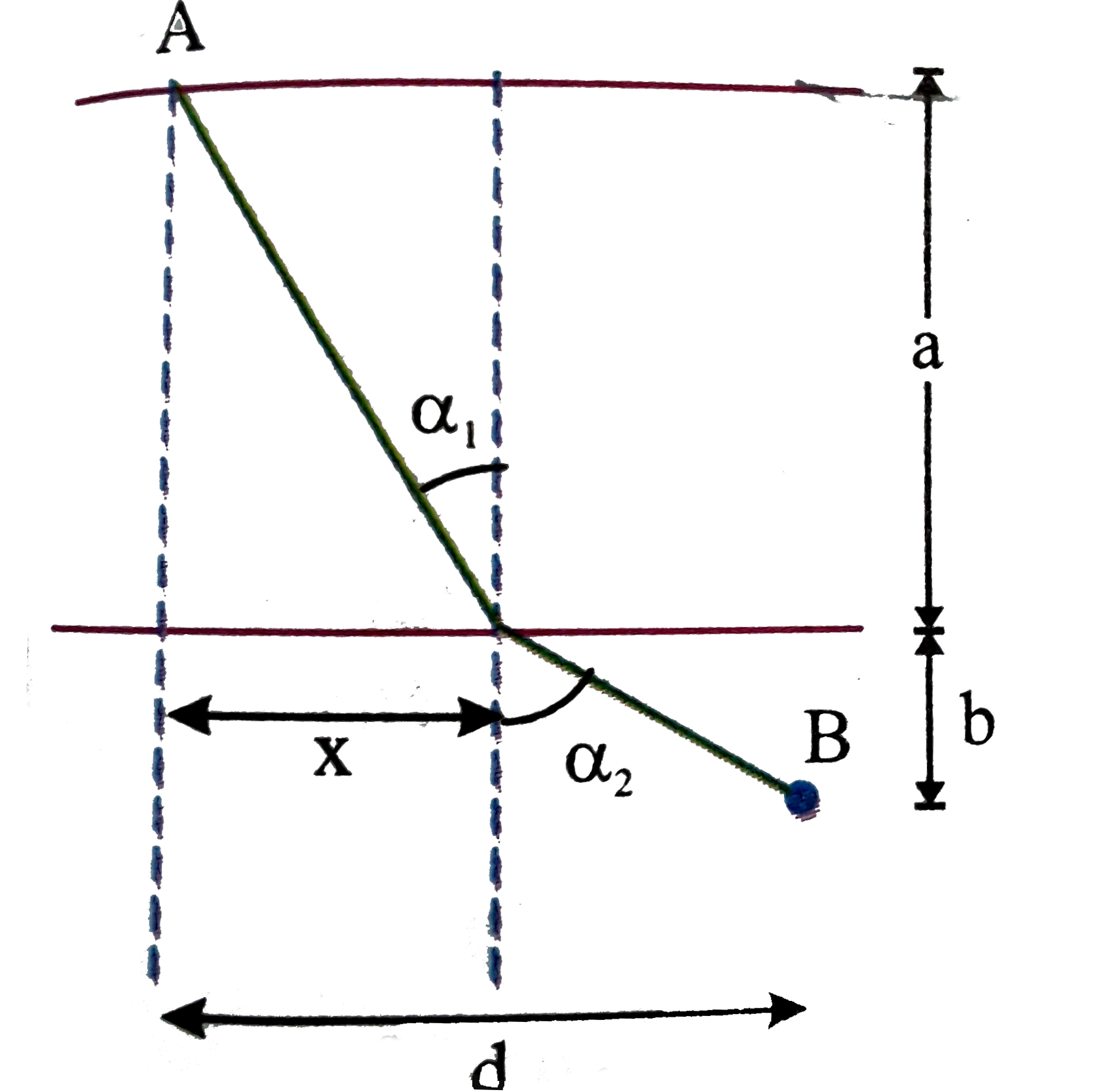
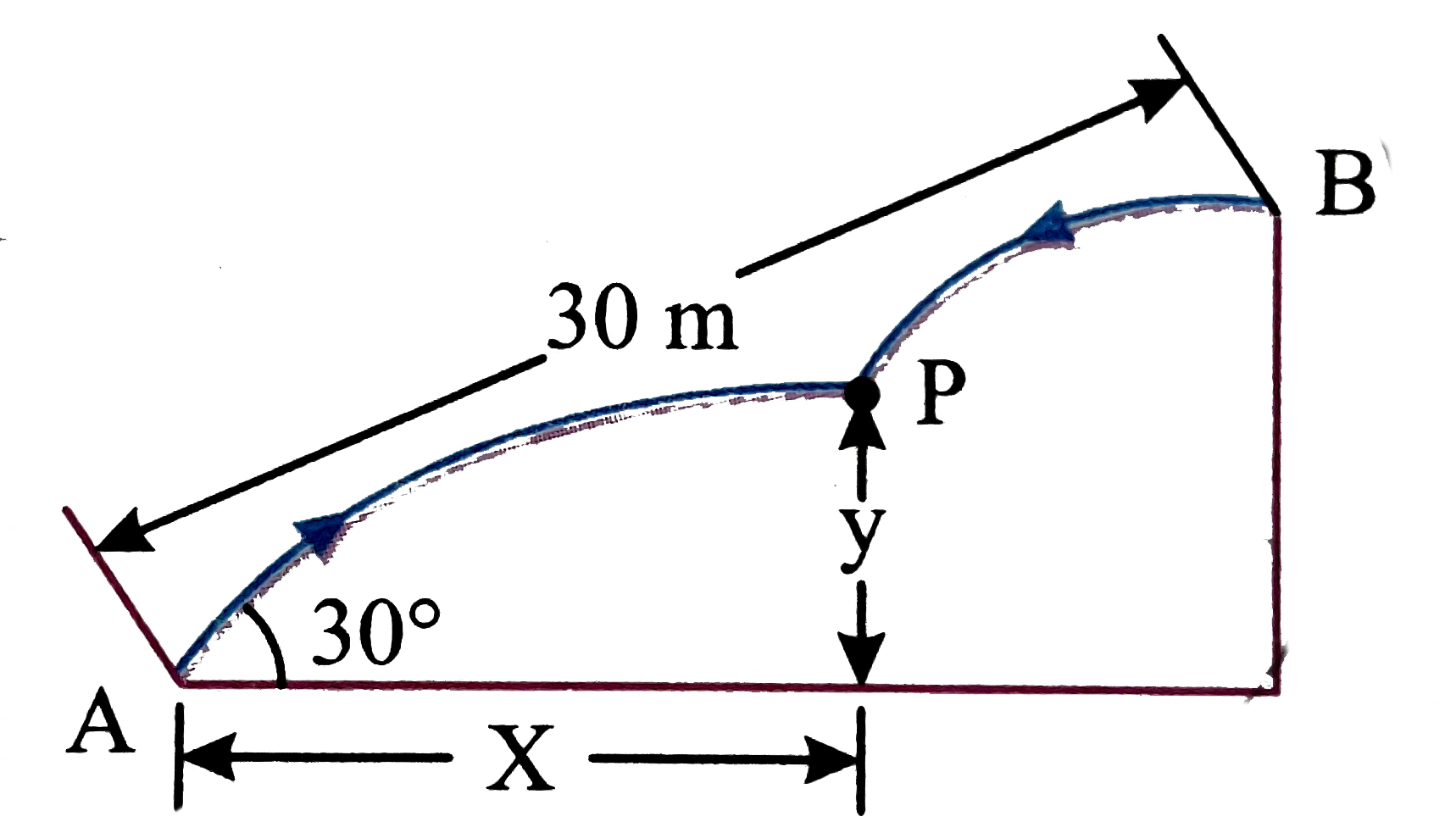
View SolutionFrom a point `A` on bank of a channel with still water a person must get to a point `B` on the opposite bank.All the distances are shown in figure.The person uses a boat to travel across the channel and then walks along the bank of point `B`.The velocity of the boat is `v_(1)` and the velocity of the walking person is `v_(2)`.Prove that the fastest way for the person to get from `A` to `B` is to select the angles `alpha_(1)` and `alpha_(2)` in such a manner that
View SolutionOn morning Joy was walking on a grass-way in a garden.Wind was also blowing in the direction of his walking with speed `u`.He suddenly saw his friend Kim walking on the parallel grass-way at a distance `x` away.Both stopped as they saw each other when they were directly opposite on their ways at a distance `x`.Joy shouted "Hi Kim".Find the time after which Kim would have heard his greeting.Sound speed in still air is `v`.
View SolutionA projectile is fired with velocity `v_(0)` from a gun adjusted for a maximum range.It passes through two points `P` and `Q` whose heights above the horizontal are `h` each.The separation of the two points is
View SolutionA shot is fired with a velocity `u` at a very high vertical wall whose distance from the point of projection is `x`.The greatest height above the level of the point of projection at which the bullet can hit the wall is.
View SolutionA stone is projected from the ground in such a direction so as to hit a bird on the top of a telegraph post of height h and attains the maximum height of 2h above the ground. If at the insatant of projection, the bird were to fly away horizontally with a uniform speed, find the ratio between the horizontal velocity of bird and the horizontal component of velocity of stone, if the stone hits the bird while descending.
View SolutionThe benches of a gallery in a cricket stadium are 1 m wide and 1 m high. A batsman strikes the ball at a levl one metre above the ground and hits a mammoth sixer. The ball starts at 35 m/s at an angle of `53^0` with the horizontal. The benches are perpendicular to the plane of motion and the first bench is 110 m from the batsman.. On which benchk will theball hit?
View SolutionIf `R` is the horizontal range for `theta` inclination and `h` is the maximum height reached by the projectile, Then maximum range is
View SolutionThe acceleration of gravity can be measured by projecting a body upward and measuring the time it takes to pass two given points in both directions.Show that if the time the body takes to pass a horizontal line `a` in both directions is `t_(A)` anytime to go by a second line `B` in both direction is `t_(B)`, then assuming that the acceleration is constant, its magnitude is `g=` (where `h` is the height of the line `B` above line `A`.)
View SolutionA particle is released from a certain height `H = 400m`. Due to the wind, the particle gathers the horizontal velocity component `v_x = ay "where a "= (sqrt5)s^(-1)` and y is the vertical displacement of the particle from the point of release, then find
(a) the horizontal drift of the particle when it strikes the ground ,
(b) the speed with which particle strikes the ground.
View SolutionA fighter plane enters inside the enemy territory, at time `t=0`, with velocity `upsilon_(o)=250m//s` a moves horizontally with constant acceleration `a=20m//s^(2)` (see figure) An enemy tank at the border, spot the plane and fire shots at an angle `theta=60^(2)` with the horizontal and with velocity `u=600 m//s`.At what altitude `H` of the plane it can be hit by the shot?
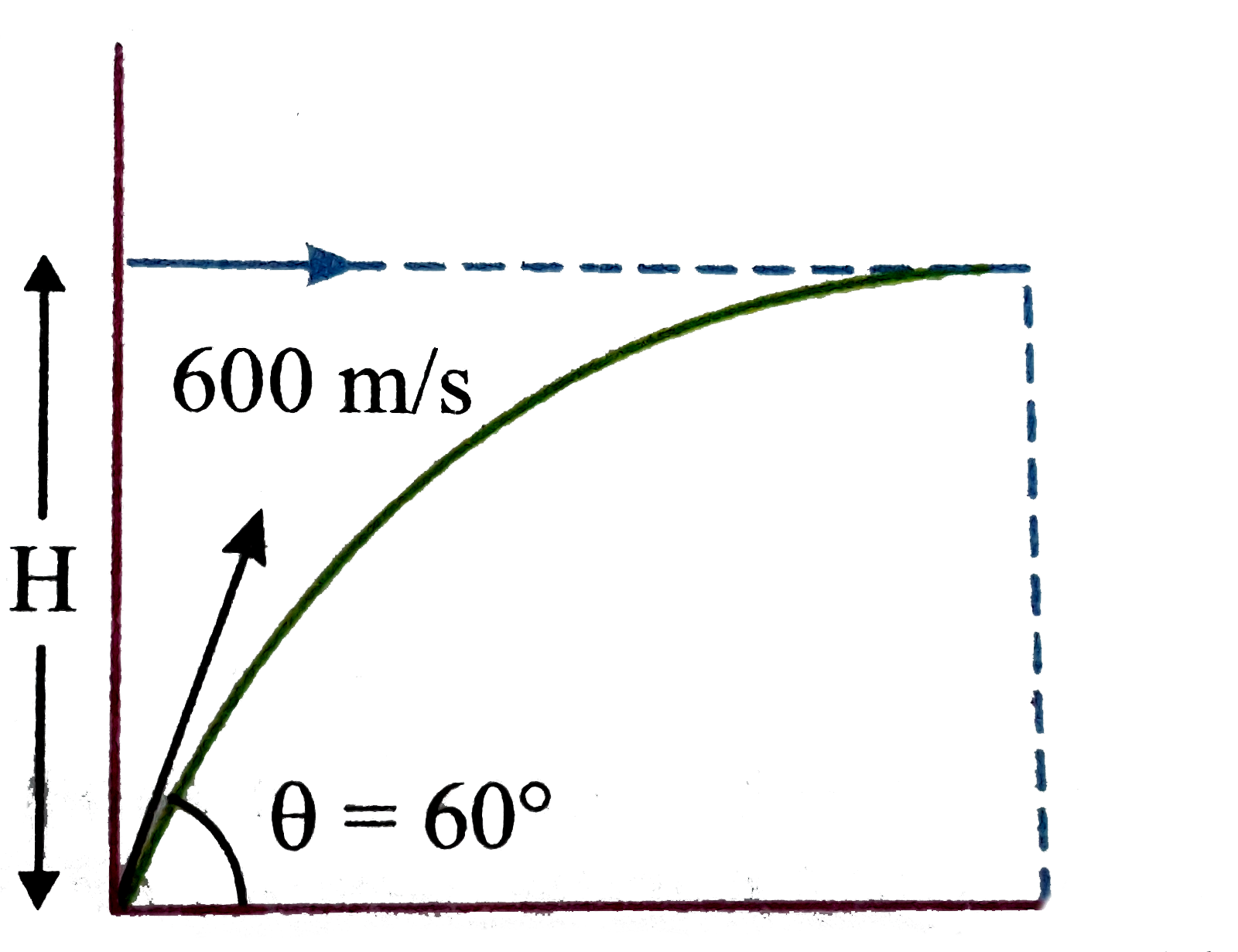
View SolutionA bomber plane moving at a horizontal speed of `20 m//s` releases a bomb at a height of `80 m` above ground as shown. At the same instant a Hunter of negligible height starts running from a point below it, to catch the bomb with speed `10 m//s`. After two seconds he relized that he cannot make it, he stops running and immediately hold his gun and fires in such direction so that just before bomb hits the ground, bullet will hit it. What should be the firing speed of bullet
(Take `g = 10 m//s^(2)`)

View SolutionA body has maximum range `R_1` when projected up the plane. The same body when projected down the inclined plane, it has maximum range `R_2`. Find the maximum horizontal range. Assume equal speed of projection in each case and the body is projected onto the inclined plane in the line of the greatest slope.
View Solution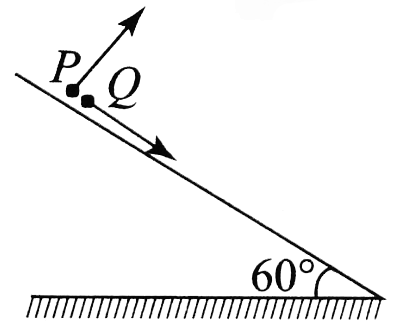
A particle P is projected from a point on the surface of smooth inclined plane (see figure). Simultaneously another particle Q is released on the smooth inclined plane from the same position. P and Q collide after`t=4`. The speed of projection of P is
View Solution
A particle is projected from surface of the inclined plane with speed u and at an angle `theta` with the horizontal. After some time the particle collides elastically with the smooth fixed inclined plane for the first time and subsequently moves in vertical direction. Starting from projection, find the time taken by the particle to reach maximum height. (Neglect time of collision).
View SolutionA perfectly elastic particle is projected with a velocity `v` on a vertical plane through the line of greatest slope of an inclined plane of elevation `alpha`.If after striking the plane, the particle rebounds vertically show that it will return to the point of projection at the end of time equal to
View SolutionTwo bodies `A and B` are projected from the same place in same vertical plane with velocities `v_(1) and v_(2)`.Form a long inclined plane as shown Find the ratio of their times of flight.
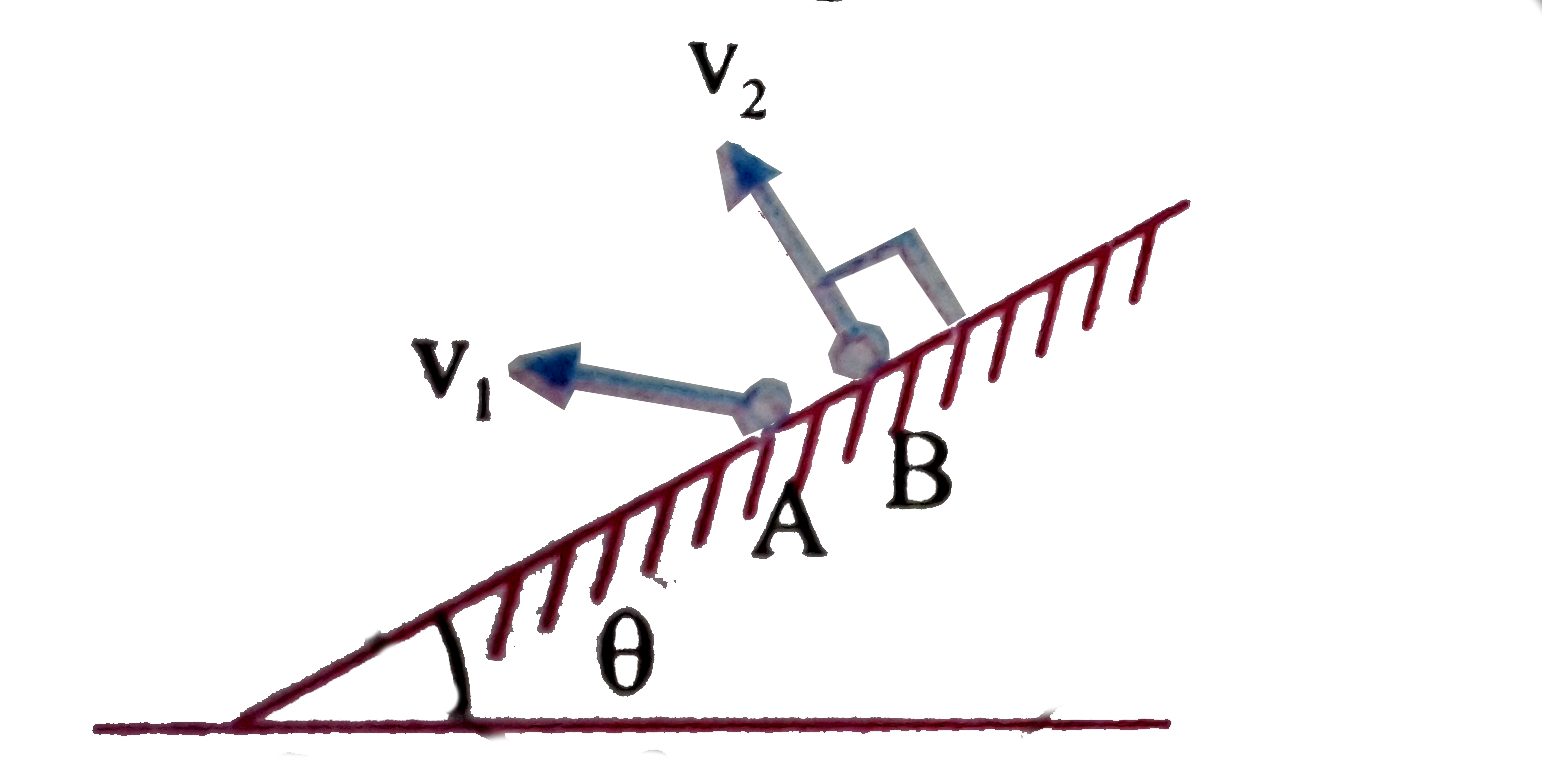
View SolutionA particle `A` is projected from the ground with an initial velocity of `10m//s` at an angle of `60^(@)` with horizontal. From what height should an another particle `B` be projected horizontally with velocity `5m//s` so that both the particles collide in ground at point `C` if both are projected simultaneously `g=10 m//s^(2)`.

View SolutionA smooth square plateform ABCD is moving towards right with a uniform speed v. At what angle `theta` must a particle be projected from A with speed u so that it strikes the point B
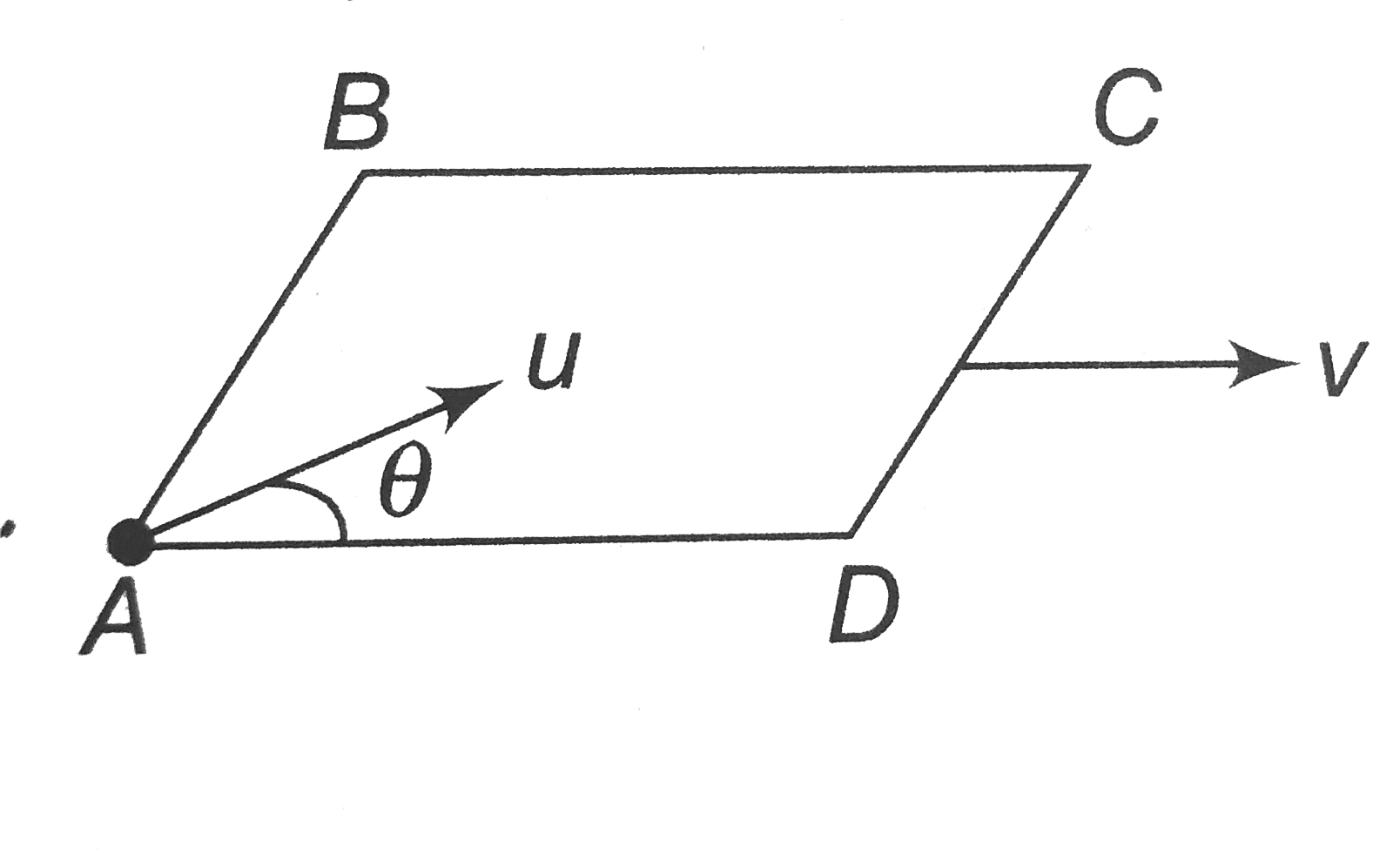
View SolutionTwo particles are projected from the same point on ground simultaneously with speeds and `20m//s and 20//sqrt3` at angles `30^(@)` and `60^(@)` with the horizontal in the same direction.The maximum distance between them till both of them strike the ground is approximately `(g=10m//s^(2))`
View SolutionTwo particles A and B projected simultaneously from a point situated on a horizontal place. The particle A is projected vertically up with a velcity `v_(A)` while the particle B is projected up at an angle `30^(@)` with horizontal with velocity `v_(B)`. After 5s the particles were observed moving mutually perpendicular to each other. The velocity of projection of the particle `v_(A) and v_(B)` respectively are:
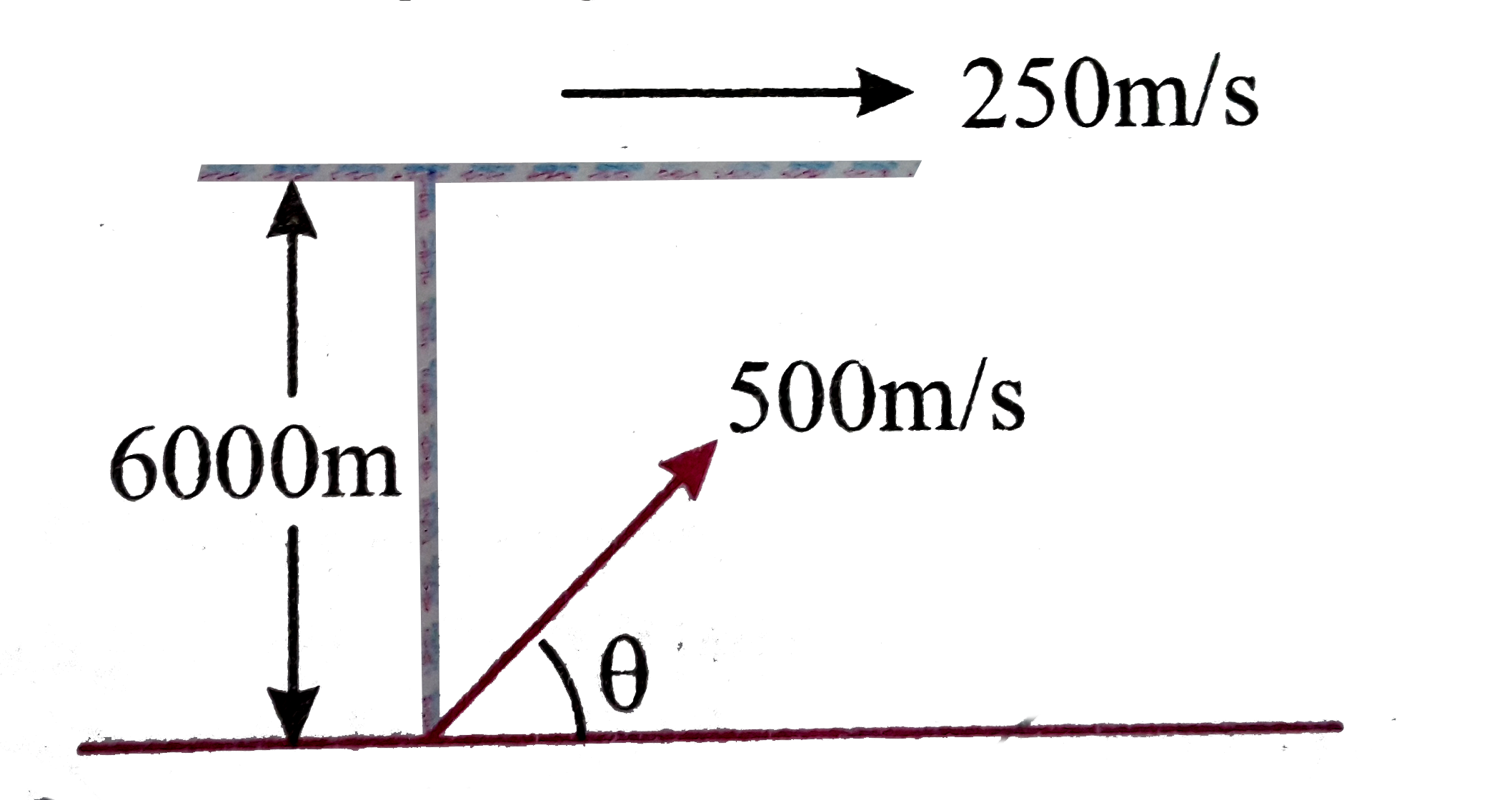
View SolutionAn aircraft moving with a speed of `250 m//s` is at a height of `6000m`,just overhead of an antiaircraft gun.If the muzzle velocity is `500m//s`,the firing angle `theta` should be:
View SolutionA cannon fires successively two shells from the same point with velocity `V_(0)=250m//s`, the first at the angle `theta_(1)=60^(@)` and the second at the angle `theta_(2)=45^(@)` to the horizontal, the azimuth being the same. Neglecting the air drag, find the approximate time interval between firings leading to the collision of the shells `(g=9.8m//s^(2))`
View SolutionA shell is projected from a gun with a muzzle velocity `v`.The gun is fitted with a trolley car at an angle `theta` as shown in the fig. If the trolley car is made to move with constant velocity `v` towards right, find the horizontal range of the shell relative to ground.

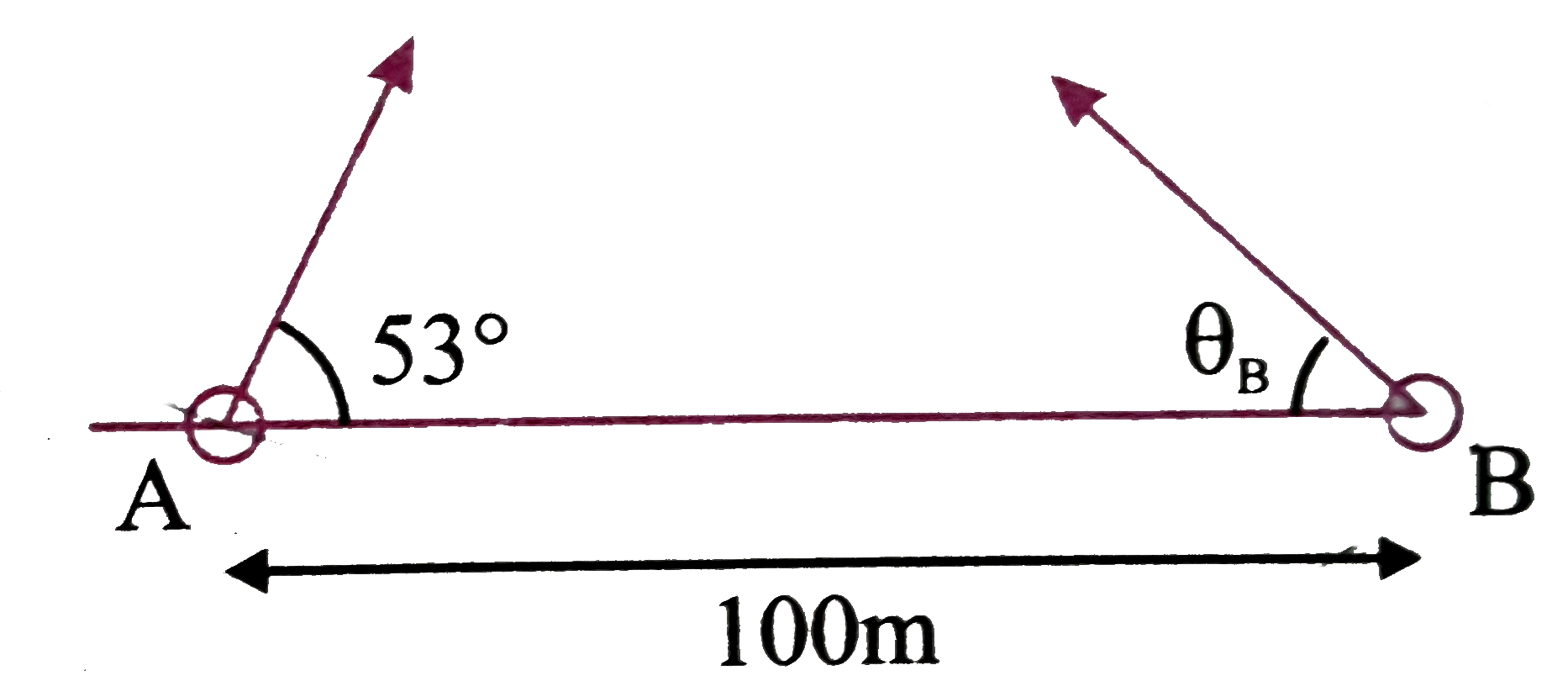
View SolutionTwo guns are projected at each other, one upward at an angle of `30^(@)` and the other at the same of depression, the muzzles being `30m` apart as shown in the figure. If the guns are shots with velocities of `350m//s` upward and `300m//s` downward respectively.where the bullets may meet.
View SolutionTwo particles `A` and `B` are projected in same vertical plane as shown in figure.Their initial positions `(t=0)`, initial spped and angle of projections are indicated in the diagram.If initial angle of projection `theta_(B)=37^(@)`, what should be initial speed of projection of particle `B`, so that it hits particle `A`.`U_(A)=60m//s`
View Solution
Level-Vi single answer questions and complete solutions for chapter MOTION IN A PLANE of NARAYANA PHYSICS (HINGLISH) of Class 11
All Exercises from NARAYANA Class 11 PHYSICS MOTION IN A PLANE
- C.U.Q
- Example
- Integer
- Level -V Multi answer
- Level -V Passage
- Level -V Single answer
- Level-I (H.W)
- Level-I(C.W)
- Level-II(C.W)
- Level-II(H.W)
- Level-III(C.W)
- Level-Vi Integer
- Level-Vi multiple answer
- Level-VI Passage answer
- Level-Vi single answer
- NCERT BASED QUES. MORE THAN ONE OPTION
- NCERT BASED QUES. PASSAGE
- NCERT BASED QUES. SINGLE ANS.