Complete Solutions to Illustration of chapter LAW OF MOTION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | LAW OF MOTION |
| NARAYANA | Illustration |
| Number Of Questions | 86 |
| Academic Year | 2023-24 |
A bomb moving with velocity `(40i+50j-25k)m//s` explodes into two pieces of mass ratio 1:4. After explosion the smaller piece moves away with velocity `(200i +70j +15k)m//s`. The velocity of larger piece after explosion is .
View SolutionA particle of mass 4 m explodes into three pieces of masses, m, m and 2 m. The equal masses move along X-axis and Y-axis with velocities 4` ms^(-1)and 6 ms^(-1) ` respectively. The magnitude of the velocity of the heavier mass is
View SolutionA rifle of 20 kg mass can fire 4 bullets/s. The mass of each bullet is `35xx10^(-3)kg` and its final velocity is `400ms^(-1)`. Then, what force must be applied on the rifle so that it does not move backwards while firing the bullets
View SolutionAll surfaces are smooth Find the horizontal displacements of the block and the wedge when the block slides down from top to bottom
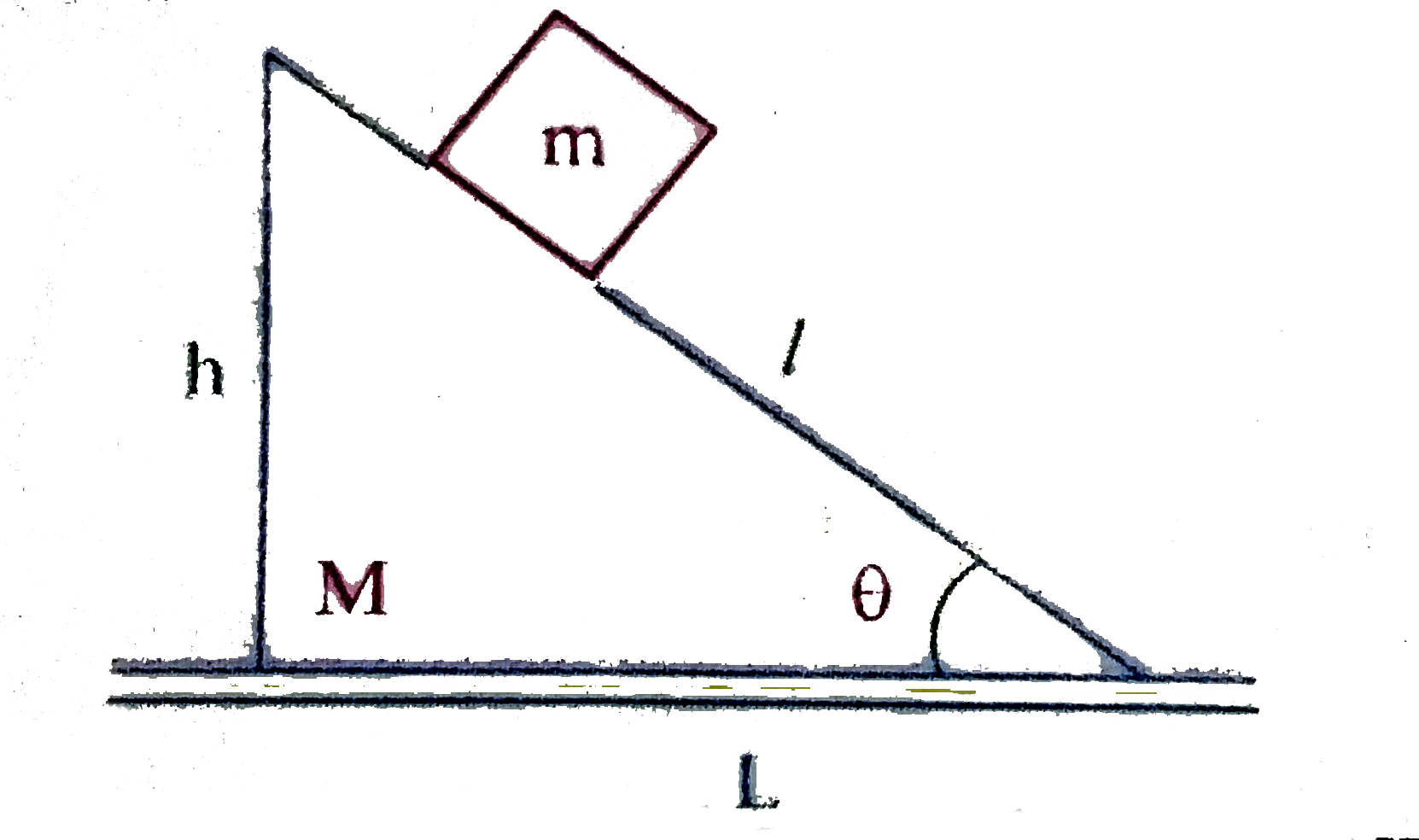 .
.
View SolutionAction and reaction forces acts on
View SolutionA particle of mass 2 is projected at an angle `45^(@)` with horizontal with a velocity of `20sqrt2m//s`. After 1 sec, explosion takes place and the particle is broken into two equal pieces. As a result of explosion one part comes to rest. The maximum height attained by the other part from the ground is `(g=10m//s^(2))`
View SolutionA force produces an acceleration `16m^(-2)` in a mass `0.5 kg` and an acceleration `4ms^(-2)` in an unknown mass when appied separately If both the masses are tied together what will be the acceleration under same force ? .
View SolutionWhen forces `F_(1)` , `F_(2)` , `F_(3)` are acting on a particle of mass m such that `F_(2)` and `F_(3)` are mutually prependicular, then the particle remains stationary. If the force `F_(1)` is now rejmoved then the acceleration of the particle is
View SolutionA very flexible chain of length `L` and mass `M` is vertically suspended with its lower end just touching the table. If it is released so that each link strickes the table and comes to rest. What force the chain will exert on the table at the moment 'y' part of length falls on the table ? .
View SolutionA body of mass `8kg` is moved by a force `F = (3x)N`, where x is the disatance covered Initial position is `x =2m` and final position is `x =10m` If initially the body is at rest find the final speed .
View SolutionAction and reaction forces are
View SolutionA particle is at rest at `x=a`. A force `vecF=(b)/(x^(2))veci` begins to act on the particle. The particle starts its motion, towards the origin, along X - axis. Find the velocity of the particle, when it reaches a distance x from the origin.
View SolutionA particle of mass m is at rest at the origin at time `t=0`. It is subjected to a force `F(t)=F_(0)e^(-bt)` in the X - direction. Its speed V(t) is depicted by which of the following curves.
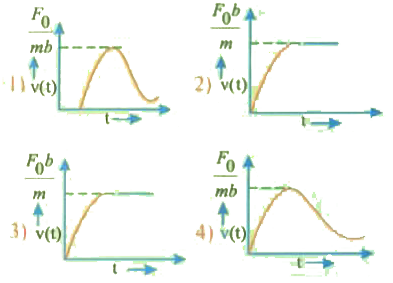
View SolutionA liquid of density `rho` flows along a horizontal pipe of uniform cross - section A with a velocity v through a right angled bend as shown in Fig. What force has to be exerted at the bend to hold the pipe in equilibrium ?
View SolutionA flat plate moves normally with a speed `v_(1)` towards a horizontal jet of water of uniform area of cross section. The jet discharges water at the rate of volume V per second at a speed of `v_(2)`. The density of water is `rho`. Assume that water splashes along the surface of the plate at right angles to the original motion. The magnitude of the force acting on the plate due to the jet is
View SolutionA gardener is watering plants at the rate 0.1 litre/sec using a pipe of cross - sectional area `1cm^(2)`. What additional force he has to exert if he desires to increase the rate of watering two times?
View SolutionA ball falling with velocity `vec(v_(i))=(-0.65hati-0.35hatj)ms^(-1)` is subjected toa net impulse `vecI=(0.6hati+0.18 hatj)Ns`. If the ball has a mass of 0.275 kg, calculate its velocity immediately following the impulse.
View SolutionA spherical mirror, whose reflecting surface is curved inwards, that is,faces towards the centre of the sphere, is called a
View SolutionImage formed by a plane mirror is always____and____
View SolutionA ball hits a floor normally with velocity u and return with same speed. If the ball is in contact with the floor for 0.1 sec, find the average impulsive force acting on the ball. Take the mass of the ball m = 200 gm and `u=2m//s.`
View SolutionFind the impulse due to the force `vecF=ahati+bthatj`, where `a=2N` and `b=4Ns^(-1)` if this force acts from `t_(i)" to "t_(f)=0.3s`
View SolutionA liquid of density `rho` is flowing inside a pipe of cross - sectional area A. The pipe is bent in the shape of a right angle as shown. What force should be exerted on the pipe at the corner to keep it fixed in the two cases shown?
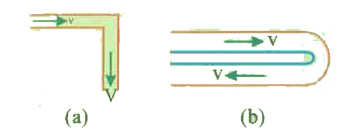
View SolutionA mass of 3kg is suspended by a rope of length 2m from the ceiling. A force of 40 N in the horizonal direction is applied at midpoint P of the rope as shown. What is the angle the rope makes with the vertical in equilibrium and the tension in part of string attached to the ceiling? (Neglect the mass of the rope, `g=10m//s^(2)`)
View SolutionA mass `M` is suspended by a weightless string The horizontal force required to hold the mass `60^(@)` with the vertical is .
View SolutionA chain of mass 'm' is attached at two points `A` and `B` of two fixed walls as shown in the Find the tension in the chain near the walls at point `A` and at the mid point `C`
 .
.
View SolutionThree blocks connected together by strings are pulled along a horizontal surface by applying a force `F. If F =36N` What is the tension `T_(2)` ?.
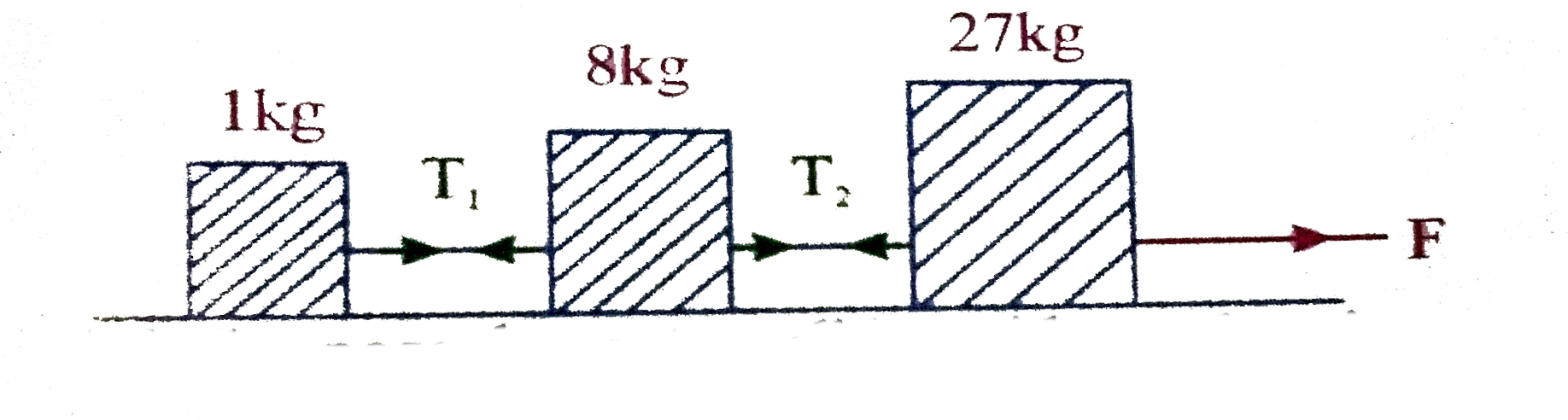 .
.
View SolutionThe maximum tension a rope can withstand is 60 kg - wt. The ratio of maximum acceleration with which two boys of masses 20kg and 30kg can climb up the rope at the same time is
View SolutionThree equal weights of mass m each are hanging on a string passing over a fixed pulley as shown in fig. The tensions in the string connecting weights A to B and B to C will respectively be -
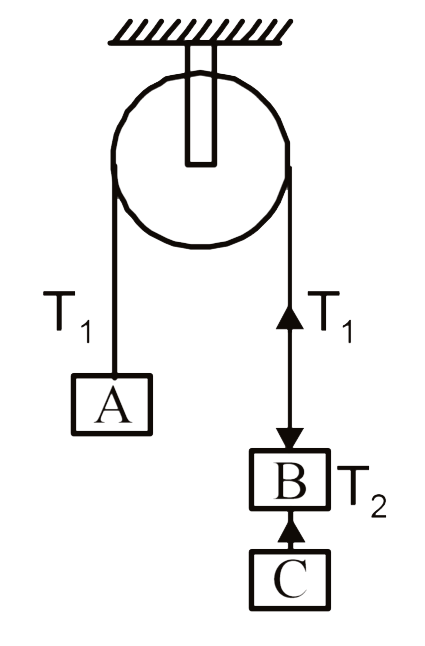
View SolutionA man of mass 60 kg is standing on a weighing machine kept in a box of mass 30 kg as shown in the diagram. If the man manages to keep the box stationary, find the reading of the weighing machine.
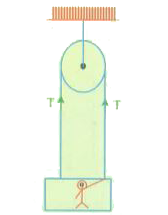
View SolutionBy what acceleration the boy must go up so that 100 kg block remains stationary on the wedge. The wedge is fixed and is smooth.`(g=10m//s^(2))`
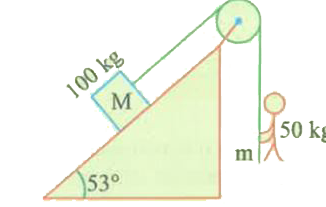
View SolutionThe system as shown in fig is released from rest. Calculate the tension in the strings and force exerted by the strings on the pulley. Assuming pulleys and strings are massless
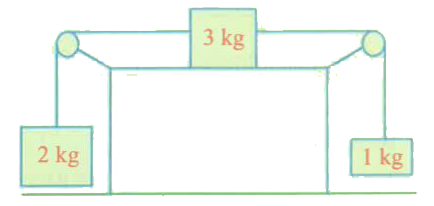
View SolutionIn the adjacent fig, masses of A, B and C are 1 kg, 3kg and 2kg respectively. Find a) the acceleration of the system b) tension in the string `(g=10m//s^(2))`
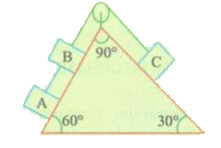
View SolutionA rod of length 'l' is inclined at an angle `'theta'` with the floor against a smooth vertical wall. If the end A moves instantaneously with velocity `v_(1)`, what is the velocity of end B at the instant when rod makes `'theta'` angle with the horizontal.
View SolutionIn the fig, find the acceleration of mass `m_(2)`
View SolutionIn the fig, find the acceleration of `m_(1) and m_(2)`
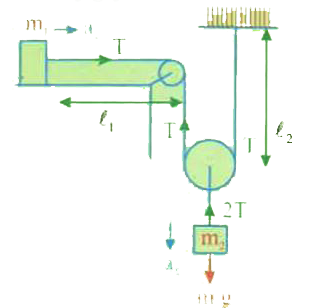
View SolutionA block of weight 100 N is suspended (as shown) with the help of there strings. Find the tensions in the three strings).
View SolutionA block of mass 10 kg is suspended with two strings as shown in the fig. Find the tensions `T_(1) and T_(2)` in the two string.
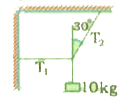
View SolutionFind the magnitude of the horizontal force F required to keep the block of mass m stationary on the smooth inclined plane as shown in the figure.

View SolutionA painter of mass 60 kg stands on a 15 kg platform. A rope attached to the platform and passing over an overhead pulley allows the painter to raise himself and the platform. (i) To get started, he pulls the rope down with force of 400 N. Find the acceleration of the platform as well as that of the painter.

(ii) What force he must exert on the rope so as to attain an upward speed of `1m//s` in 1s ?

(iii) What force should he apply now to maintain the constant speed of `1m//s`?
View SolutionTwo blocks are connected by a smooth inextensible massless string passing over a fixed and smooth pulley. Their masses are `m_(1) and m_(2)` as shown in figure. Find

(a) Acceleration of each block
(b) Tension in the string
(c) Net forec on the pulley
View SolutionTwo masses `m_(1) and m_(2)` are connected by a light string passing over a fixed pulley as shown. The system is suspended in a vertical plane. Find the tension in the string connecting `m_(1) and m_(2)` and acceleration of masses if `m_(1)=3kg` and `m_(2)=5kg`. Also find the tension in the rope connecting the centre of pulley to the ceiling.

View SolutionA body hangs from a spring balance supported from the ceiling of an elevator.
(a) If the elevator has an upward acceleration of `2.45m//s^(2)` and the balance reads 50 N, what is the true weight of the body?
(b) Under what circumstances will the balance read 30 N?
(c) What will be the reading in the balance if the cable of the elevator breaks ?
View SolutionA mass of `1kg` attached to one end of a string is first lifted up with an acceleration `4.9m//s^(2)` and then lowered with same acceleration What is the ratio of tension in string in two cases .
View SolutionThe apparent weight of a man in a lift is `W_(1)` when lift moves upwards with some acceleration and is `W_(2)` when it is accerating down with same acceleration. Find the true weight of the man and acceleration of lift .
View SolutionA pendulum is hanging from the ceiling of a car having an acceleration `a_(0)` with respect to the road. Find the angle made by the string with vertical at equilibrium. Also find the tension in the string in this position.

View SolutionFor what value of 'a' the block falls freely?

View SolutionA block of mass m is placed on a smooth wedge of incination `theta`. The whole system s acelerated horizontally so tht the block does not slip on the wedge. The force exerted by the wedge on the block has a magnitude
View SolutionTwo fixed frictionless inclined planes making an angle `30^(@) and 60^(@)` with the vertical are shown in the figure. Two blocks A and B are placed on the two planes. What is the relative vertical acceleration of A with respect to B?

View SolutionFor what value of 'a' block slides up the plane with an acceleration 'g' relative to the inclined plane.

View SolutionA solid sphere of mass 2kg rests inside a cube as shown. The cube is moving with velocity `vec(upsilon)=(5thati+2thatj)ms^(-1)` where 't' is in sec and `'upsilon'` is in m/s. What force does sphere exert on cube?

View SolutionA block is placed on an inclined plane moving towards right with an acceleration `a_(0)=g`. The length of the inclined plane is `l_(0)`. All the surface are smooth. Find the time taken by the block to reach from bottom to top.

View SolutionA pendulum of mass m hangs from a support fixed to a trolley. The direction of the string when the trolley rolls up a plane of inclination `alpha` with acceleration `a_(0)` is
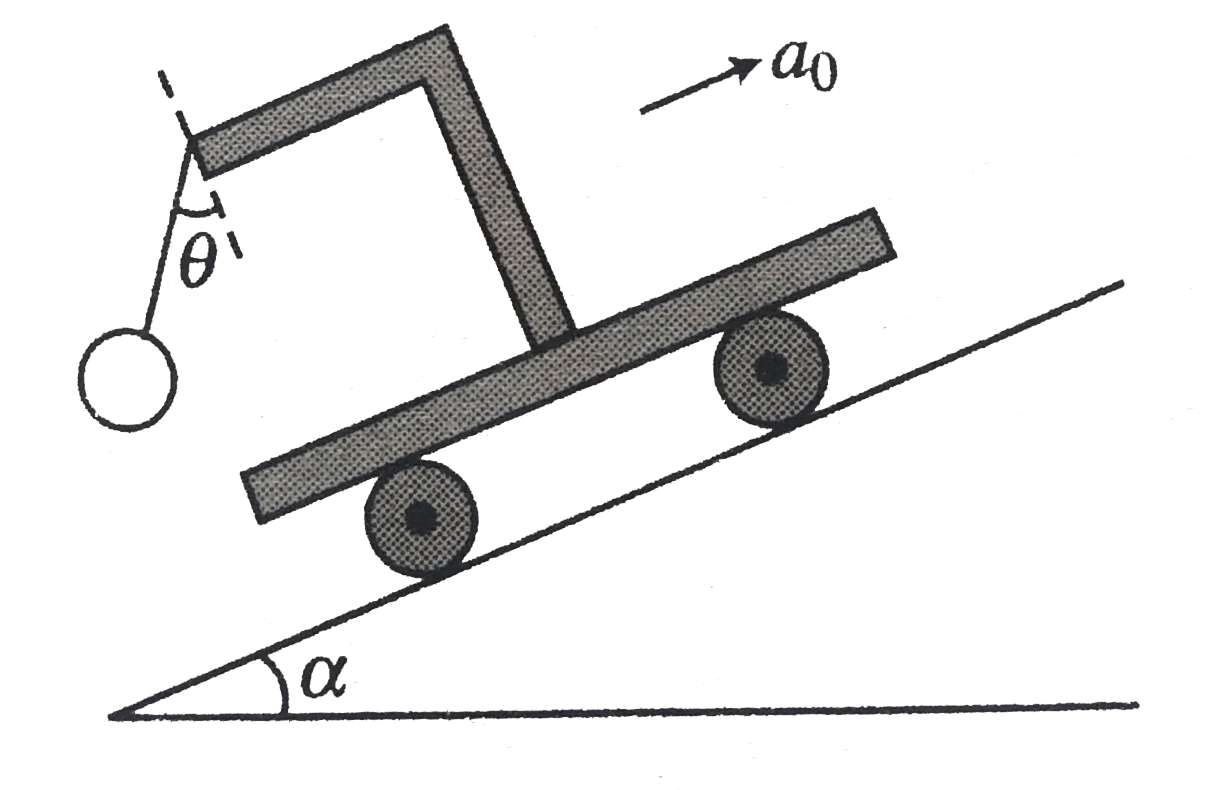
View SolutionA block slides down from to of a smooth inclined plane of elevation `theta` fixed in an elevator going up with an acceleration `a_(0)`. The base of incline has length L. Find the time taken by the block to reach the bottom.

View SolutionAn observer is standing inside a lift moving upwards with an acceleration a. A mass m is lying on the floor of the lift. Find the normal reaction between the block and the floor.
View SolutionA triangular block of mass M rests on a horizontal table. A block of mass m rests on the inclined side. What horizontal acceleration a must M have relative to table to keep m stationary relative to triangular block, assuming frictionless contacts?

View SolutionA man of mass `40kg` is at rest between the walls as shown in the If `'●'` between the man and the walls is `0.8` find the normal reactions exerted by the walls on the man .
View SolutionA `2kg` block is in contact with a vertical wall having coefficient of friction `0.5` between the surfaces. A horizontal force of `40N` is applied on the block at right angles to the wall. Another force of `15N` is applied on the plane of the wall and at right angles to `40N` force. Find the acclecration of the block .
View SolutionA block of mass `4kg` is placed on a rough horizontal plane A time dependent horizontal force `F =kt` acts on the block `(k =2N//s)`. Find the frictional force between the block and the plane at `t =2` seconds and `t =5` seconds `(mu =0.2)` .
View SolutionA block on table shown in figure is just on the edge of slipping. Find the coefficient of static friction between the block and table

View SolutionWhen a car of mass `1000kg` is moving with a veocity of `20ms^(-1)` on a rough horizontal road its engine is switched off. How far does the car move before it comes to rest if the coefficient of kintic friction between the road and tyres of the car is `0.75` ? .
View SolutionA horizontal conveyor belt moves with a constant velocity `V.A` small block is projected with a velocity of `6m//s` on it in a direction opposite to the direction of motion of the belt The block comes to rest relative to the belt in a time `4s. mu =0.3, g =10 .m//s^(2)` Find `V` .
View SolutionThe rear side of a truck is open. A box of 40 kg mass is placed 5m away from the open end as shown in figure. The coefficient of friciton between the box and the surface is 0.15. On a straight road, the truck starts from rest and accelerating with `2m//s^(2)`. At what distance from the starting point does the box fall off the trucks? (Ignore the size of the box).

View SolutionA block of mass `10kg` is pushed by a force `F` on a horizontal rough plane is moving with acceleration `5ms^(-2)` When force is doubled its acceleration becomes `18ms^(-2)` Find the coefficient of friction between the block and rough horizontal plane `(g =10ms^(-2))` .
View SolutionA block of mass `'m'` is placed on a rough surface with a vertical cross section of `y =(x^(3))/(6)`. If the coefficient of friction is `0.5`, the maximum height above the ground at which the block can be placed without slipping is .
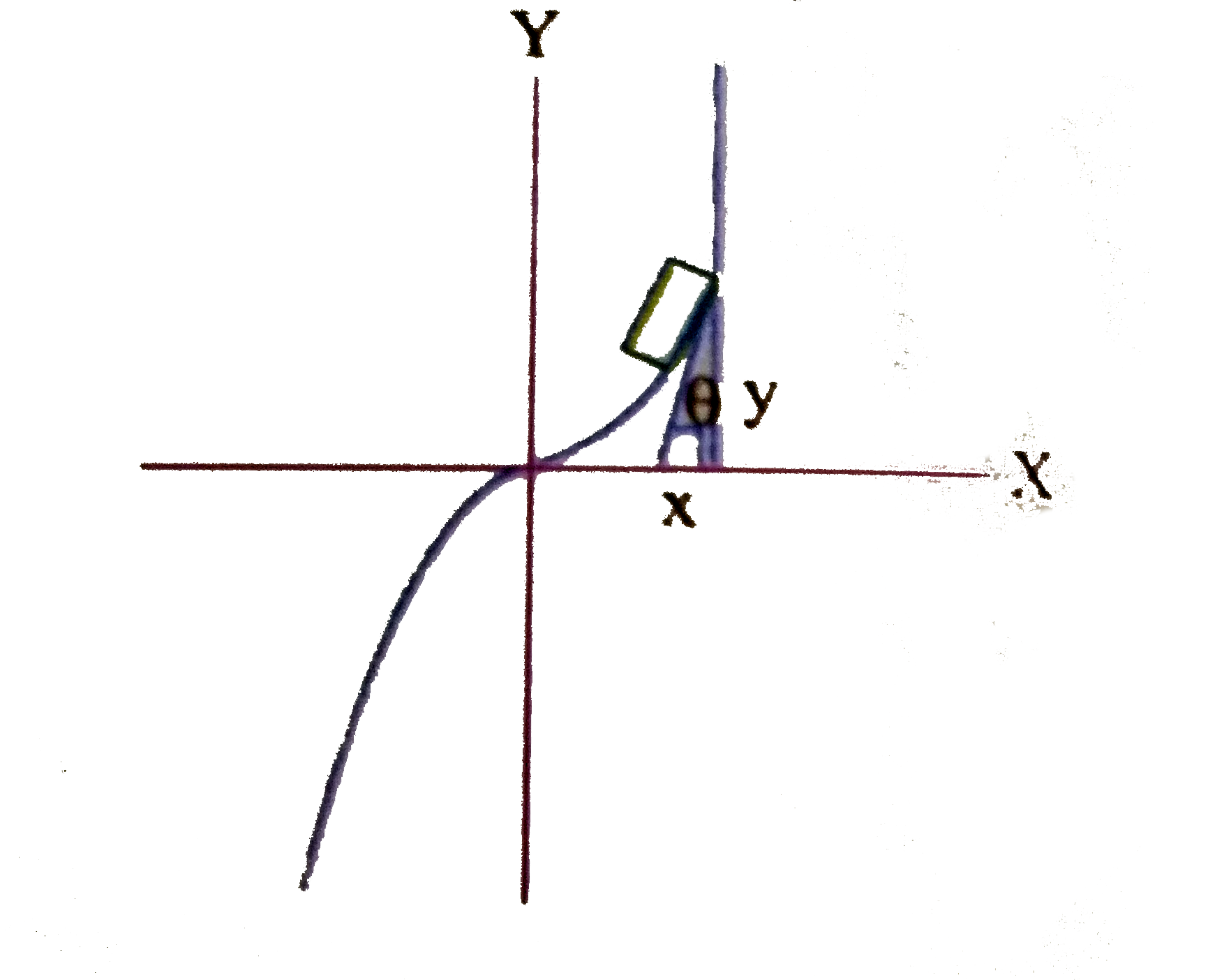
View SolutionA body is moving down a long inclined plane of angle of inclination `'theta`' for which the coefficient of friction varies with distance x as `mu`(x) = kx , where k is a constant . Here x is the distance moved by the body down the plane . The net force on the body will be zero at a distance `x_(0)` is given by ..........
View SolutionA body of mass 'm' slides down a smooth inclined plane having an inclination of `45^(@)` with the horizontal . It takes 2 s to reach the bottom . If the body is placed on a similar plane having coefficient of friction `0.5` , then what is the time taken for it to reach the bottom ?
View SolutionA blocks of masses 4kg and 2kg are in contact with each other on an inclined plane of inclination `30^(@)` as shown in the figure. The coefficient of friction between the figure. The coefficient of friction between 4kg mass and the inclined plane is 0.3, where as between 2 kg mass and hte plane is 0.2. Find the contact force between the blocks.

View SolutionA `30kg` box has to move up an inclined plane of slope `30^(@)` the horizontal with a unform velocity of `5 ms^(-1)`. If the frictional force retarding the motion is `150N`, the horizontal force required to move the box up is `(g =ms^(-2))` .
View SolutionA body is sliding down an inclined plane having coefficient of friction 0.5. If the normal reaction is twice that of resultant downward force along the inclined plane, then find the angle between the inclined plane and the horizontal .
View SolutionIn the given the wedge is acted upon by a constant horizontal force 'F'. The wedge is moving on a smooth horizontal surface A ball of mas 'm' is at rest relative to the wedge The ratio of forces exerted on 'm' by the wedge when 'F' is acting and 'F' is withdrawn assuming no friction between the edge and the ball is equal to .
View SolutionA block of mass 4kg is placed on another block of mass 5kg, and the block B rests on a smooth horizontal table, for sliding the block A on B, a horizontal force 12N is required to be applied on it. How much maximum horizontal force can be applied on 'B' so that both A and B move together? Also find out the acceleration produced by this force.

View SolutionTwo blocks of masses 'm' and 'M' are arranged as shown in the figure. The coefficient of friction between the two blocks is `'mu'`, where as between the lower block and the horizontal surface is zero. Find the force 'F' to be applied on the upper block, for the system to be under equilibrium?

View SolutionTwo cars of masses `m_(1)` and `m_(2)` are moving in circles of raddii `r_(1)` and `r_(2)` respectively. Their speeds are such that they make complete circle in the same time t The ratio of their centripetal acceleration is .
View SolutionA car is driven round a curved path of radius `18m` without the danger of skidding The coefficeient of friction between the tyres of the car and the surface of the curved path is 0.2 What is the maximum speed in kmph of the car for safe driving ? `(g =10 ms^(-2))` .
View SolutionA point P moves in a counter clock wise direction on a circular path as shown in fig. The movement of 'P' is such that it sweeps out a length `S=t^(3)+5`, where 'S' is in metres and 't' is in seconds. The radius of the path is 20m. The acceleration of 'P' when `t=2s` is nearly.

View SolutionA turn of radius `20 m` is banked for the vehicle of mass `200 kg` going at a speed of `10 m//s`. Find the direction and magnitude of frictional force (a) `5 m//s`
(b) `15 m//s`
Assume that friction is sufficient to prevent slipping. `(g=10 m//s^(2))`
View SolutionA block of weight `100N` lying on a horizontal surface just beging to when a horizontal to move when a horinzontal force of `25 N` acts on it Determine the coefficent of static friction
View SolutionA 5kg block slides down a plane inclined at `30^(@)` to the horizontal. Find
(a) The acceleration of the block if the plane is frictionless
(b) The acceleration if the coefficient of kinetic friciton is 0.2
View SolutionA block of mass ` 2 kg` is given velocity `10 m//s` on a rough horizontal surface of friction coefficent`mu = 0.2`. Find the magnitude and the direction of friction force acting on it. After how much time and at what distance it will stop ?
View SolutionA block of mass m is placed on another block of mass M lying on a smooth horizontal surface. The coefficient of static friction between m and M is `mu_(s)`.

What is the maximum vlue of the force F that can be applied to M so that the two blocks remains at rest relative to each other?
View SolutionA block of mass m is placed on another block of mass M lying on a smooth horizontal surface. The coefficient of static friction between m and M is `mu_(s)`. What is the maximum force that can be applied to m so that the blocks remains at rest relative to each other?

View SolutionA block of mass `M=10kg` is initially at rest on an inclined plane and is being pulled upward with a force F parallel to the incline as shown in figure. The coefficient of friction between the block and the incline is `mu=0.3`. Find the magnitude and direction of the friciton force acting on the block if
(i) F = 40 N
(ii) F = 80 N

View SolutionA small block of mass 2kg is placed at rest on a large block of mass 3 kg. The coefficient of friction between the two blocks is `mu=0.3`. The horizontal surface is smooth. A horizontal force F is applied on the lower block. Find the acceleration of each block when

(i) F = 10 N
(ii) F = 20 N
View SolutionA particle moves in a circular path of radius `0.5 m` at a speed that uniformly increases. Find the angular acceleration of particle if its speed changes from `2.0m//s` to `4.0 m//s` in `4.0s`
View SolutionThe speed of a particle moving in a circle of radius `r=2m` varies witht time `t` as `v=t^(2)`, where `t` is in second and `v` in `m//s`. Find the radial, tangential and net acceleration at `t=2s`.
View SolutionA disc rotates at 30 rev/min around a vertical axis. A body lies on the disc at the distance of 20 cm from the axis of rotation. What should be the minimum value of the coefficient of friction between the body and the disc, so that the body will not slide off the disc?

What is the minimum distance from center without rotates at 60 rev/min.
View Solution
Illustration questions and complete solutions for chapter LAW OF MOTION of NARAYANA PHYSICS (HINGLISH) of Class 11
All Exercises from NARAYANA Class 11 PHYSICS LAW OF MOTION
- C.U.Q
- EVALUATE YOURSELF - 1
- EVALUATE YOURSELF - 2
- EVALUATE YOURSELF - 3
- EVALUATE YOURSELF - 4
- EVALUATE YOURSELF - 5
- EXERCISE - I (C.W)(EQUILIBRIUM OF A PARTICLE)
- EXERCISE - I (C.W)(IMPULSE)
- EXERCISE - I (C.W)(LAW OF CONSERVATION OF MOMENTUM)
- EXERCISE - I (C.W)(LAWS OF FRICTION)
- EXERCISE - I (C.W)(MOTION OF A BODY ON THE INCLINED PLANE)
- EXERCISE - I (C.W)(MOTION ON A HORIZONTAL ROUGH SURFACE)
- EXERCISE - I (C.W)(NEWTON.S LAWS OF MOTION)
- EXERCISE - I (C.W)(OBJECTS SUSTENDED BY STRINGS & APPARENT WEIGHT)
- EXERCISE - I (C.W)(PULLING/PUSHING A BODY)
- EXERCISE - I (C.W)(UNIFORM CIRCULAR MOTION)
- EXERCISE - I (H.W)(CIRCULAR MOTION)
- EXERCISE - I (H.W)(IMPULSE)
- EXERCISE - I (H.W)(LAW OF CONSERVATION OF MOMENTUM)
- EXERCISE - I (H.W)(LAWS OF FRICTION)
- EXERCISE - I (H.W)(MOTION OF A BODY ON THE INCLINED PLANE)
- EXERCISE - I (H.W)(MOTION ON A HORIZONTAL ROUGH SURFACE)
- EXERCISE - I (H.W)(NEWTON.S LAWS OF MOTION)
- EXERCISE - I (H.W)(OBJECTS SUSTENDED BY STRINGS & APPARENT WEIGHT)
- EXERCISE - II (C.W)(CIRCULAR MOTION)
- EXERCISE - II (C.W)(EQUILIBRIUM OF A PARTICLE)
- EXERCISE - II (C.W)(IMPULSE)
- EXERCISE - II (C.W)(LAW OF CONSERVATION OF MOMENTUM)
- EXERCISE - II (C.W)(MOTION OF BODY ON THE INCLINED PLANE)
- EXERCISE - II (C.W)(MOTION ON A HORIZONTAL ROUGH SURFACE)
- EXERCISE - II (C.W)(NEWTON.S LAWS OF MOTION)
- EXERCISE - II (C.W)(OBJECTS SUSPENDED BY STRINGS AND APPARENT WEIGHT)
- EXERCISE - II (C.W)(PULLING/PUSHING A BODY)
- EXERCISE - II (H.W)(CIRCULAR MOTION)
- EXERCISE - II (H.W)(MOTION OF A BODY ON THE INCLINED PLANE)
- EXERCISE - II (H.W)(MOTION ON A HORIZONTAL ROUGH SURFACE)
- EXERCISE - II (H.W)(NEWTON.S LAWS OF MOTION)
- EXERCISE - II (H.W)(OBJECTS SUSPENDED BY STRINGS AND APPARENT WEIGHT)
- EXERCISE - II (H.W)(PULLING/PUSHING A BODY)
- EXERCISE - III
- EXERCISE - IV
- Illustration