Complete Solutions to Exercise-I (C.W) of chapter WAVES of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | WAVES |
| NARAYANA | Exercise-I (C.W) |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
Which of the following represents a progressive wave
View SolutionThe equation of progressive wave is `y=0.01sin(100t-x)` where `x,y` are in meter and t in second, then
`(a)` Velocity of wave is `50m//s`
`(b)` Maximum velocity of particle is `1m//s`
`(c )` Wave length of wave is `2pi` meter
View SolutionThe equation `y=A cos^(2) (2pi nt -2 pi (x)/(lambda))` represents a wave with
View SolutionA transverse wave is derscried by the equation `y=y_(0) sin 2 pi (ft - (x)/(lamda))`. The maximum particle velocity is equal to four times the wave velocity if :-
View SolutionTwo simple harmonic are represented by the equation `y_(1)=0.1 sin (100pi+(pi)/3) and y_(2)=0.1 cos pit`.
The phase difference of the velocity of particle 1 with respect to the velocity of particle 2 is.
View SolutionA transverse wave along a string is given by
`y = 2 sin (2pi (3t - x) + (pi)/(4))`
where x and y are in cm and t in second. Find acceleration of a particle located at x = 4 cm at t = 1s.
View SolutionThe frequency of a fork is `500Hz` . Velocity of sound in air is `350ms^(-1)` . The distance through which sound travel by the time the fork makes `125` vibrations is
View SolutionThe velocity of sound waves in air is `330m//s`. For a particluar sound in air, a path difference of `40 cm` is equivalent to a phase difference of `1.6pi`. The frequency of this wave is
View SolutionA wave has a frequency of `120Hz`. Two points at a distance `9m` apart have a phase difference of `1080^(@)`. The velocity of the wave is
View SolutionA source of frequency `500 Hz` emits waves of wavelength `0.2 m`. How long does it take to travel `300 m` ?
View SolutionThe displacement of a wave disturbance propagating in the positive x-direction is given by
`y =(1)/(1 + x^(2))`at `t = 0` and `y =(1)/(1 +(x - 1)^(2))` at `t =2s`
where, `x` and `y` are in meter. The shape of the wave disturbance does not change during the propagation. what is the velocity of the wave?
View SolutionA wave of angular frequency `omega` propagates so that a certain phase of oscillation moves along x-axis, y-axis, z-axis with speeds `c_(1) c_(2)` and `c_(3)` respectively.
View SolutionA travelling wave has the frequency `upsilon` and the particle displacement amplitude `A`. For the wave the particle velocity amplitude is ………………… and the particle acceleration amplitude is ……………………..
View SolutionIf Young's modulus of the material of a rod is Y and density is `rho` then time taken by sound wave to travel `l` length from bottom is
View Solutionv20.1
View SolutionA transverse wave propagating on a stretched string of linear density `3xx10^-4 kg-m^-1` is represented by the equation
`y=0.2sin(1.5x+60t)`
Where x is in metre and t is in second. The tension in the string (in Newton) is
View SolutionThe extension in a string, obeying Hooke's law, is x. The speed of sound in the stretched string is v. If the extension in the string is increased to `1.5 x`, the speed of sound will be :-
View SolutionA uniform rope of length `12m` and mass `6kg` hangs vertically from a rigid support. A block of mass `2kg` is attached to the free end of the rope. A transverse pulse of wavelength `0.06m` is produced at the lower end of the rope. What is the wavelength of the pulse when it reaches the top of the rope?
View SolutionA string of length `l` hangs freely from a rigid support. The time required by a transverse pulse to travel from bottom to half length of the string is
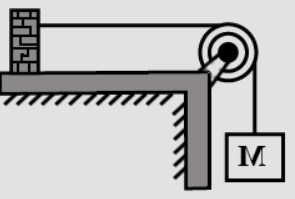
View SolutionA transverse wave is passing through a light string shown in fig.The equation of wave is `y=A sin(wt-kx)` the area of cross-section of string `A` and density is `rho` the hanging mass is
View SolutionThe equation of a wave on a string of linear mass density `0.04 kgm^(-1)` is given by
`y = 0.02(m) sin[2pi((t)/(0.04(s)) -(x)/(0.50(m)))]`.
Then tension in the string is
View SolutionIn (Q. 24.) the tension in string is T and the linear mass density of string is `mu`. The ratio of magnitude of maximum velocity of particle and the magnitude of maximum acceleration is
View SolutionThe speed of sound in air is `332m//s` at NTP. What will be its value in hydrogen at NTP, if density of hydrogen at NTP is `1//16th` that of air ?
View SolutionCalculate the ration of speed of sound in neon to that in water vapours at any temperature. Molecular weight on neon `=2.02xx10^(-2)kg//mol e` and for water vapours, molecular weight is `1.8xx10^(-2)kg//mol e`.
View SolutionThe pressure of air increases by `100mm` of Hg and the temperature decreases by `1^(@)C`. The change in the speed of sound in air at STP is `(V_(0)=333m//s)`
View SolutionThe temperature at which the velocity of sound in oxygen will be same as that of nitrogen at `15^(@) C` is
View SolutionThe ratio of the speed of sound in nitrogen gas to that in helium gas, at 300K is
View SolutionA pressure of `100kPa` causes a decrease in volume water by `5xx10^(-3)` precent. The speed of sound in water is
View SolutionThe speed of sound in hydrogen at STP is `V`. The speed of sound in a mixture containing `3` parts of hydrogen and `2` parts of oxygen at STP will be
View SolutionCalculate the velocity of sound in a mixture of two gases obtained by mixing `m_(1)` and `m_(2)` of them if the velocity of sound in them be `C_(1)` and `C_(2)`. The atomicity of the two gases is the same.
View SolutionTwo waves given by `y_(1)=asinomegat` and `y_(2)=a sin (omegat+pi//2)` reaching at a point superimpose. The resultant amplitude is
View SolutionTwo sound waves are represented by `y_(1)=sinomegat+cosomegat` and `y_(2)=(sqrt(3))/(2)sinomegat+(1)/(2)cosomegat`. The ratio of their amplitude is
View SolutionA standing wave, having `5` nodes and `4` antinodes is formed between two atoms having a distance `1.21Å` between them. The wavelength of the standing wave is
View SolutionA tuning fork of frequency 480 Hz is used to vibrate a sonometer wire having naturl frequency 410 Hz. The wire wil vibrate with frequency
View SolutionWhen the streching force of a wire is increased by `2.5kg`, the frequency of the note emitted is changed in the ratio `3//2`. Calculate the original stretching force
View SolutionIf `f_(1) and f_(2)` be the fundamental frequencies of the two segments into which a stretched string is divided by means of a bridge , then find the original fundamental frequency `f` of the complete string.
View SolutionStationary waves are produced in 10 m long stretched string. If the string vibrates in 5 segments and wave velocity 20 m/s then the frequency is :-
View SolutionThe equation `y=5sin((pix)/(25))cos(450t)` represents the stationary wave in a vibrating sonometer wire, where `x,y` are in cm and t in sec. The distances of `2nd` and `3rd` nodes from one end are (in cm).
View Solutionv20
View SolutionIf the length of a stretched string is shortened by `40 %` and the tension is increased by `44 %`, then the ratio of the final and initial fundamental frequencies is
View SolutionThe fundamental frequency of a stretched string with a weight of `9kg` is `289Hz`. The weight required to produce its octave is
View Solutionin an experiment it was found that string vibrates in n loops when a mass M is placed on the pan. What mass should be placed on the pan to make it vibrate in 2n loops with same frequency ? ( neglect the mass of pan )
View SolutionTransverse waves are generated in two uniform wires `A` and `B` of the same material by attaching their free ends to a vibrating source of frequency `200Hz`. The cross sectiona of A is half that of B while the tension on A is twice that on B. The ratio of wavelengths of transverse waves in A and B is
View SolutionA string is stretched between fixed points separated by `75.0cm`. It is observed to have resonant frequencies of `420 Hz` and `315 Hz`. There are no other resonant frequencies between these two. Then, the lowest resonant frequency for this string is
View SolutionA sound wave with an amplitude of `3cm` starts towards right from origin and gets reflected at a rigid wall after a second. If the velocity of the wave is `340ms^(-1)` and it has a wavelength of `2m`, the equations of incident and reflected waves respectively are :
View SolutionSound signal is sent through a composite tube as shown in the figure. The radius if the semicircular portion of the tube `r`. Speed of sound in air is `v`. The source of sound is capable of giving vaied frequencies in the range of `v_(1)` and `v_(2)` (where `v_(2) gt v_(1)`). If `n` is an integer then frequency for maximum intensity is given by

View SolutionFour simple harmonic vibrations
`y_(1)=8 sin omega t`, `y_(2)= 6 sin (omega t+pi//2)`, `y_(3)=4 sin (omega t+pi)`, `y_(4)=2sin(omegat+3pi//2)`
are susperimposed on each other. The resulting amplitude and phase are respectively.
View SolutionA wave pulse on a string has the dimension shown in figure.
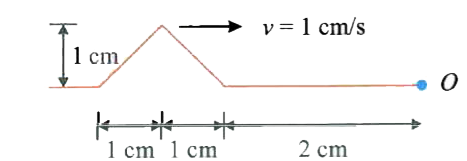
The wave speed is `v=1cm//s`. If point `O` is free end. The shape of wave at time `t=3s` is
View SolutionThe length of a sonometer wire is `90cm` and the stationary wave setup in the wire is represented by an equation `y=6sin((pix)/(30))cos(250t)` where `x` , `y` are in cm and `t` is in second. The number of loops is
View SolutionA sonometer is set on the floor of a lift. When the lift is at rest, the sonometer wire vibrates with fundamental frequency 256 Hz. When the lift goes up with acceleration a `=(9g)/(16)` , the frequency of vibration of the same wire changes to
View SolutionStanding wave produced in a metal rod of length `1m` is represented by the equation `y=10^(-6)sin.(pix)/(2)sin200pit` where `x` is in metre and t is in seconds. The maximum tensile stress at the mid point of the rod is (Young's modulus of material of rod `=10^(12)N//m^(2)`)
View SolutionAn addiditional bridge is kept below a sonometer wire so that it is divided into two segments of lengths in the ratio `2 : 3` and `n_(1)`, `n_(2)` are their respective fundamental frequencies. If the additional bridge is removed then the fundamental frequency of that sonometer wire is n, the ratio of `n,n_(1),n_(2)` is
View SolutionA piano wire `0.5m` long and mass `5gm` is streteched by a tension of `400N` .The number of highest overtone that can be heared by a person is
View SolutionAn iron load of 2Kg is suspended in the air from the free end of a sonometer wire of length 1m.A tuning frok of frequency 256 Hz, is in resonace with `1/sqrt7` times the length of the sonometer wire. If the looad is immersed in water, the length of the wire in metre that will be in resonance with the same tuning fork is (specific gravity of iron=8)
View SolutionThe third overtone of a closed pipe is found to be in unison with the firest overtone of an open pipe. Find the ratio of the lengths of the pipes.
View SolutionTwo closed organ pipes of length 100 cm and 101 cm 16 beats is 20 sec. When each pipe is sounded in its fundamental mode calculate the velocity of sound `
View SolutionA cylinder resonance tube open at both ends has fundamental frequency `F` in air. Half of the length of the tube is dipped vertically in water. The fundamental frequency to the air column now is …………………… .
View SolutionA closed organ pipe is vibrating in first overtone and is in resonance with another open organ pipe vibrating in third harmonic. The ratio of lengths of the pipes respectively is
View SolutionA glass tube of `1.0 m` length is filled with water. The water can be drained out slowly at the bottom of the tube. If a vibrating tuning fork of frequency `500 c//s` is brought at the upper end of the tube and the velocity of sound is `300 m//s`, then the total number of resonances obtained will be
View SolutionAn open and a closed pipe have same length . The ratio of frequency of their `n th` overtone is
View SolutionA tube of a certain diameter and of length `48cm` is open at both ends. Its fundamental frequency is found to be `320 Hz`. The velocity of sound in air is `320 m//sec`. Estimate the diameter of the tube.
One end of the tube is now closed. Calculate the lowest frequency of resonance for the tube.
View SolutionA closed organ pipe has length `l`. The air in it is vibrating in `3rd` overtone with a maximum amplitude of A. Find the amplitude at a distance of `l//4` from closed end of the pipe
View SolutionThe frequency of a streteched uniform wire of certain length is in resonance with the fundamental frequency of closed tube. If length of wire is decreased by `0.5m`, it is in resonance with first overtone of closed pipe. The initial length of wire is
View SolutionAn open pipe resonates to a frequency `f_(1)` and a closed pipe resonates to a frequency `f_(2)`. If they are joined together to form a longer tube, then it will resonate to a frequency of (neglect end corrections)
View SolutionThe the resonance tube experiment first resonant length is `l_(1)` and the second resonant length is `l_(2)`, then the third resonant length will be ?
View SolutionA pop- gun consists of a cylindrical barrel `3cm^(2)` in cross section closed at one end by a cork and having a well fitting piston at the other. If the piston is pushed slowly, in the cork is finally ejected, giving a pop, the frequency of which is found to be `512Hz`. Assuming that the initial distance between the cork and the piston was `25cm` and that there is no leaking of air, calculate the force required to eject the cork. Atmospheric pressure `=1kg.cm^(2)`, `v=340m//s` (in kg. wt).
View SolutionThere are two sources of sound of equal intensity with frequencies `400Hz` and `408Hz` are vibrated together. The number of beats heard per second is
View SolutionIf a tuning fork of frequency `512Hz` is sounded with a vibrating string of frequency `505.5Hz` the beats produced per sec will be
View SolutionThe natural frequency of a tuning fork `P` is `432`Hz. `3` beats/s are produced when tuning fork `P` and another tuning fork `Q` are sounded together. If P is loaded with wax, the number of beats increases to `5` beats/s. The frequency of Q is
View SolutionTwo organ (open) pipes of lengths `50cm` and `51cm` produce `6` beats/s. Then the speed of sound is nearly
View SolutionAn unknown frequency `x` produces 8 beats per seconds with a freuquency of `250` Hz and 12 beats with `270Hz`. Source then `x` is
View SolutionIn an experiment it was found that when a sonometer in its fundamental mode of vibration and a tunning fork gave `5` beats when length of wire is `1.05` metre or `1` metre. The velocity of transverse waves in sonometer wire when its length is `1m`
View SolutionA sonometer has `25` forks. Each produces `4` beats with the next one. If the maximum frequency is `288Hz`, which is the frequency of last fork. The lowest frequency is
View SolutionA tuning fork produces `6` beats/sec with sonometer wire when its tensions are either `169N` or `196N`. The frequency of that fork is
View SolutionIn an open pipe when air column is `20cm` it is in resonance with tuning fork A. When length is increased by `2cm ` then the air column is tn resonance with fork B. When A and B are sounded together `4` beats/sec are heard. Frequencies of A and B are respectively (in Hz)
View SolutionA closed organ pipe and an open organ pipe of same length produce `4` beats when they are set into vibrations simultaneously. If the length of each of them were twice their initial lengths, the number of beats produced will be
View SolutionAn air column in tun=be 32cm long, closed at one end, is in resonace with a tuning fork. The air column in another tube, open at both ends, of length 66cm is in resonance with another tuning frok.When those two tuning forks are sounded together , they produce 8 beats per second together, they produce 8 beats per second. then the frquencies of the twpo tuning forks are, (consider fundamental frequencies only)
View SolutionThe string of a sonometer is divided into two parts using wedge. Total length of string is `1m` and two parts differ by `2mm`. When sounded together they produce `2` beats/sec. The frequencies of two parts are
View SolutionOn vibrating an air column at `627^(@)C` and a tuning fork simultaneously, `6` beats/sec are heard. The frequency of fork is less than that of air column. No beats are heard at `-48^(@)C`.The frequency of fork is
View SolutionA string `25cm` long and having a mass of `2.5 gm` is under tension. A pipe closed at one end is `40cm` long. When the string is set vibrating in its first overtone and the air in the pipe in its fundamental frequency, `8` beats per second are heard. It is observed that decreasing the tension in the string decreases beat frequency. If the speed of sound in air is `320m//s`, find the tension in the string.
View SolutionTwo identical piano wires have fundemental frequency of `600` `vib//sec`, when kept under the same tension. What frectional increase in the tension of one wire will lead to the occurrence of six beats per second when both wires vibrate simuitaneously?
View SolutionThe speed at which a source of sound should move so that a stationary observer finds the apparent frequency equal to `11//12` of the original frequency
View SolutionThe difference between the apparent frequencies of whistle as received by an observe in rest during approach to recession of the train is `1%`. If velocity of sound is `320` m/sec, the velocity of the train is
View SolutionA source of sound emitting a note of frequency 200 Hz moves towards an observer with a velocity v equal to the velocity of sound. If the observer also moves away from the source with the same velocity v, the apparent frequency heard by the observer is
View SolutionAn engine giving whistle is moving towards a stationary observer with `110m//s` speed. What will be the ratio of the frequency of the whistle heard when the engine is approaching and receding from the observer (the speed of sound is `330 m//s`) ?
View SolutionTwo aeroplanes 'A' and 'B' are moving away from one another with a speed of `720kmph`. The frequency of the whistle emitted by 'A' is `1100Hz`. The apparent frequency of the whistle as heard by the passenger of the aeroplane 'B' is (velocity of sound in air is `350ms^(-1)`).
View SolutionAn engine is moving on a circular path of radius 100 m with a speed of 20 m/s. What will be frequency noted by an observer standing stationary at the centre of the circular path when the engine blows a whistle of frequency 500 Hz ?
View SolutionThe frequency of a radar is 780 MHz. After getting reflected from an approaching aeroplane, the apparent frequency is more than the actual frequency by 2.6 kHz. The aeroplane has a speed of
View SolutionAn observer moves towards a stationary source of sound, with a velocity one-fifth of the velocity of sound. What is the percentage increase in the apparent frequency?
View SolutionA train is moving at `30 m//s` in still air. The frequency of the locomotive whistle is `500 Hz` and the speed of sound is `345 m//s`. The apparent wavelengths of sound in front of and behind the locomotive are respectively
View SolutionA vehicle, with a horn of frequency n is moving with a velocity of 30 m/s in a direction perpendicular to the straight line joining the observer and the vehicle. The observer perceives the sound to have a frequency `n + n_(1)`. Then (if the sound velocity in air is 300 m/s)
View SolutionA source of sound is travelling towards a stationary observer. The frequency of sound heard by the observer is `25 %` more than the actual frequency. If the speed of sound is `v`, that of the source is
View SolutionA truck blowing horn of frequency `500Hz` travels towards a vertical mountain and driver hears echo of frequency `600Hz`. If velocity of sound in air is `340m//s` then speed of truck is
View SolutionOne train is approaching an observer at rest and another train is receding from him with the same velocity `4 m//s` . Both trains blow whistles of same frequency of `243 H_(Z)` . The beat frequency in `H_(Z)` as heard by observer is (speed of sound in air = `320 m//s`)
View SolutionA tuning fork of frequency `328Hz` is moved towards a wall at a speed of `2ms^(-1)`. An observer standing on the same side as the fork hears two sounds, one directly from the fork and the other reflected from the wall. Number of beats per second is (Velocity of sound in air `330ms^(-1)`).
View SolutionThe frequency of the sound of a car horn as recorded by an observer towards whom the car is moving differs from the frequency of the horn by `10%`. Assuming the velocity of sound in air to be `330ms^(-1)`, the velocity of the car is
View SolutionTwo trains are approaching each other on parallel tracks with same velocity. The whistle sound produced by one train is heard by a passenger in another train. If actual frequency of whistle is `620Hz` and apparent increase in its frequency is `100Hz`, the velocity of one of the two trains is (Velocity of sound in air `=335ms^(-1)`)
View SolutionA girl swings in a cardle with period `pi//4` second and amplitude `2m`. A boy standing infront of it blows a whistle of natural frequency `1000Hz`. The minimum frequency as heard by the girl is (Velocity of sound in air is `320ms^(-1)`)
View SolutionThe difference between the apparent frequency of a source of sound as perceived by an observer during its approach and recession is 2% of the natural frequency of the source. Then the speed of the source will be
View SolutionTwo different sound sources `S_(1)` and `S_(2)` have frequencies in the ratio `1:2`. Source `S_(1)` is approaching towards observer and `S_(2)` receding from same observer. Speeds of both `S_(1)` and `S_(2)` are V each and speed of sound air is `330m//s`. If no beats are heard by the observer then the value of V is
View Solution
Exercise-I (C.W) questions and complete solutions for chapter WAVES of NARAYANA PHYSICS (HINGLISH) of Class 11