Complete Solutions to Exercises of chapter MOMENT, TORQUE, EQUILIBRIUM OF BODIES of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | MOMENT, TORQUE, EQUILIBRIUM OF BODIES |
| NN GHOSH | Exercises |
| Number Of Questions | 33 |
| Academic Year | 2023-24 |
Find the centre of gravity of a thin wire bent to a semicircle of radius r.
View SolutionTwo like parallel forces P and Q act at the ends of a strianght ruler of length l. What is the distance by which the resultant of the forces will shift along the ruler If P is doubled ?
View SolutionA circular section of radius r si cut out of a uniform disc of radius R, the centre of the hole being R/2 from the centre of the original disc. Locate the centre of gravity of the remainder.
View SolutionA cylindrical vessel of radius 1 m and height 2 m is half filled with water. Find the centre of mass of the system consisting of cylinder and water. The cylinder has mass 10 kg per square metre of it and density of water 1000 kg `m^(-3)`.
View SolutionFind the centre mass of the following L and T-shaped planks with reference to the frame indicated in figure 6.10.

View SolutionFind the CG of :(a) a cone of height h from the base,(b) a hemisphere of radius r from its flat surface.
View SolutionA solid of uniform density is made of a hemisphere and a right circular cone which have a common base of radius r . Determine the value of h of the cone if the CG of the system is at the centre of the base.
View SolutionA particle of mass m is placed upon the smooth face of a prism, also of mass m, which particle is placed , is inclined at an angle `alpha` to the horizontal . Show that when the particle has moved a distance x down the face of the prism the prism itself will have moved a distance of `(1)/(2)x "cos" alpha`
View SolutionSuppose you have to pile up uniform bricks to erect an inclined wall by offsetting each brick by a constant fraction ,`(1)/(n)` , of a brick length l over the beneath. How many bricks can be piled by before the wall collapse ? How high a wall can erect if the size of each bricks is `7.5 cm xx 12.5 cm xx 25 cm` and the offset is only `(1)/(10)` of the length of each bricks? What is the total offset you get ?
[Hints: The vertical line through CG of the wall must pass through the base. The moment it goes beyond the base brick, the wall will collapse.]
View SolutionWhat is the maximum offset that can obtain by piling up four identical bricks of length l ?
[Hints :CG if any two from top must pass through the edge of the third beneath . The uppermost brick may overhang by `(1)/(2)` .]
View SolutionA flexible chain of weight W hangs between two fixed points A and B at the same level. The inclination of the chain with the horizontal at the two points of support is `theta`. Calculated the tension of the chain at end points and also at the lower most point .
[Hints : Consider equilibrium of each half of the chain. ]
View SolutionA thin horizontal bar AB of negligible mass and length l is hinged to a vertical wall at A and supported at B by thin wire BC which is tied to a point C vertically above in the same wall.BC makes angle `theta` with the horizontal . A weight W is moved along the bar. Show how the tensile force T in the thin wire changes with the distance x of the weight from the wall.
View SolutionA 50 -kg plank of length 6 m rests on the ground at one end on a frictionless roller on the top of a wall of height h=3 m at the other end. The plank projects a little beyond the wall. It remains in equilibrium for any value `theta ge 70^(@)` , where `theta` is the angle made by the plank with the horizontal. Find the cofficient of friction between the plank and the ground.
[Hints : Apply the equilibrium conditon that `sumvectau=0 "and "sumvecf=0`.]
View SolutionThe step-ladder shown in figure 6.11 is 8 m long and hinged at C.BD is a tie rod 2.5 m long half way up. A man of 86 kg climbs 6 m along the ladder . Find the tension in the tie rod the forces exerted on the ladder by the assuming the floor to frictionless and the ladder weightless.

[Hints: Consider the free-body diagram of the sides of the ladder.]
View SolutionTwo particles P and Q are initially at rest and 1 m aparts. P has a mass of 1 kg and Q a mass of 3 kg . P and Q attract each other with a constant force. At what distance from P's original position do the particles collide?
View SolutionA table has a heavy circular top of radius 1 m and mass 20 kg . It has four light legs of length 1 m fixed symmetrically on its circuference. What is the maximum weight which may be placed anywhere on this table wihtout toppling it?
View SolutionA pulley fixed to the ceiling carries a thread with bodies of masses `m_(1)` and `m_(2)` attached at its ends. The masses of the pulley and thread are negligible, friction is absent. Find the acceleration of the centre of mass`(a_("cm"))` of this system.
View SolutionA man weighting 60 kgis standing at the centre of a flat boat and he is 20 m from the shore. He walks 8 cm on the boat towards the shore and then halts. The boat weights 200 kg How far is the from the shore at the end of this time?
[Hints : As the system is free from external force, centre of mass of the system consisting of man and boat must not move.
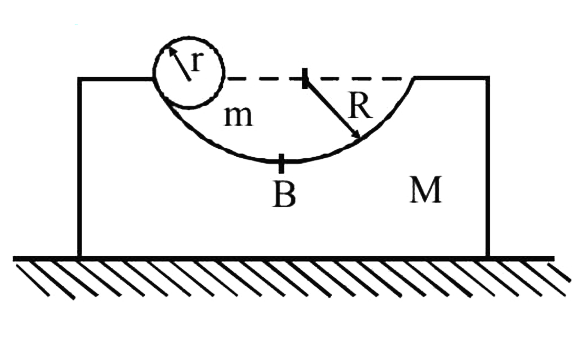
View SolutionA block of mass M with a semicircualr of radius R, rests on a horizontal frictionless surface. A uniform cylinder of radius r and mass m is released from rest the top point A The cylinder slips on the semicircular frictionless track. How far has the block moved when the cylinder reaches the bottom (point B) of the track ? How fast is the block moving when the cylinder reaches the bottom of the track?
View SolutionIn the system shown in Figure 6.13 P and Q are in equilibrium . If P is doubled, calculate the acceleration of P. The pulley is light.

View SolutionA cone of height h and semivertical angle `alpha` is just held in equilibrium against a smooth vertical wall by a string attached to the apex and tied to the wall at the other end. Calcualate the length of the string.
View SolutionWhat is the mechanical advantage of an inclined plane as a machine when inclination of the plane is `30^(@)` with the horizontal , if the force acts (i) horizontally,(ii) along the plane ? What force along the plane is needed to raise a scooter of weight 150 kg ?
View SolutionThe fulcrum of a lever of length 1 m is at its middle point. If the fulcrum is moved by 0.25 m towards the loads , how are efficiency and velocity ratio affected?
View SolutionIn a wheel and axle machine the radii of the wheel and axle are 0.5 m and 0.05 m,respectively . A weight of 100 kg is raised when it is hanging by a rope from the axle by applying a force of 12 kg on the circumference of the wheel . Find the mechanical advantage, velocity ratio and efficiency of this machine.
View SolutionA jack of pitch 4 mm is worked by a handle of length 50cm .There is friction between the screw and the nut of it. To overcome the frictional force an extra effort of 0.004 times the load has to be applied . Calculate the least force required to raise and lower a load of 500 kg (take `g=10 ms^(-2)`)
View SolutionA pulley arrangement is shown in figure 6.14 .A has a diameter of 30 cm and the cylinder B has a diameter of 20 cm. The working handle has an arm of 50 cm. The direction of winding of the rope is opposite to that of B. Calculate the mechanical advantage of the machine.

View SolutionShow that a nonhomogeneous cylinder on a horizontal plane has a position of stable equilibrium when its centre of gravity is vertically below the axis O of the cylinder.
View SolutionA dump-bell consists of two weights `W_(1)` and `W_(2)(W_(2) gt W_(1))` and is hinged at a point distant `l_(1)` and `l_(2)` from `W_(1)` and `W_(2)` , respectively . Establish the criterion of stable equilibrium for the vertical position of the dump-bell.
View SolutionA hemispherical cup of radius r with its centre of gravity at C distant c from its centre rests on the top of a spherical surface of radius R as shown in figure 6.15 .Assuming that there is sufficient friction to prevent slipping , establish the criterion of stablility of the cup in the position shown.

View SolutionA cube of uniform density and edge a is balanced on a cylindrical surface of radius r. Shown that the criterion for stability of equilibrium of the cube, assuming that fraction is sufficient to prevent slipping, is `r gt a//2`
View SolutionA bead can slide on a smooth straight wire and a particle of mass `m` attached to the bead by a light string of length `L`. The particle is held in contact with the wire and with the string taut and is then let fall. If the bead has mass `2m` then when the string makes an angle `theta` with the wire, the bead will have slipped a distance.

View SolutionA hoop with mass M and radius R lies flat on a perfectly smooth surface. An insect with mass m rests on it. On being distrubed by some noise it starts crawling on the hoop with speed v. Find the trajectory of the centre of the hoop and its speed.
View SolutionA worker wishes to pile up sand on to a circular area of radius R. No sand is to spill on to the surrounding area. If `mu_(s)` is the coefficient of static friction of sand on sand,the sand pile . Density of sand `=rho`
View Solution
Exercises questions and complete solutions for chapter MOMENT, TORQUE, EQUILIBRIUM OF BODIES of BHARATI BHAWAN PHYSICS (HINGLISH) of Class 11