Complete Solutions to Practice Exercise of chapter NEWTONS LAWS OF MOTION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | NEWTONS LAWS OF MOTION |
| PHYSICS GALAXY | Practice Exercise |
| Number Of Questions | 23 |
| Academic Year | 2023-24 |
The masses of blocks A and B in figure-2.24 are 20 kg and 10 kg, respectively. The blocks are initially at rest on the floor and are connected by a massless string passing over a massless and frictionless pulley. An upward force F is applied to the pulley. Find the acceleration `a_(1)` and `a_(2)` of the two blocks A and B when F is (a) 124 N (b) 294 N (c ) 424 N.
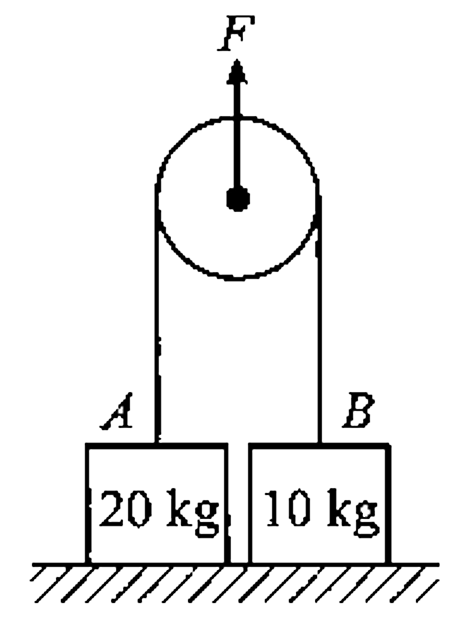
`[(a)0,0(b)0,4.7m//s^(2), (c ) 0.6m//s^(2),11.2 m//s^(2)]`
View SolutionFind the acceleration of the blocks A and B shown in figure-2.26 (a) and (b)
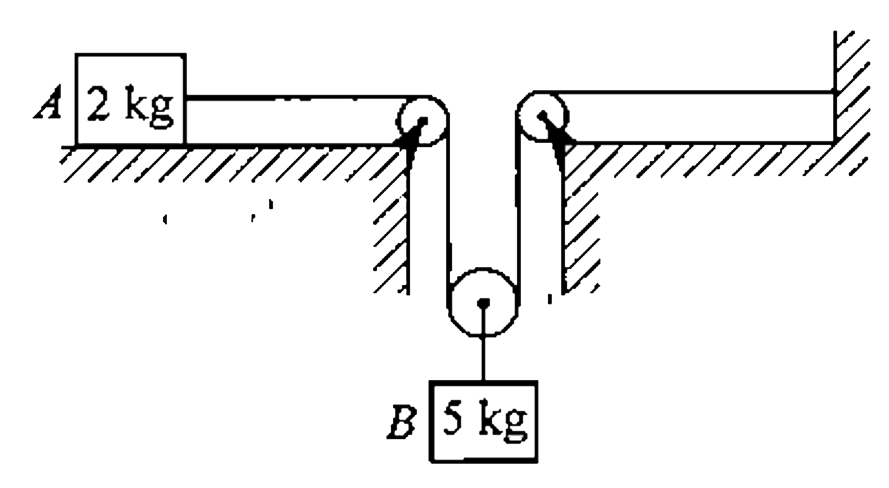
`[(a) 10g//13 " forward, "5g//13 " downward, "(b) 8g//13 " downward"]`
View SolutionTwo masses m and 2m are connected by a mass less string which passes over a light frictionless pulley shown in figure-2.28. The masses are initially held with equal lengths of the strings on either side of the pulley. Find the velocity of the masses at the instant the lighter mass moves up a distance of 6.54 mts. This string is suddenly cut at that instant. Calculate the time taken by each mass to reach the ground. Take `g = 9.8 m//s^(2)`.
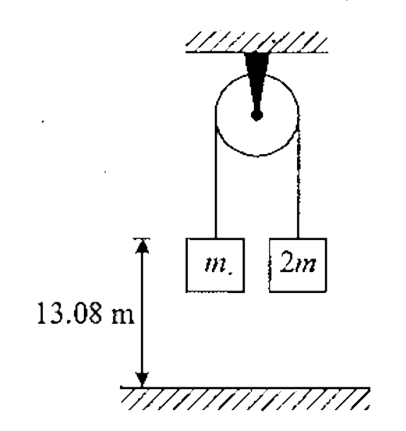
`[3.27 m//s^(2), 2.78 sec, 2//3 sec]`
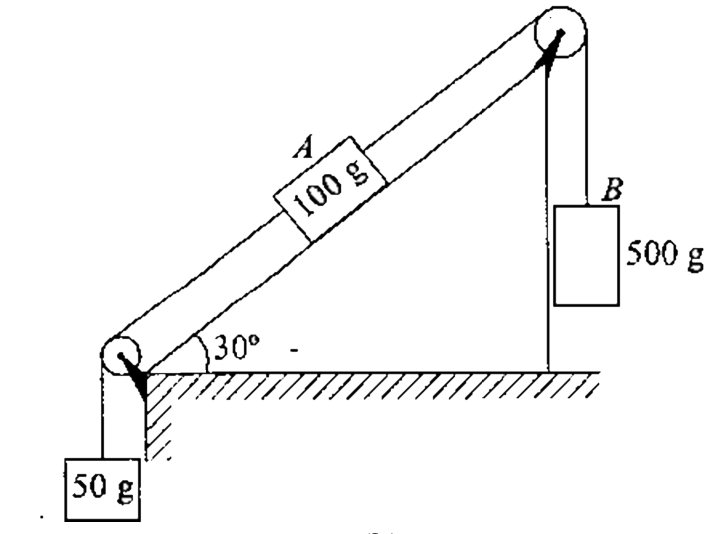
View SolutionIn figure-2.47 the tension in the diagonal string is 60 N. Find the magnitudes of the horizontal forces `F_(1)` and `F_(2)` the must be applied to hold the system in the position shown in figure-2.48. What is the weight of the suspended block.
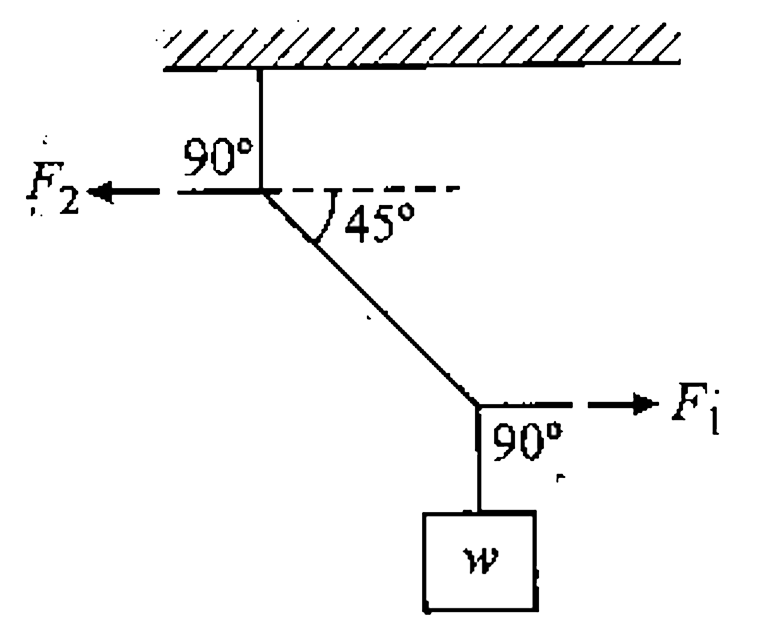
[42.4 N each, 42.4 N]
View SolutionA 1 m rod of mass 100 kg is hanging from two in extensible support strings at its ends of equal lengths. If an another mass of 20 kg is placed on rod at a distance 30 cm from the left end, find the tension in the two support strings, if rod remains horizontal. Take `g = 10m//s^(2)`
[560 N, 640 N]
View SolutionFind the tension in each cord in figure-2.48, if the weight of the suspended block is w.
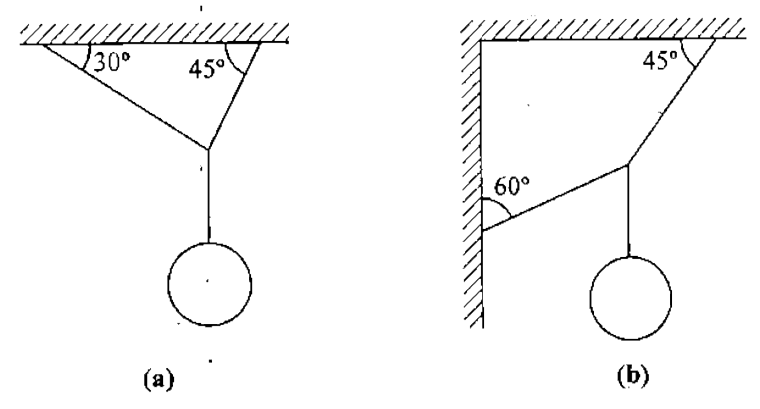
`[(a)(2w)/(1+sqrt(3)),(sqrt(6)w)/(1+sqrt(3)),(b) (2w)/(sqrt(3)-1),(sqrt(6)w)/(sqrt(3)-1)]`
View SolutionA horizontal uniform boom that weighs 200 N and is 5m long supports a load of 1000N, as shown in figure-2.49. Find all the forces acting on the boom.
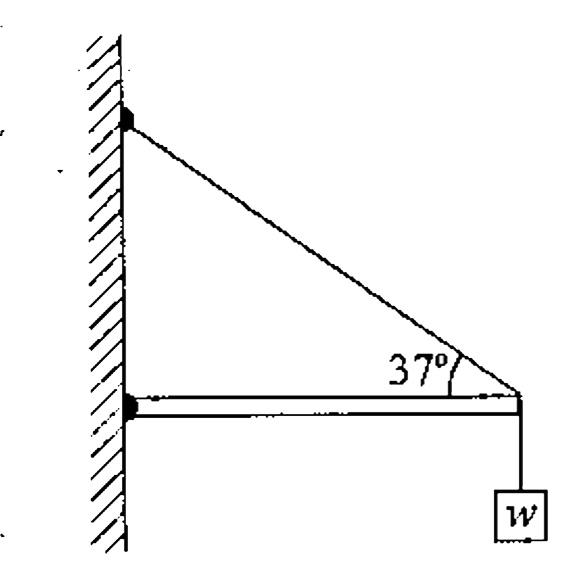
[T=1833.33 N, H = 1466.67 N, V = 100 N]
View SolutionA step pulley system is shown in figure-2.50. Find the relation in masses m and M for which the system remain in equilibrium. Assume string will not slide over the pulleys. `((r_(1))/(r_(2))=(1)/(2),(r_(3))/(r_(4))=(3)/(4))`
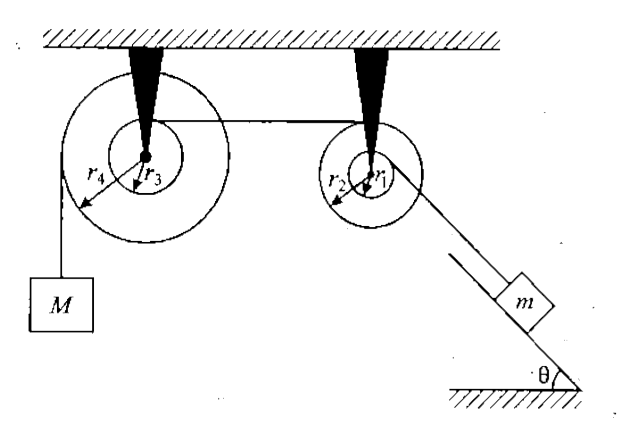
`[(M)/(m)=(3)/(8)sintheta]`
View SolutionA nut cracker is used to crack a nut. In it nut is held in two arms at a distance 2 cm from the hinge and the handle of it is at a distance 25 cm from hinge. The force required to crack a walnut is 30 N. What is the minimum force required to crack it with the nut cracker.
[2.4 N]
View SolutionTwo cubes of masses `m_(1)` and `m_(2)` lie on two frictionless slopes of the block A which rests on horizontal table. The cubes are connected by a string, which passes over a pulley as shown in the figure-2.67. To what horizontal acceleration "f" the block accelerates so that the cubes do not slide down the planes ? What is the tension in the string in this situation ?
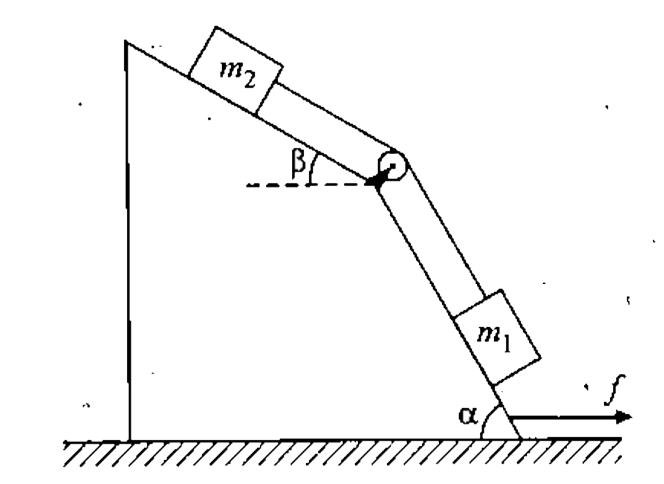
`[f=g((m_(1)sinalpha+m_(2)sinbeta)/(m_(1)cosalpha+m_(2)cosbeta)),T = (m_(1)m_(2)gsin(alpha-beta))/(m_(1)cosalpha+m_(2)cosbeta)]`
View SolutionIn the arrangement shown in figure-2.68 the masses of the wedge M and the body m are known. There is no friction at any of the surfaces. The mass of the pulleys and thread is negligible. Find the acceleration of the body m relative to the horizontal surface on which the wedge slides.
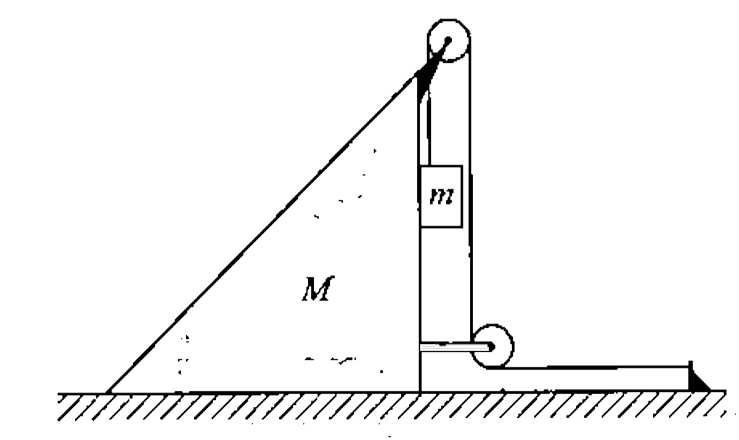
`[(sqrt(2)mg)/(M+2m)]`
View SolutionA body with a mass m slides along the surfac of a trihedral prism of mass M, whose upper plane is inclined at an angle `alpha` to the horizontal. The prism rests on a horizontal plane having a vertical ledge at the rear edge of the prism to keep it at rest as show in figure-2.69. Find the force exerted by the base of the prism on the plane ? Also find the force exerted by ledge on prism.
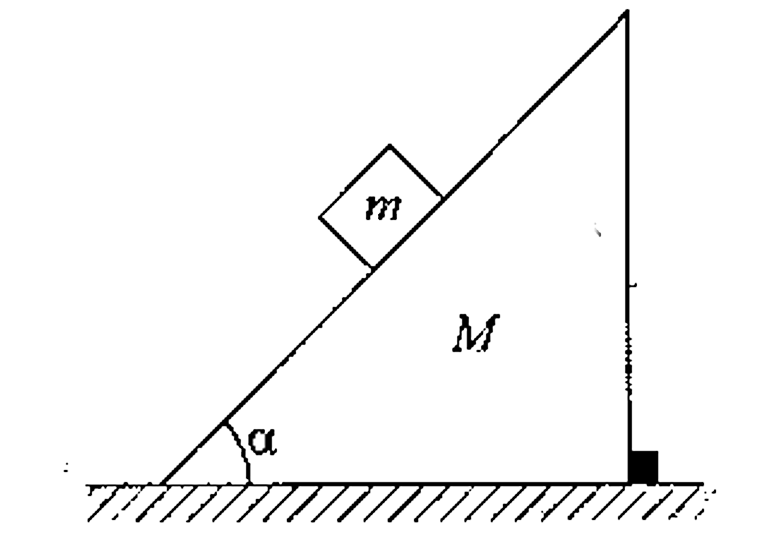
`[Mg +mgcos^(2)alpha, mg sinalphacosalpha]`
View SolutionIf the man manages to keep himself at rest on platform, as shown in figure-2.71. Find the acceleration of the system masses m and M. All pulleys, platform and string are light and frictionless. Also find the force man has to exert on string in this situation.
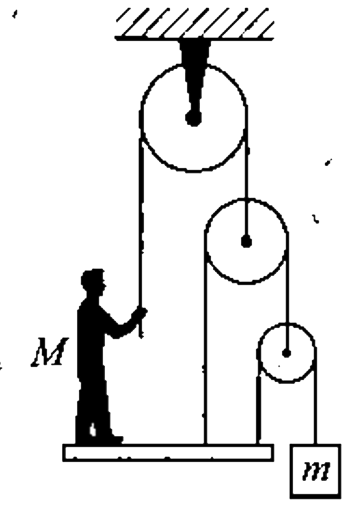
`[(7m-M)/(49m+M)g,(8mM)/(49m+M).g]`
View SolutionCalculate the force P required to cause the block of weight `W_(2)=200N` just to slide under the block of weight `W_(1)=100N` shown in figure-2.83. What is the tension in the string AB ? Coefficient of friction `mu = 0.25` for all surfaces in contact.
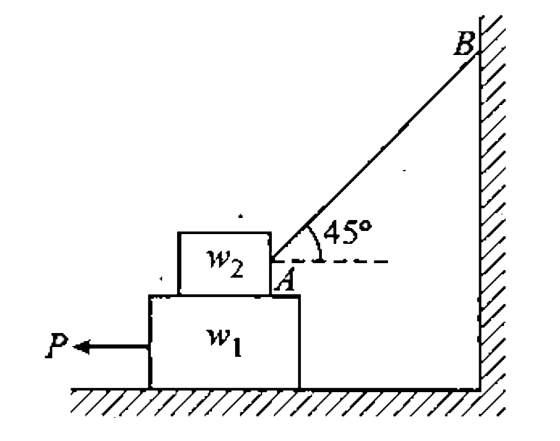
`[90 N, 20sqrt(2)N]`
View SolutionFigure-2.84 shows a man ofmass M standing on a board of mass m.What minimum force is required to exert on string to slide the board. The friction coefficient between board and floor is `mu` and there is sufficient friction between man and board so that man does not slip.
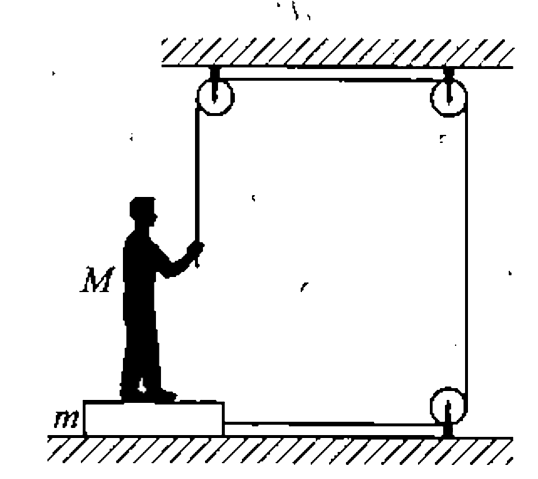
`[(mu(M+m)g)/(1+mu)]`
View SolutionFigure-2.85 shows a block B of mass m, cart C of mass M, and the coefficient of static between the block and the cart is `mu`. Neglect frictional between wheels and axles and the rotational effects of the wheels. Determine the minimum value of F which must be applied on B such that it will not slide.
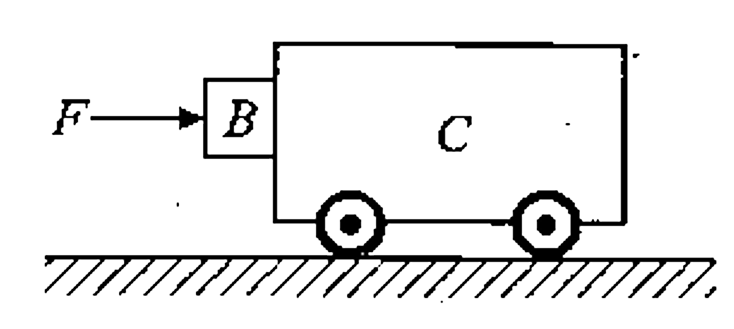
`[(mg)/(mu)(1+(m)/(M))]`
View SolutionIn figure-2.86, the blockA of mass `M_(1)` rests on a rough horizontal surface. The coefficient of friction between the block and the surface is `mu`. A uniform plank B, of mass `M_(2)` rests on A. B is prevented from moving by connecting it to a light rod R which is hinged at one end H.The coefficient of friction between A and B is `mu`. Findthe acceleration of blocks A and C.
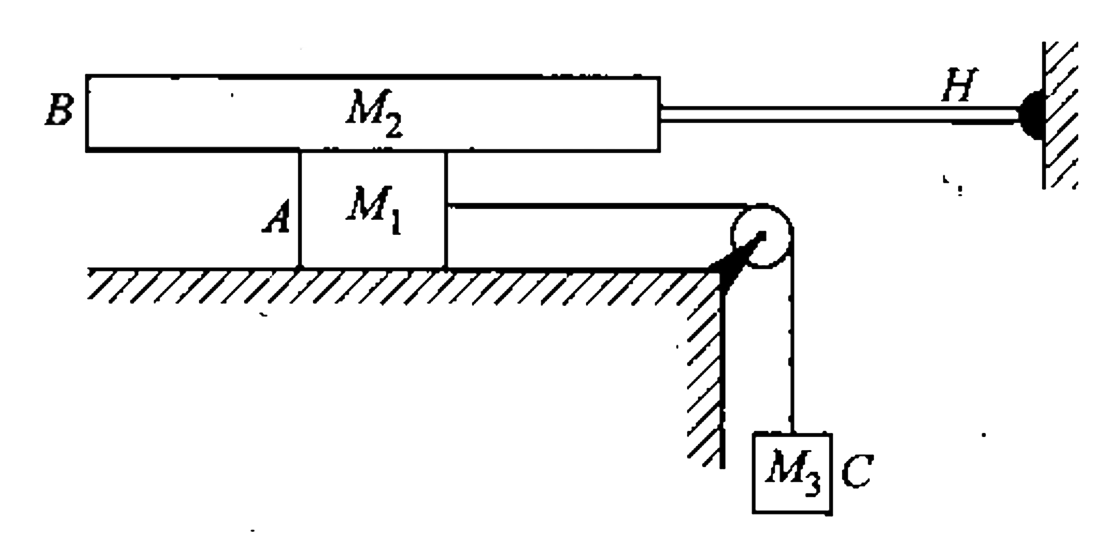
`[(M_(3)g-mug(M_(1)+2M_(2)))/((M_(1)+M_(3)))]`
View SolutionIn the arrangement shown in figure the masses of the wedge `M` and the body `m` are known. The appreciable friction exists only between the wedge and the body `m`, the friction coefficient being equal to `k`. The masses of the pulley and the thread are negligible. Find the acceleration of the body `m` relative to the horizontal surface on which the wedge slides.

View SolutionIn the situation shown in figure-2.102, (a) What minimum force will make any part for whole system move. (b) For the following values of force, find the acceleration of two blocks, nature and value of friction at the two surfaces 2 N and 6 N. Take ` g = 10 m//s^(2)`.
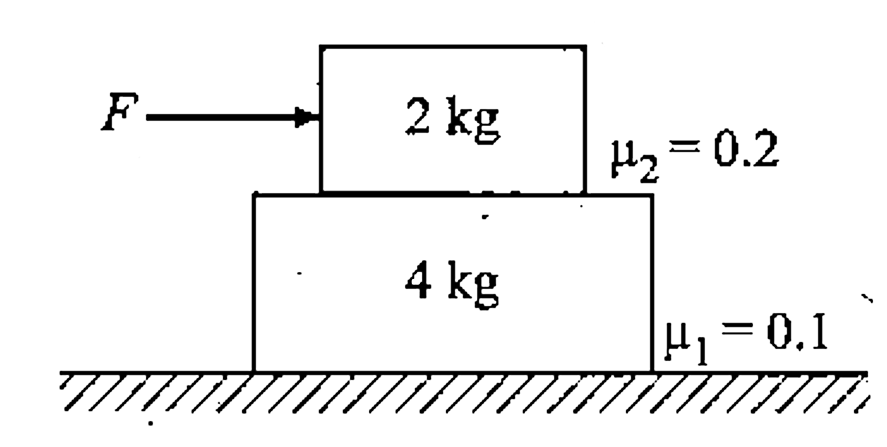
`[(a) 4N, (b) 1m//s^ (2),0]`
View SolutionIn the sitution shown in figure there is no friction between `2 kg` and ground.
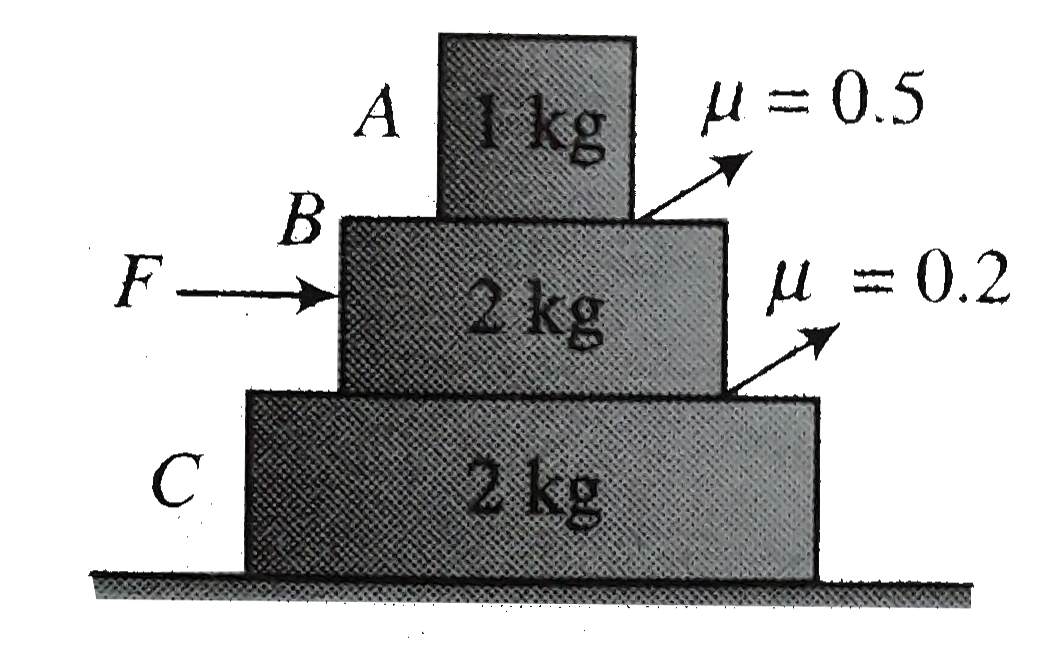
a. For what maximum value of force `F` can all three blocks move together?
b. Find the value of force `F` at which sliding starts at other rough surfaces.
c. Find acceleration of all blocks, nature and value of friction force for the following values of force `F` (i) `10N` (ii) `N` and (iii) `25 N`
View SolutionA smooth semicircular wire-track of radius R is fixed in a vertical plane. One end of a massless spring of natural length `3R//4` is attached to the lowest point O of the wire-track. A small ring of mass m, which can slide on the track, is attached to the other end of the spring. The ring is held staionary at point P such that the spring makes an angle of `60^@` with the vertical. The spring constant `K=mg//R`. Consider the instant when the ring is released, and (i) draw the free body diagram of the ring, (ii) determine the tangential acceleration of the ring and the normal reaction.
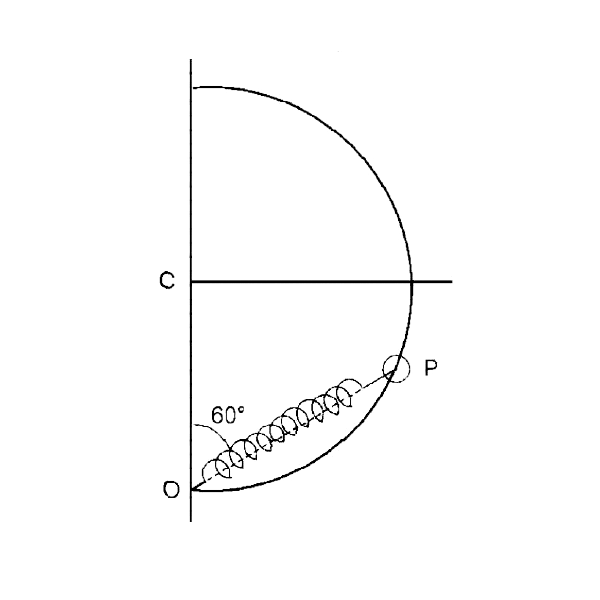
View SolutionFind the acceleration of masses `m_(1), m_(2)` and `m_(3)` shown in figure-2.119 just after the string is cut at point A.
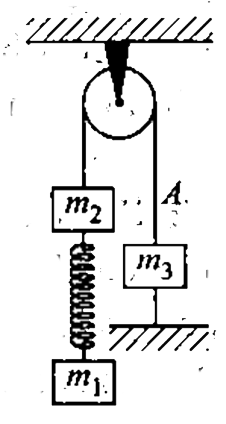
`[0,(1+(m_(1))/(m_(2)))g, g]`
View SolutionIn figure shown if 2 kg block is moving at an acceleration `2 m//s^(2)`, find the elongationin spring and acceleration of 4 kg block at this instant. Take ` g= 10m//s^(2)`.
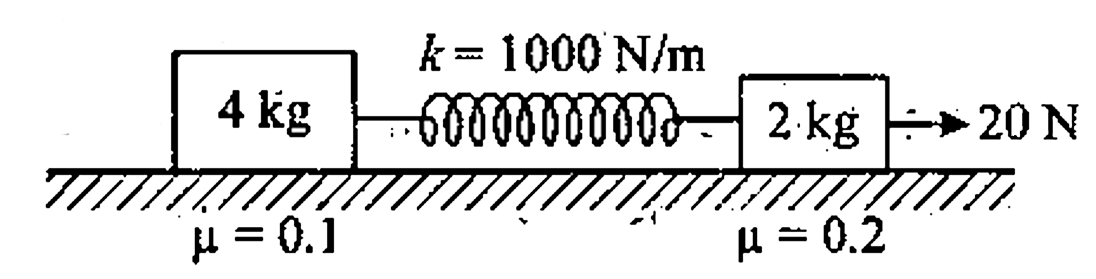
`[0.012 m, 2 m//s^(2)]`
View Solution
Practice Exercise questions and complete solutions for chapter NEWTONS LAWS OF MOTION of GK PUBLICATIONS PHYSICS (HINGLISH) of Class 11