Complete Solutions to Problem of chapter CALORIMETRY AND THERMAL EXPANSION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | CALORIMETRY AND THERMAL EXPANSION |
| RESONANCE | Problem |
| Number Of Questions | 26 |
| Academic Year | 2023-24 |
Calculate amount of heat required to convert `1kg` steam form `100^(@)C to 200^(@)C` steam
View SolutionHeat required to raise the temperature of one gram of water through `1^@C` is
View Solution`420 J` of energy supplied to `10g` of water will rises its temperature by
View SolutionThe ratio of the densities of the two bodies is `3:4` and the ratio of specific heats is `4:3` Find the ratio of their thermal capacities for unit volume?
View SolutionHeat released by `1kg` steam at `150^(@)C` if it convert into `1kg` water at `50^(@)C`.
View Solution`200 gm` water is filled in a calorimetry of negligible heat capacity. It is heated till its temperature is increase by `20^(@)C`. Find the heat supplied to the water.
View SolutionA bullet of mass `5gm` is moving with speed `400m//s`. Strike a targent and energy. Then calculate rise of temperature of bullet. Assuming all the lose in kinetic energy is converted into heat energy of bullet if is specific heat is `500 J//kg^(@)C`.
View Solution`1kg` ice at `-10^(@)C` is mixed with `1kg` water at `100^(@)C`. Then final equilirium temperature and mixture content.
View Solution`1kg` ice at `-10^(@)` is mixed with `1kg` water at `50^(@)C`. The final equilibrium temperature and mixture content.
View SolutionA small ring having small gap is shown in figure on heating what will happen to size of gap.

View SolutionAn isosceles triangles is formed with a thin rod of length `l_(1)` and coefficient of linear expansion `alpha_(1)`, as the base and two thin rods each of length `l_(2)` and coefficient of linear expansion `alpha_(2)` as the two sides. The distance between the apex and the midpoint of the base remain unchanged as the temperature is varied. the ratio of lengths `(l_(1))/(l_(2))` is

View SolutionA concrete slab has a length of 10 m on a winter night when the temperature is `0^@C`. Find the length of the slab on a summer day when the temperature is `35^@C`. The coefficient of linear expansion of concrete is `1.0 xx 10^(-5 @) C^(-1)`.
View SolutionA steel rod is clamped at its two ends and rests on a fixes horizontal base. The rod is unstrained at `20^@C`. Find the longitudinal strain developed in the rod if the temperature rises to `50^@C`. Coefficient of linear expansion of steel `=1.2 xx 10^(-5 @)C^(-1)`.
View SolutionIf rod is initially compressed by `Deltal` length then what is the strain on the rod when the temperature
(a) is increased by `Delta theta` (b) is decreased by `Delta theta`
View SolutionA pendulum clock having copper rod keeos correct time at `20^0 C`. It gains 15 seconds per day if cooled to `0^@C`. Calculate the coefficient of linear expansion of copper.
View SolutionA meter scale made of steel is calibrated at `20^@C` to give correct reading. Find the distance between 50 cm mark and 51 cm mark if the scale is used at `10^@C`. Coefficient of linear expansion of steel is `1.1 xx 10^(-5 @)C^(-1)`
View SolutionA uniform solid brass sphere is rotating with angular speed `omega_0` about a diameter. If its temperature is now increased by `100^@C`, what will be its new angular speed. (given`alpha_B=2.0xx10^-5per^@C`)
View SolutionThe volume occupied by a thin- wall brass vessel and the volume of a solid brass sphere are the same and equal to `1,000 cm^(3) at 0^(@)C`. How much will the volume of the vessel and that of the sphere change upon heating to `20^(@)C`? The coefficieny of linear expansion of brass is `alpha = 1.9 xx 10^(-5)`.
View SolutionWhen a thin rod of length `'l'` is heated from `t_(1)^(0)C` to `t_(2)^(0)C` length increases by `1%` . If plate of length `2l` and breadth `'l'` made of same material is heated form `t_(1)^(0)C` to `t_(2)^(0)C`, percentage increase in area is
View SolutionThe density of wathr at `0^@C` is `0.998 g cm^(-3)` at `4^@C` is `1.00 g cm^(-3)`. Calculate the average coefficient of volume expansion of water in the temperature range 0 to `4^C`.
View SolutionA glass vessel measures exactly `10 cm xx 10 cm xx 10 cm` at `0^@C`. It is filled completely with mercury at this temperature. When the temperature is raised 10 `10^C, 1.6cm^3` of mercury overflows. Calculate the coefficient of volume expansion of mercury. Coefficient of linear expansion of glass `= 6.5 xx 10^(-6 @)C^(-1)`.
View SolutionA metal ball immersed in alcohol weights `W_1` at `0^@C` and `W_2` at `50^@C`. The coefficient of expansion of cubical the metal is less than that of the alcohol. Assuming that the density of the metal is large compared to that of alcohol, it can be shown that
View SolutionIn figure which strip brass or steel have higher coefficient of linear expansion.
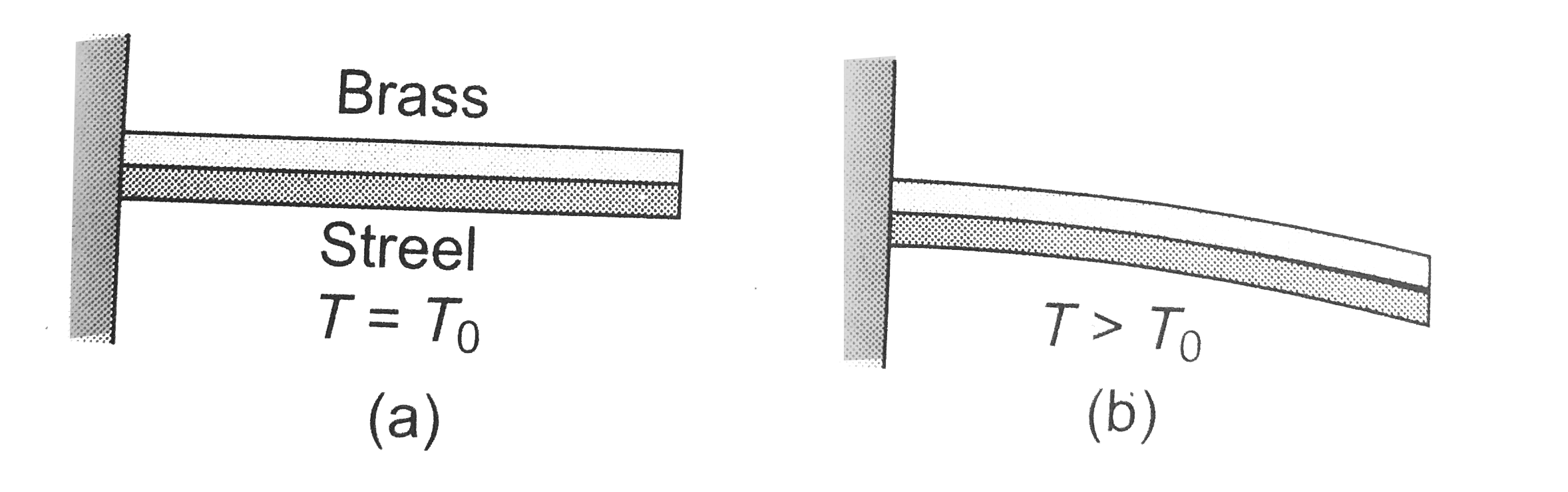
View SolutionThe upper and lower fixed points of a fualty thermometer are `5^(@)C` and `105^(@)C`. If the thermometer reads `25^(@)C`, what is the actual temperature?
View SolutionAt what temperature is the Fahrenheit scale reading equal to
(a) twice (b) half of Celsius ?
View SolutionIf the temperature of a patient is `40^(@)C` , his temperature on the Fahrenheit scale will be
View Solution
Problem questions and complete solutions for chapter CALORIMETRY AND THERMAL EXPANSION of RESONANCE ENGLISH of Class 11