Complete Solutions to Exercise of chapter FLUID MECHANICS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | FLUID MECHANICS |
| RESONANCE | Exercise |
| Number Of Questions | 45 |
| Academic Year | 2023-24 |
A large open tank has two holes in the wall. One is a square hole of side L at a depth h from the top and the other is a circular hole of radius R at a depth 4h from the top. When the tank is completely filled with water, quantities of water flowing out per second from both holes are the same. Then R is equal to
View SolutionA cubical metal block of edge 12 cm floats in mercry with one fifth of the height inside the mercury. Water poured till the surface of the block is just immersed in it. Find the height of the water column to be poured. Specific gravity of mercury =13.6.
View SolutionA vessel contains oil (density `= 0.8 g//cm^(3)`) over mercury (density `= 13.6 g//cm^(3)`). A uniform sphere floats with half its volume immersed in mercury and the other half in oil. The density of the material of sphere in `g//cm^(3)` is
View SolutionA block of iron is kept at the bottom of a bucket full of water at `2^@C`. The water exerts bouyant force on the block. If the temperature of water is increased by `1^@C` the temperature of iron block also increases by `1^@C`. The bouyant force on the block by water
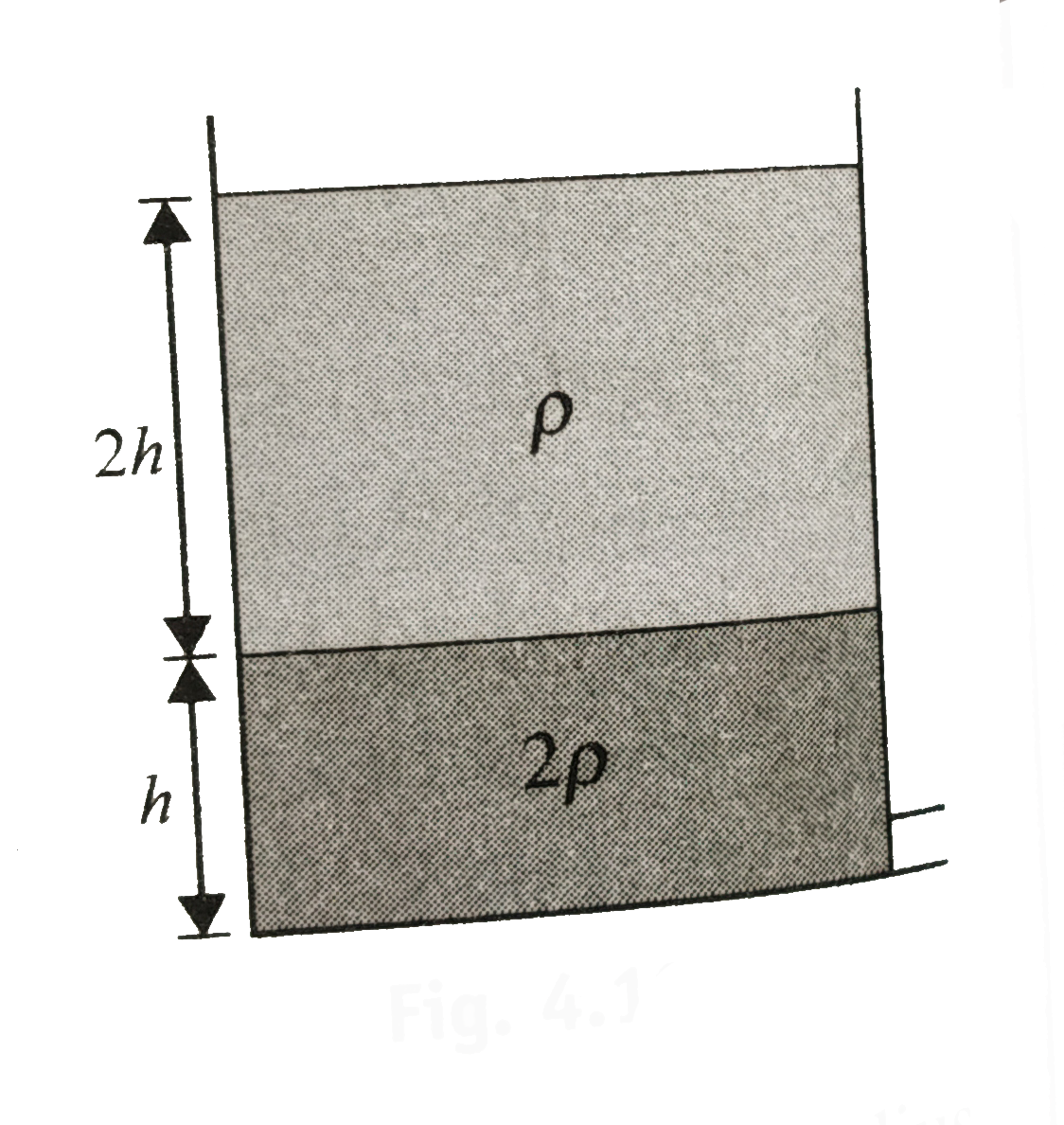
View SolutionThe velocity of the liquid coming out of a small hole of a vessel containing two different liquids of densities `2rho` and `rho` as shown in the figure is
View SolutionA block is partially immeresed in a liquid and the vessel is accelerating upwards with an acceleration 'a'. The block is observed by two observers `O_1` and `O_2`, one at rest and the other accelerating with an acceleration 'a' upward. The total buoyant force on the block is :
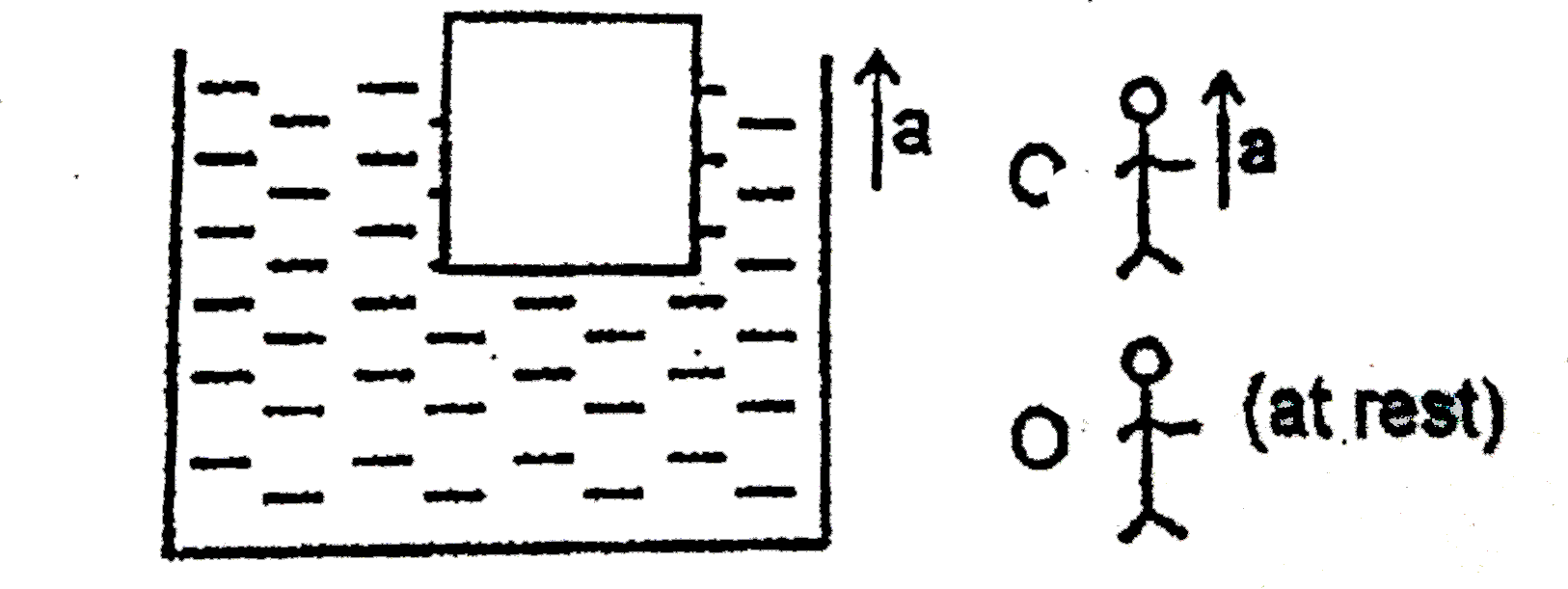
View SolutionFor a fluid which is flowing steadily, the level in the vertical tubes is best represented by
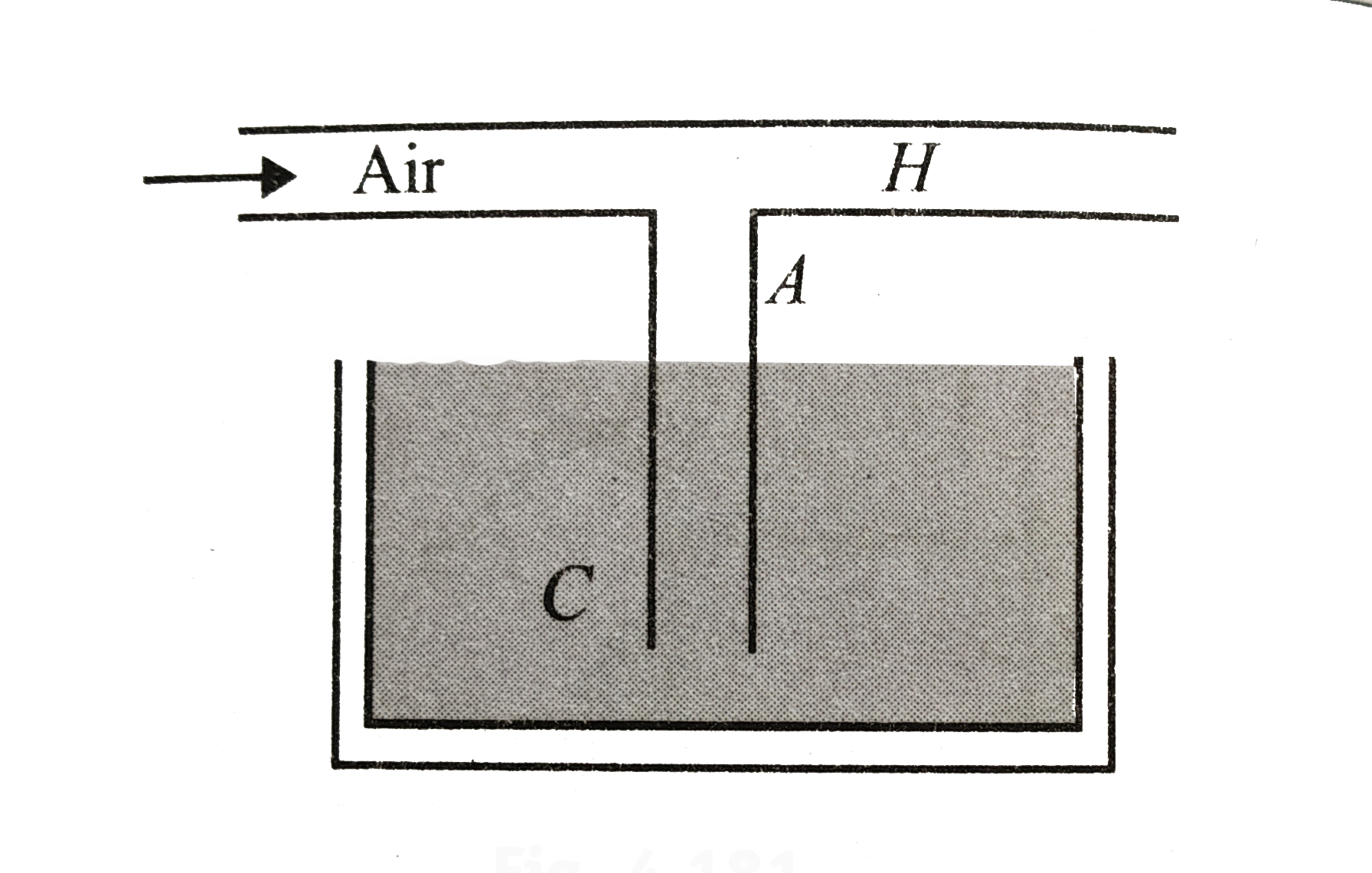
View SolutionFigure shows a capillary tube `C` dipped in a liquid that wets it. The liquid rises to a point `A`. If we blow air through the horizontal tube `H`, what will happen to the liquid column in the capillary tube?
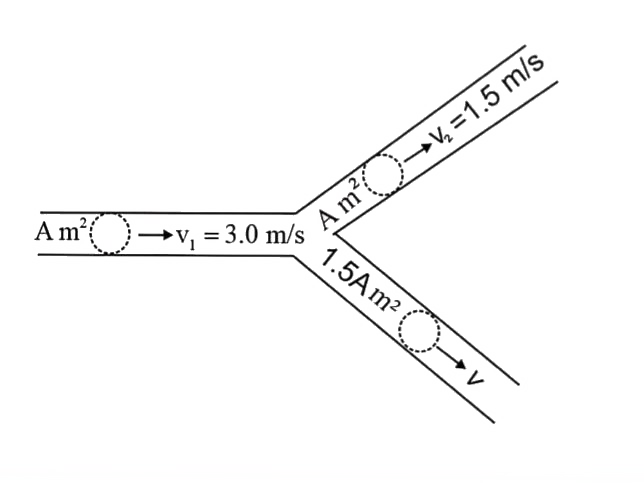
View SolutionAn incompressible liquid flows through a horizontal tube as shown in the following figure. Then the velocity v of the fluid is
View SolutionThe cubical container ABCDEFGH which is completely filled with an ideal (nonviscous and incompressible) fluid, moves in a gravity free space with a acceleration of `a=a_0 (hat i -hatj +hat k)` where `a_0` is a positive constant. Then the minimum pressure at the point will be
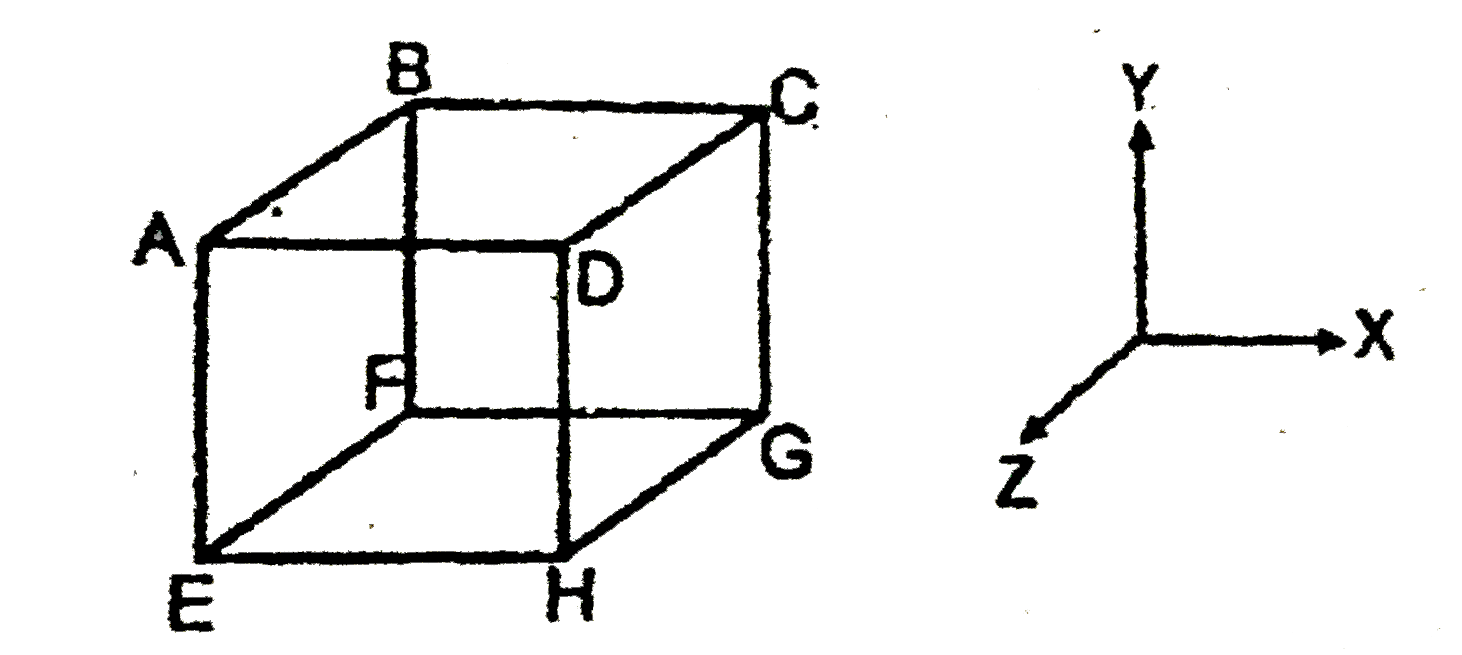
View SolutionPressure gradient in the horizontal direction in a static fluid is represented by (z-direction is vertically upwards, and x-axis is along horizontal, d is density of fluid):
View SolutionAn open tank `10 m` long and `2 m` deep is filled up to `1.5 m` height of oil of specific gravity `0.82`. The tank is uniformly accelerated along its length from rest to a speed of `20 m//s` horizontally. The shortest time in which the speed may be attained without spilling any oil is
View SolutionFollowing are some statements about buoyant force: (Liquid is of uniform density)
(i) Buoyant force depends upon orientation of the concerned body inside the liquid. (ii) Buoyant force depends upon the density of the body immersed. (iii) Buoyant force depends on the fact whether the system is on moon or on the earth. (iv) Buoyant force depends upon the depth at which the body (fully immeresed in the liquid) is placed inside the fluid. Of these statements:
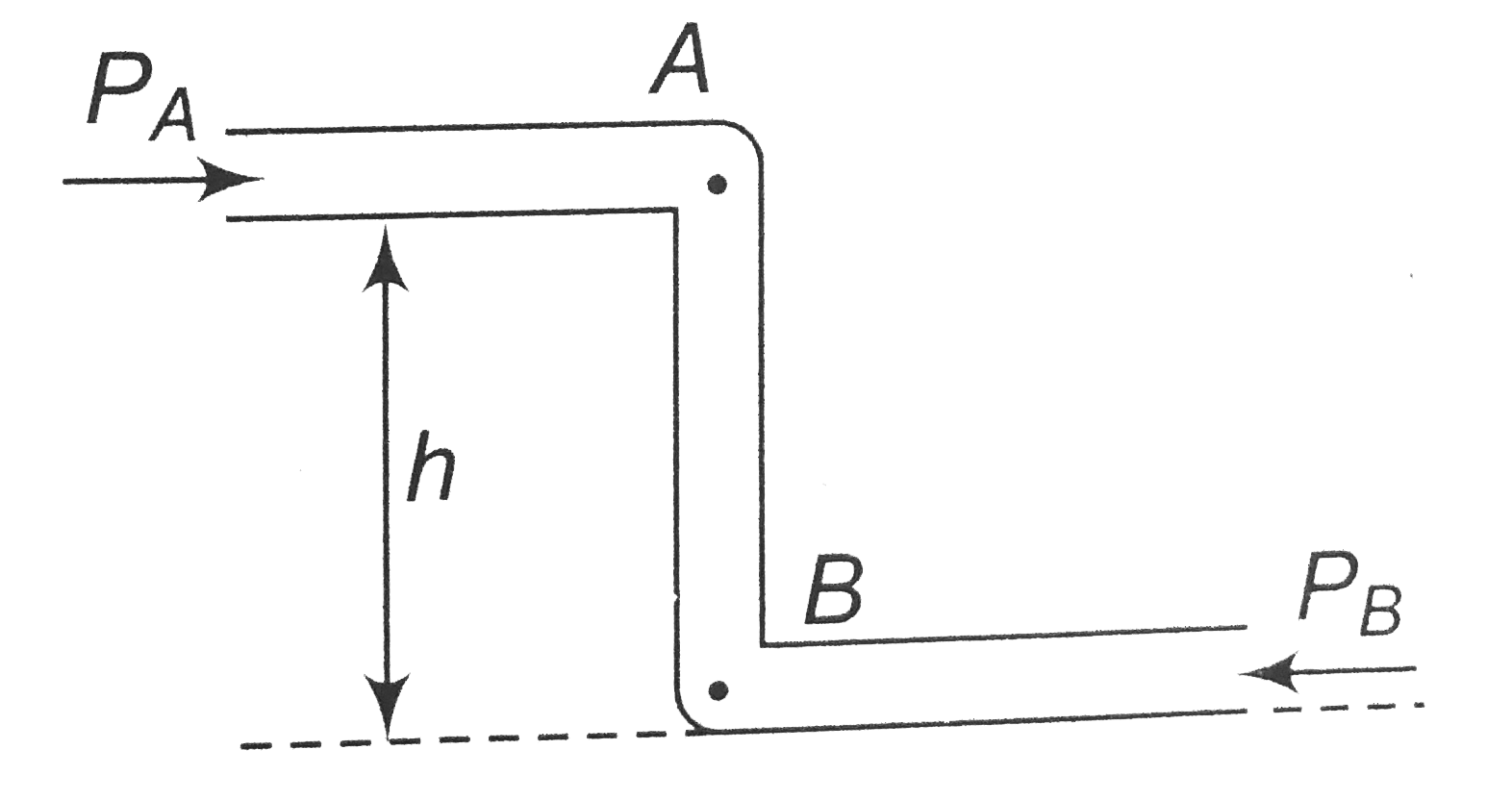
View SolutionFigure shows an ideal fluid flowing through a uniform cross-sectional tube in the vertical tube with liquid velocities `v_(A)` and `v_(B)` and pressure `P_(A)` and `P_(B)`. Knowing that offers no resistance to fluid flow then which of the following is true.
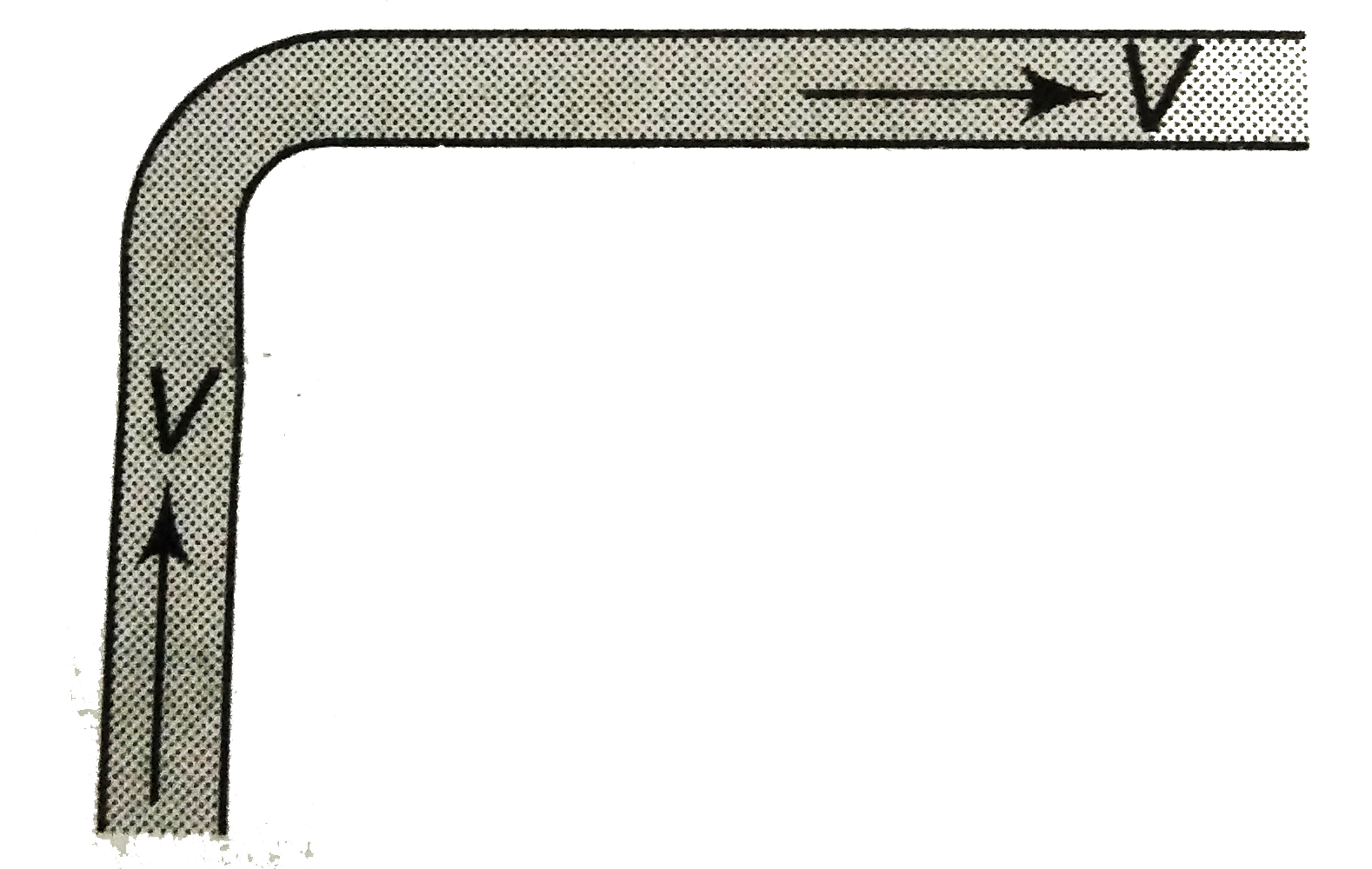
View SolutionA fire hydrant delivers water of density `rho` at a volume are `L`. The water travels vertically upward through the hydrant and then does `90^(@)` turn to emerge horizontally at speed `V`. The pipe and nozzle have uniform cross-section through out. The force exerted by the water on the corner of the hydrant is
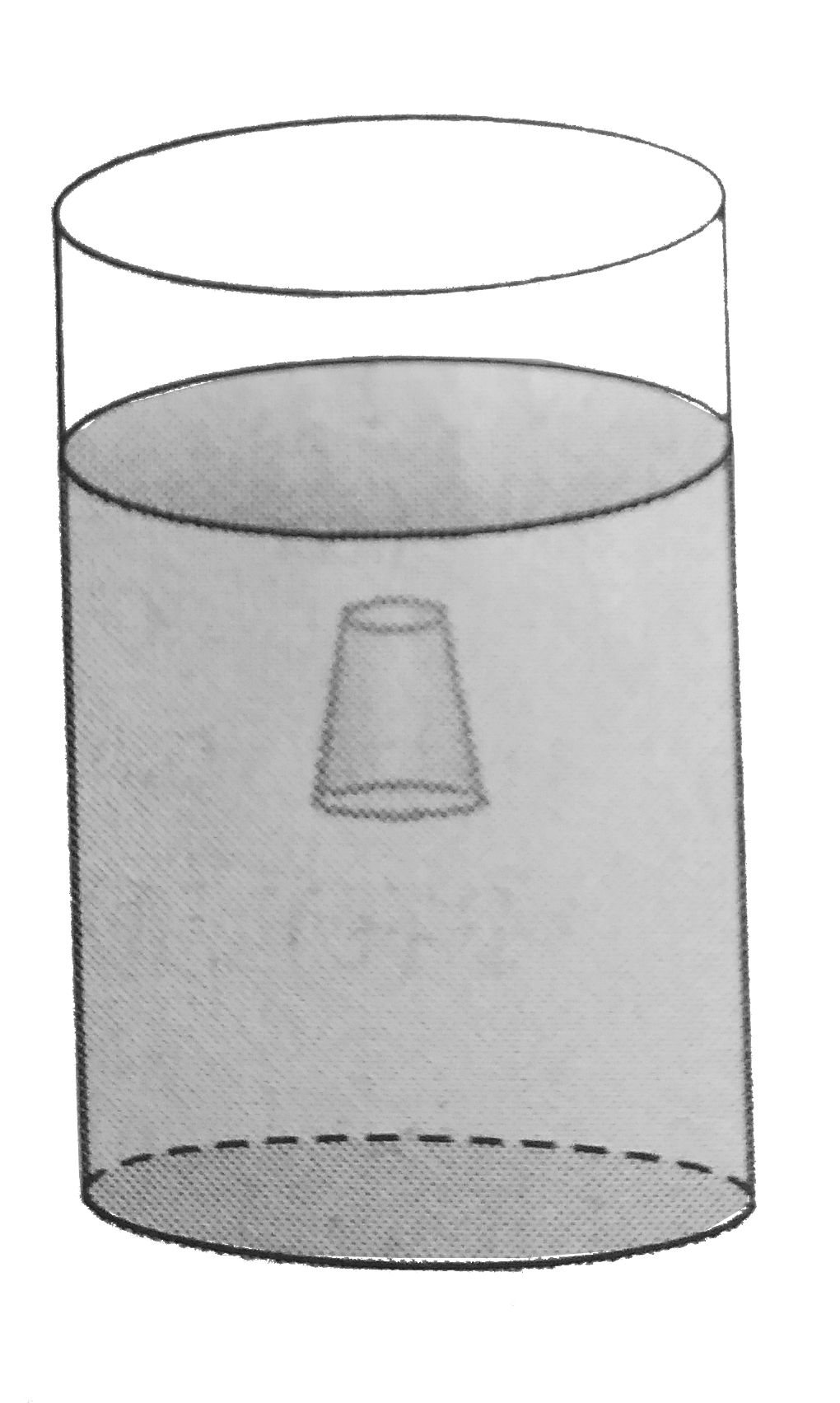
View SolutionAn empty glass jar is submerged in tank of water with open mouth of the jar downwards, so that air inside the jar is trapped and cannot get out. As the jar is pushed down slowly, the magnitude of net buoyant force on the system of volume of gas trapped in the jar and the jar.
View SolutionWhen a hole is made in the side of a container holding water, water flows out and follows a parabolic trajectory. If a hole is made in the side of the container and the container is dropped in free fall (just before the water starts coming out), the water flow (Neglect effect of surface tension)

View SolutionFigure shows a closed container completely filled with an ideal liquid of density `rho`. In the liquid there is a spherical body of volume V and density `sigma` attached to a string whose other end is attached to the roof of the container. The container is accelerating with an acceleration 'a' towards right. The force exerted by the liquid on the spherical body when it is in equillibrium with respect to the liquid, will be :
View SolutionA horizontal pipe carries water in the positive direction of x. Small identical holes are made at three different places along the pipe such that streams of water from them come out vertically upwards. The height upto which the water stream rise from the three holes
View SolutionA mosquito with 8 legs stands on water surface and each leg makes depression of radius a. If the surface tension and angle of contact are T and zero respectively , then the weight of mosquito is
View SolutionAn isolated and charged spherical soap bubble has a radius ‘ r ‘ and the pressure inside is atmospheric. If ‘ T ‘ is the surface tension of soap solution, then charge on drop is:
View SolutionA spherical drop of water has 2.5 mm radius. If the surface tension of water is `70 xx 10^(-3)` N `m^(-1)`, then the excess pressure inside the drop is
View Solution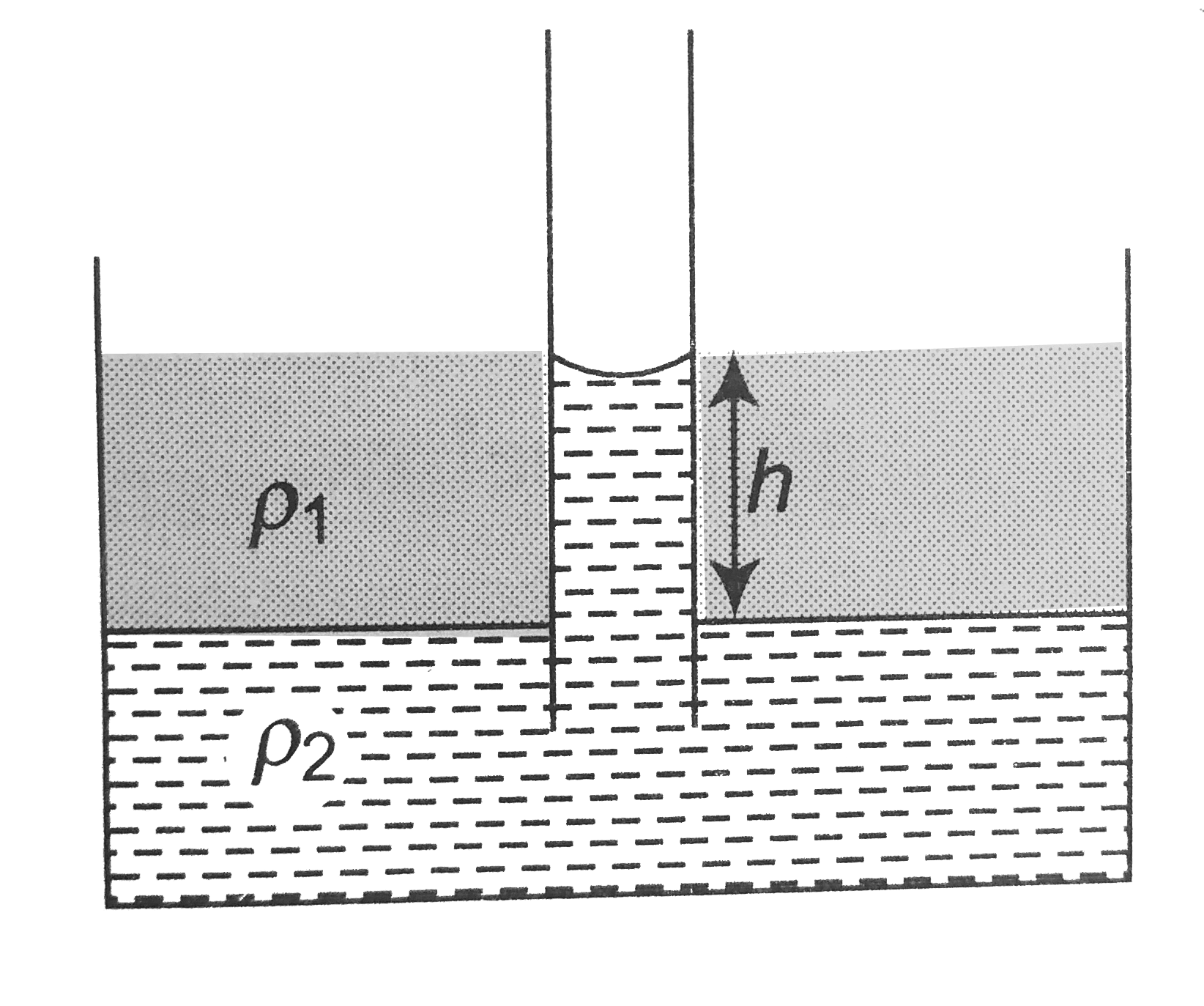
A container is partially filled with a liquid of density `rho_2` A capillary tube of radius r is vertically inserted in this liquid. Now another liquid of density `rho_1(rho_1ltrho_2)` is slowly poured in the container to a height h as shown. There is only denser liquid in the capillary tube. The rise of denser liquid in the capillary tube is also `h`. Assuming zero contact angle, the surface tension of heavier liquid is
View SolutionA space 2.5cm wide between two large plane surfaces is filled with oil. Force required to drag a very thin plate of area `0.5m^2` just midway the surfaces at a speed of `0.5m//sec` is 1N. The coefficient of vescosity in `kg-s//m^2` is:

View SolutionA sphere of mass m and radius r is projected in a gravity free space with speed v. If the coefficient of viscosity of the medium in which it moves is `1//6pi` Pa s, the distance travelled by the body before it stops is :
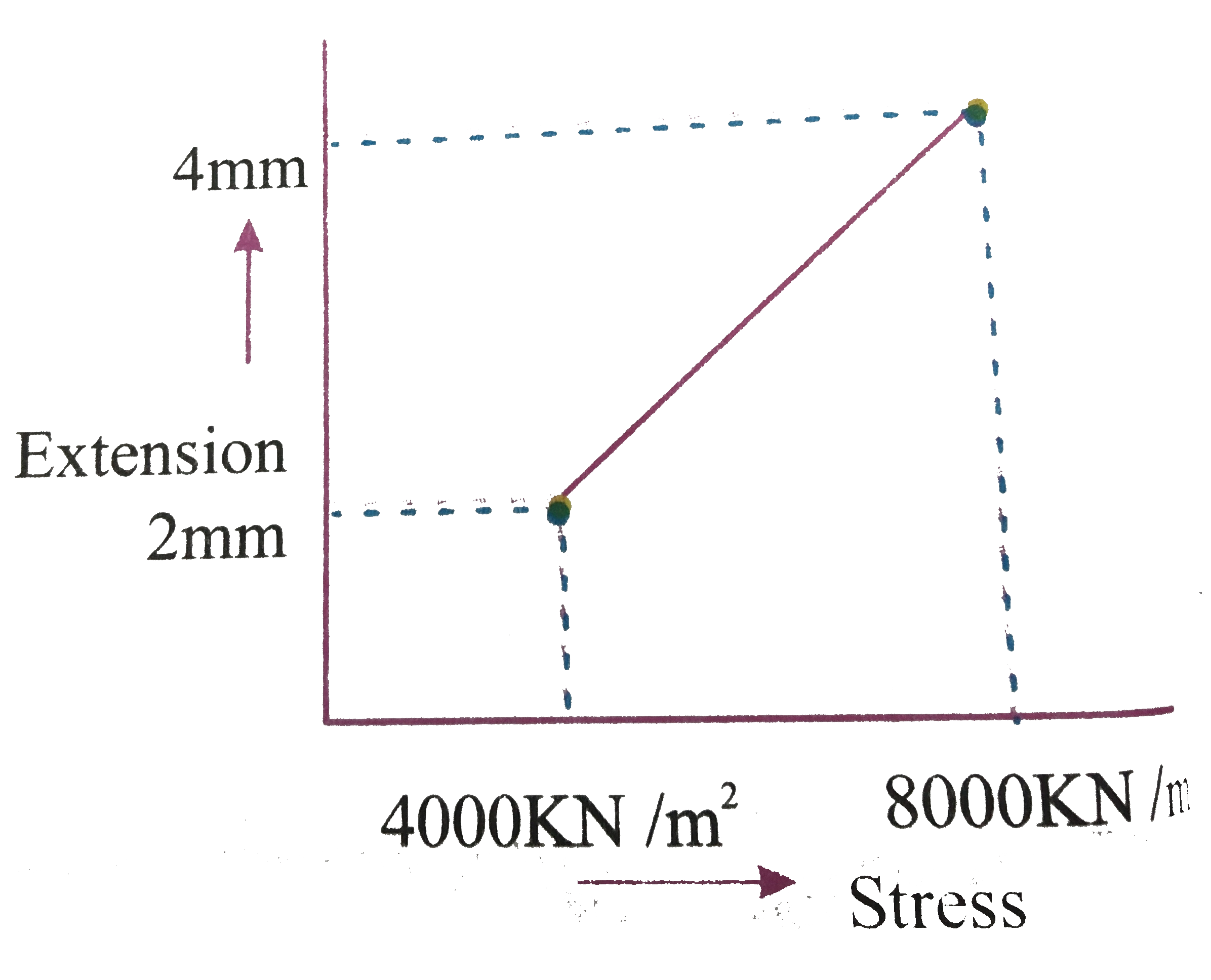
View SolutionIn determination of young modulus of elasticity of wire, a force is applied and extension is recorded. Initial length of wire `1m` . The curve between extension and stress is depicted then young modulus of wire will be:
View SolutionA steel rod of length `1 m` is heated from `25^@ "to" 75^@ C` keeping its length constant. The longitudinal strain developed in the rod is ( Given, coefficient of linear expansion of steel = `12 xx 10^-6//^@ C`).
View Solution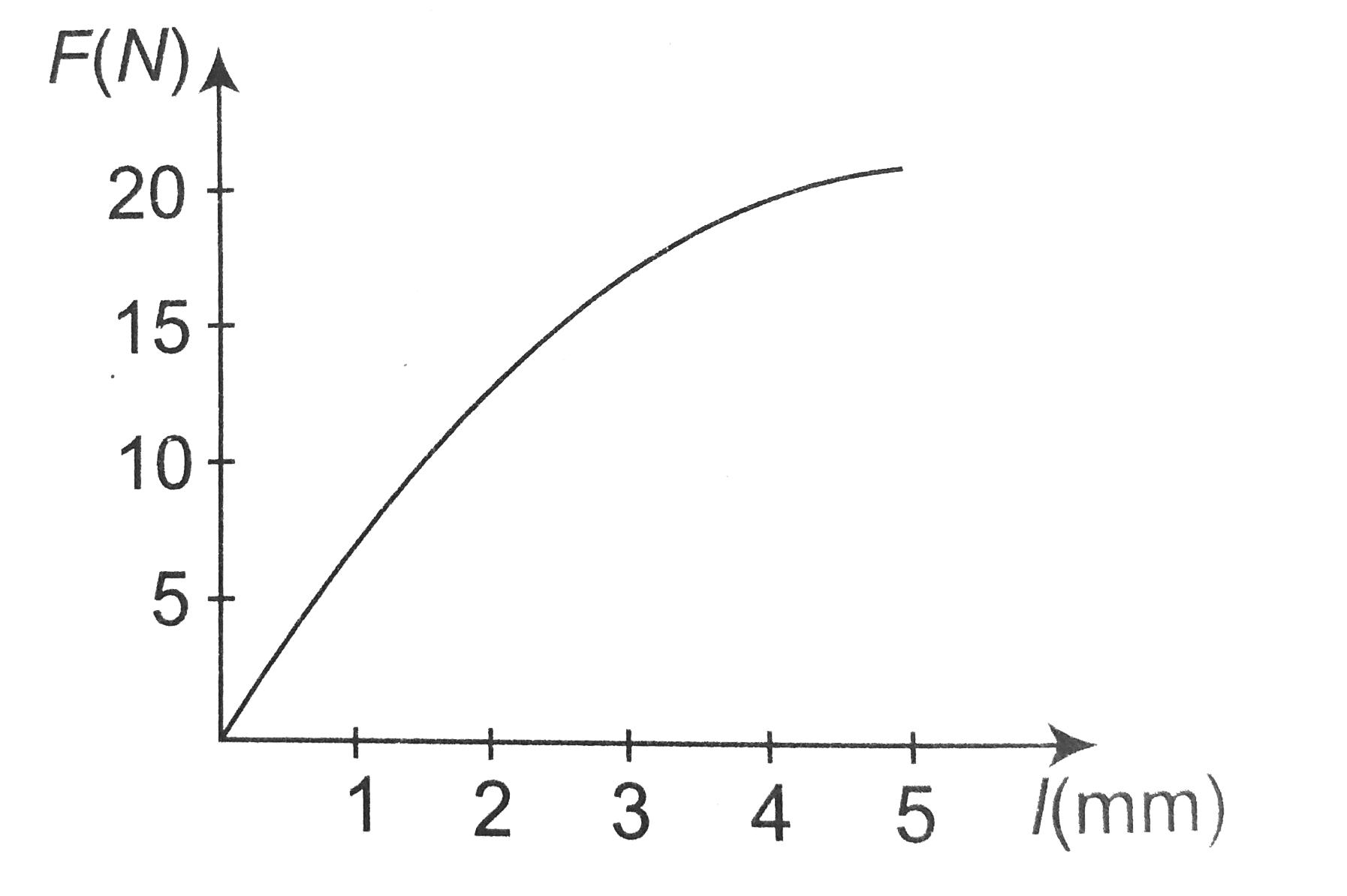
The force `(F)`- extension `(lamda)` graph shows that the strain energy stored in the material under test, for an extension of `4mm`, is greater than which of the following values?
View SolutionState true/false:
`S_1` : Hydrostatic pressure is a scalar quantity even though pressure is force divided by area and force is a vector quantity?
`S_2`: A barometer made of a very narrow tube (see figure) is placed at normal temperature and pressure. The coefficient of volume expansion of mercury is `0.00018//.^@C` and that of the tube is negligible. The temperature of mercury in the barometer is now raised by `1^@C` but the temperature of the atmosphere does not change. Then, the mercury height in the tube remains unchanged.
`S_3` A block of ice with a lead shot embedded in it is floating on water contained in a vessel. The temperature of the system is maintained at `0^@C` as the ice melts. When the ice melts completely the level of water in the vessel rises.
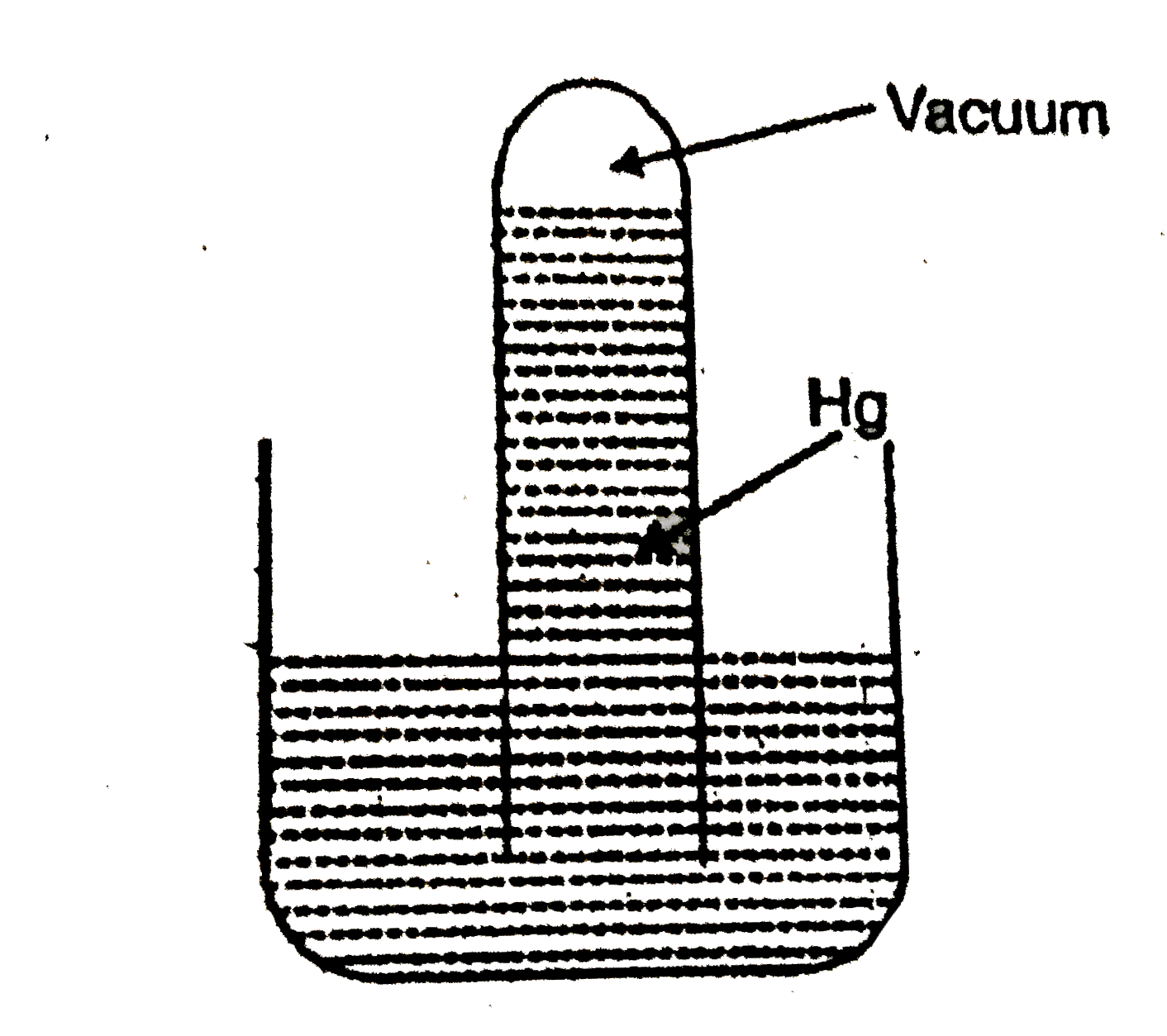
View SolutionA solid sphere of radius r made of a soft material of bulk modulus K is surrounded by a liquid in a cylindrical container. A massless piston of area a floats on the surface of the liquid, covering entire cross section of cylindrical container. When a mass m is placed on the surface of the piston to compress the liquid, the fractional decrement in the radius of the sphere, `((dr)/(r ))`, is :
View SolutionA horizontal pipeline carries water in a streamlime flow, At a point along the pipe, where the cross-sectional area is `10cm^(2)`, the water velocity is `1ms^(-1)` and the pressure is `2000 Pa`. The pressure of water at another point where the cross-sectional area is `5cm^(2)`, is .....Pa. (Density of water `=10^(3)kg-m^(3))`.
View SolutionWhat will be the stress required to double the length of a wire of Young's modulus Y?
View SolutionAn air bubble in a water tank rises from the bottom to the top. Which of the following statements are true?
View SolutionWhich of the following is possible?
View SolutionA block of density `2000 kg//m^(3)` and mass 10 kg is suspended by a spring of force constant `100 N//m`. The other end of the spring is attached to a fixed support. The block is completely submerged in a liquid of density `1000 kg//m^(3)`. If the block is in equilibrium position.
View Solution_S01_017_Q01.png)
An open water tanker moving on a horizontal straight road has a cubical block of cork floating over its surface if the tanker has an acceleration of `a` as shown, the acceleration of the cork w.r.t container is ignore viscosity.
View SolutionAssuming the xylem tissues through which water rises from root to the branches in a tree to be of uniform cross-section find the maximum radius of xylem tube in a `10m` high coconut tree so that water can rise to the top.
(Surface tension of water`=0.1(N)/(m)`, Angle of contact of water with xylem tube`=60^@`)
View SolutionWater is moving with a speed of `5.18 ms^(-1)` through a pipe with a cross-sectional area of `4.20 cm^(2)`. The water gradually descends 9.66 m as the cross-sectional area of pipe is increased to `7.60 cm^(2)`. The speed of the flow of water at lower level is
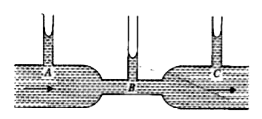
View SolutionIn the Fig. 15.5.2 below in shown the flow of liquid through a horizontal pipe. Three tubes A, B and C are connected to the pipe. The radii of the tube, A, B and C at the junction are respectively 2 cm, 1 cm , and 2 cm. It can be said that the
View SolutionA body is just floating on the surface of liquid. The density of the body is same as that of the liquid. The body is slightly pushed down. Then it will
View SolutionThe surface tension the surface tension of a liquid is 10 N/m. If a film is held on a ring of area 0.01 m square, its surface energy is about Newton
View SolutionExcess pressure in a soap bubble of radius r is proportional to:
View SolutionWater rises in a vertical capillary tube up to a length of 10 cm. If the tube is inclined at `45^@`, the length of water risen in the tube will be
View SolutionRadius of a capillary is `2xx10^(-3)m`. A liquid of weight `6.28xx10^(-4)N` may remain in the capillary, then the surface tension of liquid will be:
View SolutionWater rises to a height of 16.3 cm in a capillary of height 18 cm above the water level. If the tube is cut at a height of 12 cm,
View Solution
Exercise questions and complete solutions for chapter FLUID MECHANICS of RESONANCE ENGLISH of Class 11
All Exercises from RESONANCE Class 11 PHYSICS FLUID MECHANICS
- Advanced Level Problems
- Board Level Exercise
- Examples
- Exercise
- Exercise- 1 PART - I Section (B)
- Exercise- 1 PART - I Section (A)
- Exercise- 1 PART - I Section (C)
- Exercise- 1 PART - II Section (A)
- Exercise- 1 PART - II Section (B)
- Exercise- 1 PART - II Section (C)
- Exercise- 2 PART - I
- Exercise- 2 PART - II
- Exercise- 2 PART - III
- Exercise- 2 PART - IV
- Exercise- 3 PART - I
- Exercise- 3 PART - II