Complete Solutions to dpp 51 of chapter DAILY PRACTICE PROBLEMS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | DAILY PRACTICE PROBLEMS |
| RESONANCE | dpp 51 |
| Number Of Questions | 7 |
| Academic Year | 2023-24 |
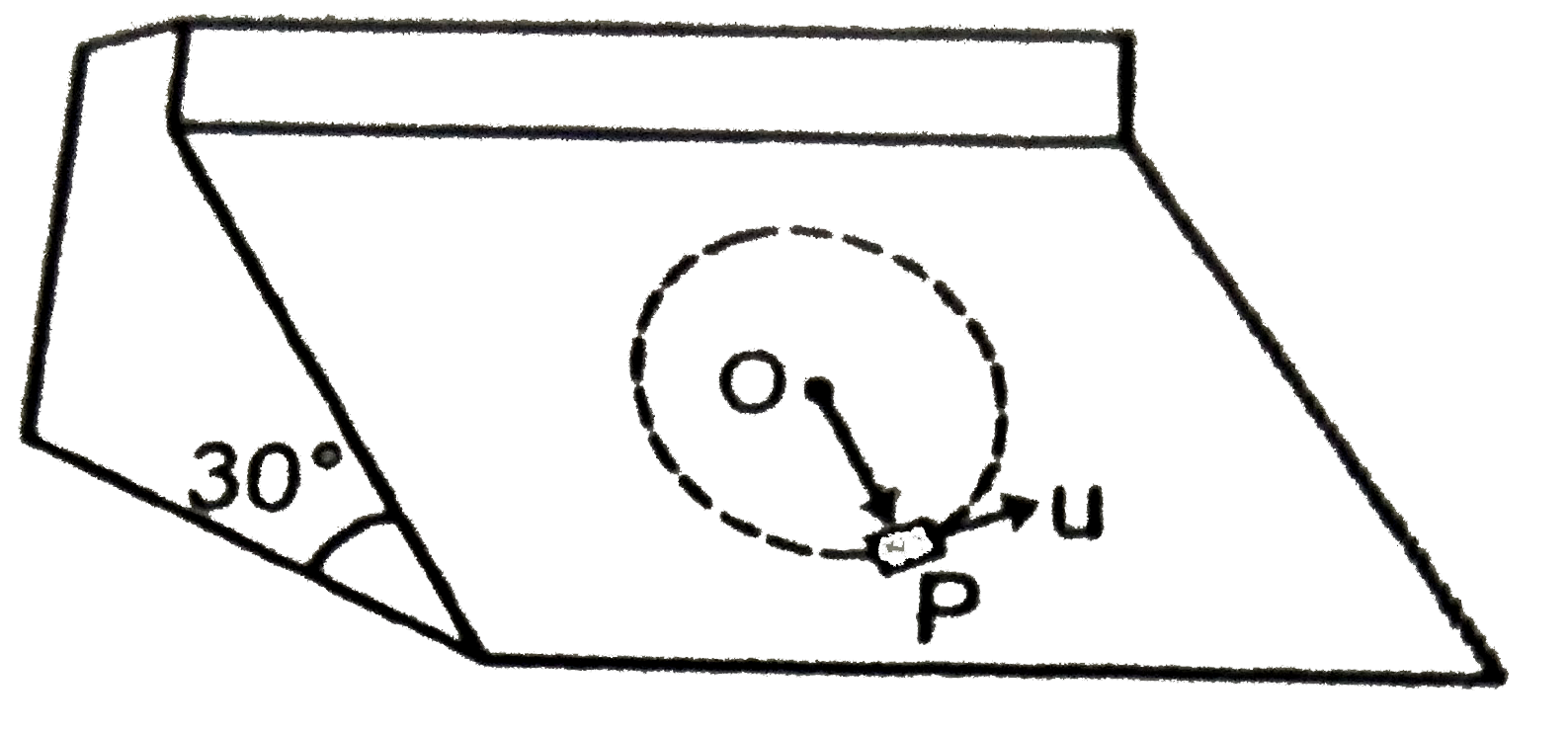
A particle is attached with a string of length `l` which is fixed at point `O` on an inclined plane what minimum velocity should be given to the particle along the incline so that it may complete a circle on inclined plane `(` plane is smooth and initially particle was resting on the inclined plane . `)`
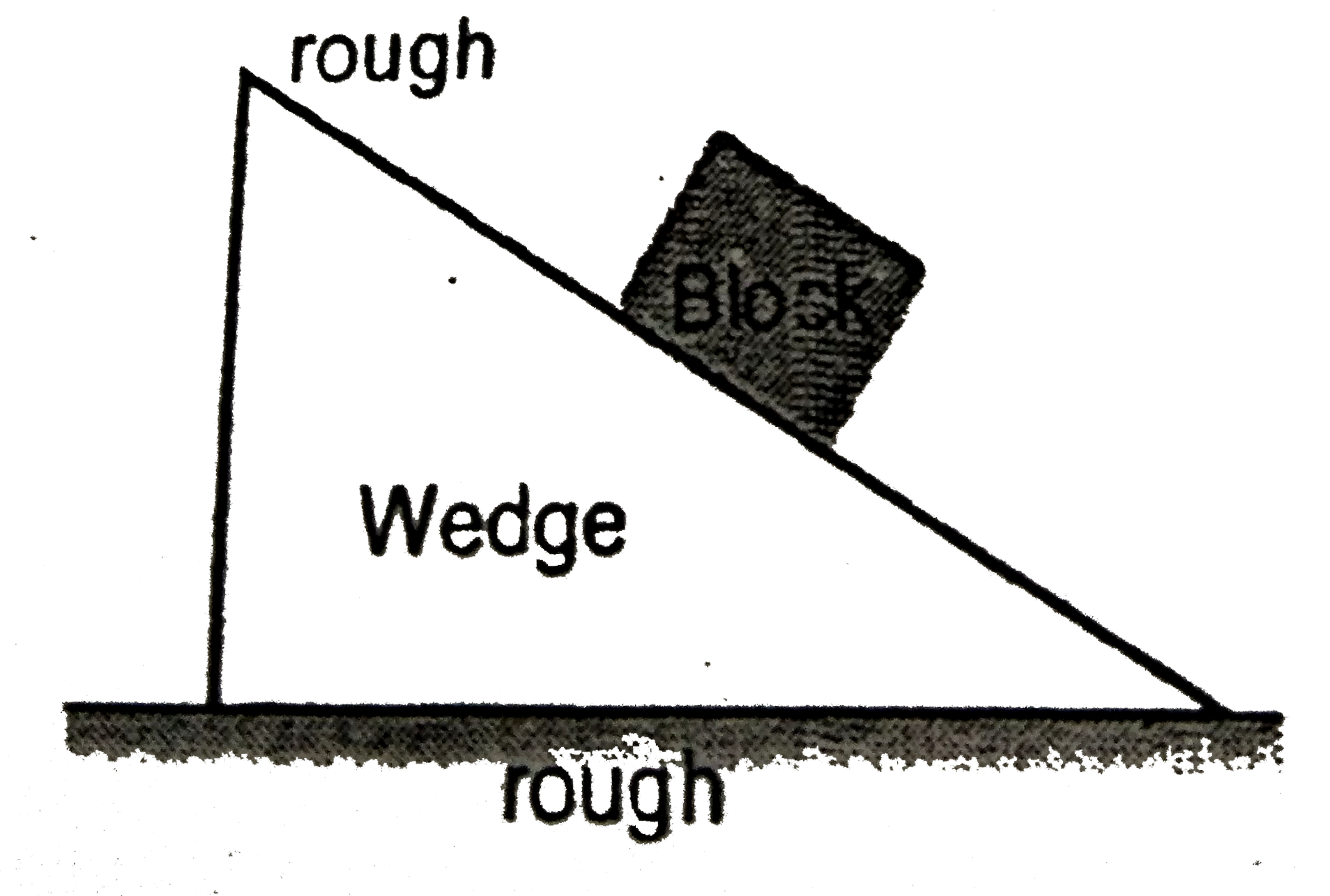
View SolutionWhen a block is placed on a wedge as shown in figure, the block starts sliding down and the wedge also start sliding on ground. All surfaces are rough. The centre of mass of `(` wedge `+` block `)` system will move.
View SolutionA shell of mass `4kg` moving with a velocoity `10m//s` vertically upward explodes into three parts at a height `50m` from ground. After three seconds, one part of mass `2kg` reaches ground and another part of mass `1kg` is at height `40m` from ground. The height of the third part from the ground is : `[g=10m//s^(2)]`
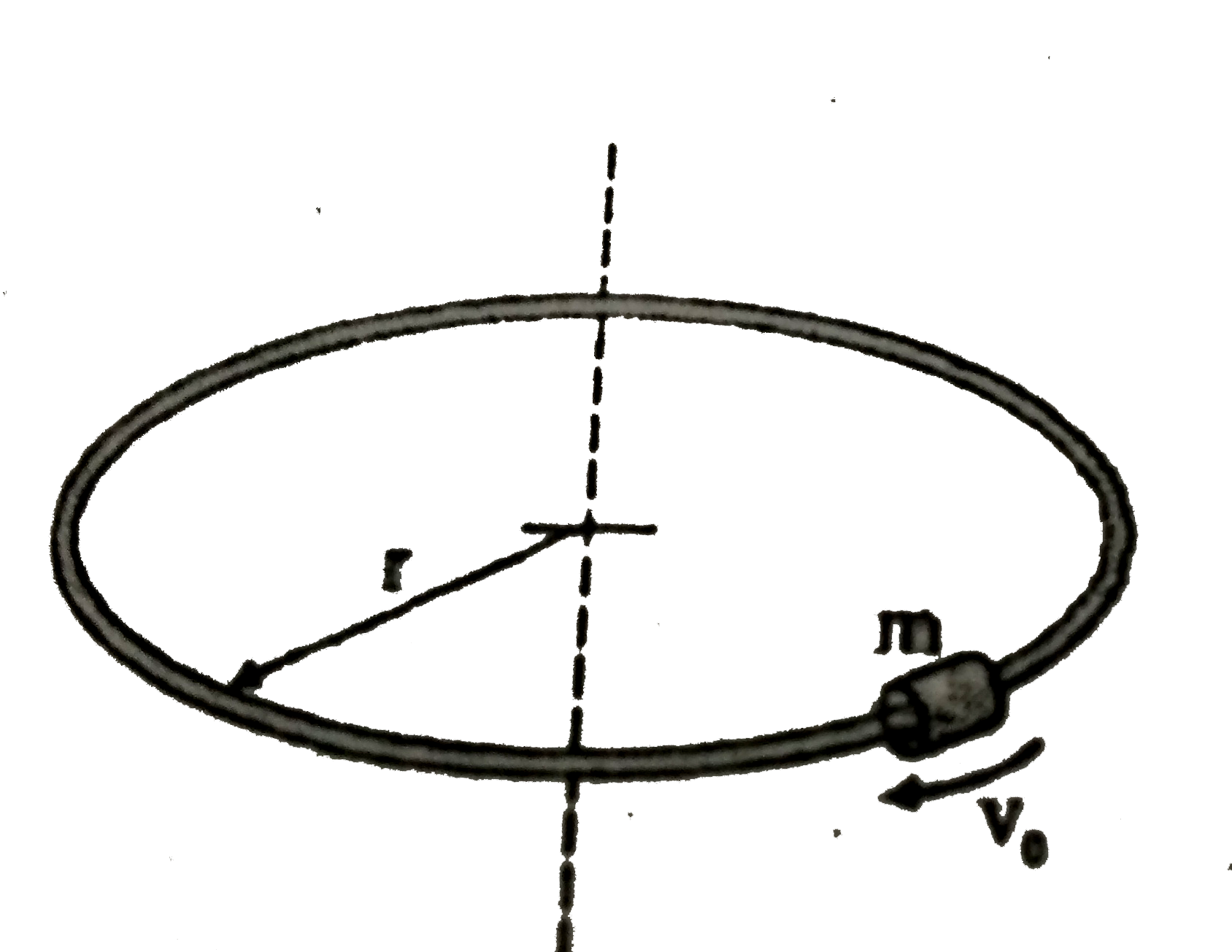
View SolutionA small hoop of mass `m` is given an initial velocity of magnitude `v_(0)` on the horizontal circular ring of radius `'r'`. If the coefficient of kinetic friction is `mu_(k)` the tangential acceleration of the hoop immediately after is release is `(` assume the horizontal ring to be fixed and not in contact with any supporting surface `)`
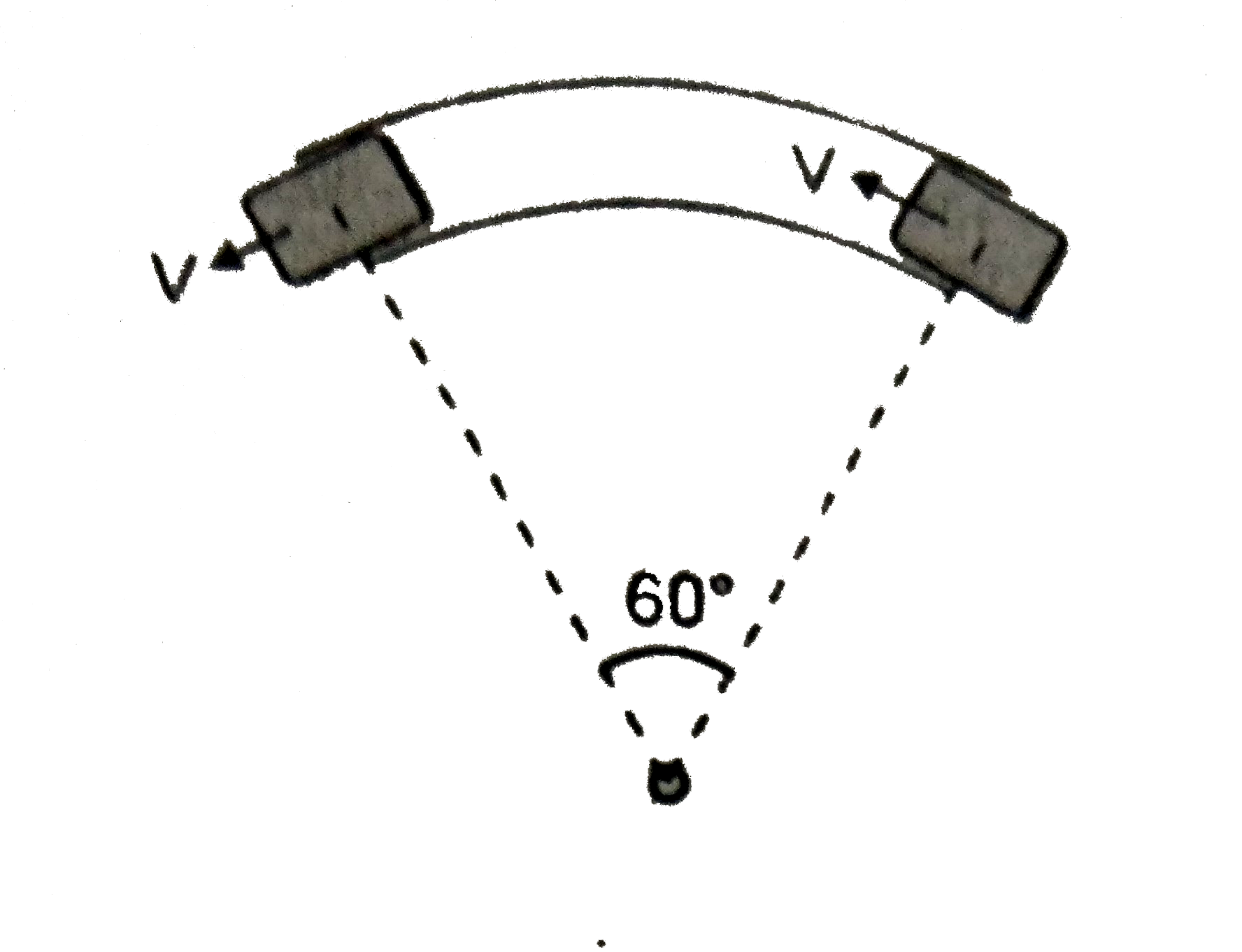
View SolutionA car moves around a curve at a constant speed. When the car goes around the arc subtending `60^(@)` at the centre, then the ratio of magnitude of instantaneous acceleration to average acceleration over the `60^(@)` arc is `:`
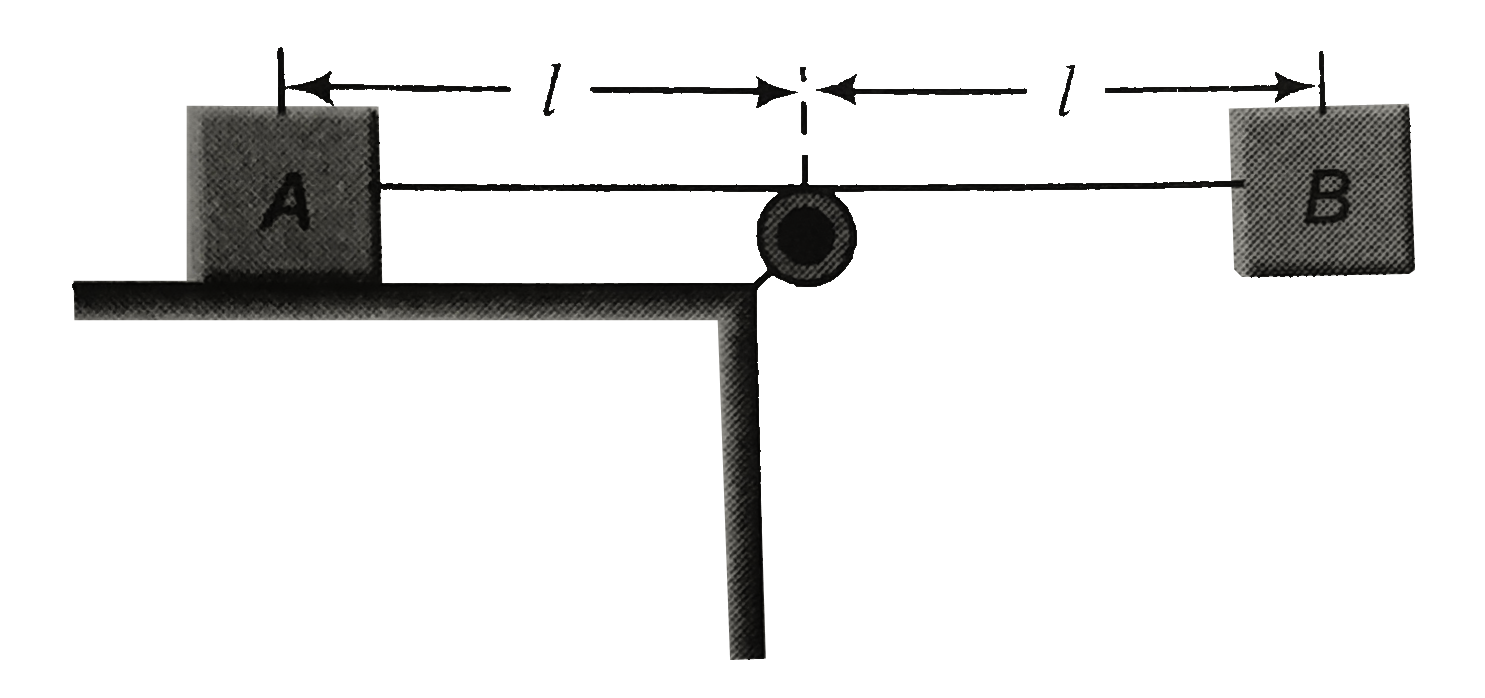
View SolutionTwo blocks `A`and `B` ,each of same mass are attached by a thin inextensible string through an ideal pulley .Initially block `B` is held in position as shown in figure.Now , the block `B` is released. Block `A` will slide to right and hit the pulley in time `t_(A)`. Block B swing and hit the surface in time `t_(B)`. Assume the surface as frictionless ,then
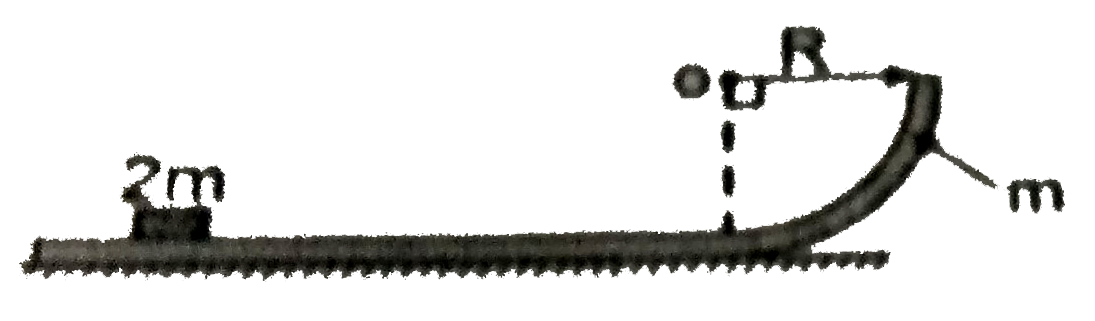
View SolutionMass `2m` is kept on a smooth circular track of mass `m` which is kept on a smooth horizontal surface. The circular track is given a horizontal velocity `sqrt(2gR)` towards left. Find the maximum height reached by `2m.`
View Solution
dpp 51 questions and complete solutions for chapter DAILY PRACTICE PROBLEMS of RESONANCE ENGLISH of Class 11
All Exercises from RESONANCE Class 11 PHYSICS DAILY PRACTICE PROBLEMS
- comprehension
- Compreshension
- dpp 15
- dpp 16 physics
- dpp 17
- dpp 18
- dpp 19
- dpp 2 PHYSICS
- dpp 20
- dpp 21
- dpp 22.
- dpp 24
- dpp 25
- dpp 26
- dpp 27
- dpp 28
- dpp 30
- dpp 31
- dpp 32
- dpp 33
- dpp 34
- DPP 35
- dpp 36
- Dpp 37
- dpp 38
- dpp 39
- dpp 40
- dpp 41
- dpp 42
- dpp 43
- dpp 44
- DPP 45
- dpp 46
- dpp 47
- dpp 48
- dpp 49
- DPP 50
- dpp 51
- dpp 52
- dpp 53
- dpp 54
- DPP 55
- dpp 56
- dpp 57
- dpp 58
- dpp 59
- dpp 60
- DPP 62
- dpp 63
- dpp 64
- DPP 65
- dpp 66
- dpp 67
- dpp 68
- dpp 69
- dpp 70
- dpp 71
- dpp 72
- dpp 74
- dpp 75
- dpp 76
- DPP 77
- dpp 78
- dpp 79
- DPP 8 PHYSICS
- dpp 80
- dpp 81
- dpp 82
- dpp 83
- dpp 84
- dpp 85
- dpp 86
- dpp 87
- dpp 88
- dpp 89 illustration
- dpp 90 Illustration
- dpp 91 illustration
- dpp 92 illustration
- Dpp no 10 physics
- Dpp no 11 physics
- Dpp no 12 Physics
- Dpp no 6 physics
- DPP NO 7 PHYSICS
- DPP no 9 physics
- Dpp no. 13 physics
- DPP NO. 14 PHYSICS
- DPP NO. 5 Physics
- dpp23
- dpp29
- dpp61
- dpp73
- Now answer the following :
- PHYSICS
- TRUE/FALSE