Complete Solutions to Problem of chapter ROTATIONAL MOTION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | ROTATIONAL MOTION |
| SL ARORA | Problem |
| Number Of Questions | 24 |
| Academic Year | 2023-24 |
An isolated particle of mass m is moving in a horizontal plane (x-y), along the x-axis at a certain height above the ground. It suddenly explodes into two fragments of masses `m//4` and `3m//4`. At instant later, the smaller fragment is at `y = +5 cm`. What is the position of larger fragment at this instant ?
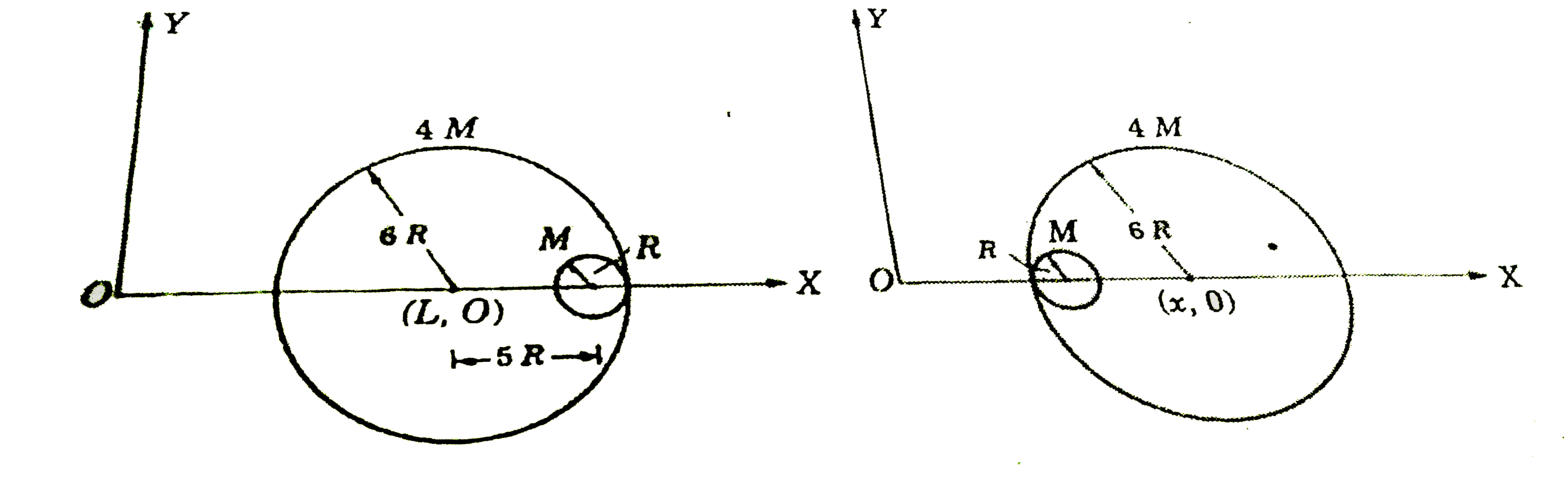
View SolutionA small sphere of radius R is held against the inner surface of `alpha` larger sphere of radius 6R. The masses of large and small spheres are 4M and M, respectively. This arrangement is placed on a horizontal table. There is no friction between any surfaces of contact. The small sphere is now released. find the co-ordinations of the centre of the larger sphere when the smaller sphere reaches the other extreme position.
View SolutionA boat of `90 kg` is floating in still water. A boy of mass 30 kg walks from the stern to the bow. The length of the boat is 3 m. Calculate the distance through which the boat will move.
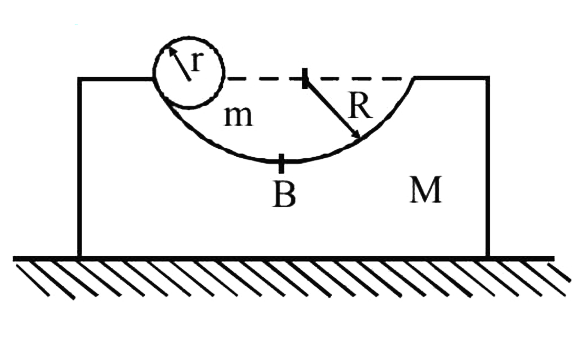
View SolutionA block of mass M with a semicircualr of radius R, rests on a horizontal frictionless surface. A uniform cylinder of radius r and mass m is released from rest the top point A The cylinder slips on the semicircular frictionless track. How far has the block moved when the cylinder reaches the bottom (point B) of the track ? How fast is the block moving when the cylinder reaches the bottom of the track?
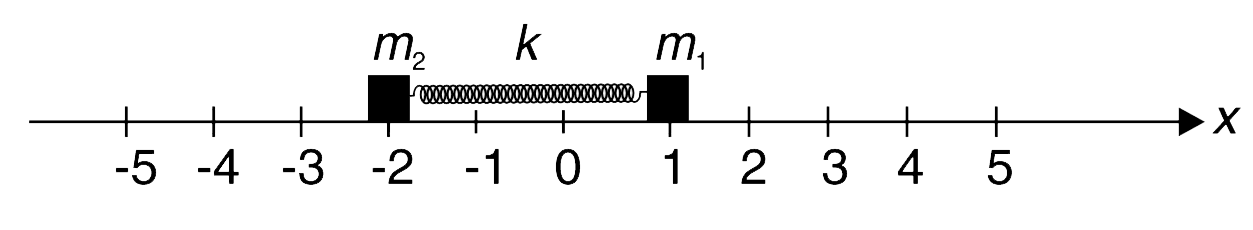
View SolutionTwo blocks of mass `m_(1) and m_(2)` are connected to the ends of a spring. The spring is held compressed and the system is placed on a smooth horizontal table. The block of mass `m_(1) = 2 kg` is kept at `x = 1 cm` mark and the other block is at `x = 2 cm` mark. The system is released from this position. It was observed that at the instant `m_(1)` was at `x = 5 cm` mark its velocity was zero and at that moment `m_(2)` was located at `x = -4 cm`. Find mass `m_(2)` and unstretched length `(l_(0))` of the spring
View SolutionFrom a uniform circular disc of diameter D, a circular disc or hole of diameter `D//6` and having its centre at a distance of `D//4` from the centre of the disc is scopped out. Determine the centre of mass of the remaining portion.
View SolutionFind the coordination of the centre of mass of a uniform semicircular wire of radius R and mass M.
View SolutionDetermine the centre of mass of a uniform hemisphere of radius R.
View SolutionDetermine the position of the centre of mass of a hemisphere of radius R.
View SolutionDetermine the coordinate of the centre of mass of a right circular solid cone of base radius R and height h.
View SolutionThe driver of a car travelling at speed v suddenly sees a wall at a distance r directly infront of him. To avoid collision, should he apply the brakes or turn the car sharply away from the wall ? Give reason.
View SolutionA very small particle rests on the top of a hemisphere of radius 20 cm. Calculate the smallest horizontal velocity to be given to it if it is to leave the hemisphere without sliding down its surface. Take `g = 9.8 ms^(-2)`.
View SolutionA tube of length L is filled completely with an incompressible liquid of mass M and closed at both the ends. The tube is then rotated in a horizontal plane about one of its ends with a uniform angular velocity `omega`. Determine the force exerted by the liquid at the other end.
View SolutionA particle describes a horizontal circle on the smooth surface of an inverted cone. The height of the plane of the circle aove the vertex is 9.8 cm. Find the speed of the particle. Take `g = 9.8 ms^(-2)`.
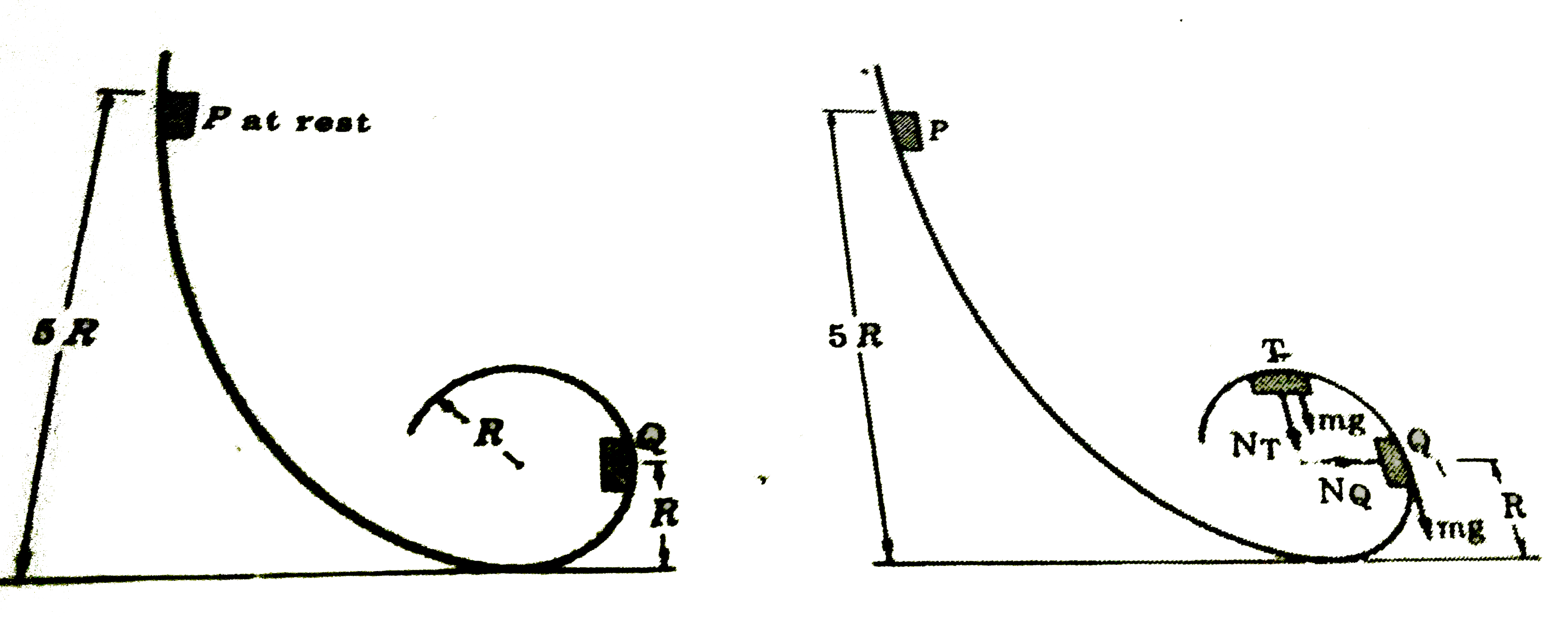
View SolutionA small block of mass m slides along a smooth track, as shown in fig. (i) If it starts from rest at P, what is the resulting force acting on it at Q ? (ii) at what height above the bottom of the loop should the block be released so that the force it exerts against the track at the top of the loop equals its weight?
View SolutionPoint masses `m_(1)` and `m_(2)` are placed at the ends of a rigid rod of length L and negligible mass. The rod is to be set rotating about an axis perpendicular to its length. Locate a point on the rod through which the axis of rotation should pass in order that the work required to set the rod rotating with angular velocity `omega_(0)` is minimum.
View SolutionA thin uniform bar lies on a frictionless horizontal surface and is free to move in any way on the surface. Its mass is 0.16 kg and lengths is `sqrt(3)m`. Two particles, each of mass `0.08 kg`, are moving on the same surface and towards the bar in a direction perpendicular to the bar, one with a velocity of `10 ms^(-1)` and the other with `6ms^(-1)`, as shown in fig. The first particle strikes the bar at point A and the other at point B. Points A and B are at a distance 0.5 m from the centre of the bar. The particles strike the bar at same instant of time and stick to the bar on collision. Calculate the loss of kinetic energy of the system in the above collision process.

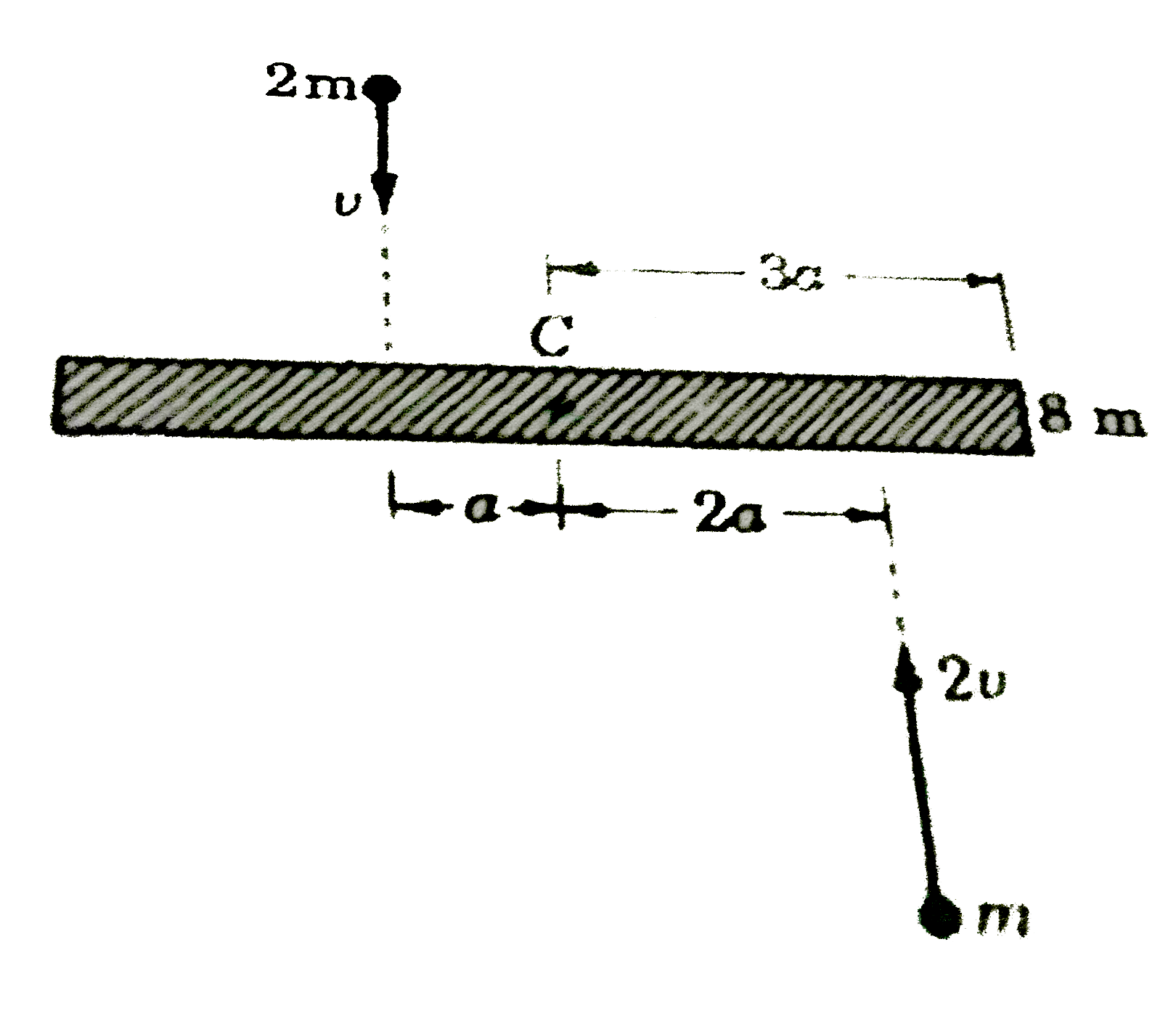
View SolutionA uniform bar of length 6a and mass 8 m lies on a smooth horizontal table. Two point-masses m and 2m moving in the same horizontal plane with speeds 2v and v respectively strike the bar as shown in Fig, and stick to the bar after collision.
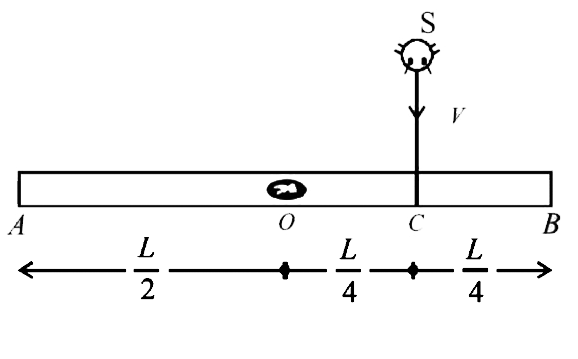
View SolutionA homogeneous rod AB of length L = 1.8 m and mass M is pivoted at the center O in such a way that it can rotate it can rotate freely position. An insect S of the same mass M falls vertically with speed V on the point C, midway between the points O and B. Immediately after falling, the insect moves towards the end B such that the rod rotates with a constant angular velocity omega.
(a) Determine the angular velocity `omega` in terms of V and L.
(b) If the insect reaches the end B when the rod has turned through an angle of `90^@`, determine V.
View SolutionA solid cylinder at rest at top of an inclined plane of height 2.7 m rolls down without slipping. If the same cylinder has to slide down a frictionless inclined plane and acquires the same velocity as that acquired by centre of mass of rolling cylinder at the bottom of the incline, what should be the height of inclined plane?
View SolutionA carpet of mass `M` is rolled along its length so as to from a cylinder of radius `R` and is kept on a rough floor. When a negligibly small push is given to the cylindrical carpet, it stars unrolling itself without sliding on the floor. Calculate horizontal velocity of cylindrical part of the carpet when its radius reduces to `R//2`.
View SolutionA particle of mass 3 kg is moving under the action of a central force whose potential energy is given by U(r) = 10r3 joule. For what energy and angular momentum will the orbit be a circle of radius 10 m ?
View SolutionA small sphere rolls down without slipping from the top of a track in a vertical plane. The track has an elevated section and a horizontal part, The horizontal part, is 1.0 metre above the ground lenvel and the top of the track is 2.4 meters above the ground. Find the distance on the ground with respect to the point B (which is vertically below the end of the track as shown i fig.) where the sphere lands. During its flight as a projectlie, does the sphere continue to rotate about its centre of mass? Explain.

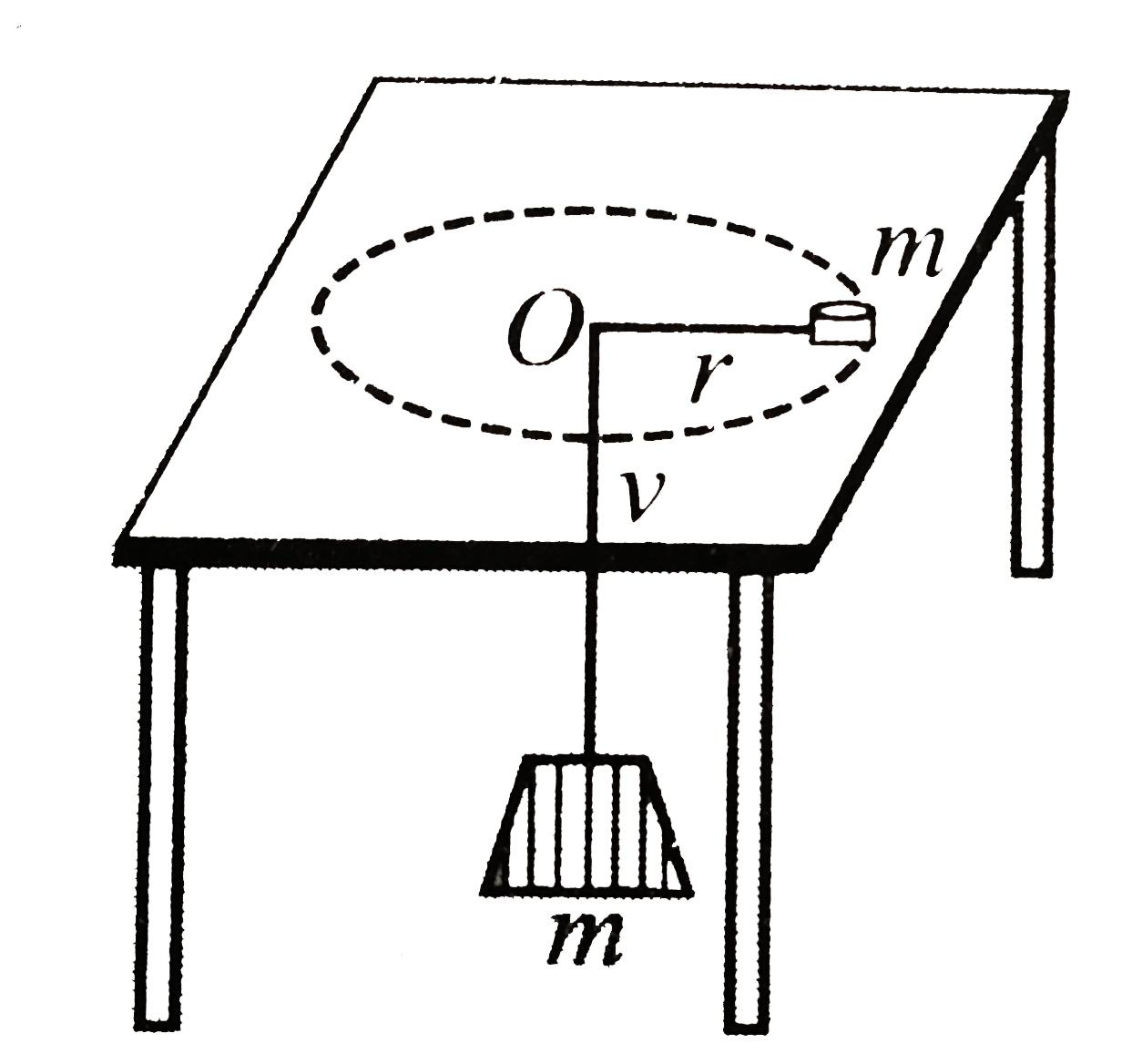
View SolutionA thread is passing through a hole at the centre of frictionless table. At the upper end a block of mass `0.5 kg` is tied and a block of mass `8.0 kg` is tied at the lower end which is freely hanging. The smaller mass is rotate, on the table with a constant angular velocity about the axis passing through the hole so as to balance the heavier mass. If the mass of the hanging block is changed from `8.0 kg` to `1.0 kg`, what is the fractional change in the radius and the angular velocity of the smaller mass so that it balances the hanging mass again?
View Solution
Problem questions and complete solutions for chapter ROTATIONAL MOTION of DHANPAT RAI & CO PHYSICS (HINGLISH) of Class 11