Complete Solutions to Problems for self practice of chapter THERMODYNAMICS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | THERMODYNAMICS |
| SL ARORA | Problems for self practice |
| Number Of Questions | 97 |
| Academic Year | 2023-24 |
An ideal monoatomic gas is taken around the cycle ABCDA, wher co-ordinates of A, B, C and D on and D on `P-V` diagram are A`(p,V), B(2p,V), C(2p, 2V) and D(p, 2V)`. Calculate work done during the cycle.
View SolutionCalculate net work done by the gas whose thermodynamical behaviour is represented by right angled triangle ABC on `P-V` diagram. The `P-V` diagram co-ordinates are : `A(20, 6), B(10, 12)` and `C(10, 6)` where `P` is in `Nm^(-2)` and `V` is in `m^(3)`
View SolutionOne mole of an ideal gas undergoes a cyclic change ABCD where the (P-V) co-ordinates are A(5, 1), B(5, 3), C(2, 3) and D(2, 1). P is in atmosphere and V is in litre. Calculate work done along AB , BC, CD and DA and also net work done in the process. Given 1 atmosphere `= 1.01 xx 10^(5) Nm^(-2)`.
View SolutionCalculate the change in internal energy of a block of copper of mass `200g` when it is heated from `25^(@)C t o 75^@C`. Given specific heat of copper `= 0.1 cal. g^(-1) .^(@)C^(-1)` and assume that change in volume is negligible.
View SolutionOne kg of water at `373K` is converted into steam at the same temperature. The volume `1 cm^(3)` of water becomes `1671 cm^(3)` on boiling. Calculate the change in internal energy of the system , if heat of vaporisation is `540 cal g^(-1)`. Given standard atmospheric pressure `=1.013xx10^(5) Nm^(-2)`.
View SolutionCalculate the change in internal energy when 5g of air is heated from `0^(@)` to `4^(@)C`. The specific heat of air at constant volume is `0.172 cal g^(-1) .^(@)C^(-1)`.
View SolutionA volume of `10m^(3)` of a liquid is supplied with `100 kal` of heat and expands at a constant pressure of `10 atm` to a final volume of `10.2m^(3)`. Calculate the work done and change in internal energy.
View SolutionThe internal energy of monoatomic ideal gas is 1.5 nRT. One mole of helium is kept in cylinder of cross section `8.5cm^2`. The cylinder is closed by a light frictionless piston. The gas is heated slowly in a process during which a total of 42 J heat is given to the gas. If the temperature rises through `2^@C` find the distance moved by the piston. Atmospheric pressure`=100kPa`
View SolutionA certain gas at atmospheric pressure is compressed adiabatically so that its volume becomes half of its original volume. Calculate the resulting pressure in `Nm^(-2)` . Given `gamma` for air = 1.4.
View SolutionA gas is suddenly compressed to `1/4th` of its original volume. Caculate the rise in temperature when original temperature is `27^(@)C. gamma= 1.5`.
View SolutionA tyre pumped to a pressure of `6 atmosphere` bursts suddenly. Calculate the temperature of escaping air. Given initial room temperature is `15^(@)C` and gamma for air is 1.4`.
View SolutionA tyre pumped to a pressure of 3 atmospheres suddenly bursts. Calculate the fall in temperature due to adiabatic expansion. The temperature of air before expansion is `27^(@)C` and value of `gamma = 1.4`.
View Solution`200 cm^(3)` of a gas is compressed to `100cm^(3)` at atmospheric pressure `(10^(6) "dyne"//cm^(2))`. Find the resultant pressure if the change is (i) slow (ii) sudden Take `gamma= 1.4`.
View SolutionA quantity of air at `27^(@)C` and atmospheric pressure is suddenly compressed to half its original volume. Find the final (i) pressure and (ii) temperature.
Given `gamma for air = 1.42`.
View SolutionA quantity of air at normal temperature is compressed (a) slowly (b) suddenly to one third of its volume. Find the rise in temperature, if any in each case, `gamma= 1.4`.
View SolutionA quantity of air is kept in a container having walls which are slightly conducting. The initial temperature and volume are `27^0C` (equal to the temperature of the surrounding) and `800cm^(3)` respectively. Find the rise in the temperature of the gas is compressed to `200 cm^(3)` (a) in a short time (b) in a long time . Take gamma= `1.4`.
View SolutionA litre of hydrogen at `127^(@)C` and `10^(6) dyne cm^(-2)` pressure expands isothermally until its volume is doubled and then adiabatically until its volume is redoubled. Find the final pressure of the gas. Given `gamma = 1.4`.
View SolutionDry air at `15^@ C` and `10 atm` is suddenly released at atmospheric pressure. Find the final temperature of the air `[C_p / C_v = 1.41]`.
View SolutionCalculate the rise in temperature when a gas, for which `gamma = 1.5` is compressed to 27 times its original pressure, assuming the initial temperature to be `27^(@)C`.
View Solution`1000 cm^(3)` of argon at `27^(@)C` is adiabatically compressed so that the temperature is `127^(@)C`. Calculate the resulting volume. Given `gamma = 5//3`.
View SolutionCalculate the work done if one mole of an ideal gas is compressed isothermally at a temperature `27^(@)C` from volume of 5 litres to 1 litre.
Given `R = 8.31 J mole ^(-1) K^(-1)`.
View SolutionOne kilogram molecule of a gas at 400 K expands isothermally until its volume is doubled. Find the amount of work done and heat produced.
Given `R = 8.3 xx 10^(-3) J ( kg mole )^(-1) K^(-1)`.
View SolutionFind the final value of a gram molecule of a gas after an isothermal expansion at `127^(@)C`, if the original volume is `400 c.c` Given amount of work done by a gram molecule of a gas during expansion is `2302.6 "joule", R= 8.3 "joule mole"^(-1)K^(-1)`
View SolutionOne gram mole of an ideal gas at `N.T.P` is first expanded isothermally to twice the origional volume. It is then compressed at constant volume, till its pressure is raised to the original value. Calculate the total amount of work done. Given `R= 8.3 mol e^(_1)K^(-1)`.
View SolutionCalculate work done to compress isothermally 1g of hydrogen gas at N.T.P. to half its initially volume. Find the amount of heat evolved and change in internal energy. Given `R = 8.31 J mol^(-1) K^(-1)`.
View SolutionTen mole of hydrogen at `N.T.P` is compressed adiabatically so that it temperature becomes `400^(@)C`. How much work is done on the gas? Also, Calculate the increase in internal energy of the gas. Take `R=8.4J mol e^(-1)K^(-1) and gamma= 1.4`.
View SolutionIf `1 gram` of oxygen at `760 mm` pressure and `0^(@)C` has its volume double in an adiabatic change , calculate the change in internal energy. Take `R=2 cal. Mol e^(-1)K^(-1)= 4.2J cal^(-1) and gamma=1.4`.
View SolutionA sample of hydrogen of mass `6g` is allowed to expand isothermally at `27^(@)C` till its volume is doubled.
(a) How may moles of hydrogen are there?
(b) What is final temperature of `H_(2)`?
(c ) Caculate work done during expansion.
View Solution50 g of oxygen at N.T.P. is compressed adiabatically to a pressure of 5 atmosphere. Caluculate the work done on the gas, if `gamma = 1.4` and `R = 8.31 J mol^(-1) K^(-1)`
View SolutionFind the efficiency of a Carnot's engine working between `127^(@)C` and `27^(@)C`.
View SolutionA Carnot engine, whose temperature of the source is `400K` receives `200 calories` of heat at this temperature and rejects `150 calaroies` of heat to the sink. What is the temperature of the sink? Also, calculate the efficiency of the engine
View SolutionCalculate the efficiency of a Carnot engine operating between 450 K and 300 K. If this engine takes 5,00,000 J from the surce, how much work does it perform ?
View SolutionA Carnot engine whose low temperature reservoir is at `7^(@)C` has an efficiency of `50%`. It is desired to increase the efficiency to `70%` By low many degrees should the temperature of the high temperature reservoir be increased
View SolutionEfficiency of a carnot engine is `0.4`, when temp. of sink is `300K`. What is temp. of source? If temp. of source is kept same and that of sink is lowered by `50^(@)C`, what would be the efficiency?
View SolutionA carnot engine absorbs `1000J` of heat energy from a reservoir at `127^(@)C` and rejecs `600J` of heat energy during each cycle. Calculate (i) efficiency of the engine, (ii) temperature of sink, (iii) amount of useful work done per cycle.
View SolutionA reversible heat engine operates with an efficiency of 50 %. If during each cycle it rejects 150 cal to a reservoir of heat at `30^(@)C`, then (i) what is the temperature of the other reservoir and (ii) how much work does it carry out per cycle ?
View SolutionA reversible engine converts one-sixth of the heat input into work. When the temperature of the sink is reduced by `62^@C` , the efficiency of the engine is doubled. The temperatures of the source and sink are
View SolutionCalculate the difference in efficiencies of a Carnot engine working between (i) 400 K and 350 K and (ii) between 350 K and 300 K
View SolutionAn ideal gas heat engine operates in a Carnot's cycle between `227^(@)C and 127^(@)C`. It absorbs `6 xx 10^(4) J` at high temperature. The amount of heat converted into work is
View SolutionThe boling point of water at a pressure of 50 atmosphere is `250^(@)C`. Compare the theoretical efficincies of the engine oprating between the boling of water at (i) 1 atmosphere (ii) 50 atmosphere, assuming the temperature of sink the wach case as `27^(@)C`.
View SolutionA perfect carnot engine has source temp. `227^(@)C` and sink temp. `127^(@)C`. Find the efficiency of the engine, If `10000J` of external work is to be done, find heat received from source and heat released to the sink.
View SolutionA Carnot engine takes in heat from a reservoir of heat at `427^(@)C`. How many calories of heat must it take from the reservoir in order to procuce useful mechanical work at the rate of `357 watt`?
View SolutionA Carnot's engine operates with an efficiency of `40%` with its sink at `27^(@)C`. By what amount should the temperature of the source be increased with an aim to increase the efficiency by `10%`
View SolutionA Carnot engine works between `200^(@)C` and `0^(@)C` and another carnot engine works between `0^(@)C` and `-200^(@)C` . In both caes the working substance absorbes 4 kcal of heat from the source. The efficiency of first engine will be
View SolutionTwo carnot engines A and B are operated in series. The first one A receives heat at `900K` and rejects it to a reservoir at `T K`. The second engine B receives the heat rejected by the first engine and rejects it to a heat reservoir at `400K`. Calculate the value of `T`, when the efficiency ot two engines is the same.
View SolutionOne mole of an ideal gas is taken in a Carnot engine working between `27^(@)C` and `227^(@)C`. The useful work done in one cycle is 600 J. calculate the ratio of volume of gas at the end and beginning of the isothermal expansion. Given 8.3 J mole `.^(-1) K^(-1)`.
View SolutionIn a double acting steam engine, the average pressure in `90Ncm^(-2)`, the area of the piston is `7.46 cm^(2)`, the length of the strokes is 1 meter and the number of revolution per second is 5. find the horse power of the engine.
View SolutionIn a reciprocating steam engine, the avearge pressure of steam is `50 Nm^(-2)`. The length of half stroke is 50 cm and area of cross section of the piston is `400 cm^(2)`. If the engine is making 300 strokes per minute, calculate power of the engine in k W.
View SolutionAn engine develops 33250 W power, consuming 10 kg fule per hour. If the clorific value of the fuel is 11450 cal `g^(-1)`, find the efficiency of the engine.
View SolutionA petrol engine consumes 5 kg of petrol in one hour, the calorific value of petrol being `10^(8)` calorie `kg^(-1)` . Assuming that only 12% of heat is converted into mechanical energy, find the average power developed in kilowatt.
View SolutionOne kilograme of coal produces `3.6xx10^(6)` cal of heat during combustion. Assuming that only 1/10 th energy so generated is utilised for useful purpose, find the mass of coal required per hour for running 210 kW engine.
View SolutionIn a petrol engine, the rate of production of heat due to combustion of the fuel is `5xx10^(6) cal h^(-1)`. The efficiency of the engine is 30%. Calculate the H.P. of the engine. Given, J=4.2 J `cal^(-1)` and 1 h.p. =746 W.
View SolutionIn a petrol engine,the rate of production of heat due to the combustion of petrol is `7.46xx10^(5) cal h^(-1)` . The efficiency of the engine is 30%. Calculate the horse power of the engine, if J is `4.2xx10^(7)` erg `cal^(-1)`
View SolutionIn a refrigerator, heat from inside at `270 K` is transferred to a room at `300 K`. How many joule of heat energy is drawn from the sink for each joule of electrical energy consumed ideally ?
View SolutionRefrigerator A works between `-10^@C` and `27^@C`, while refrigerator B works between `-27^@C` and `17^@C`, both removing heat equal to 2000J from the freezer. Which of the two is the better refrigerator?
View SolutionAssuming that a domestic refrigerator can be regarded as a reversivle engine working between the temperature of melting ice and that of the atmosphere `(170^(@)C)`, calculate the energy which muct be supplied to freeze one kilogram of water already at `0^(@)C`.
View SolutionA refrigerator whose coefficient of performance `eta'` is 4, extracts heat from the cooling compartment at the rate of 400 J per cycle.
(a) How much work per cycle is required to operate the refrigerator cycle?
(b) How much heat per cycle is discharged to the room which acts as the high temperature reservoir?
View SolutionA refrigerator has to transfer an average of 263J of heat per second from temperature `-10^@C` to `25^@C`. Calculate the average power consumed, assuming no enegy losses in the process.
View SolutionA refrigerator freezes 5 kg of water at `0^(@)C` into ice at `0^(@)C` in a time internal of 20 minutes. Assume that the room temperature is `20^(@)C`. Calculate the minimum power needed to accomplish it.
View SolutionIce in cold storage melts at the rate of 3 kg per hour. The temperature outside is `27^(@)C`. Find the minimum power output of a motor used to drive the refrigerator, which just prevents ice from melting.Latent heat of ice `80 cal g^(-1)`, `J=4.2xx10^(7)` erg `cal^(-1)`. given 1h.p. =746 W.
View SolutionThe temperature gradient in a rod of `0.5 m` length is `80^(@)C//m`. It the temperature of hotter end of the rod is `30^(@)C`, then the temperature of the cooler end is
View SolutionCalculate the rate of loss of heat through a glass window of area `1000 cm^(2)` and thickness 0.4 cm when temperature inside is `37^(@)C` and outside is `-5^@)C` . Coefficient of thermal conductivity of glass is `2.2 xx 10^(-3) cal s^(-1) cm^(-1) K^(-1)`.
View SolutionCalculate the difference in temperature between two sides of an iron plate 20 m m thick, when heat is conducted at the rate of `6xx10^(5)` cal/min/`m^(2)`. K for metal is 0.2 cal`s^(-1)cm^(-1).^(@)C^(-1)`
View SolutionThe length, a rod of aluminium is 1.0 m and its area of cross-section is `5.0 cm^(2)`. Its one end is kept at `250^(@)C` and the at `50^(@)C`. How much heat will flow in the rod in 5.0 minutes . K for Al =`2.0xx10^(-1) k J s^(-1) m^(-1) .^(@)C^(-1)` and `J=4.18 cal^(-1)`
View SolutionA flat-bottom kettle placed on a stove is being used to boil water and the thermal conductivity of the material is `0.5 cal s^(-1) cm^(-1)`. If the amount of steam being produced in the kettle is at rate `10 g min^(-1)`, calculate the difference of temperature between the inner and outer surface of of the bottom. the latent heat of steam is `540 cal g^(-1)`
View SolutionAn iron boiler is 10 mm thick and has a heating area `2m^(2)`. The two surfaces of the boiler are at `240^(@)C` and `100^(@)C` respectively. Find the mass of the water evaporated into steam per minute. Given that latest heat of steam is `536 kcal kg^(-1)` and thermal conductivity of iron is `1.6 xx 10^(-2) kcal s^(-1) m^(-1) .^(@)C^(-1)`.
View SolutionOne end of a 0.25 m long metal bar is in steam and the other is in contact with ice . If 12 g of ice melts per minute, what is the thermal conductivity of the metal? Given cross-section of the bar `=5xx10^(-4) m^(2)` and latent heat of ice is `80 cal g^(-1)`
View SolutionA layer of ice 0.15 m thick has formed on the surface of a deep pond. If the temperature of upper surface of ice is constant and equql to that of air which is `-12^(@)C`, determine the time it will take to increase of ice layer by 0.2 mm. take latent heat of ice =80 cal `g^(-1)`, density of ice =0.91 g `cm^(-3)` and thermal conductivity of ice =0.5 cal `s^(-1)m^(-1)K^(-1)` .
View SolutionWater is boiled in a rectangular steel tank of thickness 2 cm by a constant temperature furnace. Due to vaporisation, water level falls at a steady rate of 1 cm in 9 minutes. Calculate the temperature of the furnace. Given K for steel `0.2 cal s^(-1) m^(-1) .^(@)C^(-1)`
View SolutionEstimate the rate at which ice would melt in a wooden box 20 mm thick and of inside measurement 200 cm x 100 cm x 100 cm assumming that external temperature is `27^(@)C` and coefficient of thermal conductivity of wood is `0.0004 cal s^(-1) cm^(-1) .^(@)C^(-1)`.
View SolutionSteam at `100^(@)C` is passed through a tubeof radius 5 cm and length 3 m. If the thickness of tube be 2 mm and conductivity of its material be `2xx10^(-4)" cal "cm^(-1) K^(-1) s^(-1)`, calculate the rate of loss of heat in `js^(-1)` . The outside temperature is `20^(@)C`.
View SolutionThe outer face of a rectangular slab of equal thickness of iron and brass are maintained at `100^(@)C` and `0^(@)C`, respectively. Find the temperature of the interface. The conductivities of iron and brass are 14 and `126 Wm^(-1) K^(-1)` respectively.
View SolutionThe thermal conductivity of copper is four times that of brass. Two rods of copper and brass of same length and cross-section are joined end to end. The free end of copper rod is at `0^(@)C` and that of brass rod at `100^(@)C`. Calculate the temperature of junction at equilibrium. neglect radiation losses.
View SolutionA composite wall of area A is made of equal thickness of lead and iron having thermal conductivities K and 2K, respectively. The temperature on the two sides of the composite wall are `100^(@)C` and `0^(@)C` with the layer on the hotter side. Calculate the steady -state temperature of the lead-iron interface.
View SolutionA wall consists of two layer, one of thickness 3 cm and other of 6 cm. the thermal conductivities of the materials of these layers are K and 3K respectively. The temperature of the outer surfaces of the two layers are `-5^(@)C` and `20^(@)C` respectively. determine the temperature of the surface of contact of the two layers in the steady state.
View SolutionThe plane surface of two sheets of different metals are kept in contact with each other. The thickness of sheets `2.5 cm` and 3 cm respectively and the ratio of their thermal conductivities in the same order is `5:6`. If the outer surfaces of the sheets are at constant temperatures of `100^(@)C` and `10^(@)C` respectively, calculate the temperature of interface.
View SolutionThe temperature difference between the two ends of a bar 1.0 m long is `50^(@)C` and that for the other bar 1.25 m long `75^(@)C`. Both the bars have same area of cross-section. If the rates of conduction of heat in the two bars are the same, find the ratio of the coefficients of thermal conductivity of the materials of the two bars.
View SolutionThe ratio of the areas of cross-section of two rods of different materials is `1:2`, and the ratio of the thermal conductivities of their materials is `4:3`. On keeping equal temperature-difference between the ends of these rods, the rate of conduction of heat are equal. Determine the ratio of the lengths of the rods.
View SolutionIn fig, two bars of the same metal are connected. The length of the first bar is half of that of the second, but the cross-sectional area is double. What is the temperature of the junction of the bars ?
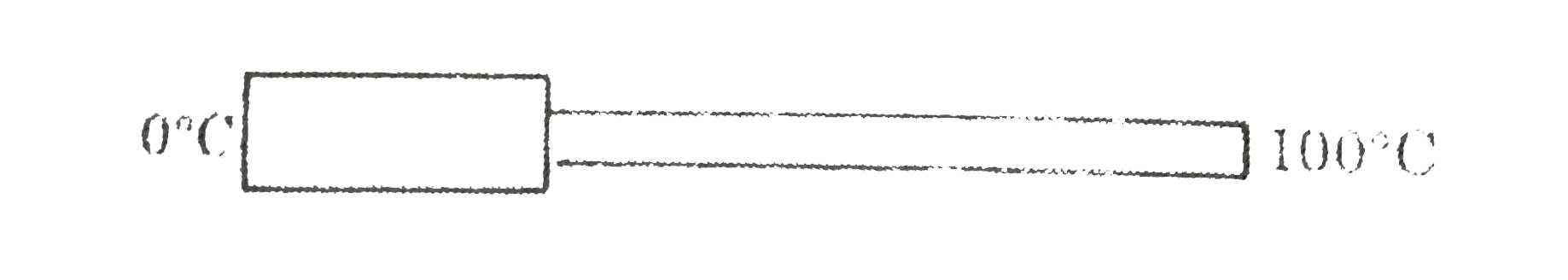
View SolutionA room at `20^@C` is heated by a heater of resistence 20 ohm connected to 200 VV mains. The temperature is uniform throughout the room and the heati s transmitted through a glass window of area `1m^2` and thickness 0.2 cm. Calculate the temperature outside. Thermal conductivity of glass is `0.2 cal//mC^@` s and mechanical equivalent of heat is `4.2 J//cal`.
View SolutionThe sun radiates maximum energy at wavelength `4753 Å`. Estimate the surface temperature of the sun, if `b = 2.888 xx 10^(-3) mK`.
View SolutionThe temperature of an ordinary electric bulb is around 3000 K. At what wavelength will it radiate maximum energy ? Will this wavelength be within visible region? Given `b = 0.288 cm K`.
View SolutionA furnace is at a temperature of 2000K. At what wavelength will it radiate maximum intensity. Is it in visible region?
View SolutionA black body radiates energy at the rate of `1.452 xx 10^(10) erg s^(-1) m^(-2)`. Calculate the value of Stefan's constant.
View SolutionCalculate the temperature (in K) at which a perfect black body radiates energy at the rate of `5.67W cm^(-2)`. Given `sigma = 5.67 xx 10^(8)Wm^(-2)K^(-4)`.
View SolutionA full radiator at `0^(@)C` radiates energy at the rate of `3.2 xx 10^(4) erg cm^(-2) s^(-1)`. Find (i) Stefan's constant and (ii) the amount of heat radiated per second by a sphere of radius 4 cm and at a temperature of `1000^(@)C`.
View SolutionSurface temperature of sun is `6000 K`. Considering sun as a perfectly black body, calculate the energy given out by sun per second in radiation. Radius of sun`= 6.9 xx 10^(8) m` and `sigma = 5.67 xx 10^(-8) J s^(-1)m^(-2) K^(-4)`
View SolutionThe original temperature of a black body is . `727^(@) C` . The temperature at which this black body must be raised so as to double the total radiant energy, is
View SolutionThe temperature of a body in increased from `27^(@)C` to `127^(@)C`. By what factor would the radiation emitted by it increase?
View SolutionA black body initially at `27^(@)C` is heated to `327^(@)C` is heated to `327^(@)C`. How many times is total heat emitted at the higher temperature than emitted at lower temperature ? What is the wavelength of the maximum energy radiation at the higher temperature ? Wien's constant `= 2.898 xx 10^(-3) mK`.
View SolutionAn electric bulb with tungsten filament having an area of `0.25 cm^(2)` is raised to a temperature of `3000 K`, when a current passes through it. Calculate the electrical energy being consumed in watt, if the emissivity of the filament is 0.35. Stefan's constant, `sigma = 5.67 xx 10^(-5) erg^(-1) cm^(-2) K^(-4)`. If due to fall in main voltage the fialment temperature falls to 2500 K, what will be wattage of the bulb?
View SolutionThe energy emitted per second by a black body at `1227^(@)C`. If the temperature of the black body is increased to `2727^(@)C`, calculate the energy emitted per second in terms of E in the second case.
View SolutionA black ened metal sphere of radius 7 cm is encllosed in as evacuated chamber maintained at a temperature of `27^(@)C`. At what rate must energy be supplied to the sphere so as to keep its temperature constant at `127^(@)C` ?
`sigma=5.7xx10^(-8) W m^(-2) K^(-4)`.
View SolutionA sphere of radius 10 cm is hung inside an oven walls are at a temperature of 1000 K. Calculate total energy incident per second (in `Js^(-1)`) on the sphere. Given `sigma = 5.67 xx 10^(-8) SI` units.
View SolutionA body which has a surface area `5.0 cm^(2)` and a temperature of `727^(@)C` radiater 300 J energy each minute. What is its emissivity? Stefan's Boltzmann's constat is `5.76 xx 10^(-8) Wm^(-2)K^(-4)`.
View SolutionA thin brass rectangular sheet of sides 15.0 and 12.0 cm is heated in a furnace to `600^@` C and taken out. How much electric power is needed to maintain the sheet at this temperature, given that its emissivity is 0.250?
Neglect heat loss due to convection (Stefan-Boltzmann constant, `sigma=5.67xx10^-8 W//m^(2)-K^(4)`).
View SolutionA metal ball of surface area `200 cm^(2)` and temperature `527^(@)C` is surrounded by a vessel at `27^(@)C` . If the emissivity of the metal is 0.4, then the rate of loss of heat from the ball is `(sigma = 5.67 xx10^(-8)J//m^(2)-s-k^(4))`
View Solution
Problems for self practice questions and complete solutions for chapter THERMODYNAMICS of DHANPAT RAI & CO PHYSICS (HINGLISH) of Class 11