Complete Solutions to Exercise (Passage Based Questions) of chapter VECTOR ALGEBRA of Class 12 book with complete answers and questions

Let OABCD be a pentagon in which the sides OA and CB are parallel and the sides OD and AB are parallel. Also, OA:CB=2:1 and OD:AB=1:3.

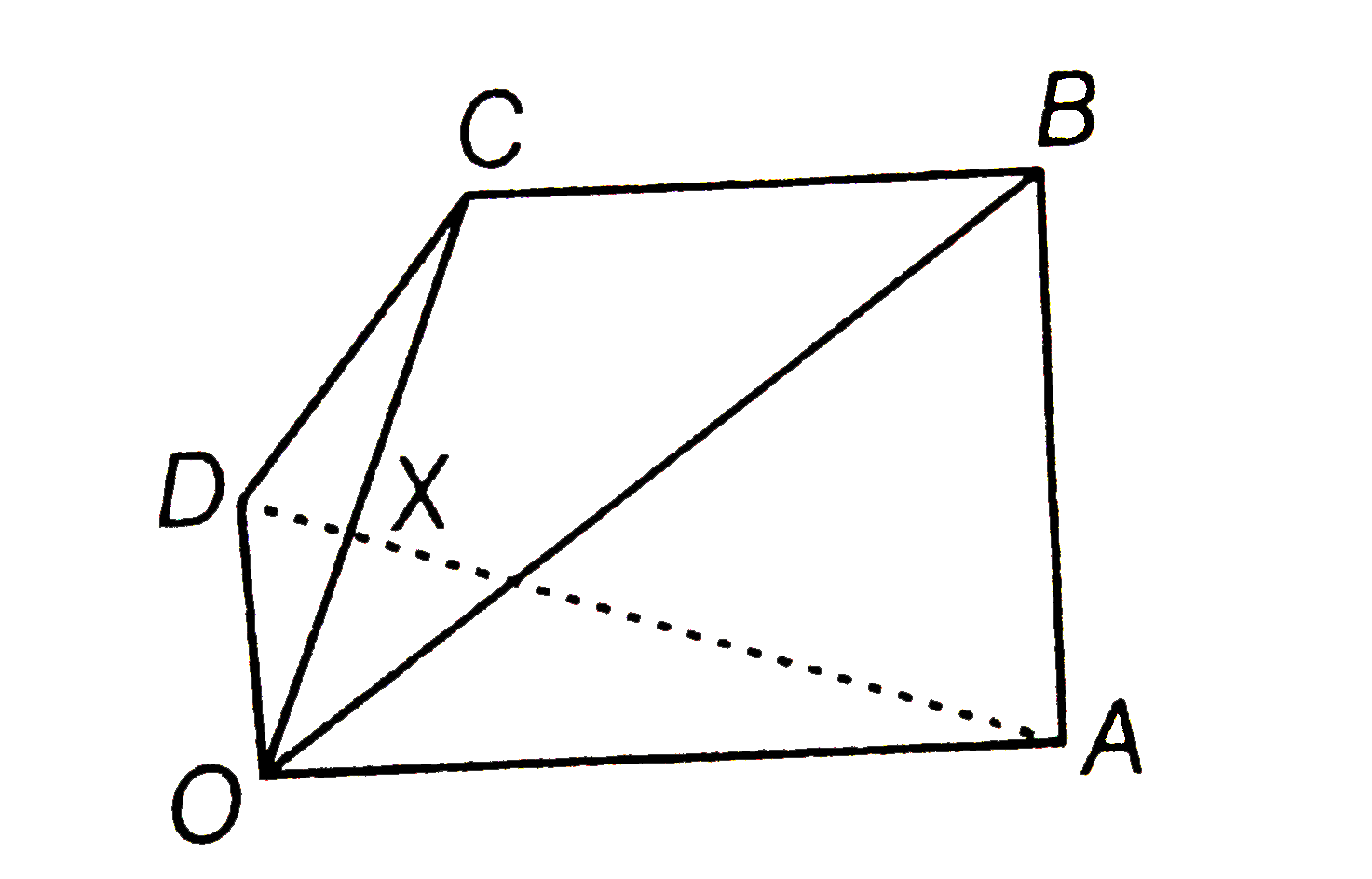
Q. The ratio `(AX)/(XD)` is

Let OABCD be a pentagon in which the sides OA and CB are parallel and the sides OD and AB are parallel as shown in figure. Also, OA:CB=2:1 and OD:AB=1:3. if the diagonals OC and AD meet at x, find OX:OC.

If ABCDEF is a regular hexagon then `vec(AD)+vec(EB)+vec(FC)` equals :

Consider the regular hexagon ABCDEF with centre at O (origin).
Q. Five forces AB,AC,AD,AE,AF act at the vertex A of a regular hexagon ABCDEF. Then, their resultant is (a)3AO (b)2AO (c)4AO (d)6AO

Three points A,B, and C have position vectors `-2veca+3vecb+5vecc, veca+2vecb+3vecc` and `7veca-vecc` with reference to an origin O. Answer the following questions?
Which of the following is true?

Three points A,B, and C have position vectors `-2veca+3vecb+5vecc, veca+2vecb+3vecc` and `7veca-vecc` with reference to an origin O. Answer the following questions?
Which of the following is true?

Three points A,B, and C have position vectors `-2veca+3vecb+5vecc, veca+2vecb+3vecc` and `7veca-vecc` with reference to an origin O. Answer the following questions?
B divided AC in ratio

If two vectors OA and OB are there, then their resultant OA+OB can be found by completing the parallelogram OACB and OC=OA+OB. Also, if |OA|=|OB|, then the resultant will bisect the angle between them.
Q. A vector C directed along internal bisector of angle between vectors `A=7hati-4hatj-4hatk and B=-2hati-hatj+2hatk` with `|C|=5sqrt(6)` is

Find `dy/dx if 2x-3y=sinx`

Let `C:vecr(t)=x(t)hati+y(t)hatj+z(t)hatk` be a differentiable curve i.e., `exists lim_(hto0) (vecr(t+h)-vecr(t))/(h) forall t`
`therefore vecr'(t)=x'(t)hati+y'(t)hatj+z'(t)hatk`
`vecr'(t)` is tangent to the curve `C` at the point `P[x(t),y(t),z(t)], vecr'(t)` points in the direction of increasing `t`.
The point `P` on the curve `vecr(t)=(1-2t)hati+t^(2)hatj+2e^(2(t-1))hatk` at which the tangent vector `vecr'(t)` is parallel to the radius vector `vecr(t)` is:

Let `C:vecr(t)=x(t)hati+y(t)hatj+z(t)hatk` be a differentiable curve, i.e. `exists lim_(hto0) (vecr(t+h)-vecr(t))/(h) AA t`
`therefore vecr'(t)=x'(t)hati+y'(t)hatj+z'(t)hatk`
`vecr'(t)` is tangent to the curve `C` at the point `P[x(t),y(t),z(t)]` and points in the direction of increasing `t`.
The tangent vector to `vecr(t)=(2t^(2))hati+(1-t)hatj+(3t^(2)+2)hatk` at `(2,0,5)` is: