Complete Solutions to Subjective Type of chapter COULOMB LAW AND ELECTRIC FIELD of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | COULOMB LAW AND ELECTRIC FIELD |
| CENGAGE PHYSICS | Subjective Type |
| Number Of Questions | 13 |
| Academic Year | 2023-24 |
Calculate the number of electrons in a small, electrically neutral silver pin that has a mass of `10.0 g`. Silver has 47 electrins per atom, and its molar mass is `107.87 gmol^(-1)`.
View SolutionA charged particle of radius `5xx10^(-7)m` is located in a horizontal electric field of intensity `6.28xx10^(5)Vm^(-1)`. The surrounding medium has the coeffiecient of viscosity `eta=1.6xx10^(5)Nsm^(-2)`. The particle starts moving under the effect of electric field and finally attains a uniform horizontal speed of `0.02 ms^(-1)`. Find the number of electrons on it. Assume gravity free space.
View SolutionIndentical isolated conduction sphers 1 and 2 have equal charges and are separated by a distance that is large compared with their diameters (Fig.a). The electrostatic force acting on spkhere 2 due to sphere 1 is F. Suppose now that a third identical sphere 3, having an insulation handle and initially neutral, is touched first to sphere 1 (Fig. b), then to sphere 2 (Fig. c), and dinally removed (Fig. d). The electrostatic force that now acts on sphere 2 has magnitude F'. What is the `(F')/(F)`?
View SolutionIn Fig. a, particle 1 (of charge `q_(1)`) and particle 2 (of charge `q_(2)`) are fixed in place on an x axis, `8.00` cm apart.
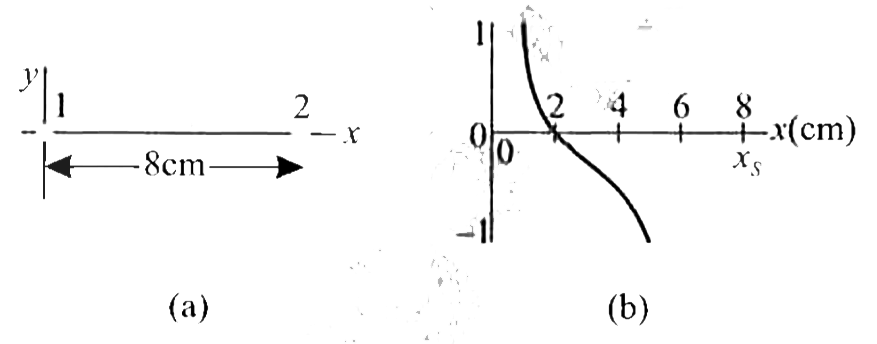
Particle 3 (of charge `q_(3)=+8.00xx10^(-19)C`) is to be placed on the line between particles 1 and 2 so that they produce a net electrostatic force `F_("net")=2F_(0)` on it Figure b gives the x component of that force versus the coordinate x at which particle 3 is placed. The scale of the x-axis is set by `x_(s)=8.0`cm. What are (a) the sign of charge `q_(1)` and (b) the ratio `q_(2)//q_(1)`?
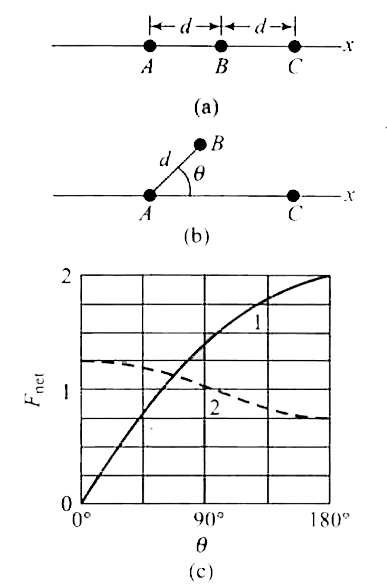
View SolutionFigure (a) shows an arrangement of three charged particles separted by distance d. Particles A and C are fixed on the x-axis, but particle B can be moved along a circle centered on particle A. During the movement, a radial line between A and B makes an angle `theta` relative to the positive direction of the x-axis (Fig. b). The curves in Fig. c give, for two situations, the magnitude `F_("net")` of the net electrostatic force on particle A due to the other particles. That net force if given as a function of angle `theta` and as a multiple of a basic amount `F_(0)`. For example on curve 1, at `theta=180^(@)` we see that `F_("net")=2F_(0)`. (a) For the situation corresponding to curve 1, what is the ratio of the charge of particle C to that of particle B (including sign)? (b) For the situation corresponding to curve 2, what is that ratio?
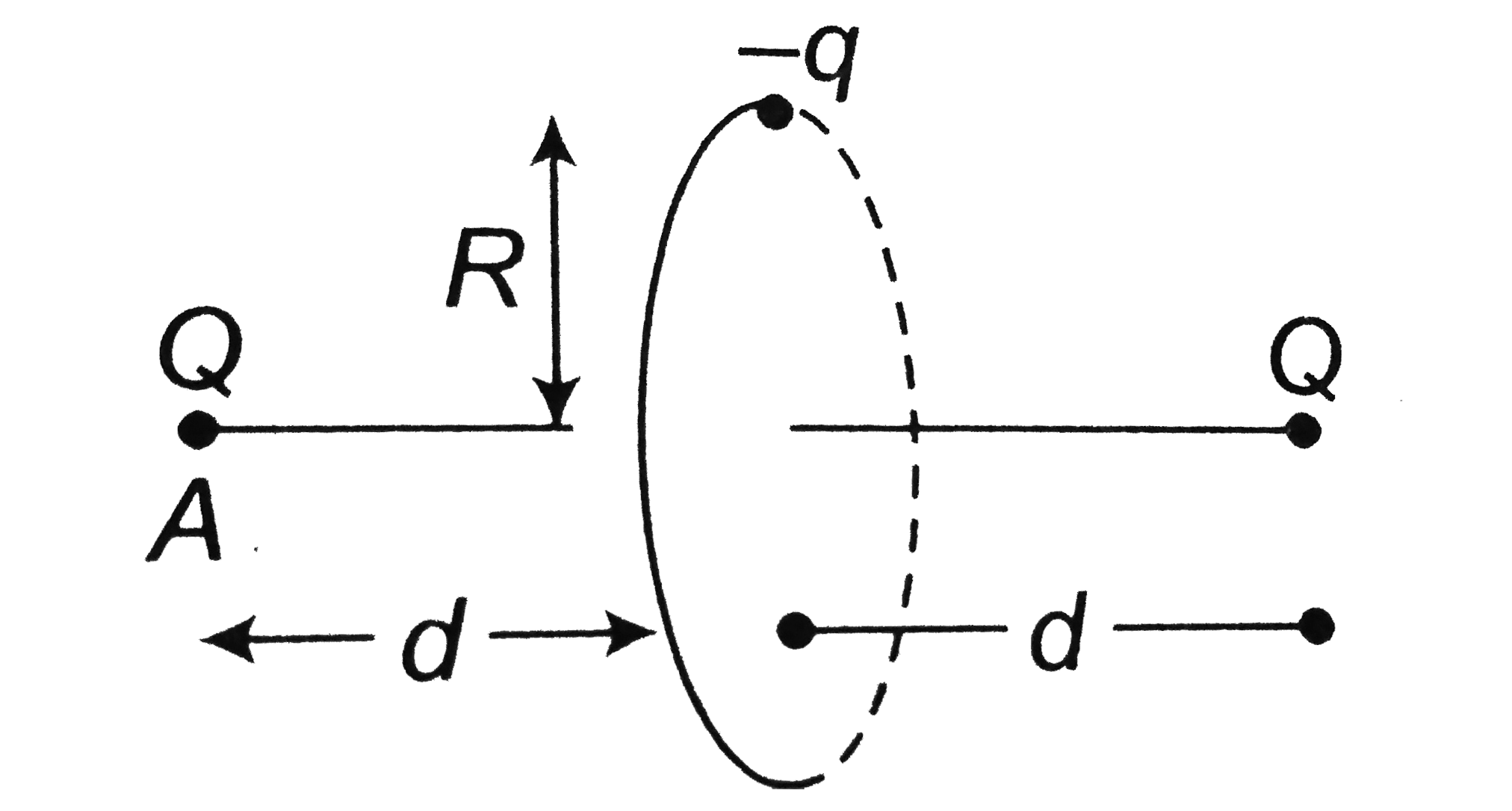
View SolutionTwo positive point charges each of magnitude `10 C` are fixed at positions `A` &`B` at a seperation `2d= 6m`. A negatively charged particle of mass `m= 90gm` and charge of magnitude `10xx10^(-6)C` is revolving in a circular path of radius `4m` in the plane perpendicular to the line `AB` and bisecting the line `AB`. Neglect the effect of gravity. Find the angular velocity of the particle
View SolutionFour point positive charges are held at the vertices of a square in a horizontal plane. Their masses are `1kg, 2kg, 3kg, & 4kg`. Another point positive charge of mass `10 kg` is kept on the axis of the sqaure. The weight of their fifth charge is balanced by the electrostatic force due to those four charges. If the four charge on the vertices are released such that they can freely move in any direction(vertical, horizontal etc.) then the acceleration of the centres of mass of the four charges immediately after the release is (Use `g= 10m//s^(2)`)
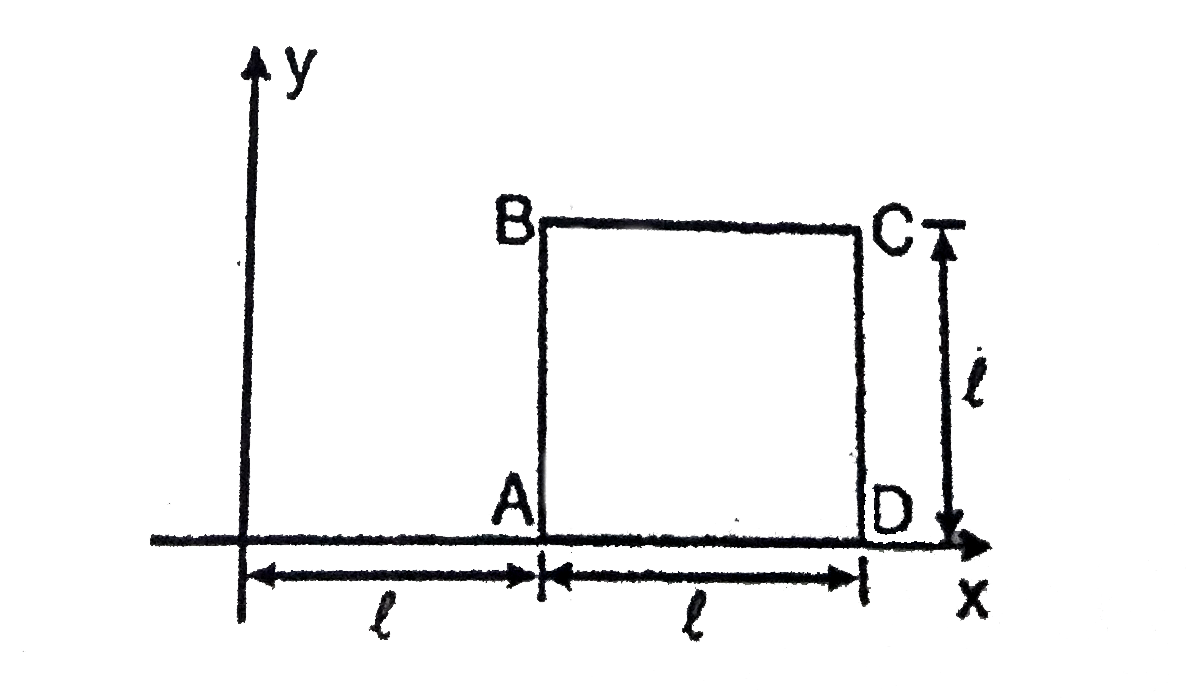
View SolutionA square loop of side 'l' having uniform linear charge density '`lambda`' is placed in 'xy' plane as shown in the figure. There is a non uniform electric field `vec E= a/l (x+l) hat i` where a is a constant. Then resultant electric force on the loop if `l=10cm, a=2N//C` and charge density `lambda = 2muC//m` is:
View SolutionFig shows the electric field lines around three points charges A,B,C.
(a) Which charges are positive ?
(b) Which charge has the largst magnitude ? Why ?
(c ) In which region or regions of the picture could be the electric field be zero? Justify your answer.
(i) near A, (ii) near B, (iii) near C, (iv) nowhere.
View SolutionIn a certain region of space, electric field is along the z-direction throughout. The magnitude of electric field is, however, not constant but increases uniformly along the positive z-direction at the rate of `10^(5)NC^(-1)m^(-1)` . What are the force and torque experienced by system having a total dipole moment equal to `10^(-7)`Cm in the negative z-direction?
View SolutionElectric dipole of moment `vec(p)=p hat(i)` is kept at a point (x, y) in an electric field `vec(E )=4x y^(2)hat(i)+4x^(2)y hat(j)`. Find the magnitude of force acting on the dipole.
View SolutionA dipole with an electric moment p is located at a distance r from a along thread charge uniformly with a linear density `lambda`. Find the force F acting on the dipole if the vector p is oriented
(a) along the thread
(b) along the radius vector r
(c ) at the right angles to the thread and the radius vector r.
View SolutionFigure shows a charge rod, bent in the from of an arc of a circle. The charge distribution on the rod is shown in figuire. The assembly is kept in a uniform electric field.

(a) Find the dipole moment of the rod.
(b) For small angular displacement the system find the time period of oscillation of the system.
View Solution
Subjective Type questions and complete solutions for chapter COULOMB LAW AND ELECTRIC FIELD of CENGAGE PHYSICS (HINGLISH) of Class 12