Complete Solutions to Illustration of chapter COULOMB LAW AND ELECTRIC FIELD of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | COULOMB LAW AND ELECTRIC FIELD |
| CENGAGE PHYSICS | Illustration |
| Number Of Questions | 40 |
| Academic Year | 2023-24 |
A glass rod is rubbed with a silk cloth. The glass rod acquires a charge of `+19.2xx10^(-19)C`.
(i) find the number of electrons lost by glass rod.
(ii) Find the negative charge acquired by silk.
(iii) Is there transfer of mass from glass to silk?
View SolutionIf an object made of substance A is rubbed with an object made of substance B, then A becomes positively charged and B becomes negatively charged. If however, an object made of substance C, then A becomes negatively charged, What will happen if an object made of substance B is rubbed against an object made of substance C?
(a) B becomes positively charged and C becomes positively
(b) B becomes positively charged and C becomes negatively charged.
(c ) B becones negatively charged and C becomes positively charged.
(d) B becomes negatively charged and C becomes negatively charged.
View SolutionObject A, B and C, are three identical, insulated, spherical conductors. Originally, A and B have charges of +3 mC, whereas C has a charge of -6mC. Objects A and C are touched and moved apart, Objects B and C are touched before they moved apart.
(i) If pbjects A and B are now held near each other they will
(a) attract (b) repel (c ) have no effect on each other.
(ii) If instead object A and C held near each other, they will
(a) attract (b) repel (c ) have no effect on each other.
View SolutionFigure shows that a positively charged rod is brought near two ucharged metal spheres A and B clamped on insulated stands and palced in contact with each other.

(i) What would happen if the rod is removed before the spheres are separated?
(ii) Would the induced charges be equal in magnitude even if the spheres had different sizes or different conductors?
(iii) What will happen if the spheres are separated first and then the rod is rmoved far away?
View SolutionCalculate the total positive (or negative) charge on a 3.11 g copper penny. Given Avogadro's number `=6.023xx10^(23)g mol^(-1),`, and atomic mass=63.5.
View SolutionAn electron and a proton, initiall sepatated by a distance d in air are released from rest simultaneously. The two particles are free to move. When they collide, are they (a) at the midpoint of their initial separation, (b) closer to the initial position of the proton or (c ) closer to the initial position of the electron?
View SolutionTwo large conduction spheres carrying charges `Q_(1)` and `Q_(2)` are brought close to each other. Is the magnitude of the force between them exactly given by `Q_(1)Q_(2)//4piepsilon_(0)r^(2)`. Where r is the distance between their centers.
View SolutionFour identical point charges are placed at the corners of a square, A fifth point charge placed at the center of the square experiences zero net force . Is this a stable equilirium for the fifth charge? Explain.
View SolutionTwo particles (free to move) with charges `+q` and `+4q` are a distance L apart. A third charge is placed so that the entire system is in equilibrium.
(a) Find the location, magnitude and sign of the third charge.
(b) Show that the equilibrium is unstable.
View SolutionThree positive charges of equal magnitude q are placed at the vertices of equilateral triangle of side l. How can the system of charges be placed in equilibrium?
View SolutionA charge Q located at a point `vec r` is in equilibrium under the combined field of three charges `q_(1), q_(2)` and `q_(3)`. If the charges `q_(1)` and `q_(2)` are located at point `vec r_(1)` and `vec r_(2)` respectively, find the direction of the force on Q due to `q_(3)` in terms of `q_(1),(q_2), vec r_(1) vec r_(2)`, and `vec(r)`.
View SolutionTwo identical conducting spheres 1 and 2 carry equal amounts of charge and are ficed apart at a certain distance larger than their diameters. The spheres repel each other with an electrical force of 88mN. A thired identical sphere 3, having an insulating handle and initially uncharged, is touched first to sphere 1 and then to sphere 2 and finally removed. Find the force between spheres 1 and 2 as shown if Figure.
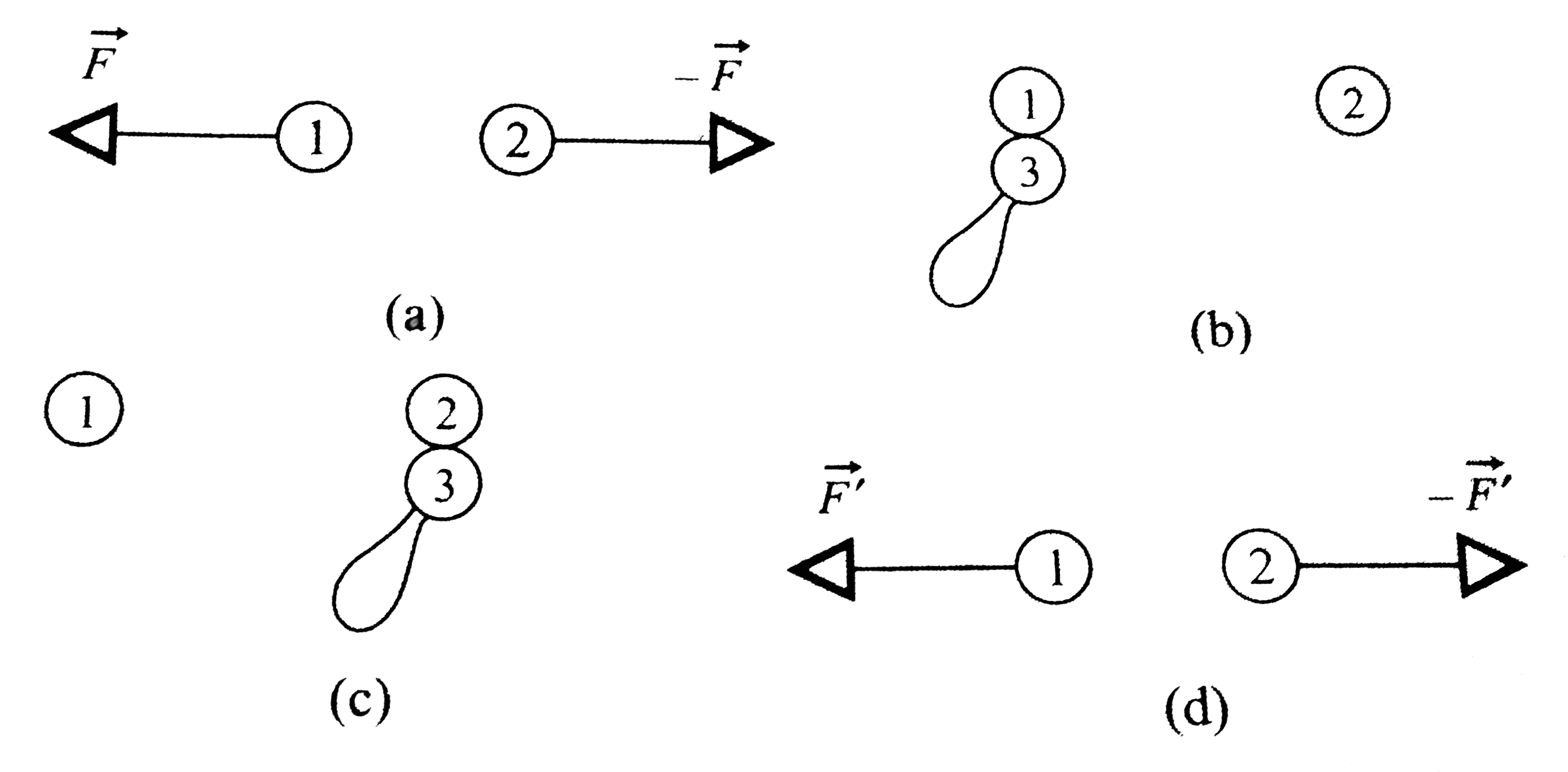 ,
,
View SolutionTwo identical He-filled spherical balloons each carrying a charge q are tied to a weight W with strings and float in equlibrium, as shown in figure.
(i) Find the magnitude of q, assuming that the charge on each balloon acts as if it were concentrated at the center.
(ii) Find the volume of each balloon. Take the density of He as `rho_(He)` and the density of air as `rho_(a)`. Ignore the weight of the unfilled balloons .
 ,
,
View SolutionConsider three charges `q_(1), q_(2), q_(3)` each equal to q at the vertices of an equilateral triangle of side l. What is the force on a charge Q (with the same sign as q) placed at the centroid of the triangle, as shown in Fig. ?
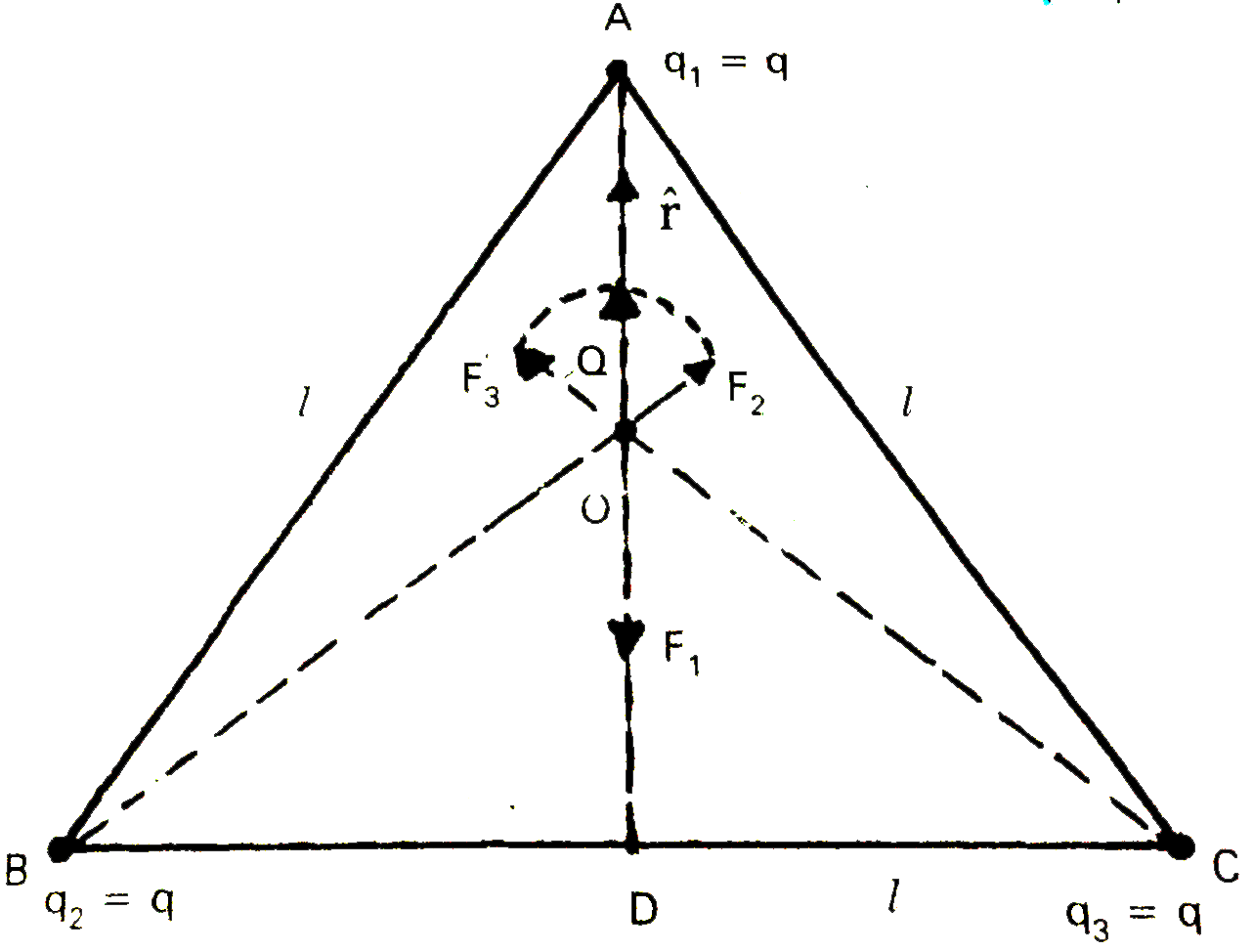
View SolutionPoint charge are placed at the verticas of a squre of side a as shown in fig. What should be the sign of charge q and magnitude of the ratio `|q//Q|` so that
(i) net force on each Q is zero?
(ii) net force on each q is zero?
Is it possible that the entire system could be in electrostatic equilibrium?

View SolutionTwo identical small charged spheres, each having a mass m, hang in equilibrium as shown in fig. the ligth of each string is l, and the angle made by any string with the vertical is `theta`. Find the magnitude of the charge on each sphere.
View SolutionTwo identical balls each having a density `rho` are suspended from as common point by two insulating strings of equal length. Both the balls have equal mass and charge. In equilibrium each string makes an angle `theta` with vertical. Now, both the balls are immersed in a liquid. As a result the angle `theta` does not change. The density of the liquid is `sigma`. Find the dielectric constant of the liquid.
View SolutionThree paricles, each of mass m and carrying a charge q each, are suspended from a common pointby insulating massless strings each of length L. If the particles are in equilibrium and are located at the corners of an equilateral triangle of side a, calculate the charge q on each particle. Assume `Lgtgta`.
View SolutionA thin fixed ring of radius a has a positive chage q unformly distributed over it.A particle of mass m having a megative charge Q, is placed on the axis at a distance of `x(xltlta)` form the center of the ring. Show that the motion of the negatively chaged particle is approximatly simple harmonic. Calculate the time period of oscillation.

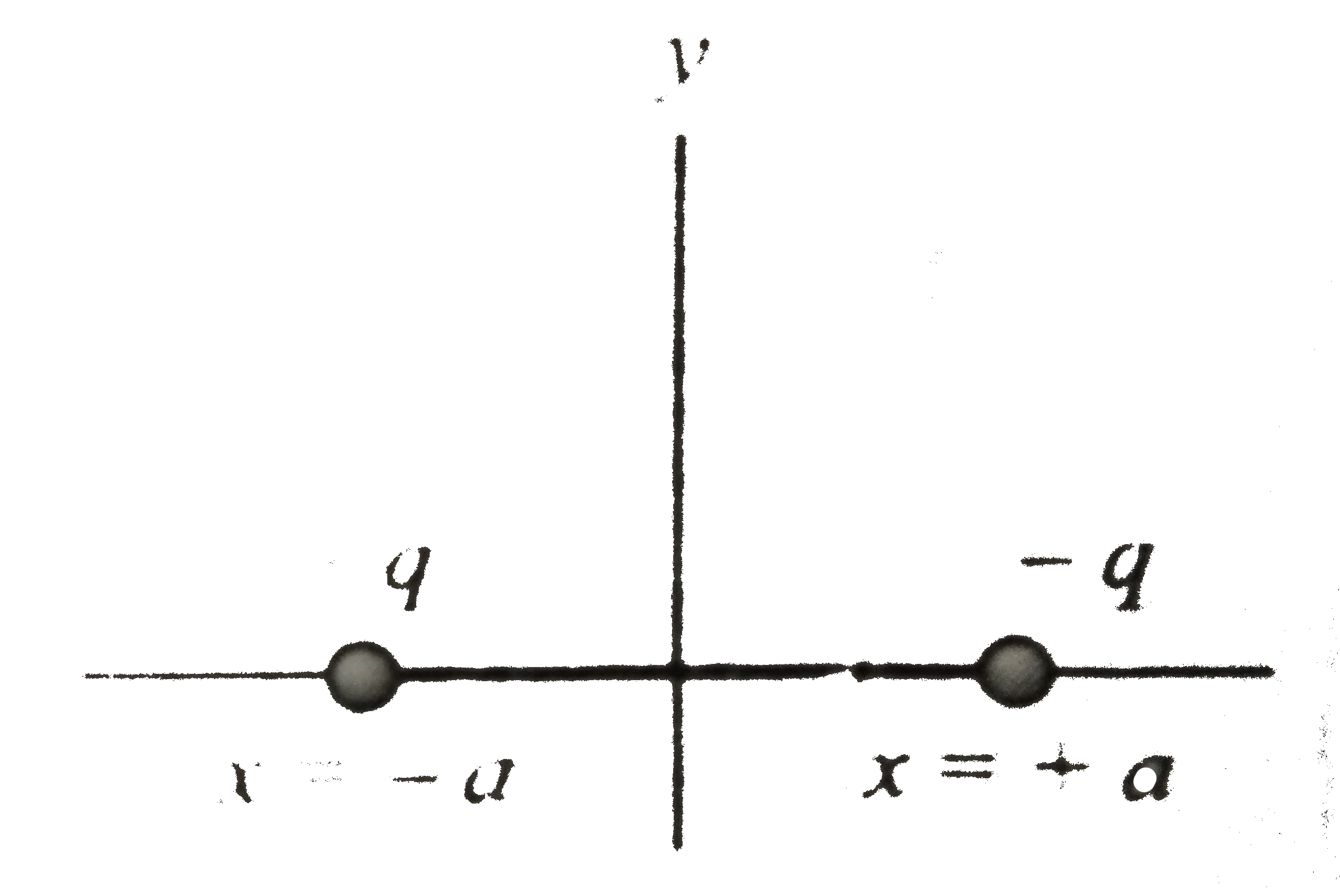
View SolutionTwo point charge `+-q` are placed on the axis at `x= -a` and `x= +-a`, as shown in fig.
(i) Plot the variation of E along the x-axis.
(ii) Plot the variation of E along the y-axis.
View SolutionTwo negative charges of a unit magnitude and a positive charge q are placed along a straight line. At what position and value of q will the system be in equilibrium? (Negative charges are fixed).
View SolutionTwo point charges `+5xx10^(-19)C and +20xx10^(-19)C` are separated by a distance of 2m. The electric field intensity will be zero at a distance d=………. From the charges of `5xx10^(19)C`.
View SolutionThe positions of two point charges `q_(1)` and `q_(2)` are `vecr_(1)` and `vecr_(2)` , respectively. Find the position of the point where the net field is zero due to thses charges.
View SolutionA rod of length l, has a uniform positive charge per unit length and a total charge Q. calculate the electric foeld at a point P located along the long axis of the rod and at a distance a from one end

View SolutionFind the electric field at the centre of a uniformly charged semicircular ring of radius R. Linear charge density is `lamda`
View SolutionA long wire with a uniform charge density `lambda` is bent in two configurations shown in fig. Determine the electric field intensity at point O.
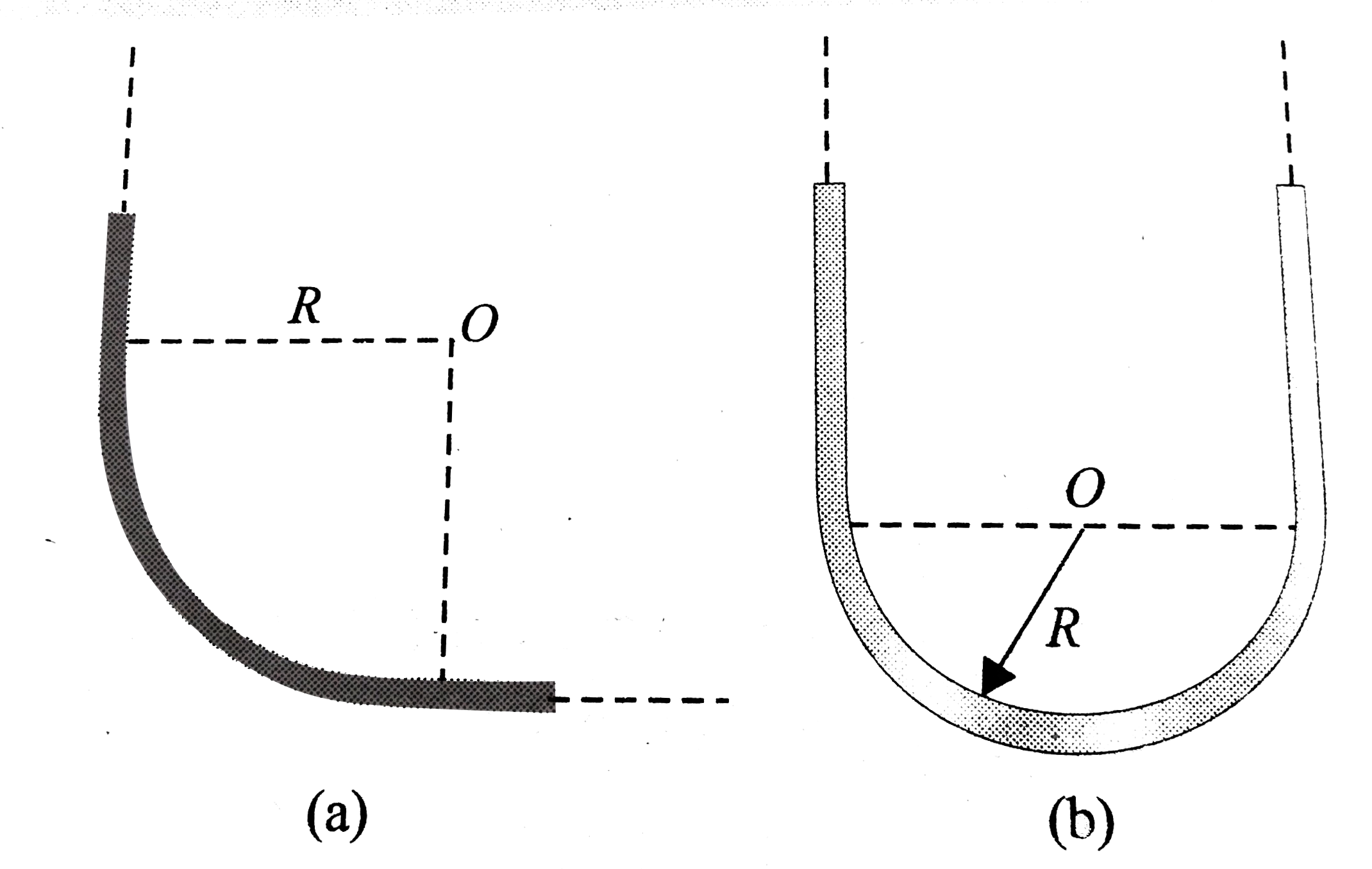
View SolutionA segment of a charged wire of length l, charge density `lambda_(2)` and an infinitely long chaged wire. Charge density `lambda_(1)`. Lie in a plate at right angles to each other. The sepatation between the wires is `r_(0)`. Determine the force of interction between the wires.
View SolutionAn infinite dielectric sheet having charge density `sigma` has a holeof radius R in it. An electrom is released from point P on the axis of the hole at a distance `sqrt(3)R` from the center. Find the speed with which it crosses the plane of the sheet.
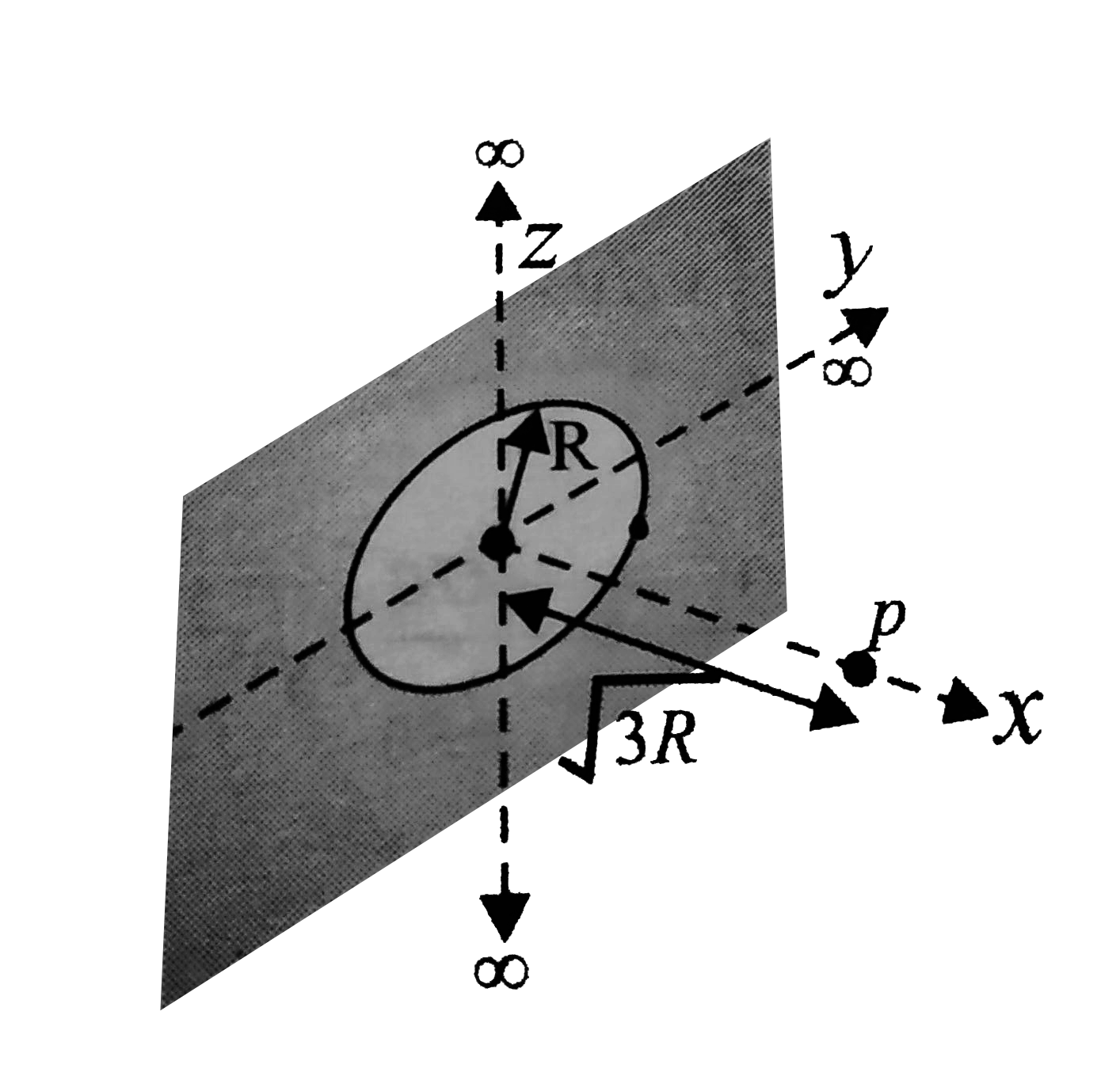 .
.
View SolutionCharges `+q` and `-2q` are fixed at distance d apart as shown in fig.
(i) sketch roughly the pattern of electric field lines, showing the positon of neutral point.
(ii) Where should a charge partical q be placed so that it experience no force ?
 .
.
View SolutionThe field lines for two point charges shown in fig.

(i) Is the field uniform?
(ii) Determine the ratio `q_(A)//q_(B)`.
(iii) What are the sign of `q_(A)` and `q_(B)` ?
(iv) Apart from infinity, where is the neutral point?
(v) If `q_(A)` and `q_(B)`are separated by a distance `10(sqrt(2)-1)` cm, find the position of neutral point.
(vi) where will the lines meet which are coming from A and are not meeting at `q_(B)`?
(vii) Will a positive charge follow the line of force if free to move?
View SolutionA particle of mass ma and charge q is released from rest in a uniform filed of magnitude E. the uniform field is created between two parallel plates of charge densities `+sigma` and `-sigma`, respectively. The particle accelerates toward the other plate a distance d apart. Determine the speed at which it stirkes the opposite plate.

View SolutionA uniform E exiest between two metal plates one negative and other positive. The plate length is l and the seperation of the plates is d.
(i) An electron and a proton start fron the negative plate and positive plane, respactively, and go the opposite plates. which one of them wins this race?
(ii) An electron and a proton start moving parallel to the plates towards the other end from the midpoint of the separation of plates at one end of the plates. Which of two will have greater deviation when they come out of the plates if they start with the
(a) same initial velocity
(b) same initial kinetic energy, and (c ) same initial momentum.
View SolutionA partical having charge that of an electron and mass `1.6xx10^(-30)`kg is projected with an initial speed u at the angle `45^(@)` to the horizontal from the lower plate of a parallel-plate capacitor as shown in fig. The plates are suffiecienly long and have a separation of 2 cm, find the maximum value of the velocity of he particle so that it does not hit the upper plate. Take the electric field between the plates as `10^(3)Vm^(-1)` directed upward.

View SolutionA system has two charges `q_(A)= +2.5xx10^(-7)C and q_(B)= +2.5xx10^(-7)C` located at point A: (0,0,-15 cm)and B: (0,0,+15 cm)`, respectively. What are the total charge and eletric dipole moment of the system?
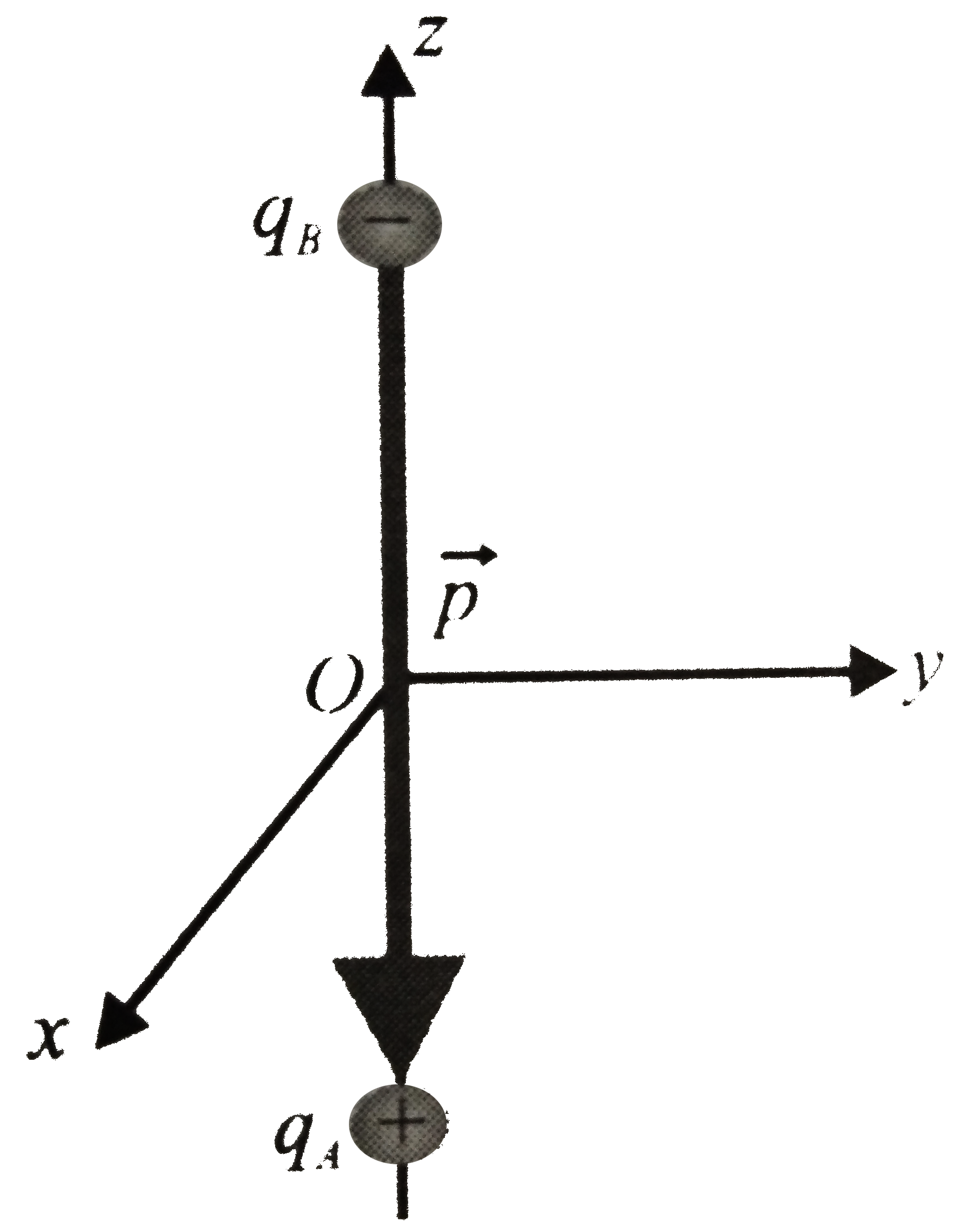
View SolutionThree charges +q, +q and -2q are placed at the vertices of an equilareral triagle. What is the dipole moment of the system?
View SolutionThree charges -q,+2q, and -q are arranged on a line as shown in fig. Calculate the field at a distance rgtgta on the line.
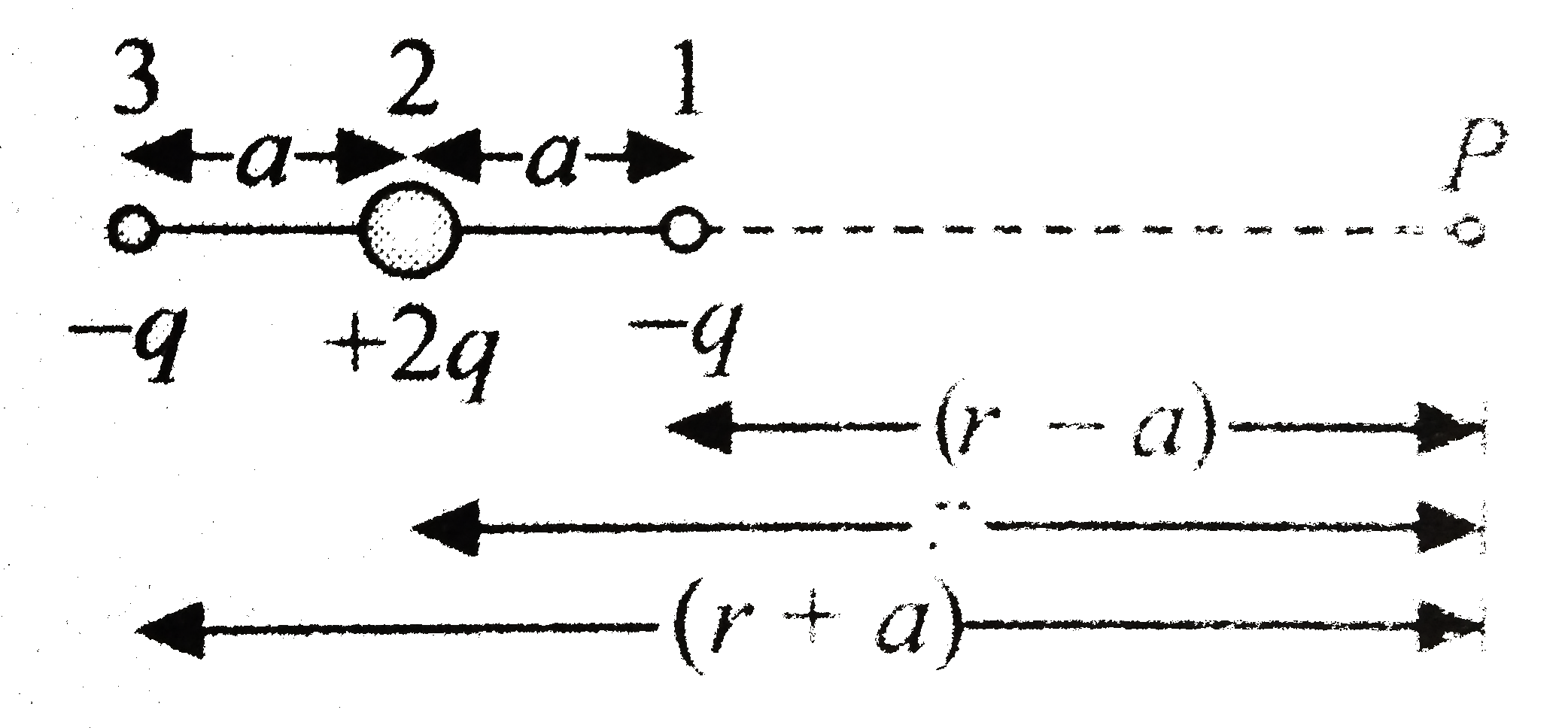
View SolutionWhat is the force on a dipole of dipole moment p placed as shown in fig.

View SolutionAn electric dipole of dipole miment `vec(p)` is placed in a uniform electric field `vec(E )`. Write the expression for the torque `vec tau` experienced by the dopole. Identify two pairs of perpendicular vectors in the expression. Show diagrammatically the orientation of hte dipole in the field for which the torque is (i) maximum and (ii) half the minimum value, and (iii) zero.
View SolutionAn electric dipole consists of two charges of `0.1muC` separated bu a distance of `2.0cm`. The dipole is placed in an external field of `10^(5)NC^(-1)`. What maximum torqur does the field exert on the dipole?
View SolutionIn a certain region of space, electric field is along the z-direction throughout. The magnitude of electric field is, however not constant but increases uniformly along the positive z-direction at the rate of `10^(5)NC^(-1)m^(-1)`. What are the force and torque experienced by a system having a total dipole moment equal to `10^(-7)` Cm in the negative z-direction?
View Solution
Illustration questions and complete solutions for chapter COULOMB LAW AND ELECTRIC FIELD of CENGAGE PHYSICS (HINGLISH) of Class 12