Complete Solutions to Subjective of chapter ELECTRIC POTENTIAL of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | ELECTRIC POTENTIAL |
| CENGAGE PHYSICS | Subjective |
| Number Of Questions | 28 |
| Academic Year | 2023-24 |
The variation of electric potential for an electric field directed parallel to the x - axis is shown in (Fig. 3.110). Draw the variation of electric field strength with the x- axis.
 .
.
View SolutionIf identical charges `(-q)` are placed at each corner of a cube of side `b`, then electric potential energy of charge `(+q)` which is palced at centre of the cube will be
View SolutionAt s point due to a point charge, the values of electric field intensity and potential are `32 NC^-1` and `16 JC^-1`, respectively. Calculate the
a. magnitude of the charge, and
b. distance of the charge from the point of observation.
View SolutionFour charges `+q, -1, +q` and `-q` are placed in order on the four consecutive corners of a square of side a. The work done in interchanging the positions of any two neighbouring charges of the opposite sign is
View SolutionWater from a tap, maintained at a costant potential V, is allolwed to fall by drops of radius r through a small hole into a hollow conducting sphere of radius R standing on an insulating stand until it fills the entire sphere. Find the potential of the hollow conductor after it is completely filled with water.
View SolutionThree charges ` 0.1` coulomb each are placed on the corners of an equilateral triangle of side `1 m`. If the energy is supplied to this system at the rate of ` 1 kW` how much time would be required to move one to the charges on to the midpoint of the line joining the two ?
View Solution(Figure 3.111). Shows a large conducting ceiling having uniform charge particle of charge density `sigma` below which a charge particle of charge `q_0` and mass m is hung from point O, through a small string of length l. Calculate the minimum horizontal velocity v required for the string to become horizontal.
 .
.
View SolutionA nonconducting sphere of radius `R = 5 cm` has its center at the origin O of the coordinate system as shown in (Fig. 3.112). It has two spherical cavities of radius `r = 1 cm`, whose centers are at `0,3 cm` and `0,-3 cm`, respectively, and solid material of the sphere has uniform positive charge density `rho = 1 // pi mu Cm^-3`. Calculate the electric potential at point `P (4 cm, 0)`.
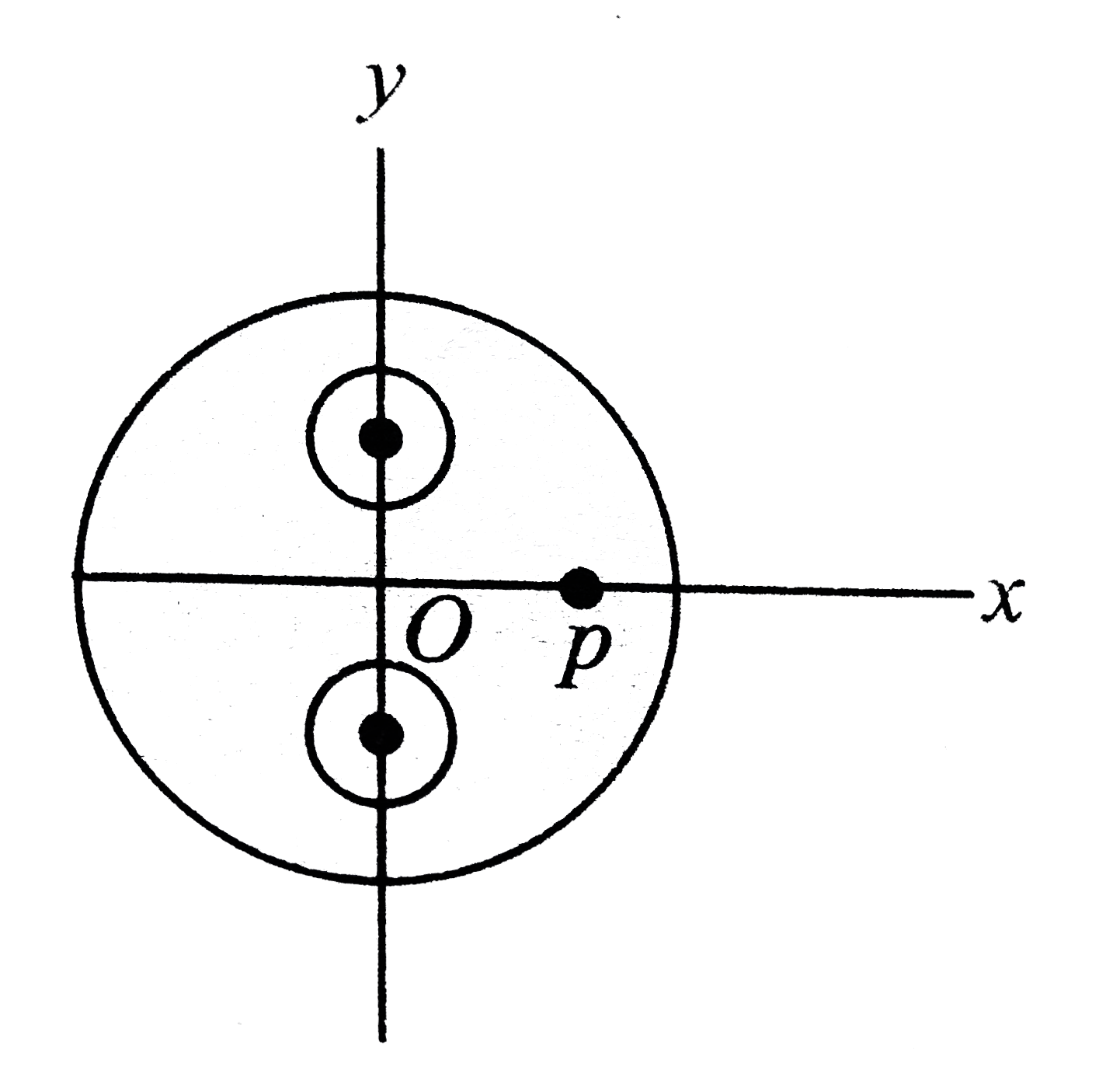 .
.
View SolutionTwo identical thin ring, each of radius R meters, are coaxially placed a distance R metres apart. If `Q_1` coulomb, and `Q_2` coulomb, are repectively the charges uniformly spread on the two rings, the work done in moving a charge q from the centre of one ring to that of the other is
View SolutionThree conducting spherical shells have radii (a, b, and c) such that `a lt b lt c` (Fig. 3.113). Initially, the inner shell is uncharged, the middle shell has a positive charge Q, and the outer shell has a negative charge `-Q`.
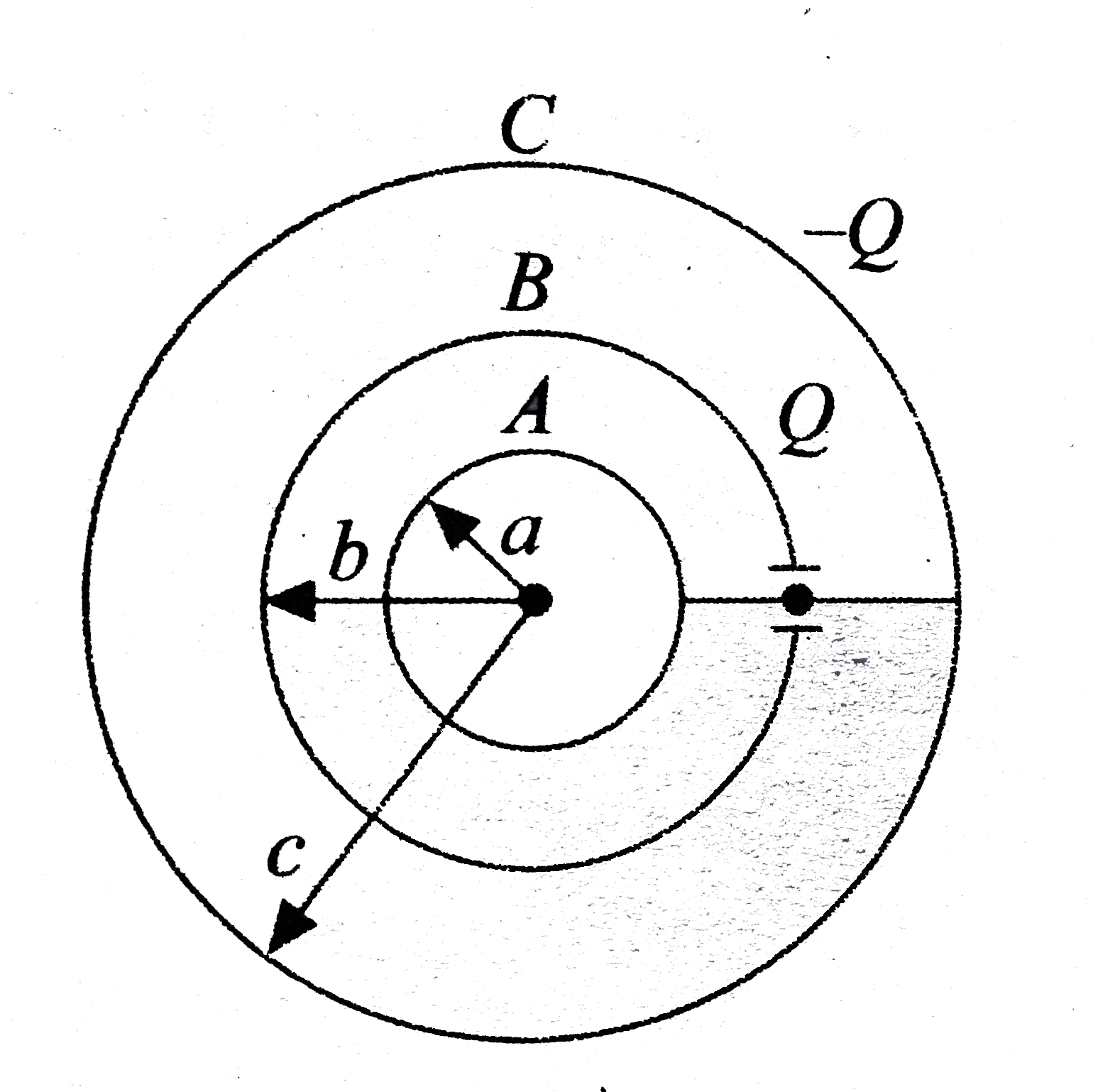 .
.
a. Find the electric potential of the three shells.
b. If the inner and outer shells are now connected by a wire that is insulated as it passes through the middle shell, what is the electric potential of each of the three shells ? Also, what is the final charge on each shell ?
View Solution(Figure 3.114) shows three concentric spherical conductors `A, B, and C` with radii `R, 2R`, and `4R`, respectively. A and C are connected by a conducting wire, and `B` is uniformly charged `("charge" = +Q)`. Find
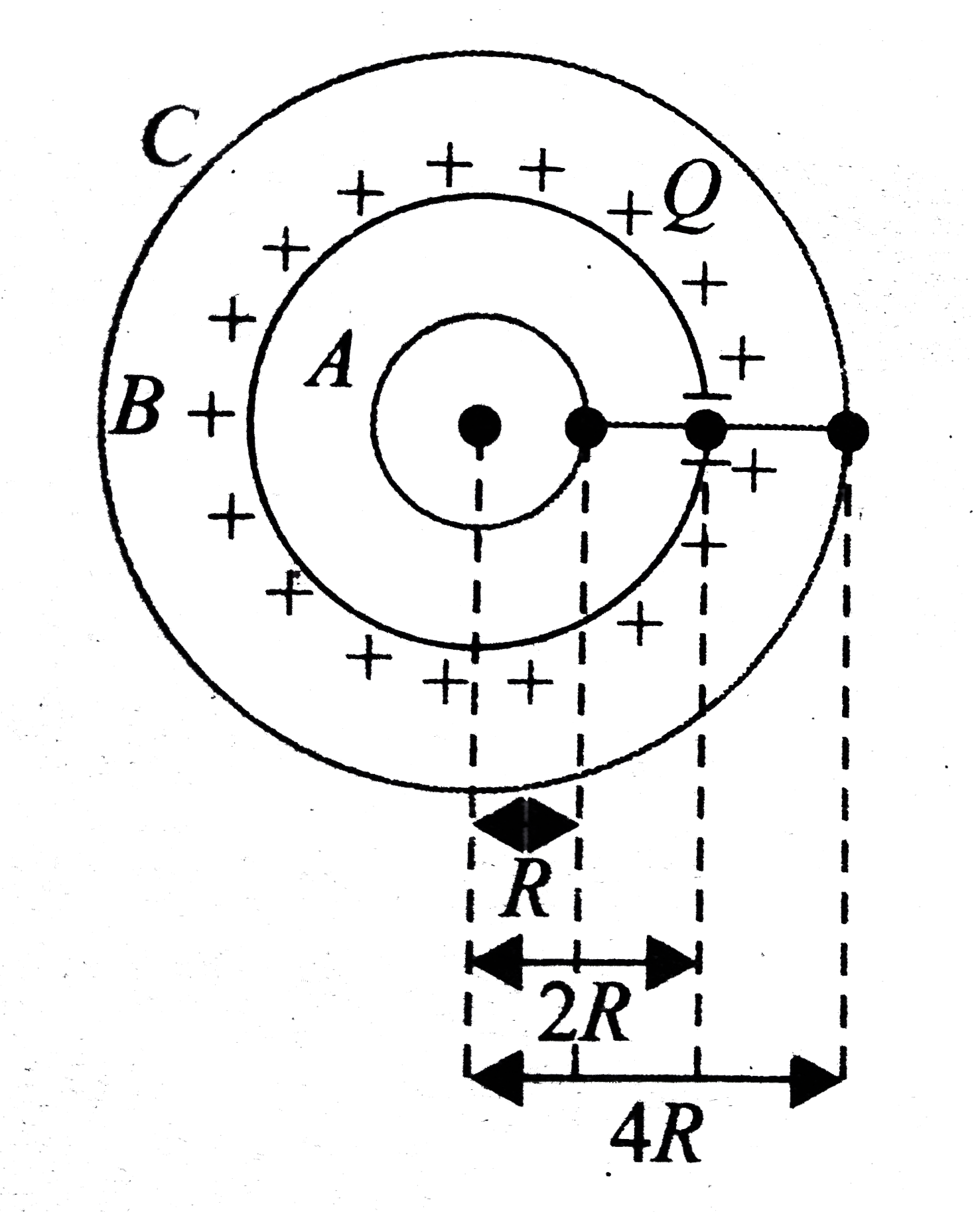 .
.
a. charges on conductors A and C, and
b. potentials of A and B.
View SolutionTwo concentric shells of radii `R` and `2 R` are shown in (Fig. 3.115). Initially, a charge q is imparted to the inner shells. Now, key `K_1` is closed and opened and then key `K_2` is closed and opened. After the keys `K_1 and K_2` are alterbately closed n times each, find the potential difference between the shells. Note that finally key `K_2` remains closed.
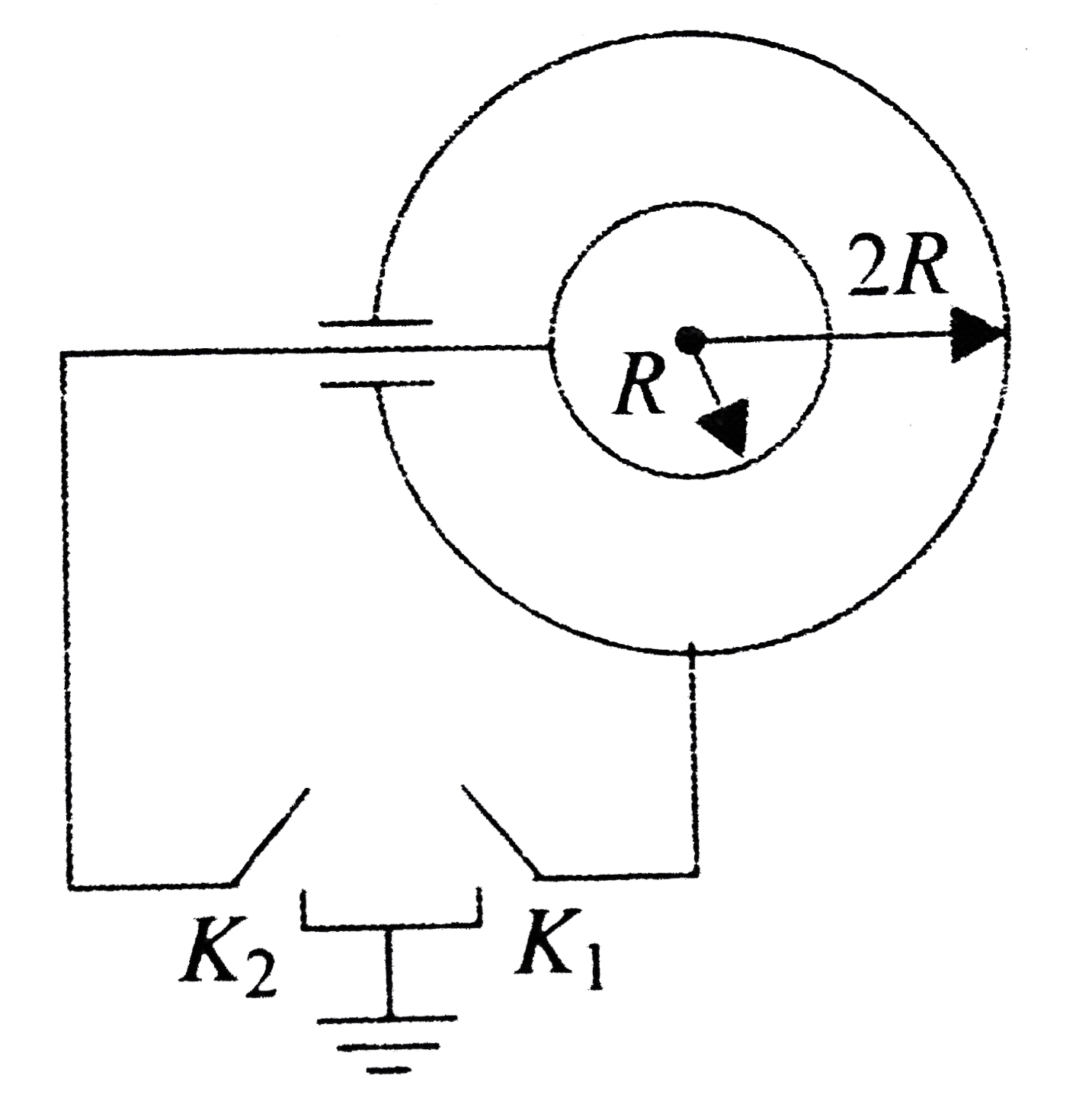 .
.
View SolutionThree charges each of value q are placed at the corners of an equilateral triangle. A fourth charge Q is placed at the center of the triangle.
a. Find the net force on charge q.
b. If `Q = -q`, will the charges at the corners move toward the center or fly away from it ?
c. For what value of Q and O will the charges remain stationary ?
d. In situation (c), how much work is done in removing the charges to infinity ?
View SolutionA small ball of mass `2 xx 10^-3 kg`, having a charge `1 mu C`, is suspended by a string of length `0.8 m`. Another identical ball having the same charge is kept at the point of suspension. Determine the minimum horizontal velocity that should be imparted to the lower ball so that it can make a complete revolution.
View SolutionTwo charges `-2Q` abd `Q` are located at the points with coordinates `(-3a, 0)` and `(+3a, 0)` respectively in the x-y plane. (i) Show that all points in the x-y plane where the electric potential due to the charges is zero, on a circle. Find its radius and the location of its centre (ii) Give the expression V(x) at a general point on the x-axis and sketch the function V(x) on the whole x-axis. (iii) If a particle of charge +q starts from rest at the centre of the circle, shown by a short quantitative argument that the particle eventually crosses the circule. Find its speed when it does so.
View SolutionA point charge `q` is located at the centre `O` of a spherical uncharged coducting layer provided with small orifice. The inside and outside radii of the layer are equal to a and `b` respectively. The amount of work that has to be performed to slowly transfer the charge `q` from teh point `O` through the orifice and into infinity is

View SolutionFour point `+ 8 mu C, -1 mu C, -1 mu C`, and `+ 8 mu C` are fixed at the points `-sqrt(27//2) m, - sqrt(3//2) m, +sqrt(3//2) m`, and `+sqrt(27//2) m`, respectively, on the y - axis. A particle of mass `6 xx 10^-4 kg` and charge `+ 0.1 mu C` moves along the x- direction. Its speed at `x = + oo` is `V_0`. Find the least value of `V_0` for which the particle will cross the origin. Find also the kinetic energy of the particle at the origin. Find also the kinetic energy of the particle at the origin. Assume that space is gravity free. Given `(1// 4 pi epsilon_0) = 9 xx 10^9 Nm^2 C^-2`.
View SolutionCharges `+q` and `-q` are located at the corners of a cube of side as show in the figure. Find the work done to separate the charges to infinite distance
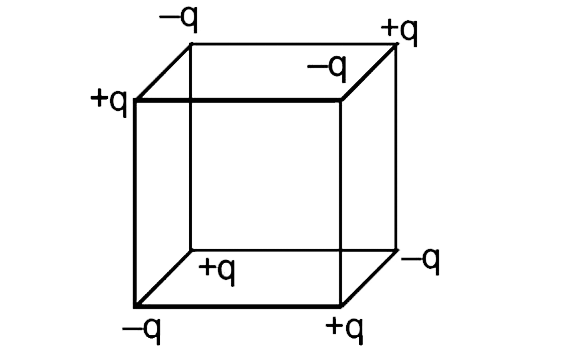
View SolutionTwo uniformly charged large plane sheets `S_1` and `S_2` having charge densities `sigma_1` and `sigma_2` (`sigma_1gtsigma_2`) are placed at a distance d parallel to each other. A charge `q_0` is moved along a line of length a(altd) at an angle `45^@` with the normal to `S_1`. Calculate the work done by the electric field
View Solution(Figure 3.118) shows two dipole moments parallel to each other and placed at a distance x apart. What is the magnitude of force of interaction ? What is the nature of force, attractive or repulsive ?
 .
.
View SolutionTwo dipoles `p_1 and p_2` are placed along the same axis at a distance x apart as shown in (Fig. 3.119). What is the magnitude of the force of interaction ? What is the nature of force, attractive or repulsive ?
 .
.
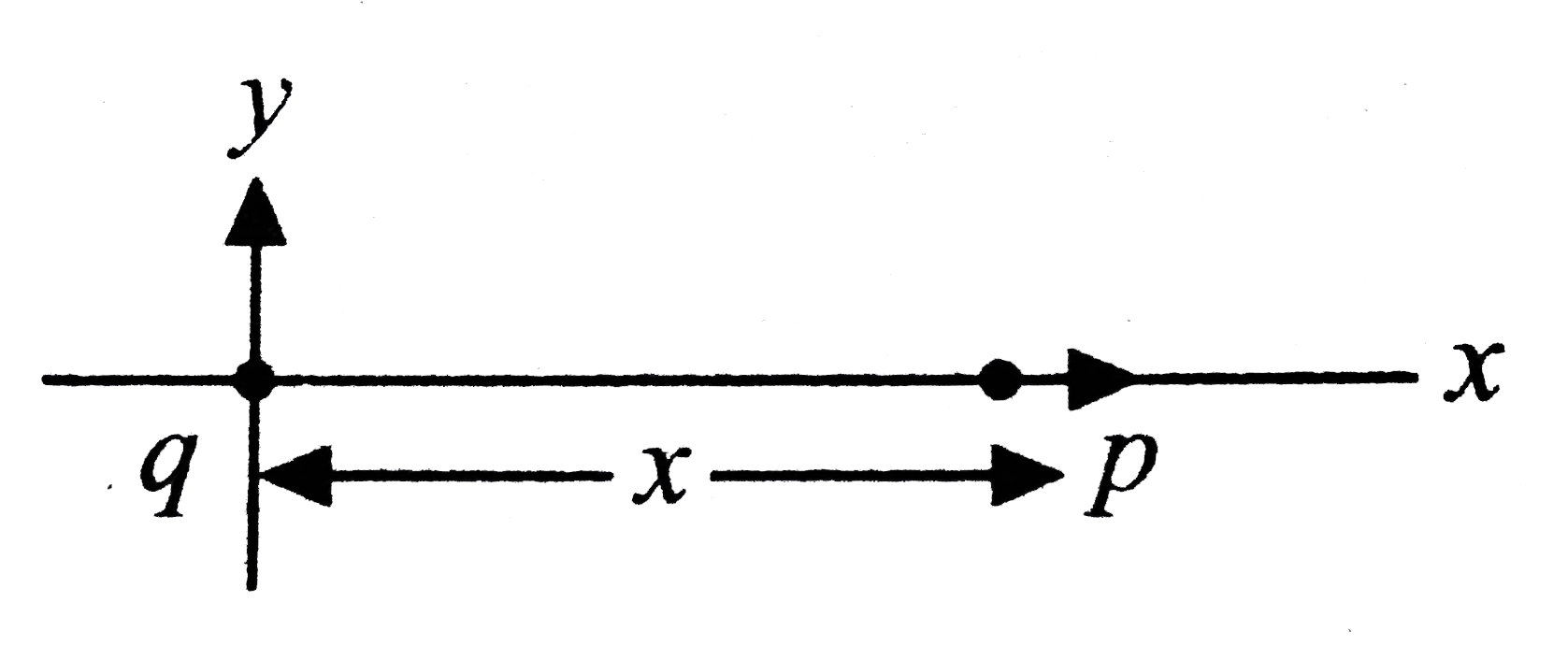
View SolutionA short dipole is placed along the x - axis at `x = x` (Fig. 3.120).
 .
.
a. Find the force acting on the dipole due to a point charge q placed at the origin.
b. Find the force on the dipole if the dipole is rotated by `180^(@)` about the z- axis.
c. Find the force on dipole if the dipole is rotated by `90^(@)` anticlockwise about z - axis, i.e., it becomes parallel to the y - axis.
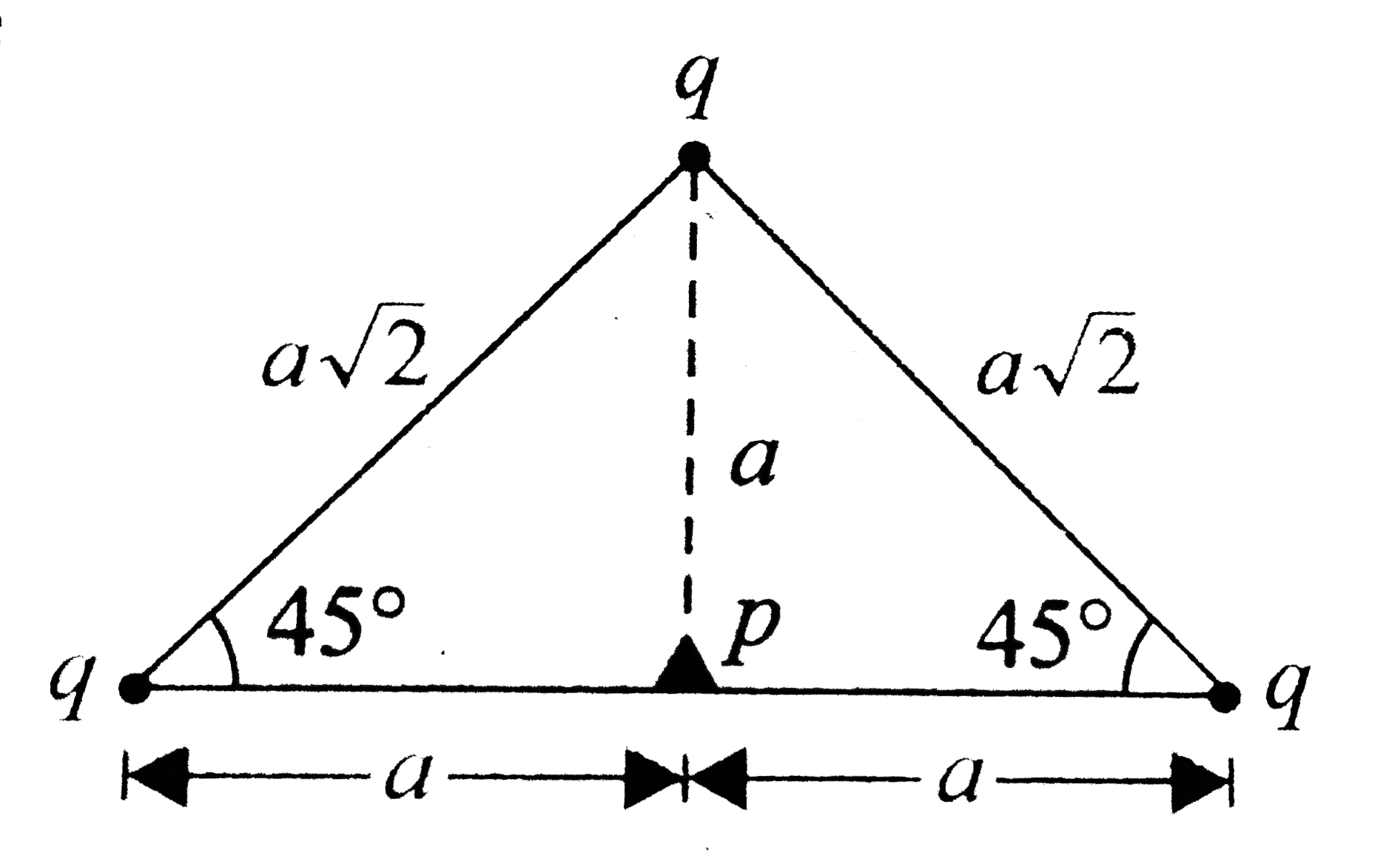
View SolutionFor the electrostatic charge system as shown in , find
 .
.
a. the net force on electric dipole, and
b. electrostatic energy of the system.
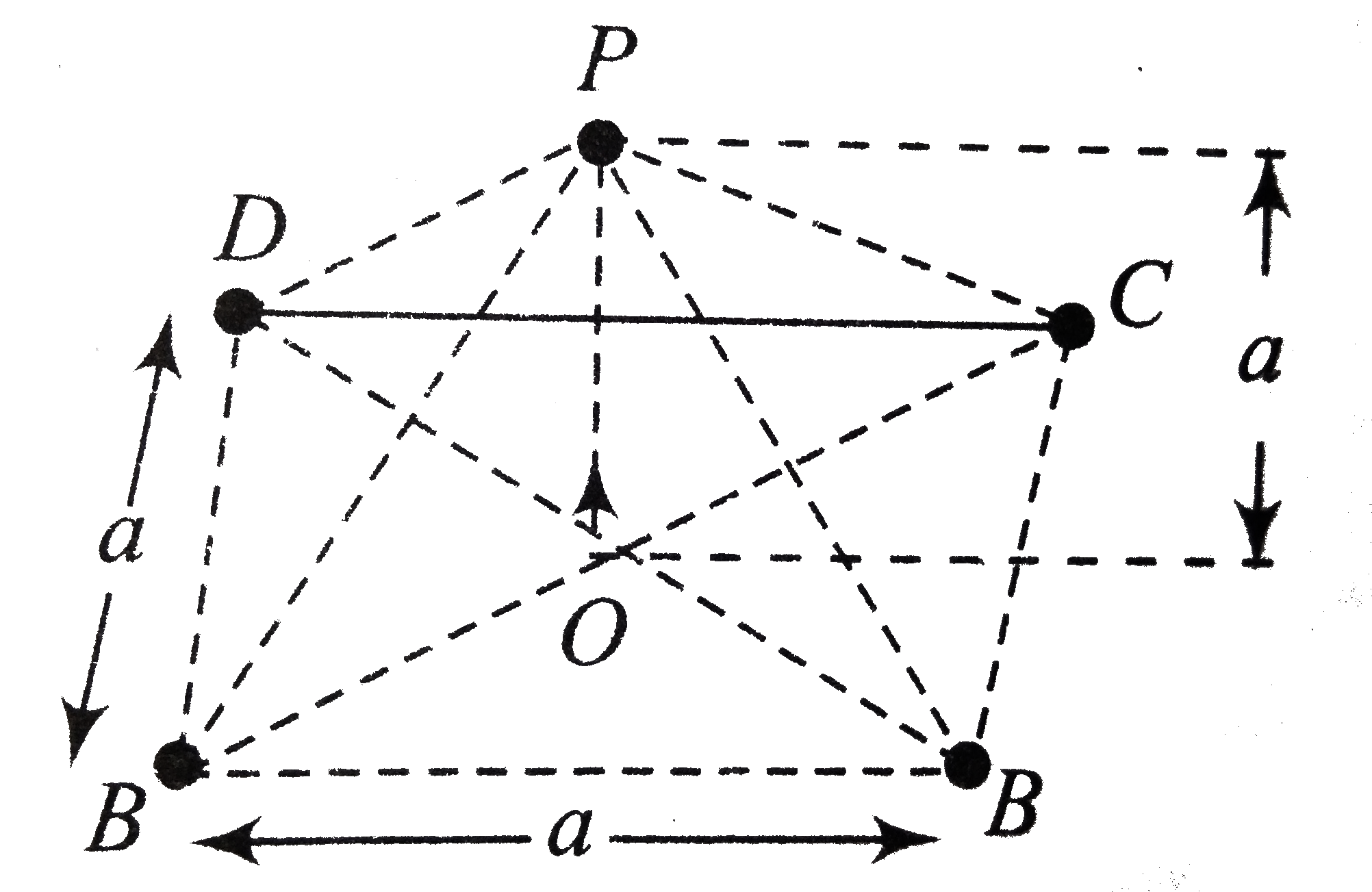
View SolutionFour charge particles each having charge Q=1 C are fixed at the corners of the base(A,B,C, and D) of a square pyramid with slant length `a(AP=BP=PC=a=sqrt(2)m)`, a charge -Q is fixed at point P. A dipole with dipole moment p=1 Cm is placed at the center of the bases and perpendicular to its plane as shown in fig. Force on the dipole due to the charge particles is `(square)/(4 pi epsilon_(0))N`.
View SolutionThree identical dipoles with charges q and `-q`, and separation A between the charges, are placed at the corners of an equilateral triangle of side D as shown in fig. Find the interaction energy of the system `(a lt lt d)`.
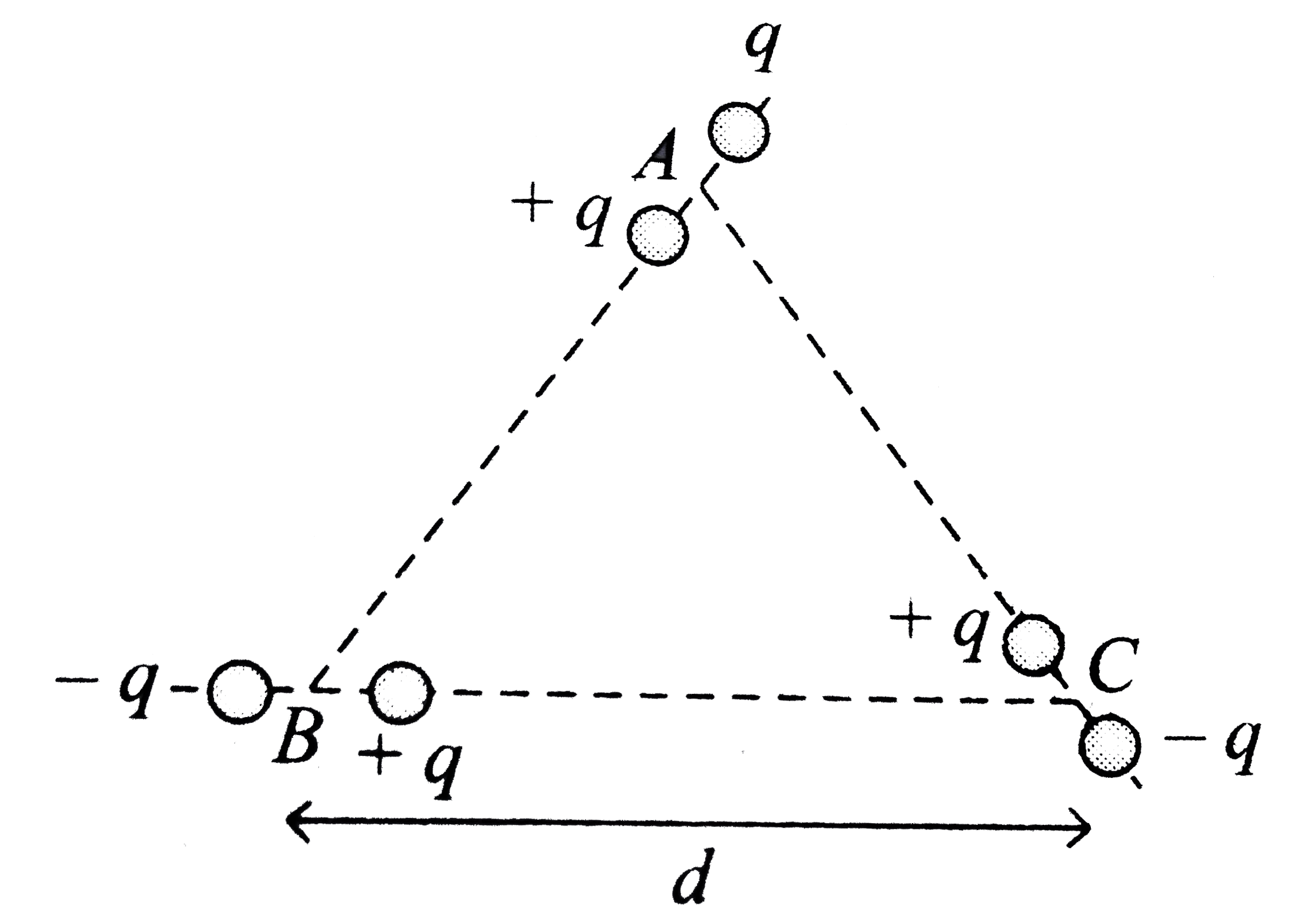 .
.
View SolutionA radioactive source, in the form of a metallic sphere of radius `10^(-2)m` emits `beta-`particles at the rate of `5xx10^(10)` particles per second. The source is electrically insulated. How long will it take for its potential to be raised by `2V`, assuming that `40%` of the emitted `beta-`particles escape the source.
View SolutionThree charges ` 0.1` coulomb each are placed on the corners of an equilateral triangle of side `1 m`. If the energy is supplied to this system at the rate of ` 1 kW` how much time would be required to move one to the charges on to the midpoint of the line joining the two ?
View SolutionWhen a small uncharged conducting ball of radius `a = 1 cm` and mass `m = 50 g` is dropped from a height h above the center of another large conducting sphere of radius `b( = 1 m)` having charge `Q( =(100 mu C)`. It rises to a height `h_1 (= 2 m)` after the collision. Find the value of h. Assume that during the impact there is no dissipation of energy.
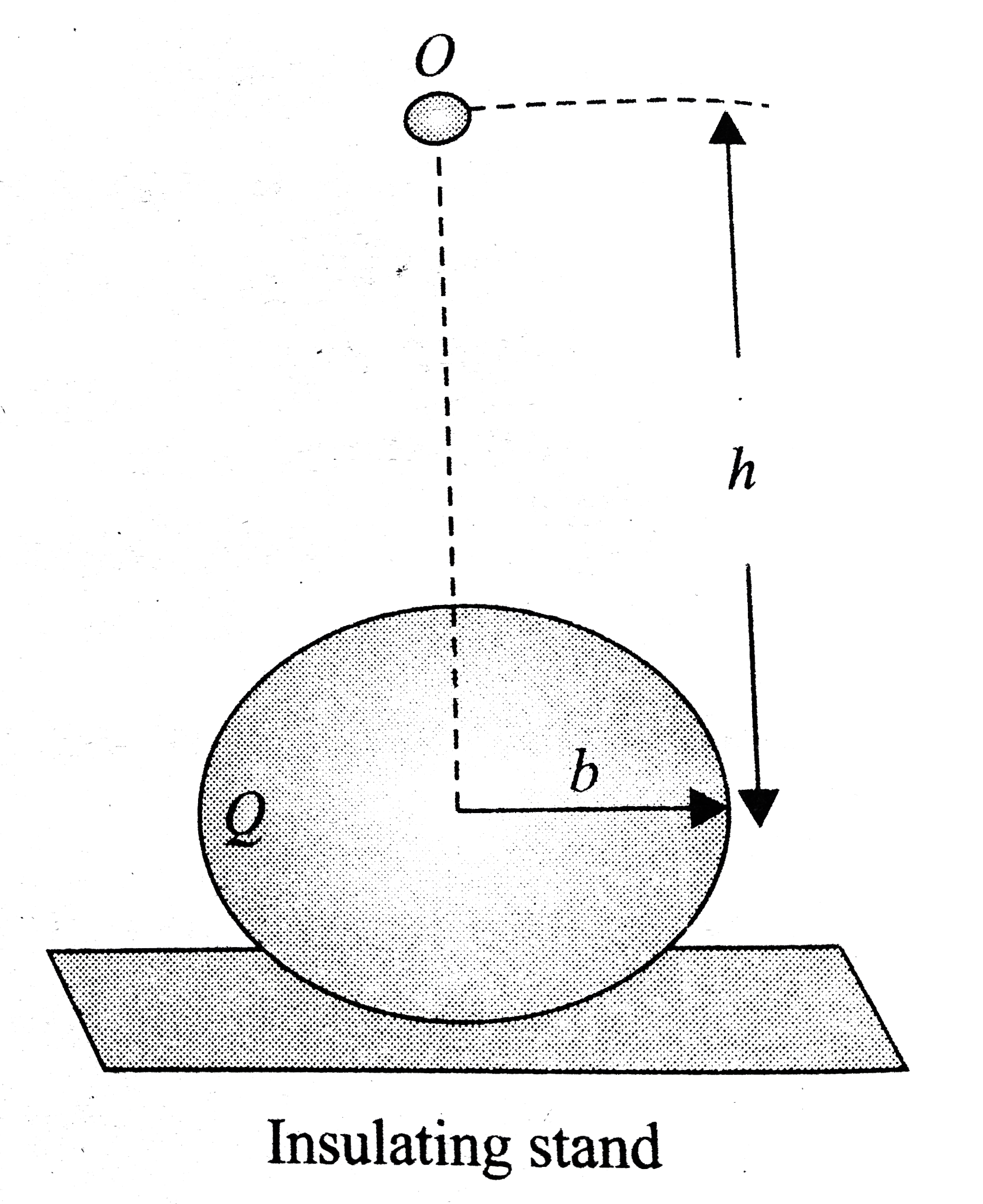 .
.
View Solution
Subjective questions and complete solutions for chapter ELECTRIC POTENTIAL of CENGAGE PHYSICS (HINGLISH) of Class 12