Complete Solutions to All Questions of chapter ELECTROMAGNETISM of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | ELECTROMAGNETISM |
| D MUKHERJEE | All Questions |
| Number Of Questions | 91 |
| Academic Year | 2023-24 |
If `C` the velocity of light, which of the following is correct?
View SolutionIf `E` and `B` denote electric and magnetic fields respectively, which of the following is dimensionless?
View SolutionA vertical wire carriers a current upwards. The magnetic field at a point due north of the wire is directed
View SolutionTwo parallel beams of protons and electrons, carrying equal currents are fixed at a separation d. The protons and electrons move in opposite directions. There is a point P on the straight perpendicular line joining the two beams at a distance x from one beam. The magnetic field at this point is B. If B is plotted against x, it can be represented by the curve.
View Solution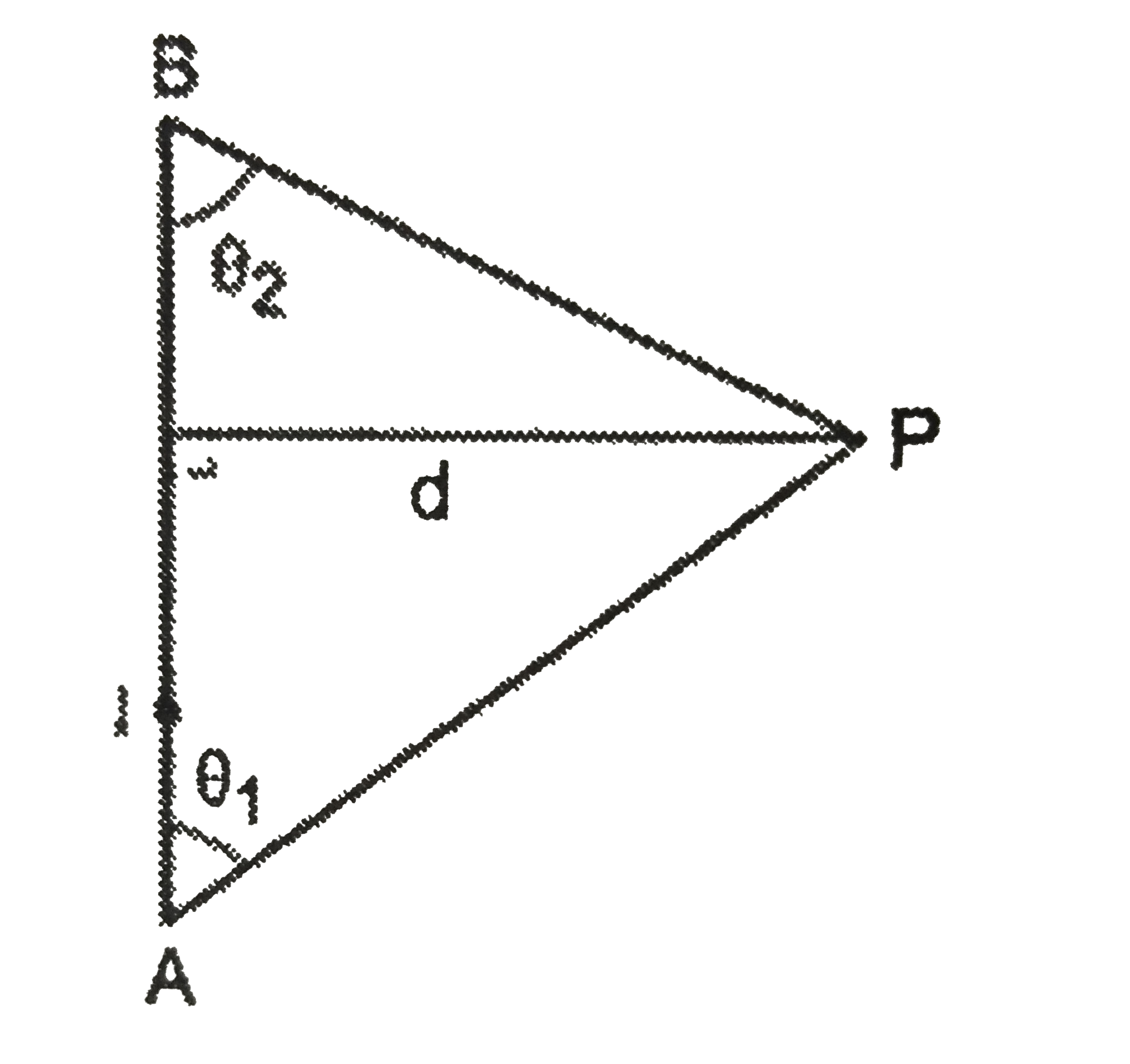
`AbB` is a section of a straight wire carrying a current `I`. `P` is a point at a distance `d` from `AB`. The magnetic field at `P` due to `AB` has magnitude
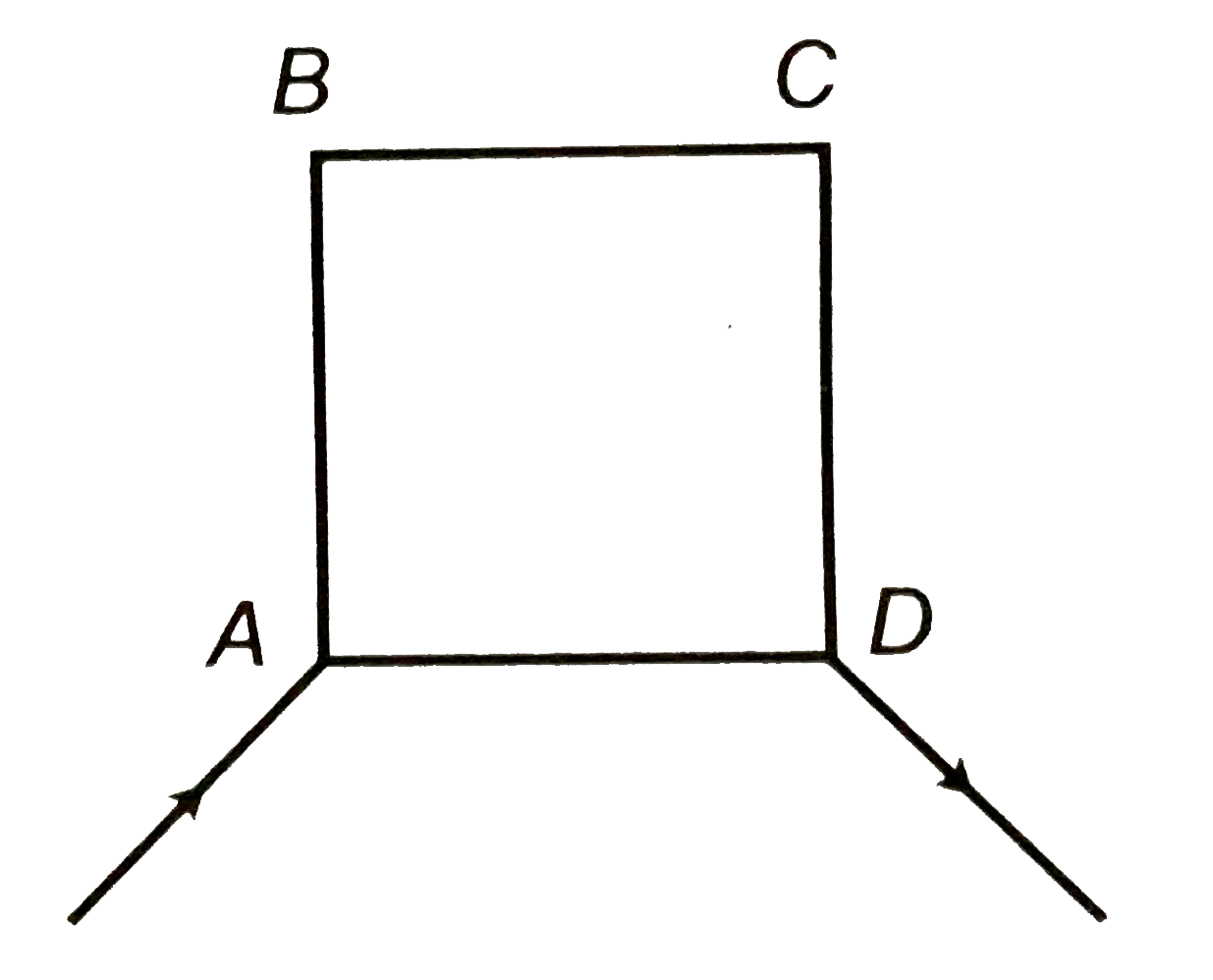
View Solution`ABCD` is a square loop made of a uniform conducting wire. A current enters the loop at `A` and leaves at `D`. The magnetic field is
View SolutionTwo long thin wires ABC and DEF are arranged as shown in Fig. They carry equal currents I as shown. The magnitude of the magnetic field at O is
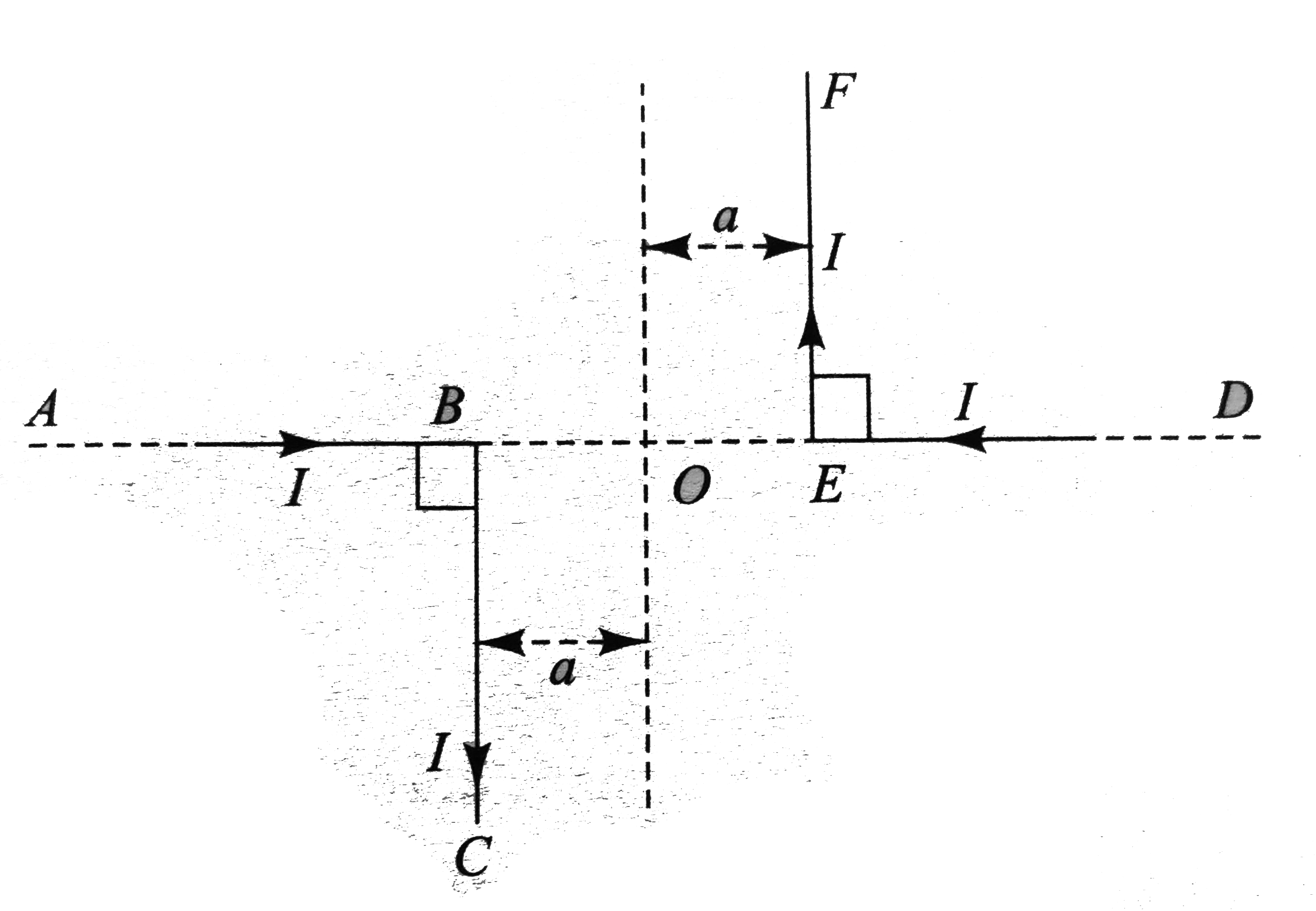
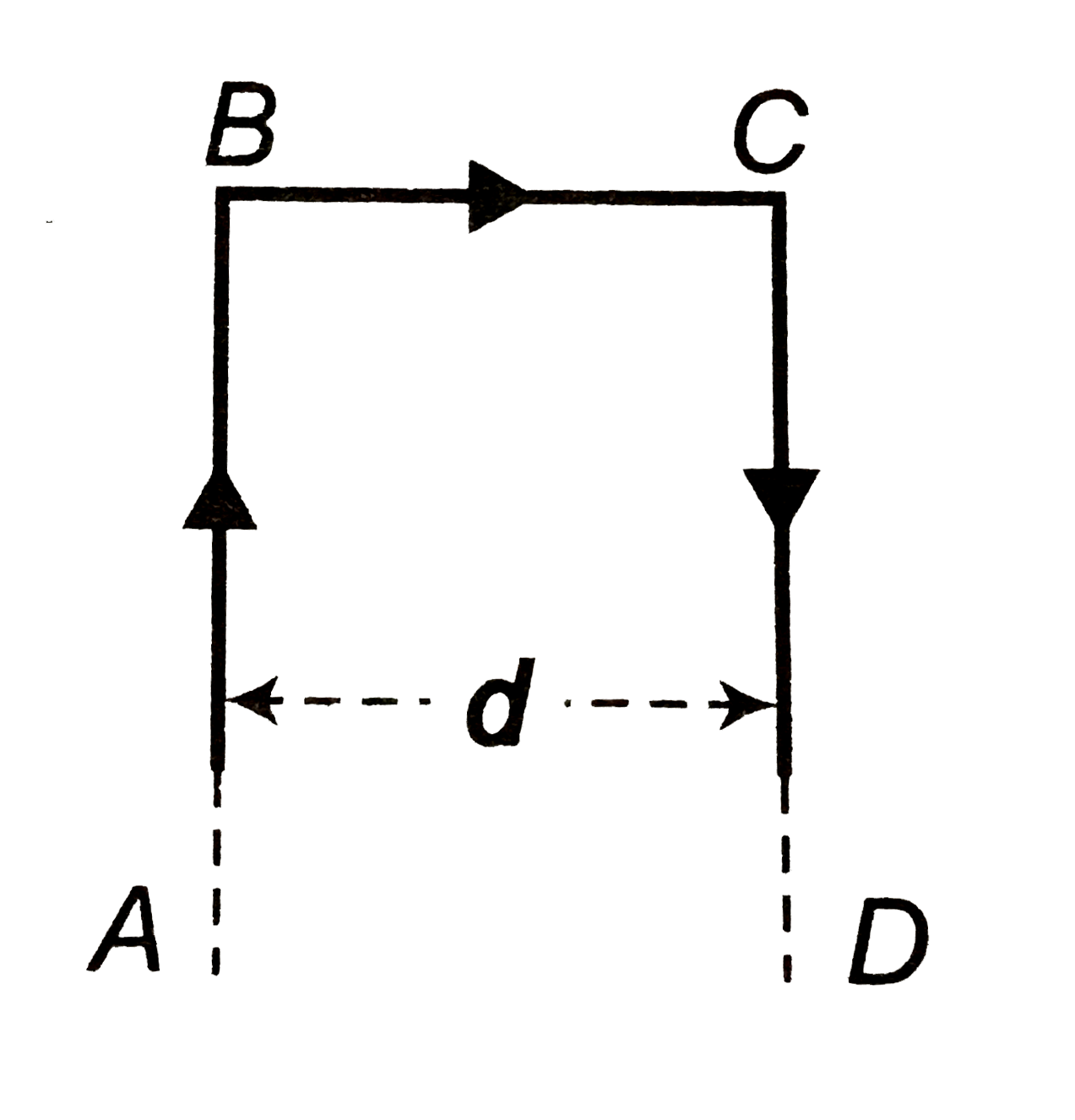
View Solution`AB` and `CD` are long staright conductors, distance `d` apart, carrying a current `I`. The magnetic field on `BC` due to the currents in `AB` and `CD`
View SolutionA wire carrying current `I` is shaped as shown. Section `AB` is a quarter circle of radius `r`. The magnetic field is directed
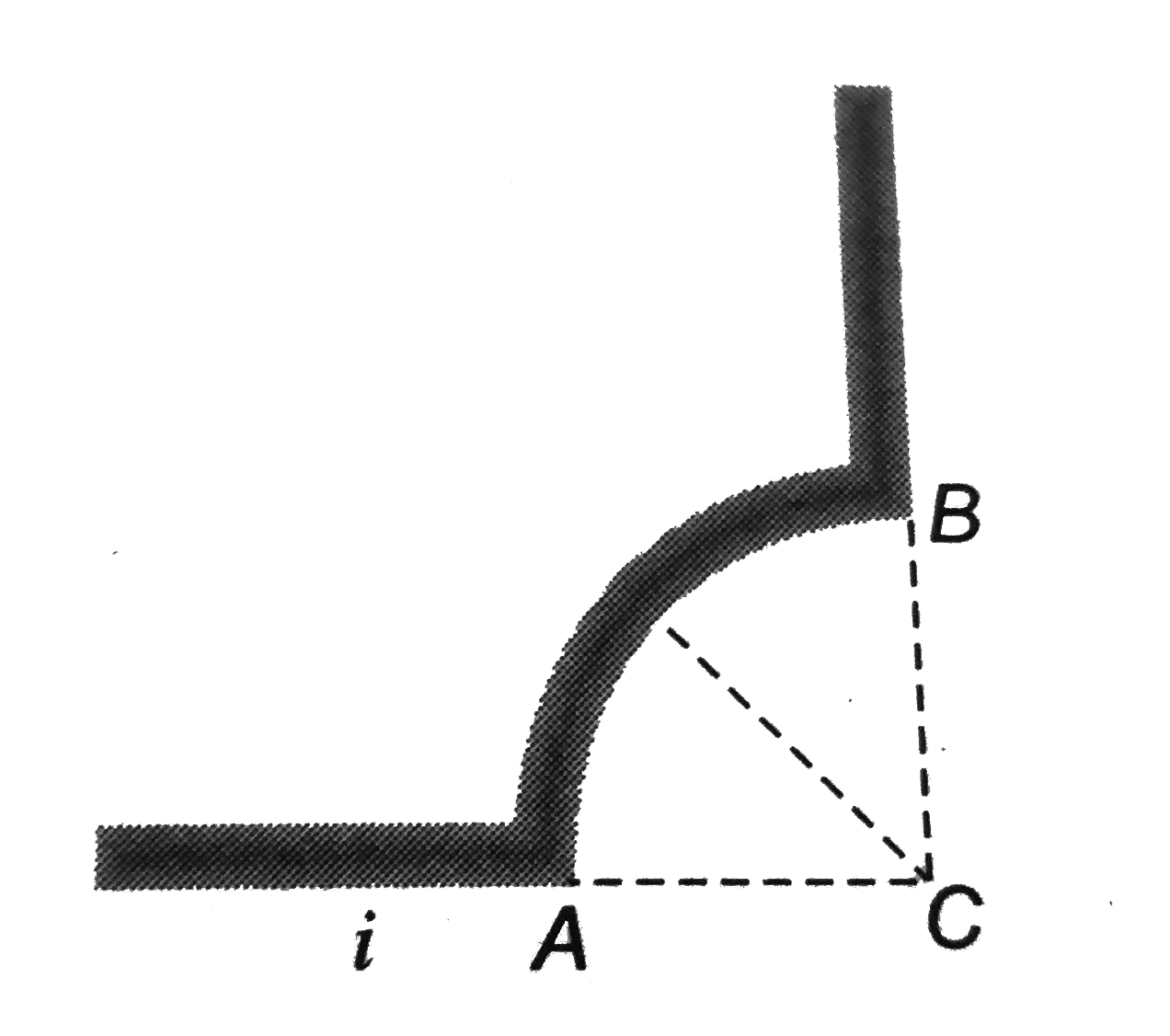
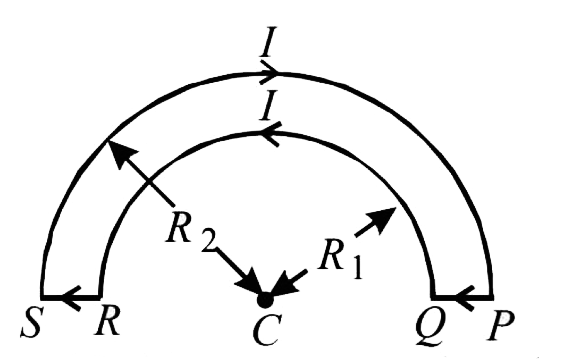
View SolutionThe wire loop `PQRSP` formed by joining two semicircular wires of radii ` R_(1)` and `R_(2)` carries a current `I` as shown . The magnitude of the magnetic induction at the center `C` is ………..
View SolutionAn electron moving in a circular orbit of radius `r` makes `n` rotation per secound. The magnetic field produced at the centre has magnitude
View SolutionA circular coil of n turns and radius r carries a current I. The magnetic field at the centre is
View SolutionA point `P` lies on the axis of a flat coil carrying a current. The magnetic moment of the coil is `mu`. The distance of `P` from the coil is `d`, which is large compared to the radius of the coil. The magnetic field at `P` has magnitude
View SolutionCurrent flows through a straight cylindrical conductor of radius r. The current is distributed uniformly over its cross-section. The magnetic field at a distanace x from the axis of the conductor has magnitude B:
View SolutionA coaxial cable consists of a thin inner conductor fixed along the axis of a hollow outer conductor. The two conductor carry equal currents in opposite directions. Let `B_1 and B_2` be the magnetic fields in the region between the conductors and outside the conductor, respectively. Then,
View SolutionA long straight conductor carrying a current lies along the axis of a ring. The conductor will exert a force on the ring if the ring
View SolutionA wire `ABCDEF` ( with each side of length `L`) bent as shown in figure and carrying a current `I` is placed in a uniform magnetic induction `B` parallel to the positive ` y- direction `. The force experienced by the wire is ……….. In the ………. direction .
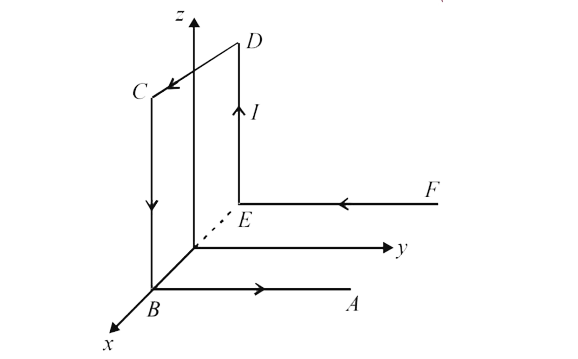
View SolutionA closed loop lying in the `xy` plane carries a current. If a uniform magnetic field `B` is present in the region, the force acting on the will be zero if `B` is in
View SolutionAn irregular closed loop carrying a current has a shape such that the entire loop cannot lie in a single plane. If it is a placed in a uniform magnetic field, the force acting on the loop
View SolutionA horizontal straight conductor of mass m and length l is placed in a uniform vertical magnetic firled of magnitude B. An amount of charge Q passes through the rod in a very short time such that the conductor begins to move only after all the charge has passed throught is. Its initial velocity will be
View Solution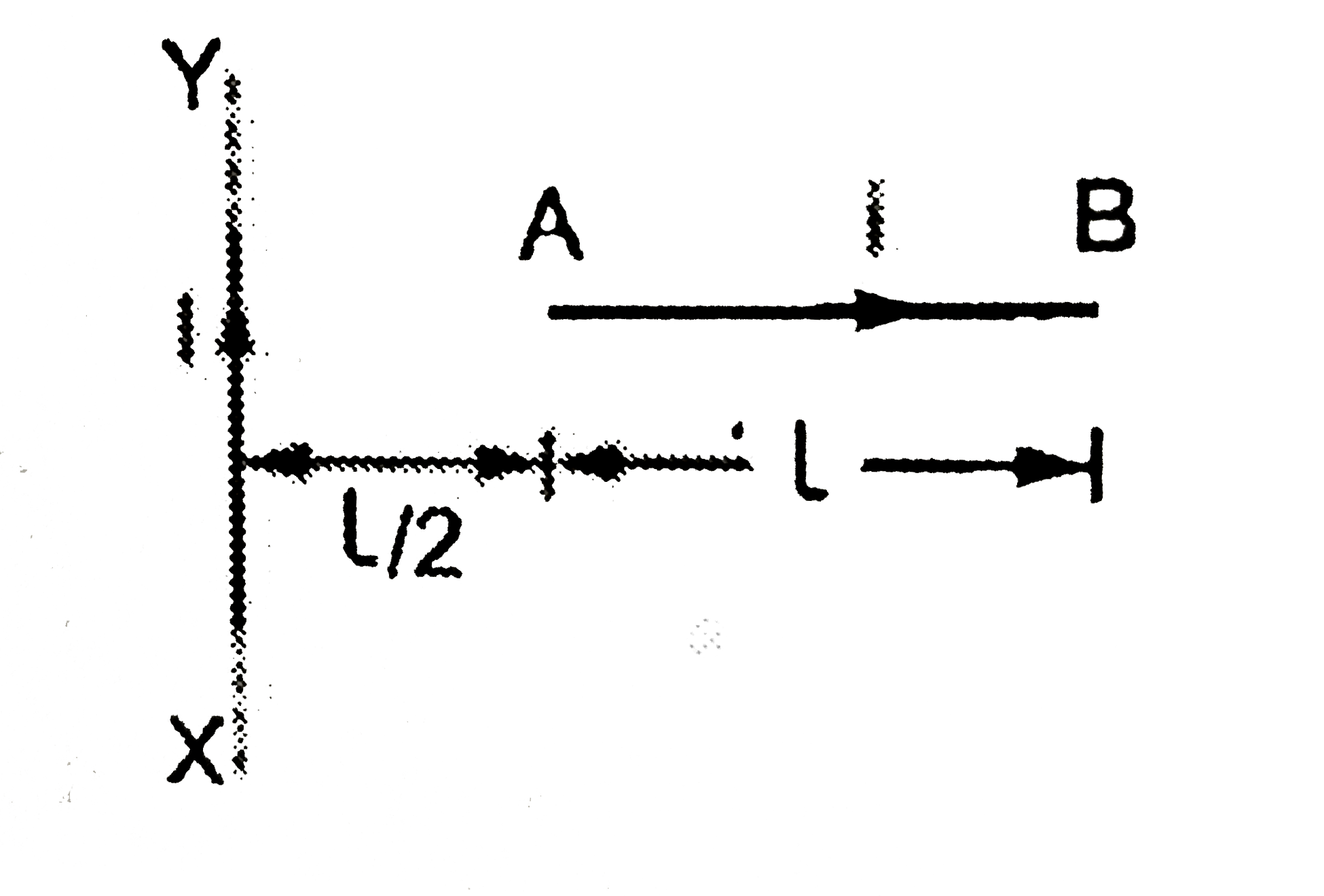
A conductor `AB` of length `l` carrying a current `i` is placed perpendicular to a long straight conductor `XY` carrying a current `I`, as shown. The force on `AB` will act
View SolutionA flat coil of `n` turns, area `A` and carrying a current `I` is placed in a uniform magnetic field of magnitude `B`. The plane of the coil makes an angle `theta` with the direction of the field. The torque acting on the coil is
View SolutionA flat coil carrying a current has a magnetic moment `mu`. It is initially in equilibrium, with its plane perpendicular to a magnetic field of magnetic `B`, If it now rotated through an angle `theta`, the work done is
View SolutionThe square loop `ABCD`, carrying a current `i`, is placed in uniform magnetic field `B`, as shown. The loop can rotate about the axis `XX'`. The plane of the loop makes and angle`theta(theta lt 90^(@))` with the direction of `B`. Through what angle will the loop rotate by itself before the torque on it becomes zero
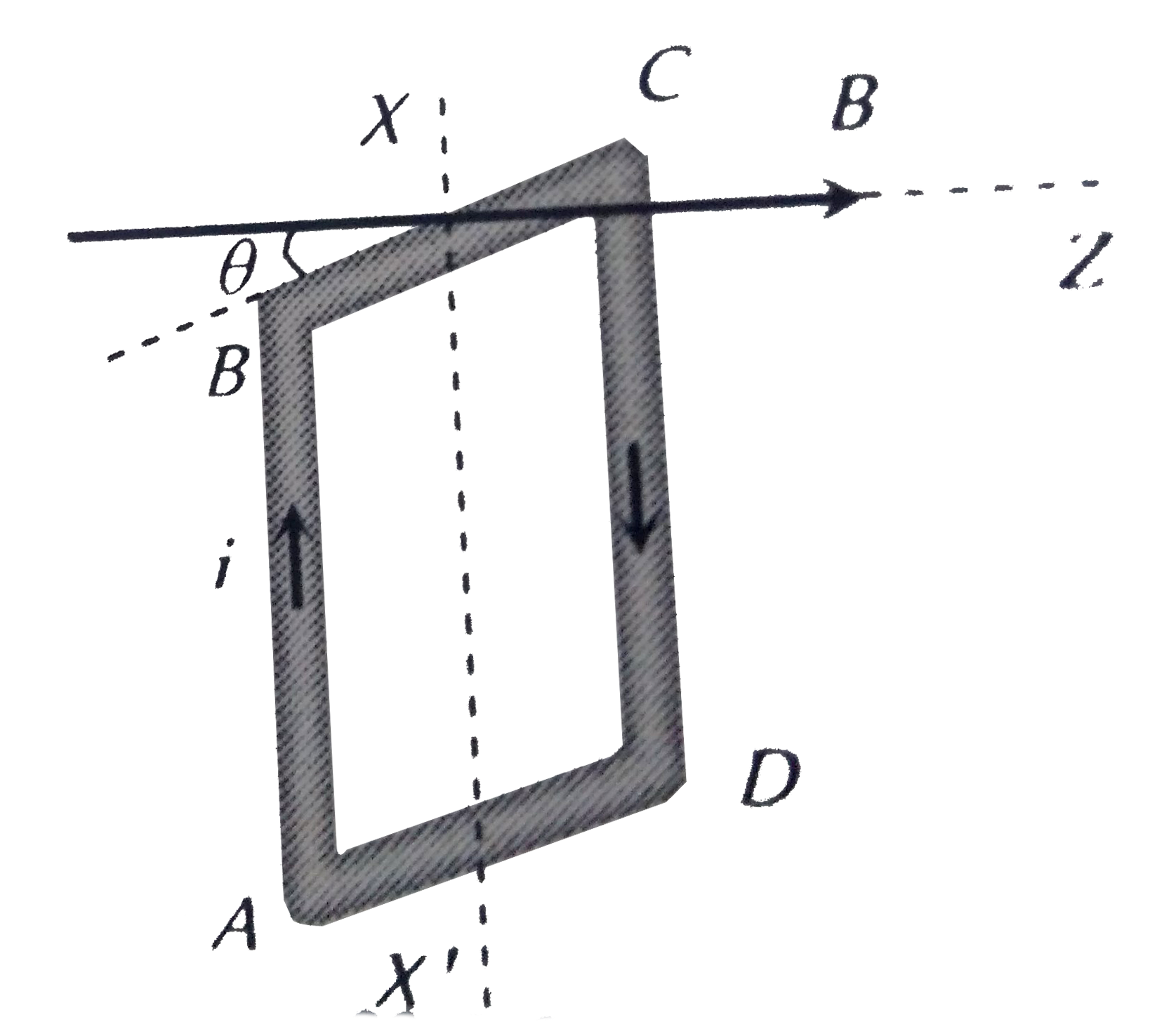
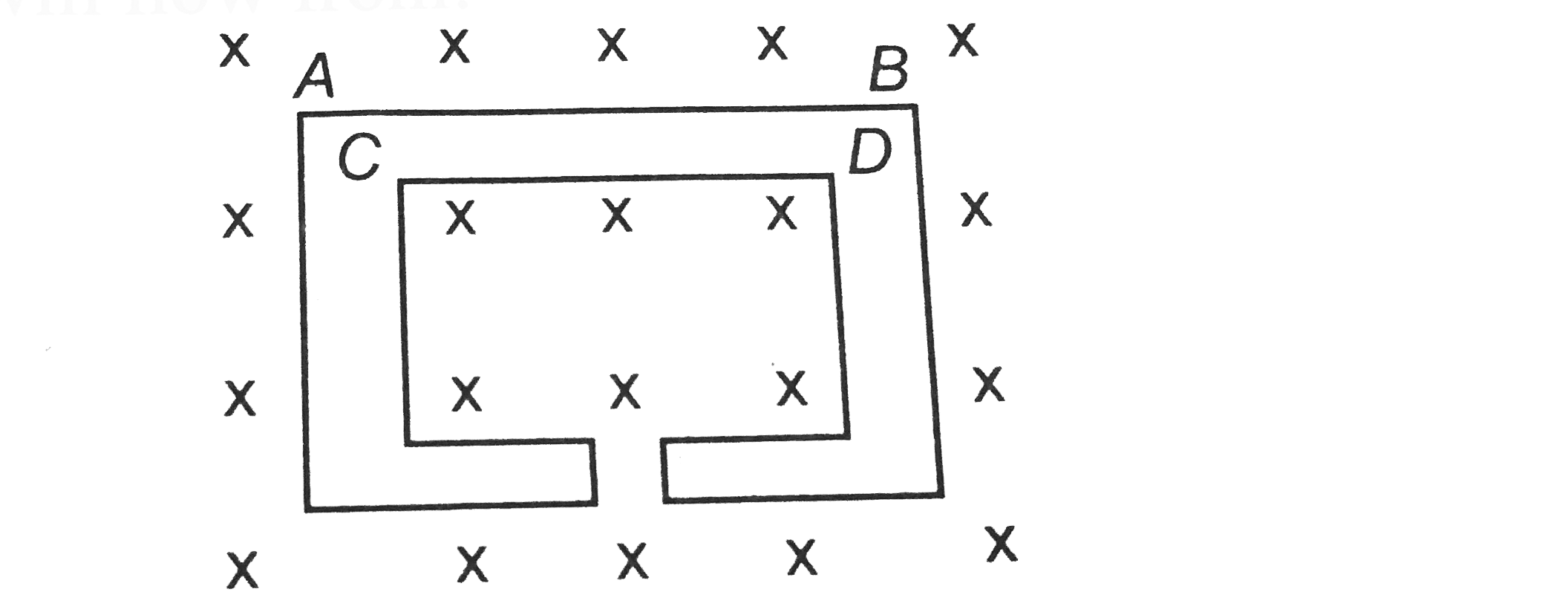
View SolutionA wire is bent to form the double loop shown in figure. There is a uniform magnetic field directed into the plane of the loop. If the magnitude of this field is decreasing current will flow from:
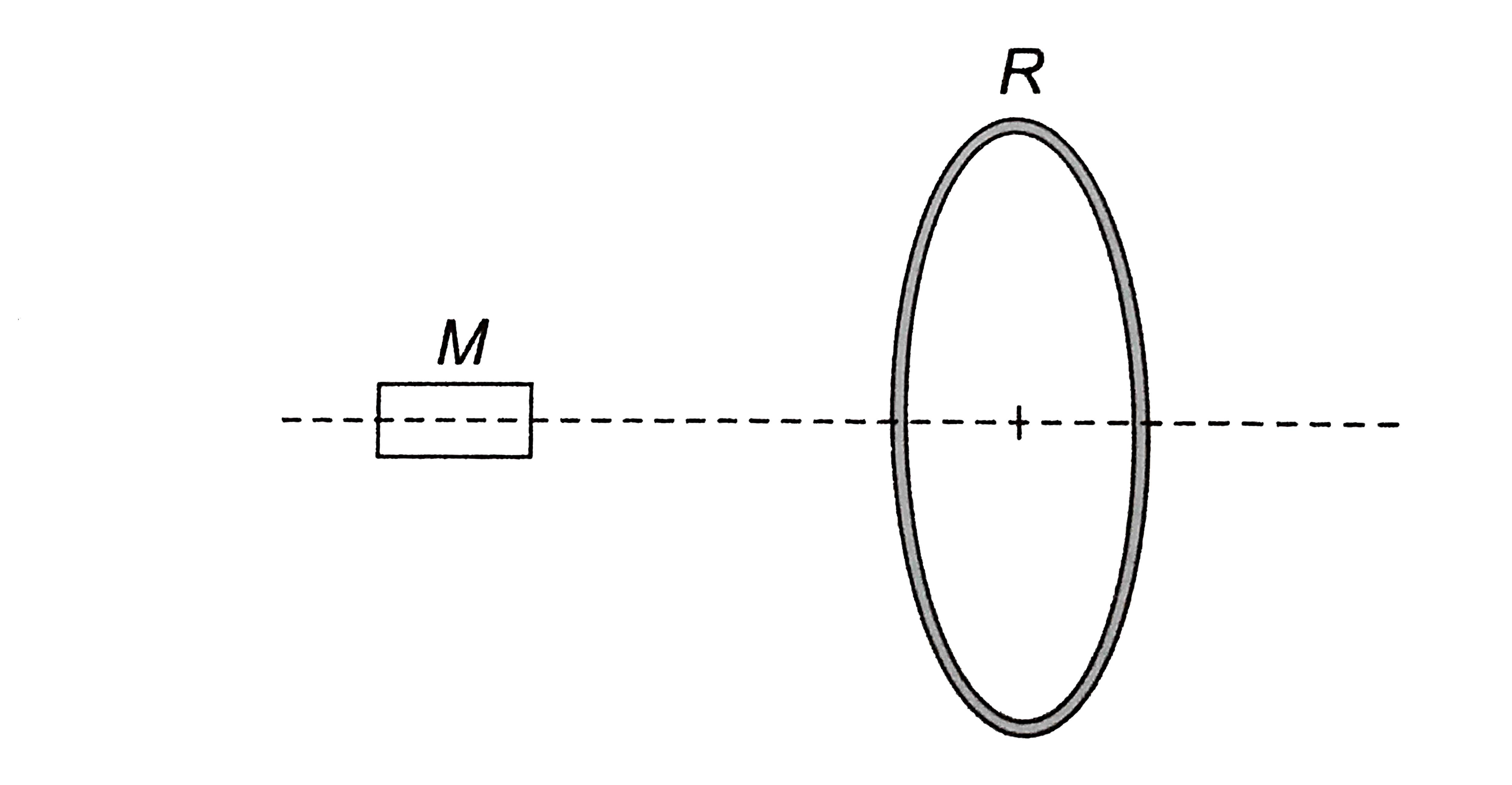
View SolutionA conduting ring `R` is placed on the axis of a bar magnet `M` . The plane of `R` is perpendicular to this axis. `M` can move along this axis
View SolutionA conductor `PQ` , with `PQ=r` , moves with a velocity `v` in a uniform magnetic field of induced `B` . The emf induced in the rod is
View SolutionA thin semicircular conducting ring of radius `R` is falling with its plane verticle in a horizontal magnetic inducting `B`. At the position `MNQ`, the speed of the ring is `V` and the potential difference developed across the ring is

View SolutionThe magnitude opf the earth's magnetic field at a place is `B_(0)` and the angle of dip is `delta` . A horizontal conductor of length `l` , lying north-south, moves eastward with a velocity `v` . The emf induced across the rod is
View SolutionThe two ends of a horizontal conducting rod of length `l` are joined to a voltmeter. The whole arrangement moves with a horizontal velocity `v` , the direction of motion being perpendicular to the rod. The vertical component of the earth's magnetic field is `B` . The voltmeter reading is
View SolutionA vertical ring of radius `r` and resistance on `R` falls vertically. It is in contact with two vertical rails which are joined at the top. The rails are without friction and resistance. There is a horizontal uniform, magnetic field of magnitude `B` perpendicular to the plane of the ring and the rails. When the speed of the ring is `v` , the current in the section `PQ` is
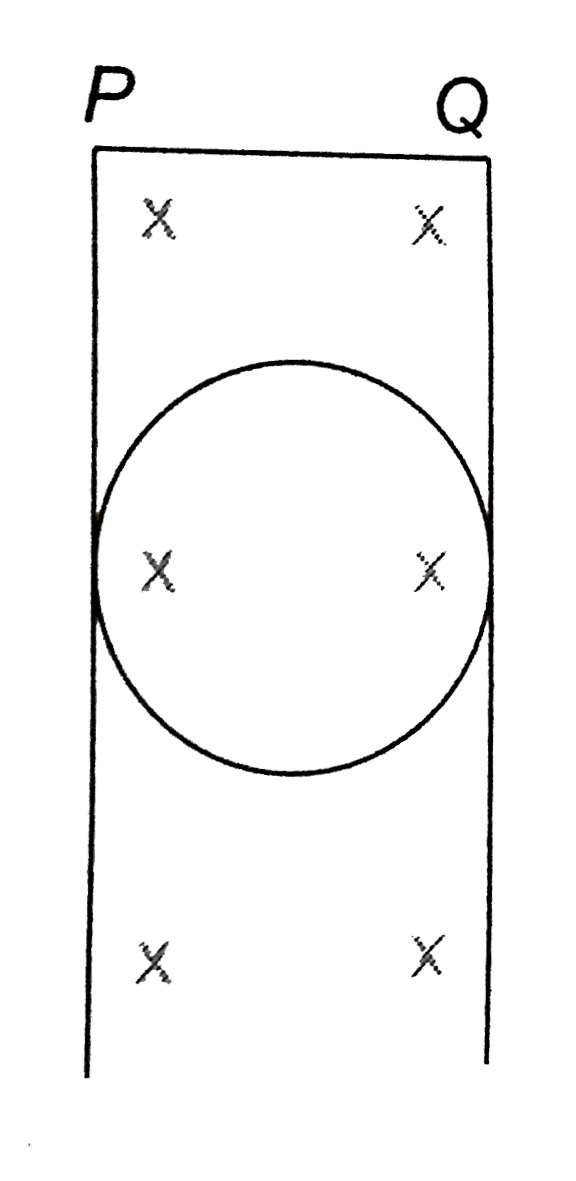
View SolutionA horizontal ring of radius `r` spins about its axis with an angular velocity `omega` in a uniform vertical magnetic field of magnitude `B`. The emf induced in the ring is
View SolutionA metal rod of resistance `R` is fixed along a diameter of a conducting ring of radius `r` . There is a magnetic field of magnitude `B` perpendicular to the plane of the loop. The ring spins with an angular velocity `omega` about its axis. The centre of the ring is joined to its rim by an external wire `W` . The ring and `W` have no resistance. The current in `W` is
View SolutionThree indential rings move with same speed on a horizontal magnetic field normal to plane of rings. The first (a) slips without rolling, the second(b) rolls without slipping and the third rolls with slipping:
View SolutionA long solenoid of `N` turns has a self-induced `L` and area of cross-section A. When a current `i` flows through the solenoid, magnetic field inside it has magnitude `B` . The current `i` is equal to
View SolutionThe network shown in the figure is part of a complete circuit. If at a certain instant, the current `I` is `5A` and is decreasing at a rate `10^(3)A//s` then `V_(B)-V_(A)` is
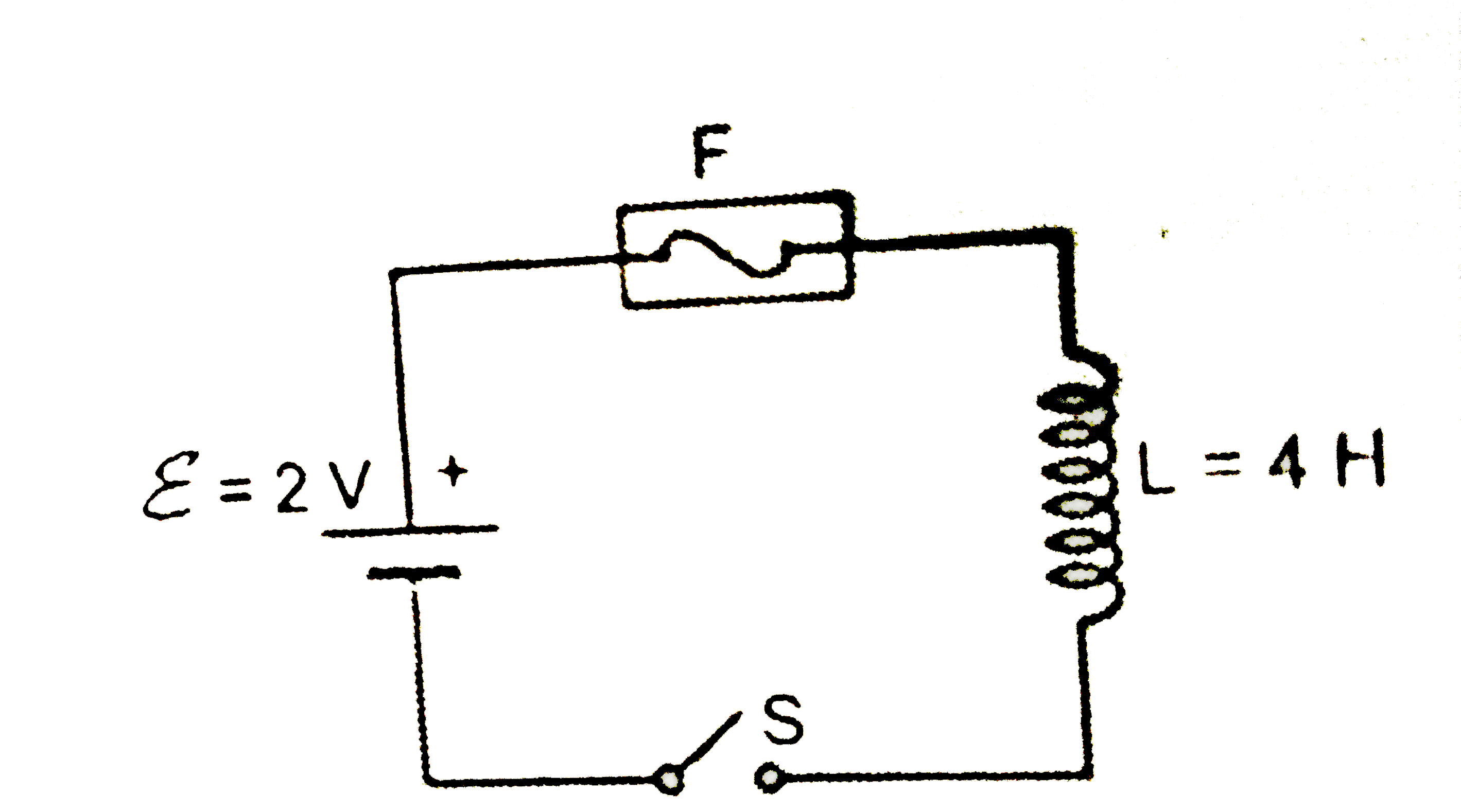
View SolutionIn the circuit shown, the cell is deal. The coil has an inductance of `4H` and zero resistance. `F` is a fuse of zero resistance and will blow when the current through it reaches `5 A`. The switch is closed at `t=0`. The fuse will blow:
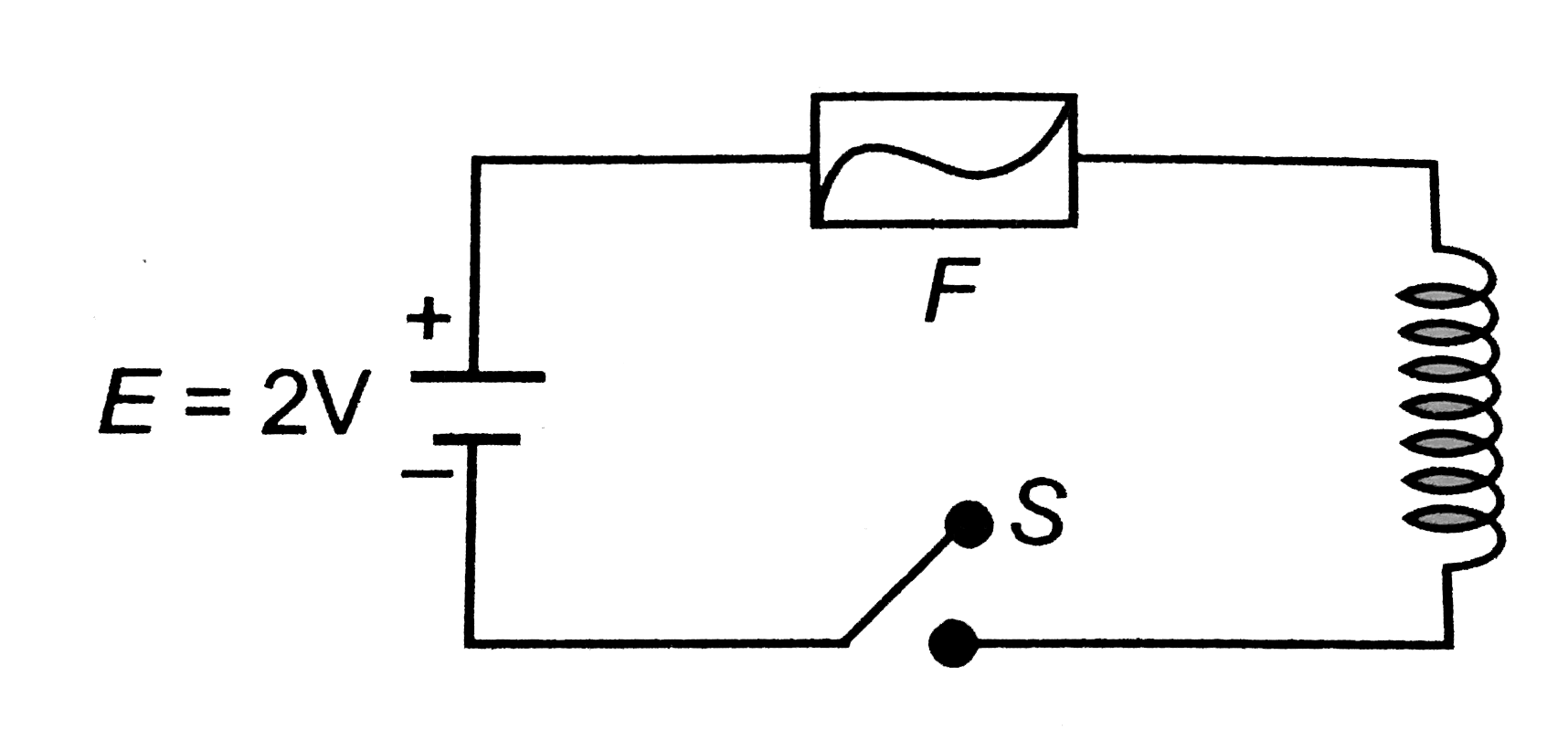
View SolutionIn the current shown Fig., `X` is joined to `Y` for a long time and then `X` is joined to `Z`. The total heat produced in `R_(2)` is

View SolutionTwo coils `X` and `Y` are linked such that emf `E` is induced in `Y` when the current in `X` is changing at the rate `I' (= dI//dt)`. If a current `I_(0)` is now made to flow through `Y`, the flux linked with `X` will be
View SolutionTwo coils of inductances `L_(1)`, and `L_(2)` are linked such that their mutual inductance is `M`
View SolutionA small coil of radius `r` is placed at the centre of a large coil of radius `R`, where `R gtgt r`. The two coils are coplanar. The mutual inductance between the coils is proportional to
View SolutionA uniformly wound long solenoid of inductance `L` and resistance `R` is cut into two parts in the ratio `eta:1`, which are then connected in parallel. The combination is then connected to a cell of emf `E`. The time constant of the circuit is
View SolutionIn the circuit shows in Fig, the coil has inductance and resistance. When `X` is joined to `Y`, the time constant is `tau` during the growth of current. When the steady state is reached, heat is produced in the coil at a rate `P`. `X` is now joined to `Z`. After joining `X` and `Z`:

View SolutionAt `t=0`, an inductor of zero resistance is joined to a cell of emf `varepsilon` through a resistance. The current increases with a time constant `tau`. The emf across the coil after time `t` is
View SolutionA coil carrying a steady current is short-circuited. The current in it decreases `alpha` times in time `t_(0)`. The time constant of the circuit is
View Solution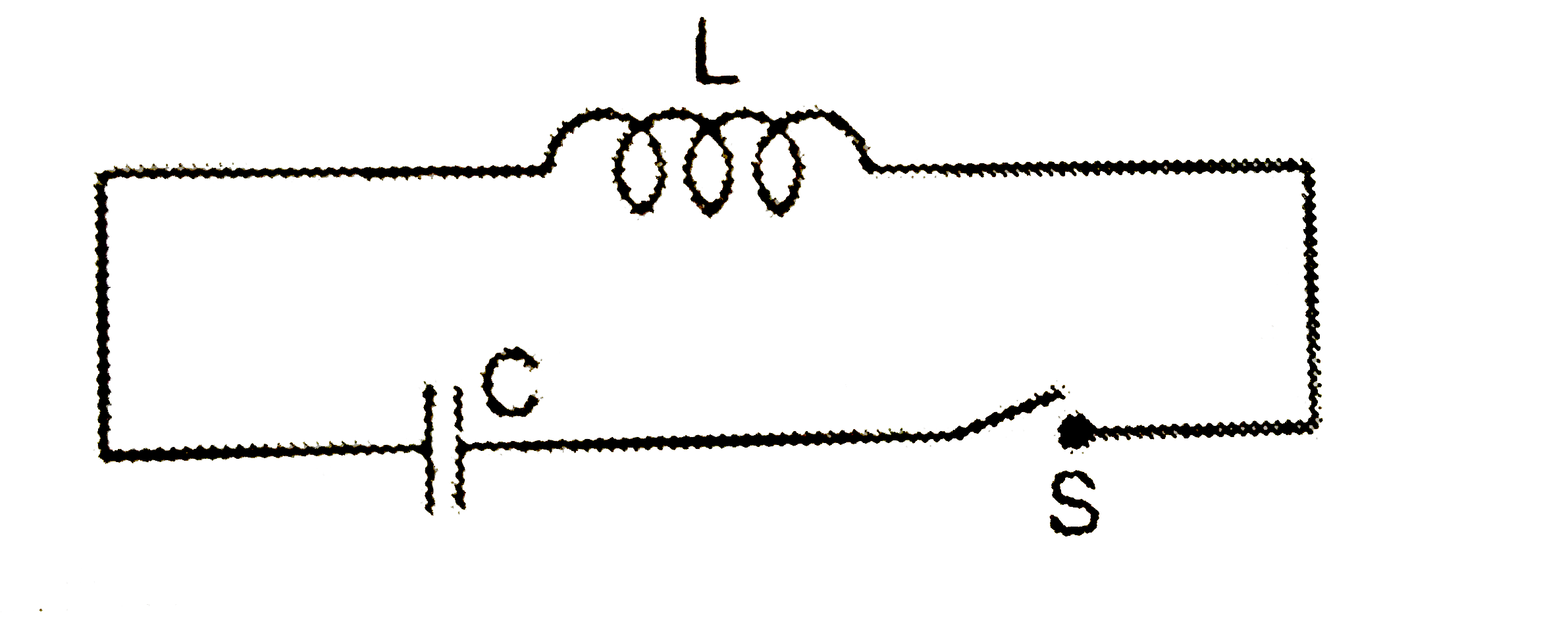
The capacitor of capacity `C` is given charge `Q` and then connected to the coil of inductance `L` by closing the switch `S`. The maximum current flowing in the circuit at any later time will be
View Solution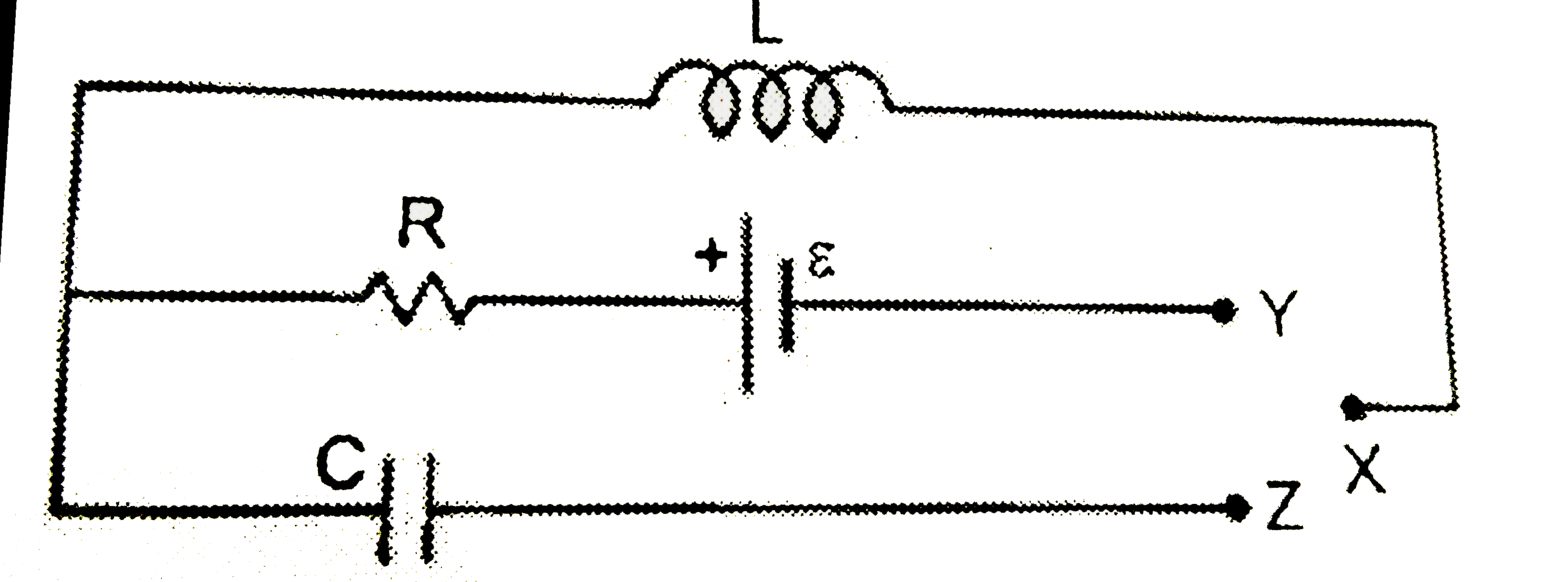
In the circuit shown, the symbols have their usual meanings. The cell has emf `varepsilon.X` is initially joined to `Y` for a long time. Then `X` is joined to `Z`. The maximum charge on `C` at any later time will be
View SolutionA coil of self-inductance `L` is placed in an external magnetic field (no current flows in the coil). The total magnetic flux linked with the coil is `varphi`. The magnetic field energy stored in the coil is
View SolutionWhen a charged capacitor is made to discharge through an inductance, the charge `Q` on the capacitor and the current `i` in the inducrtor vary sinusoidally. The phase different between `Q` and `i` is
View SolutionA charged capacitor discharges through a resistance `R` with time constant `tau`. The two are now placed in series across an `AC` source of angular frequency `omega =1/(tau)` . The impedance of the circuit will be
View SolutionAn electric lamp designed for operation on `10V AC` is connected to a `220V AC` supply, through a choke coil of inductance `2H` for proper operation. The angular frequency of the `AC` is `100sqrt(10)rad//s`. If a capacitor is to be used in place of the choke coil its cpacitance must be
View SolutionA resistance `R` draws power `P` when connected to an `AC` source. If an inductance is now placed in series with the resistance, such that the impedence of the circuit becomes `Z`, the power drawn will be
View SolutionAn inductor and a capacitor are joined in series to an `AC` source. The frequency of the `AC` is gradually increased. The phase difference `varphi` between the emf and the current is plotted against nthe angular frequency `omega`. Which of the following best represents the resulting curve?
View SolutionA resistance `R` and a capacitor `C` are joined to a source of `AC` of consant emf and variable frequency. The potential difference across `C` is `V`. If the frequency of `AC` is gradually increased `V` will

View SolutionAn inductance `L`, a cpacitance `C` and a resistance `R` may be connected to an `AC` souorce of angular frequency `omega` in three different combinations of `RC, RL` and `LC` in series. Assume that `omegaL=1/(omegaC)`. The power drawn by the three combinatios are `P_(1), P_(2), P_(3)` respectively. THen
View SolutionAn electrical heater and a capacitor are joined in series across a `220V, 50Hz, AC` supply. The potential difference across the heater is `90V`. The potential difference across the capacitor will be about:
View SolutionIn an `AC` circuit, the reactannce is equal to the resistance. The power factor of the circuit will be
View Solution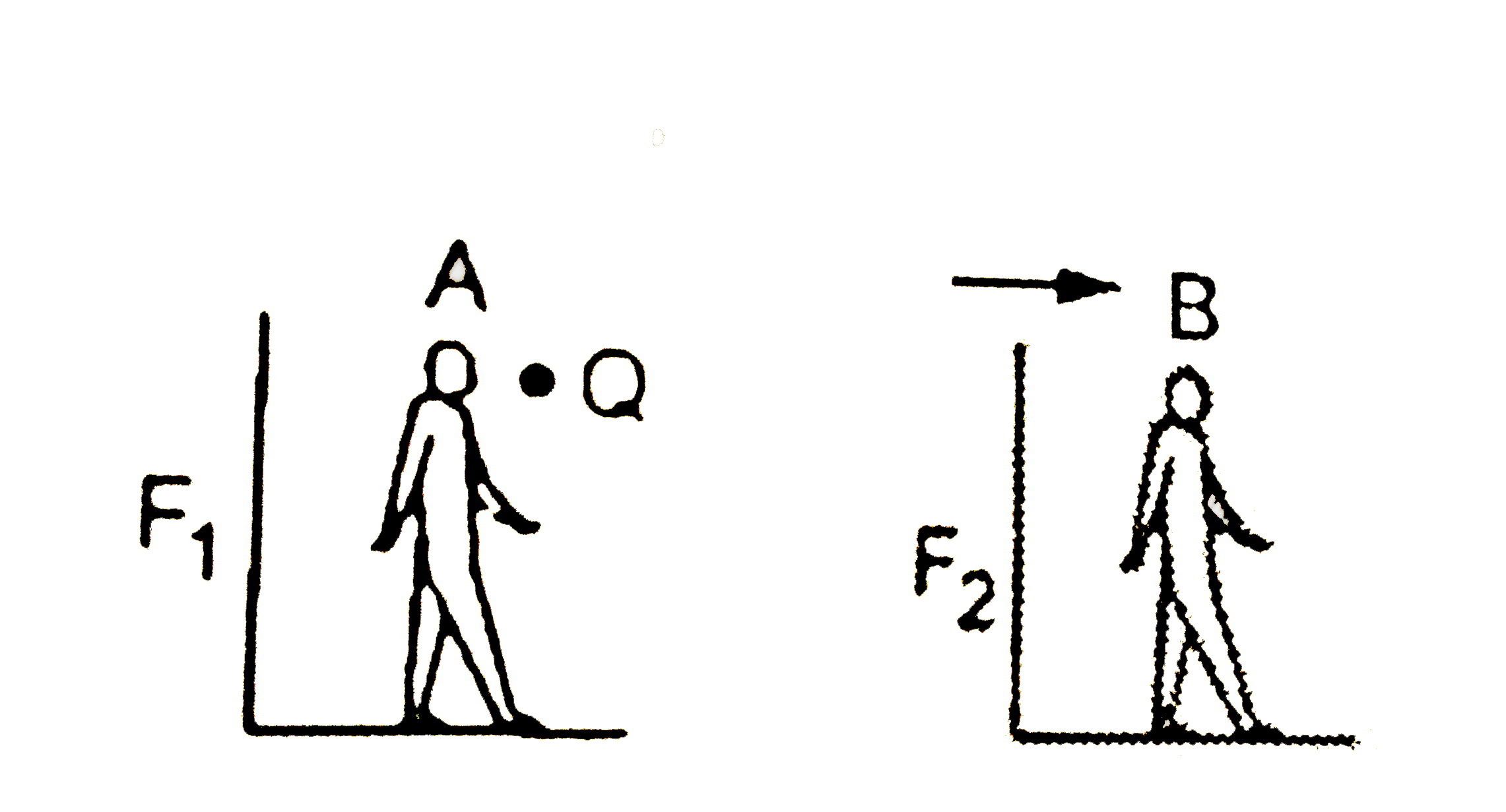
An observer `A` and `a` charge `Q` are fixed in a stationary frame `F_(1)`. An observer `B` is fixed in a frame `F_(2)` which is moving with respect to `F_(1)`
View SolutionA long straight wire carries the current along +ve x-direction. Consider four points in space `A(0,1,0), B(0,1,1), C(1,0,1), and D(1,1,1)`. Which of the pairs will have the same magnitude of magnetic field?
View SolutionTwo long parallel wires, AB and CD, carry equal currents in opposite directions. They lie in the x-y plane, parallel to the x-axis, and pass through the points (0,-a,0) and (0,a,0) respectively, Figure. The resultant magnetic field is:
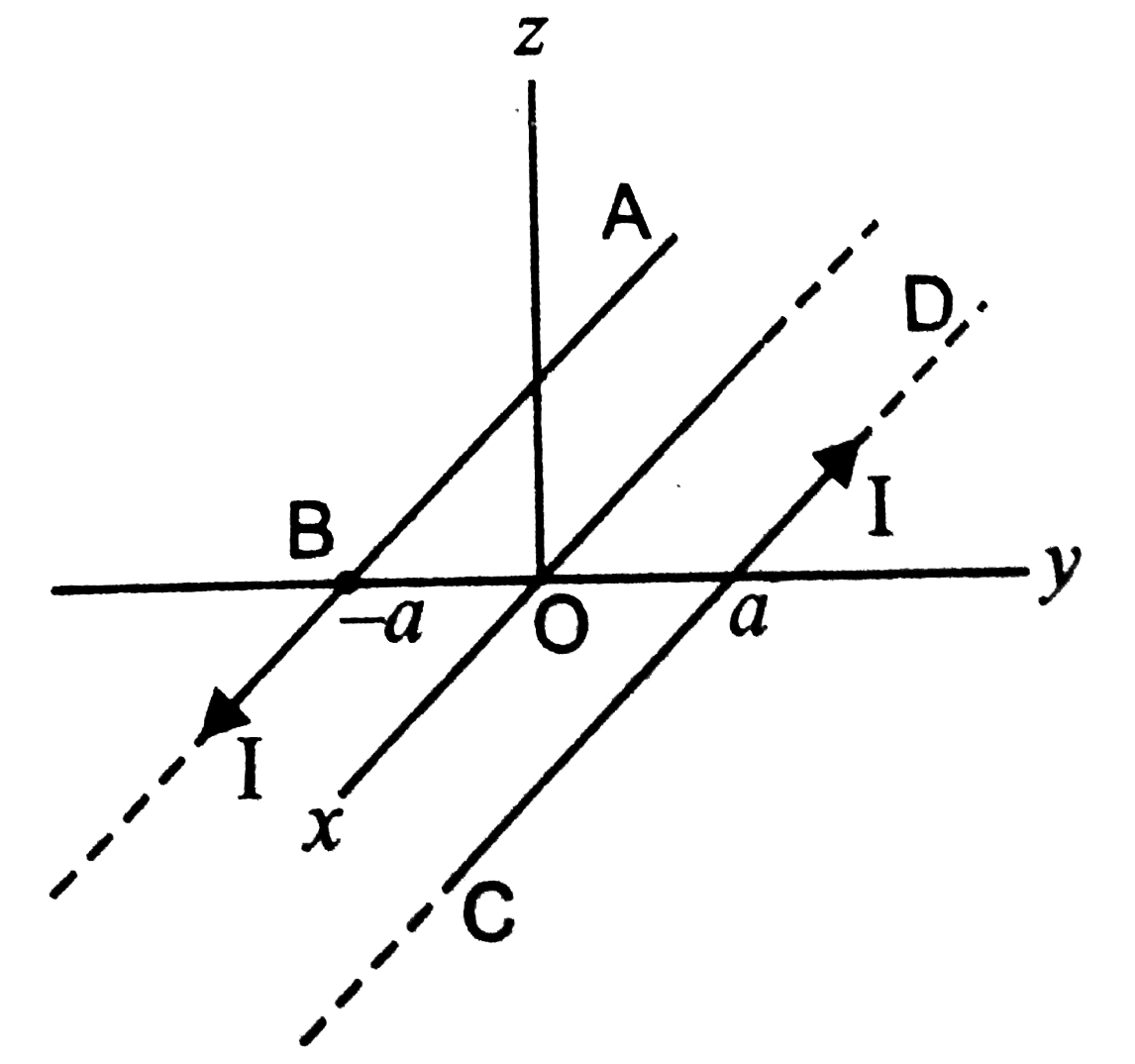
View SolutionA straight conductor carries a current. Assume that all free electrons in the conductor move with the same drift velocity `v`. `A` and `B` are two observers on a striaght line `XY` parallel to the conductor. `A` is stationary `B` moves along `XY` with a velocity `v` in the direction of the free electrons.
View SolutionA straight conductor carriers a current alon the `z`-axis Consider the points `A(a,0,0),B(0,-a,0),C(-a,0,0)` and `D(0,a,0)`
(i) All four points have magnetic fields of the same magnitude.
(ii) All four points have magnetic fields of the different direction.
(iii) The magnetic fields at `A` and `C` are in opposite directions
(iv) The magnetic fields at `A` and `B` are mutually perpendicular
View Solution`L` is a circular ring made of a uniform wire, currents enters and leaves the ring through straight conductors which, if produces, would have passed through the centre `C` of ring. The magnetic field at `C`
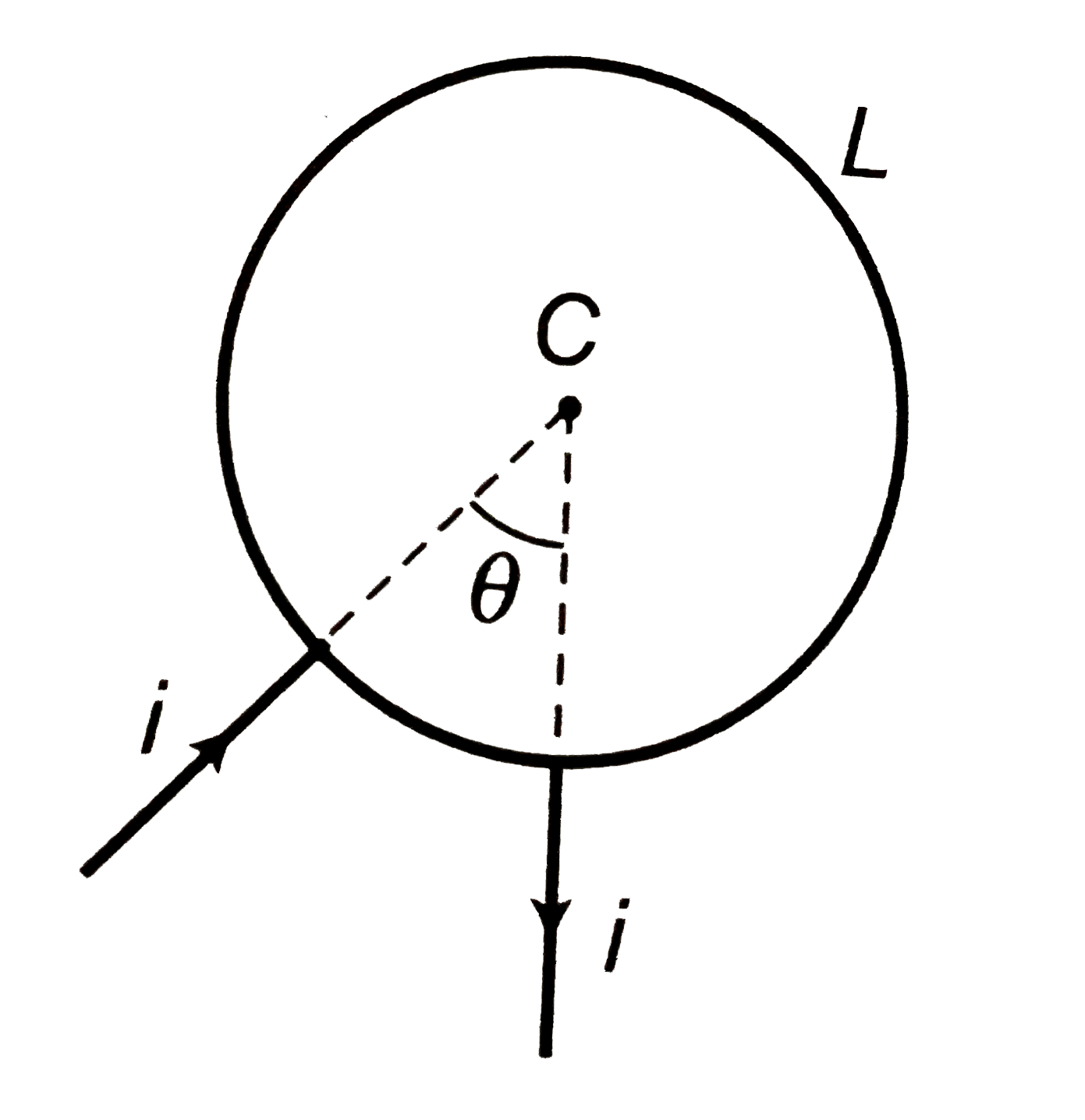
(i) due to the straight conductors is zero
(ii) due to the loop is zero
(iii) due to the loop is proportional to `theta`
(iv) due to loop is proportional to `(pi-theta)`
View SolutionL is a circular loop (in y-z plane) carrying an anticlockwise current. P is a point on its axis OX dl is an element of length on the loop at a point A on it. The magnetic field at P

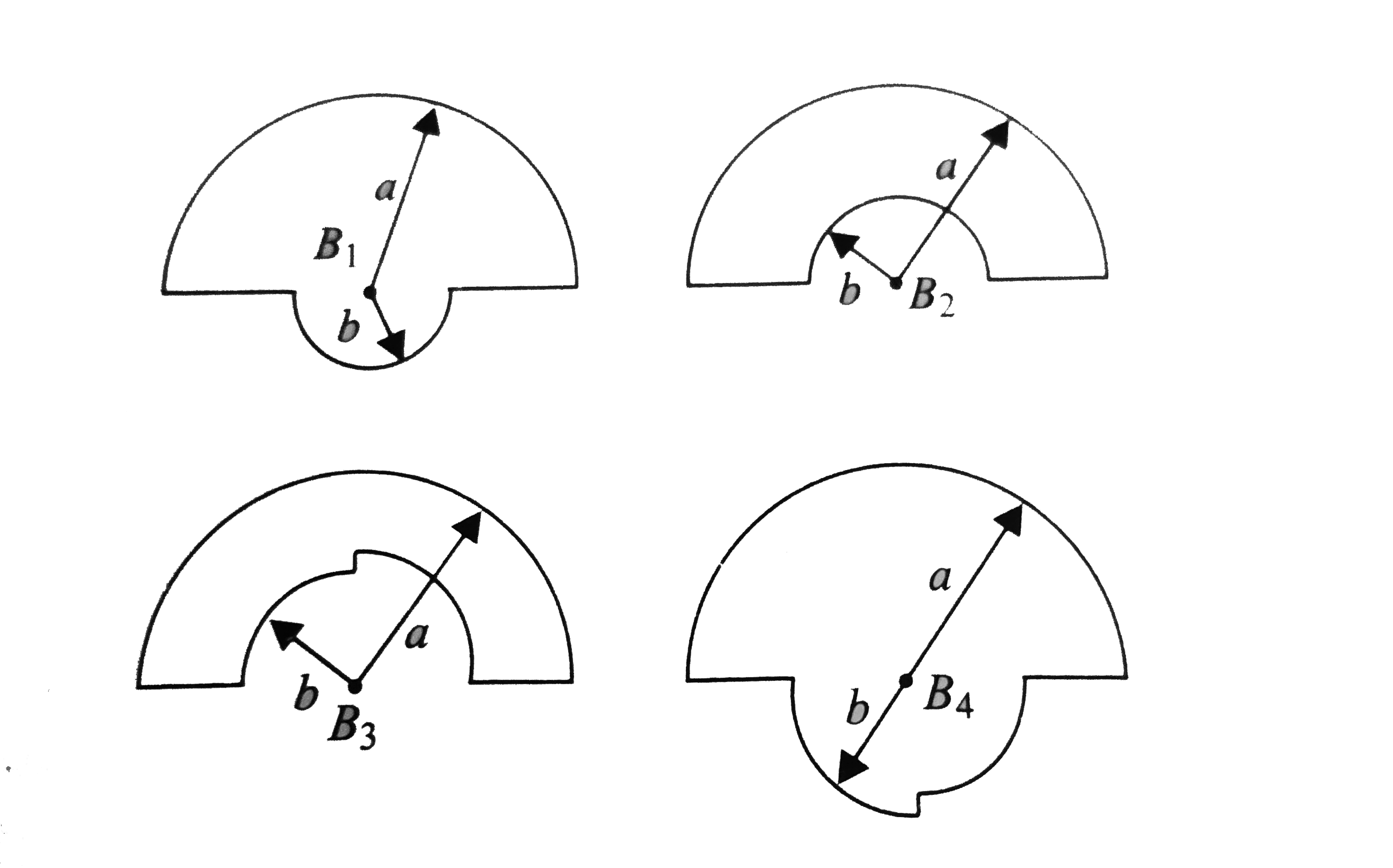
View SolutionIn the loops shown in fig. all curved section are either semicircles or quarter circles. All the loops carry same current. The magnetic fields at the centre have magnitudes `B_1, B_2,B_3 and B_4`. Then,
View SolutionA long straight conductor carrying a current `i` is bent to form an almost complete circular loop of radius `r` on it. The magnetic field at the centre of the loop
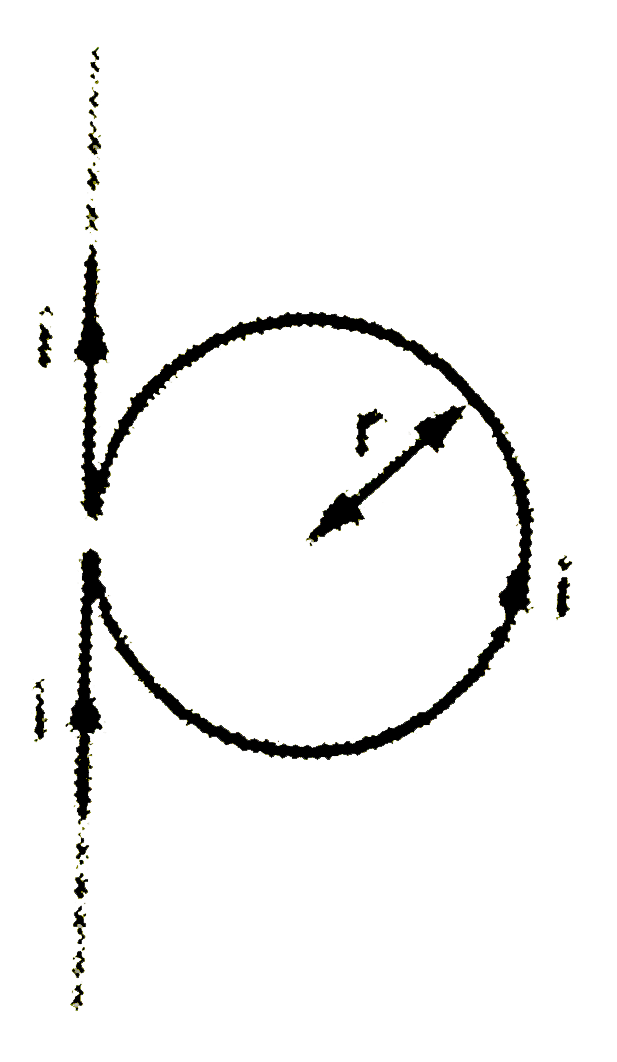
View SolutionA flat circular coil, carrying a current, has a magnetic moment `mu`
View SolutionCurrent flows through a straight cylindrical conductor of radius r. The current is distributed uniformly over its cross-section. The magnetic field at a distanace x from the axis of the conductor has magnitude B:
View SolutionA long, straight, hollow conductor (tube) carrying a current has two sections A and C of unequal cross sections joined by a conical section B. 1,2 and 3 are points on a line parallel to the axis of the conductor. The magnetic fields at 1,2 and 3 have magnitudes `B_1, B_2 and B_3`. Then,
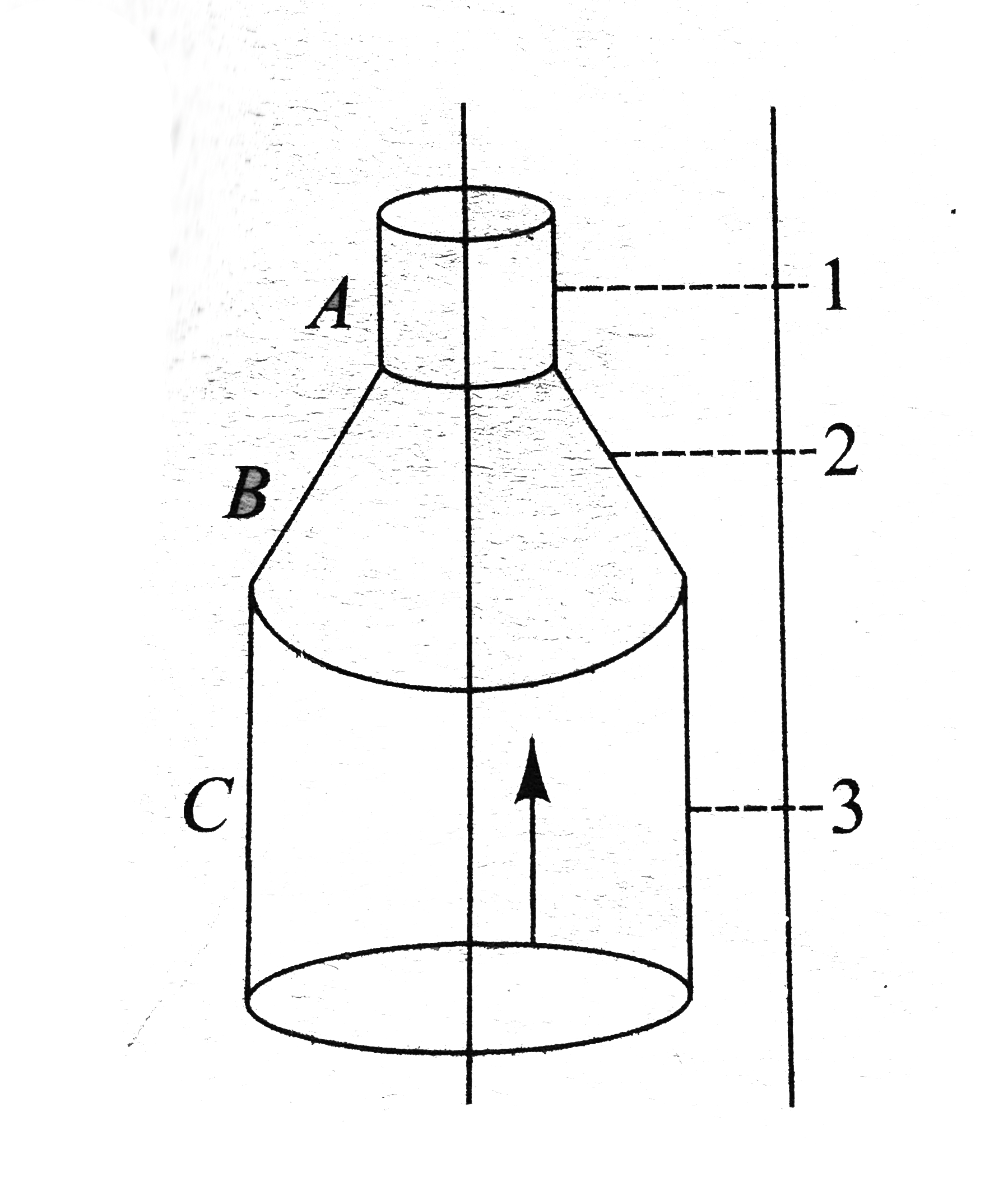
View SolutionA conductor `AB` carries a current `i` in a magnetic field `B`. If `AB = r` and the force on the conductor is `F`,
(i) `vec(F)` does not depend on shape of `AB`
(ii)`vec(F) = i(vec(r ) xx vec(B))`
(iii) `vec(F) = i(vec(B) xx vec( r))`
(iv) `|vec(F)| = i r B`
View SolutionA semicircular wire of radius `r`, carrying a current `I`, is placed in a magnetic field of magnitude `B`. The force acting on it
View SolutionA conductor ABCDE, shaped as shown, carries current I. It is placed in the x-y plane with the end A and E on the x-axis. A uniform magnetic field of magnitude B exists in the region. The force acting on it will be

View Solution`AB` and `CD` are smooth, parallel, horizontal rails on which a conductor `T` can slide. `A` cell, `E`, drives current `I` through the rails and `T`
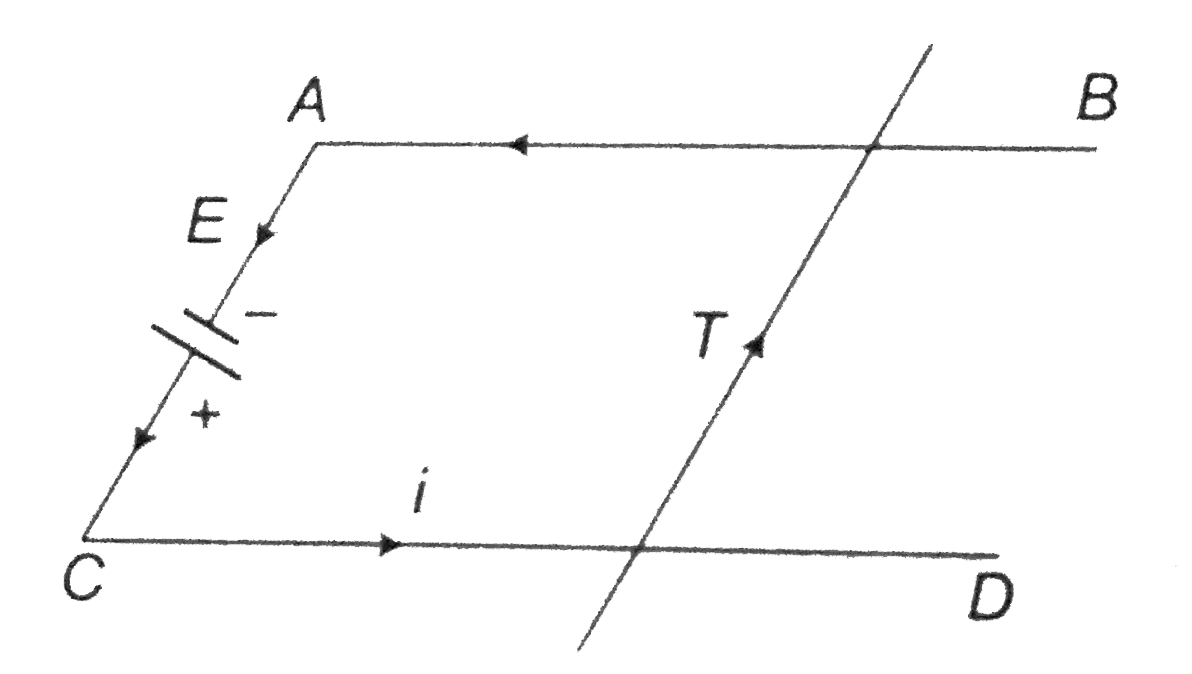
(i) The current in the rails will set up a magnetic field over `T`
(ii) `T` will experienced a force to the right
(iii) `T` will experienced a force to the left
(iv) `T` will not experienced any force
View SolutionTwo long, thin parallel conductors, separated by a distance `d`, carrying currents `i_(1)` and `i_(2)`. The force acting on unit length of any one conductor is `F`
View SolutionA current-carrying ring is placed in a magnetic field. The direction of the field is perpendicular to the plane of the ring
(i) There is no net force on the ring
(ii) The ring will tend to expand
(iii) The ring will tend to contract
(iv) Either (ii) or (iii) depending on the directions of the current in the ring and the magnetic field
View Solution`AB` and `CD` are smooth, parallel rails, separated by a distance `L` and inclined to the horizontal at an angle `theta`. A uniform magnetic field of magnitude `B`, directed vertically upwards, exists in the region. `EF` is a conductor of mass `m`, carrying a current `i`. For `EF` to be in equilibrium
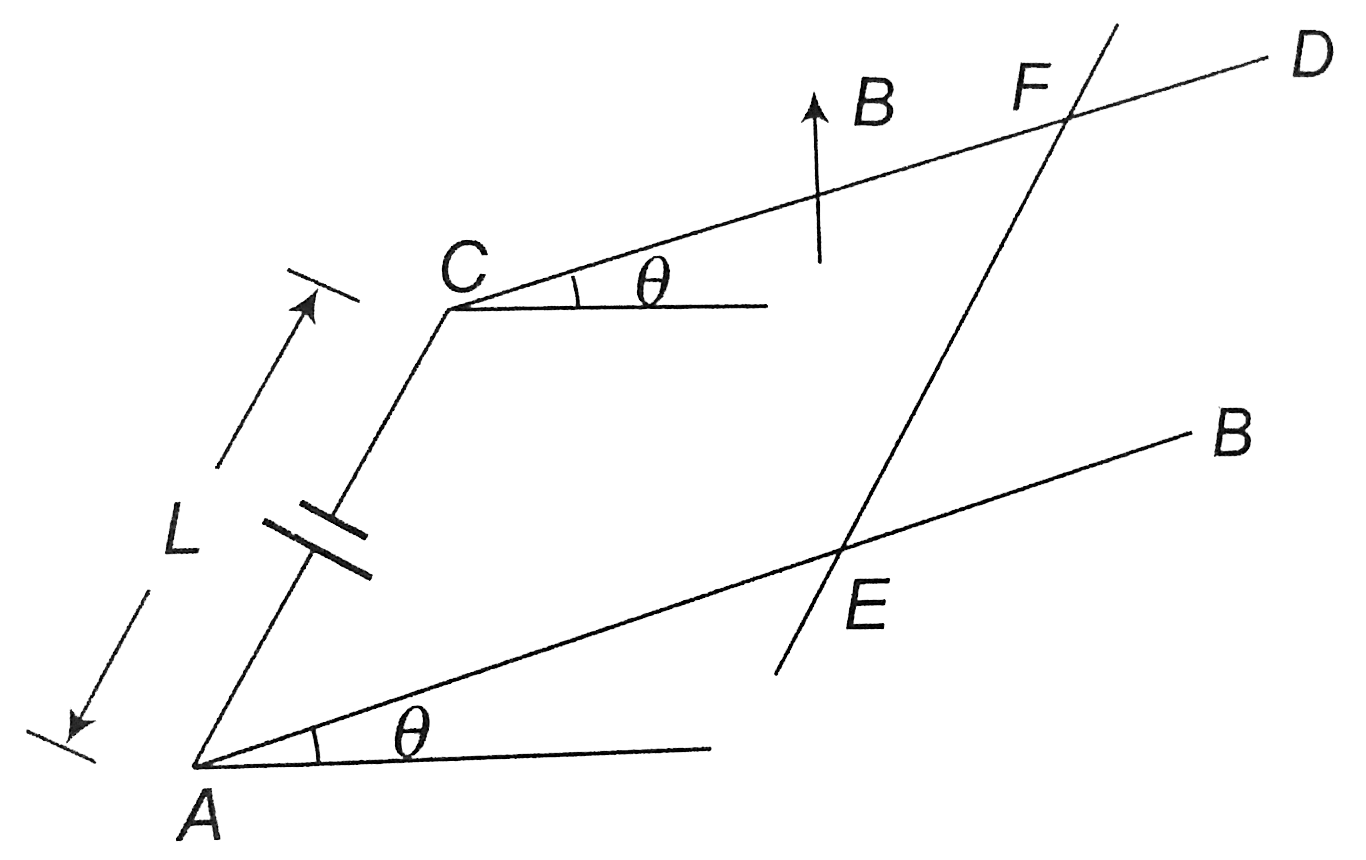
(i) `i` must flow from `E` to `F`
(ii) `B i L = m g tan theta`
(iii) `B i L = m g sin theta`
(iv) `B i L = m g`
View SolutionA flat coil carrying a current has a magnetic moment `vecmu`. It is placed in a magnetic field `vecB`. The torque on the coil is `vec(tau)`
View SolutionA flat coil carrying a current has a magnetic moment `mu`. It is placed in a magnetic field `B` such that `mu` is anti-parallel to `B`. The coil is
View SolutionThe magnetic flux `(phi)` linked with the coil depends on time `t` as `phi=at^(n)`, where `a` and `n` are constants. The emf induced in the coil is `e`
View SolutionAn aluminium ring `B` faces an electromagnet `A`. The current `I` through `A` can be altered
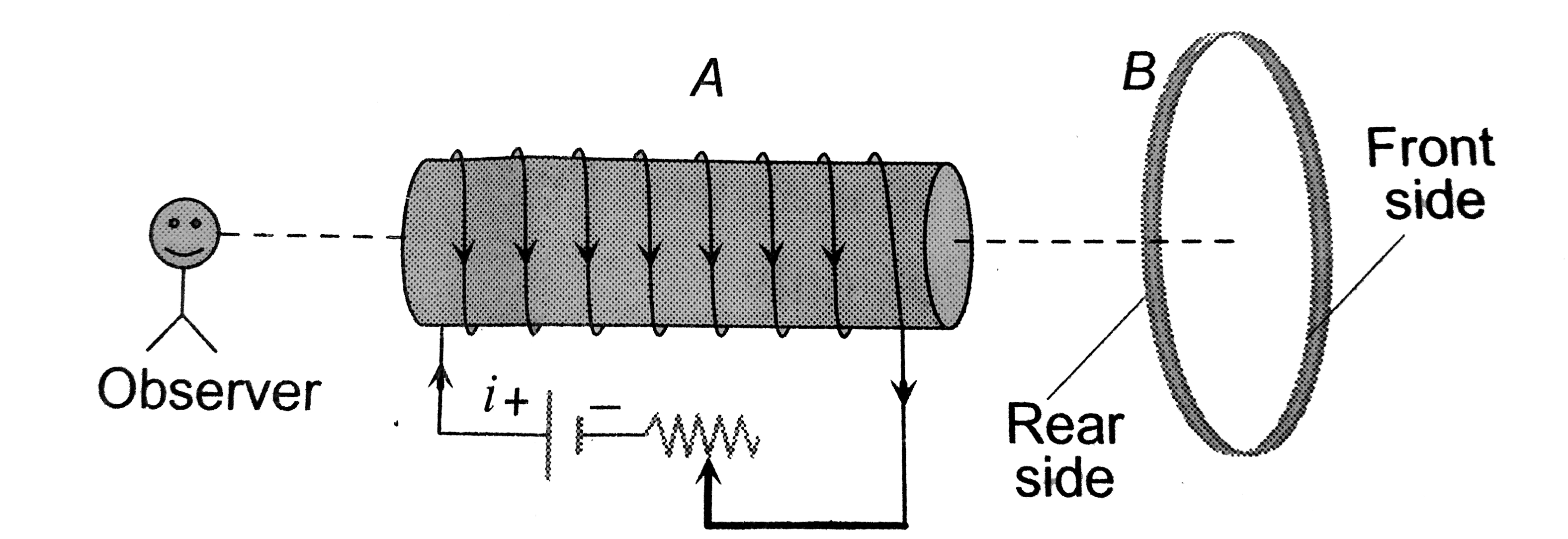
View SolutionA small magnet `M` is allowed to fall through a fixed horizontal conducting ring `R`. Let `g` be the acceleration due to gravity. The acceleration of `M` will be
View SolutionThe magnetic flux linked with a coil is `phi` and the emf induced in it is `e`.
View SolutionThe counductor `AD` moves to the right in a uniform magnetic field directed into the plane of the paper.
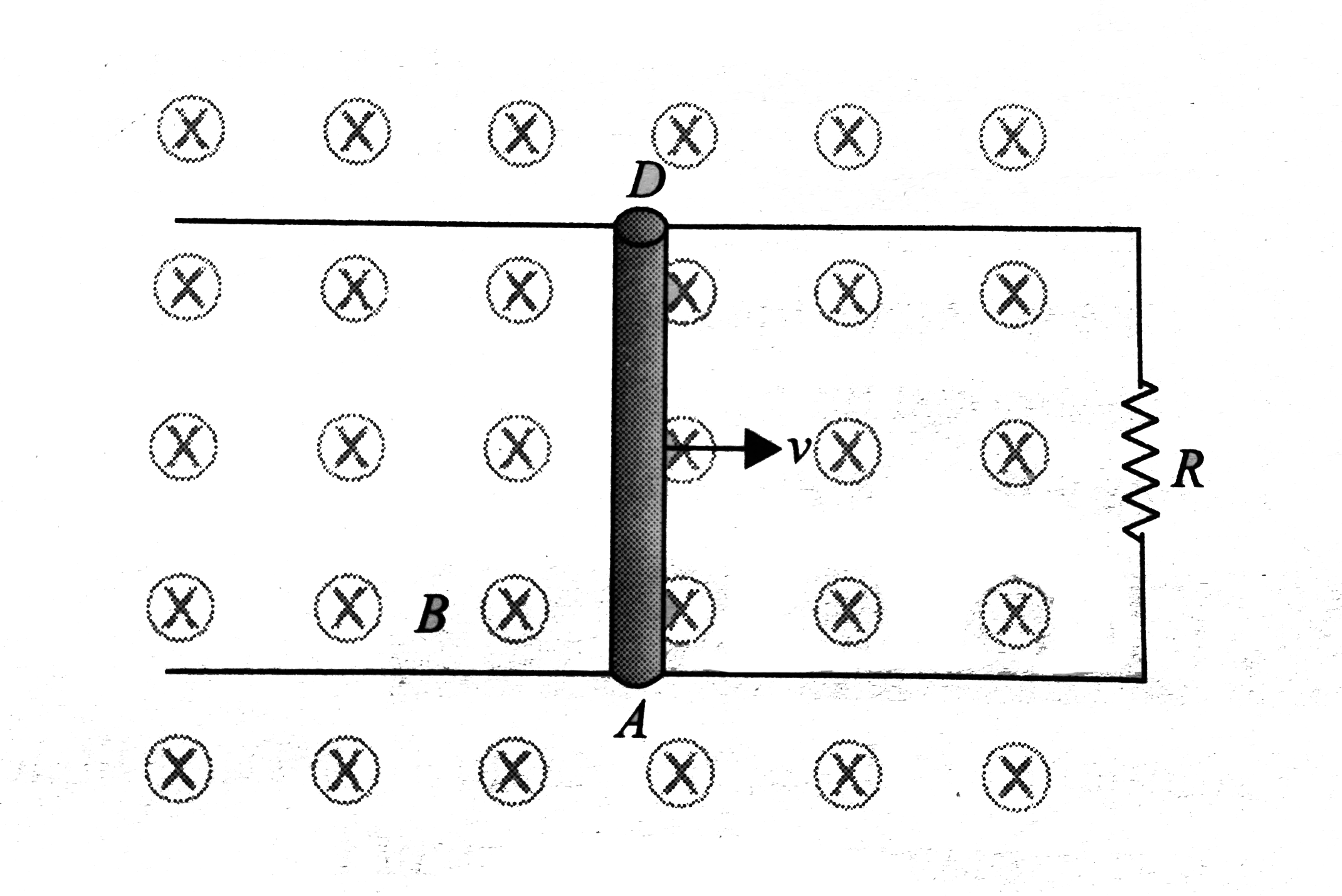
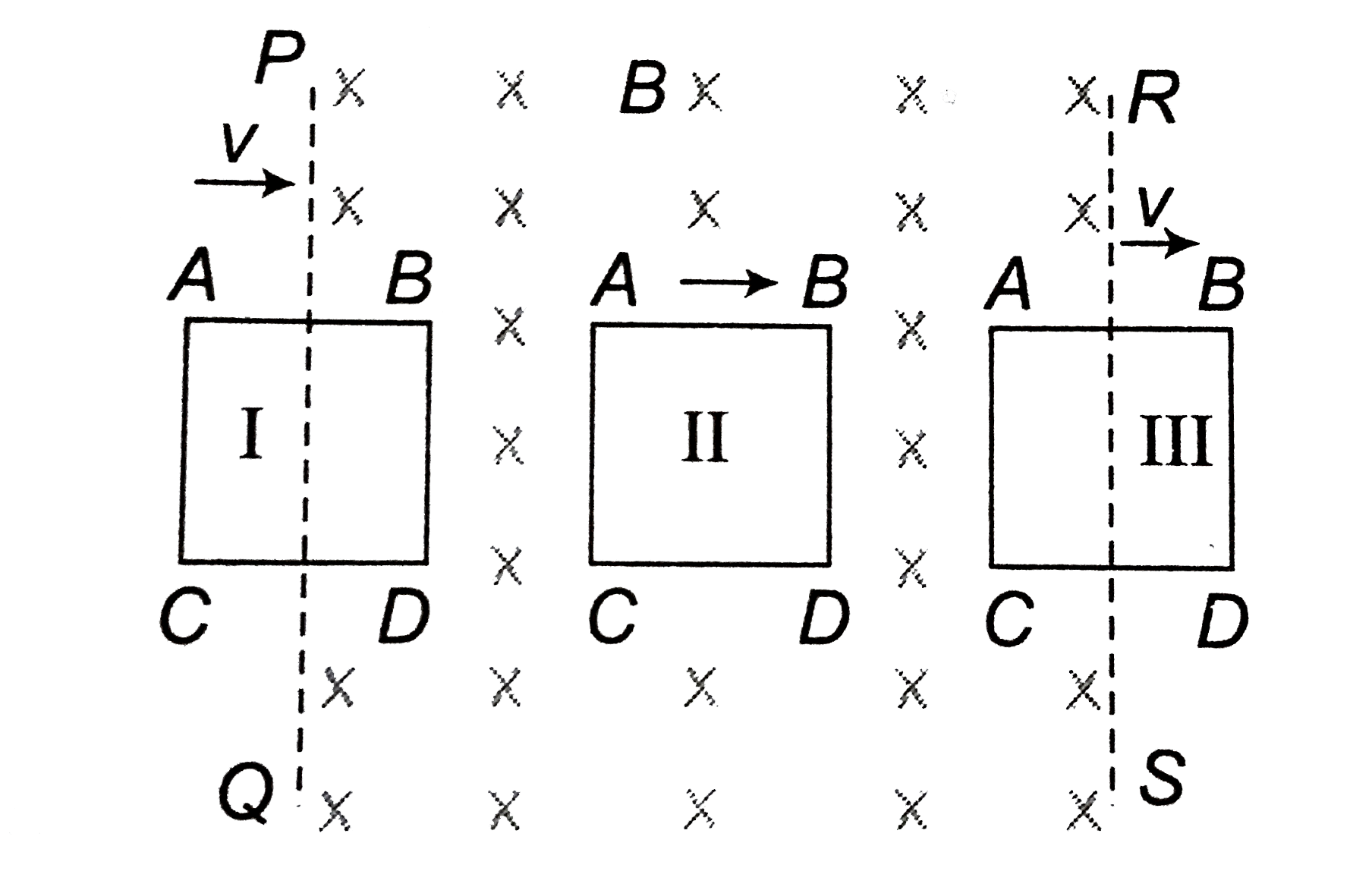
View SolutionA square loop `ABCD` of edge a moves to the right with a velocity `v` parallel to `AB` . There is a uniform magnetic field of magnitude `B` , direction into the paper, in the region between `PQ` and `RS` only. I, II and III are three ppositions of the loop.
(i) The emf induced in the loop has magnitude `B` a `v` in all three position
(iii) Induced emf is anticlockwise in position II
(iv) The induced emf is clockwise in position III
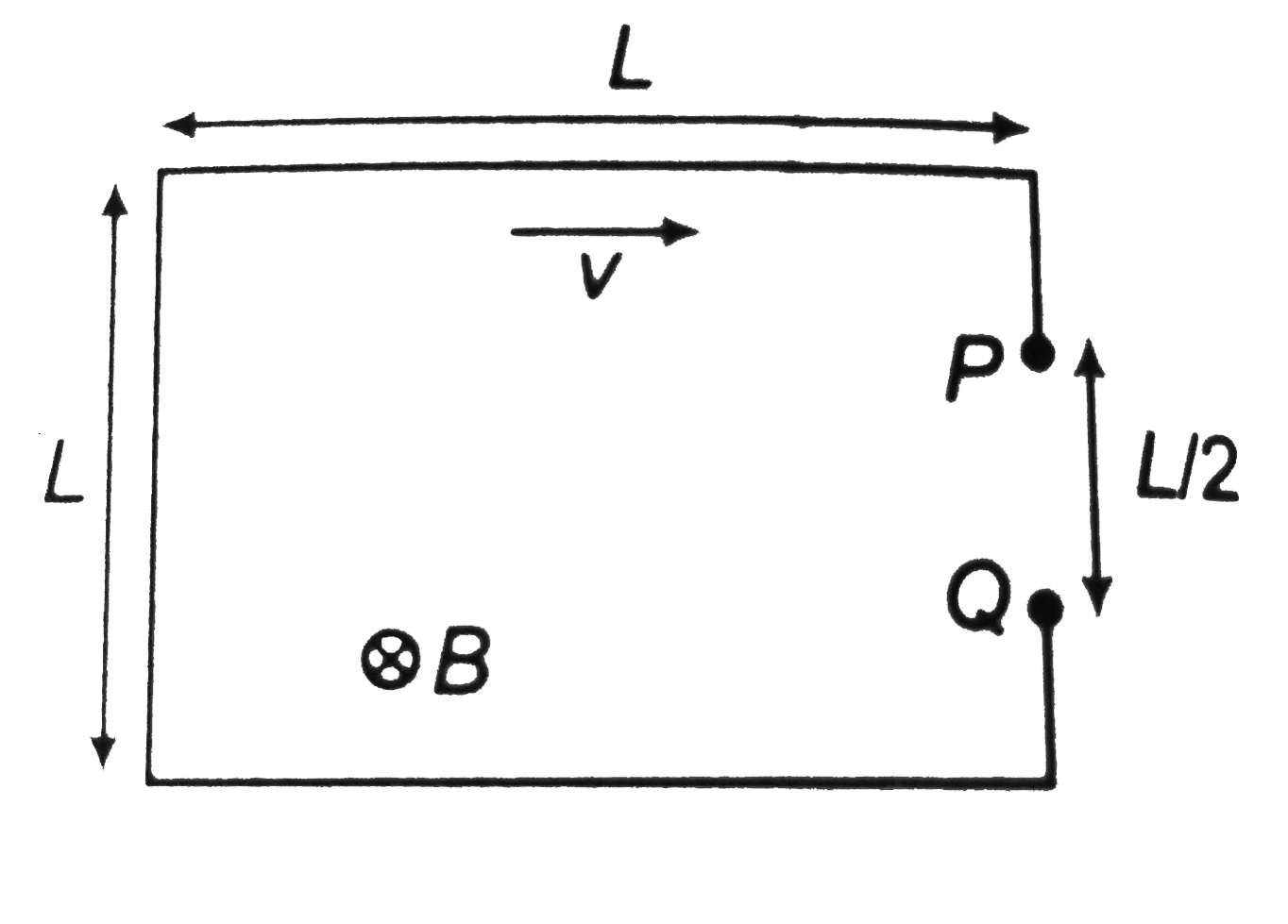
View SolutionThe loop shown moves with a velocity `v` in a uniform magnetic field of magnitude `B`, directed into the paper. The potential differene between point `P` and `Q` is e. Then
View SolutionThe magnitude of the earth's magnetic field at the north pole is `B_(0)` . A horizontal conductor of length `l` moves with a velocity `v` . The direction of `v` is perpendicular to the conductor. The induced emf is
(i) zero, if `v` is vertical
(ii) `B_(0)lv` , if `v` is vertical
(iii) zero, if `v` is horizontal
(iv) `B_(0)lv` , if `v` is horizontal
View SolutionA vertical conducting ring of radius `R` falls vertically in a horizontal magnetic field of magnitude `B`. The direction of `B` is perpendicular to the plane of the ring. When the speed of the ring is `v,
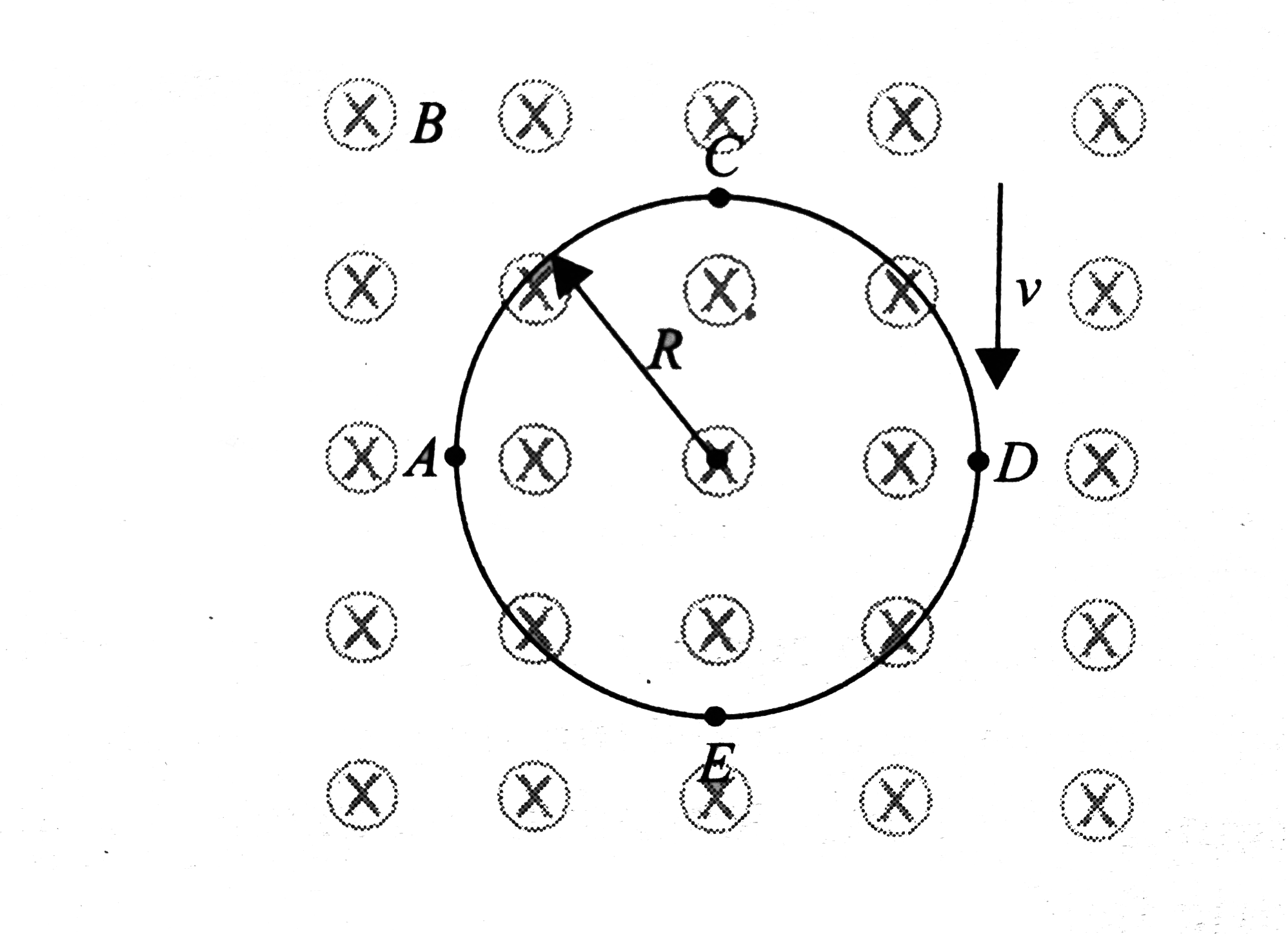
View SolutionTwo conducting rings of radii `r` and `2r` move in apposite directions with velocities `2 upsilon` and `upsilon` respectively on a conducting surface `S`. There is a uniform magnetic field of magnitude `B` perpendicular to the plane of the rings. The potential difference between the highest points of the two rings is
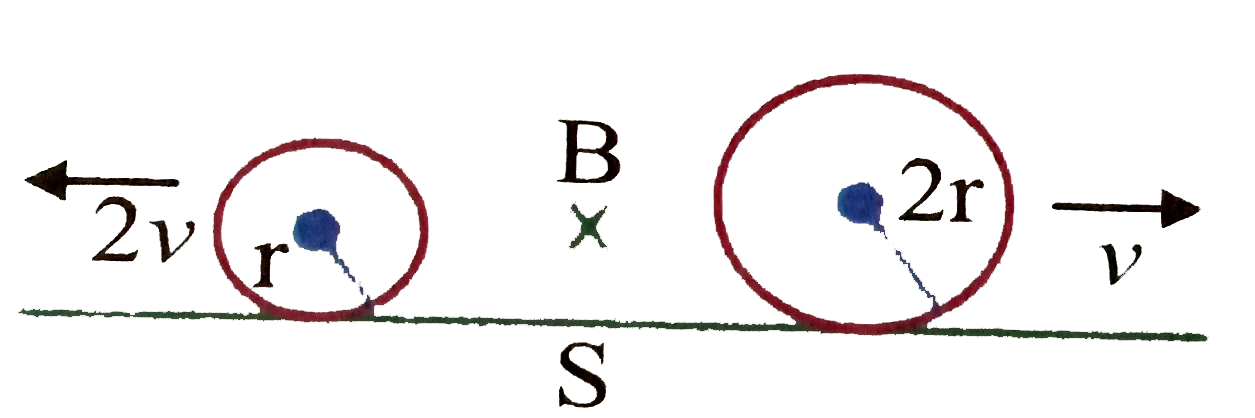
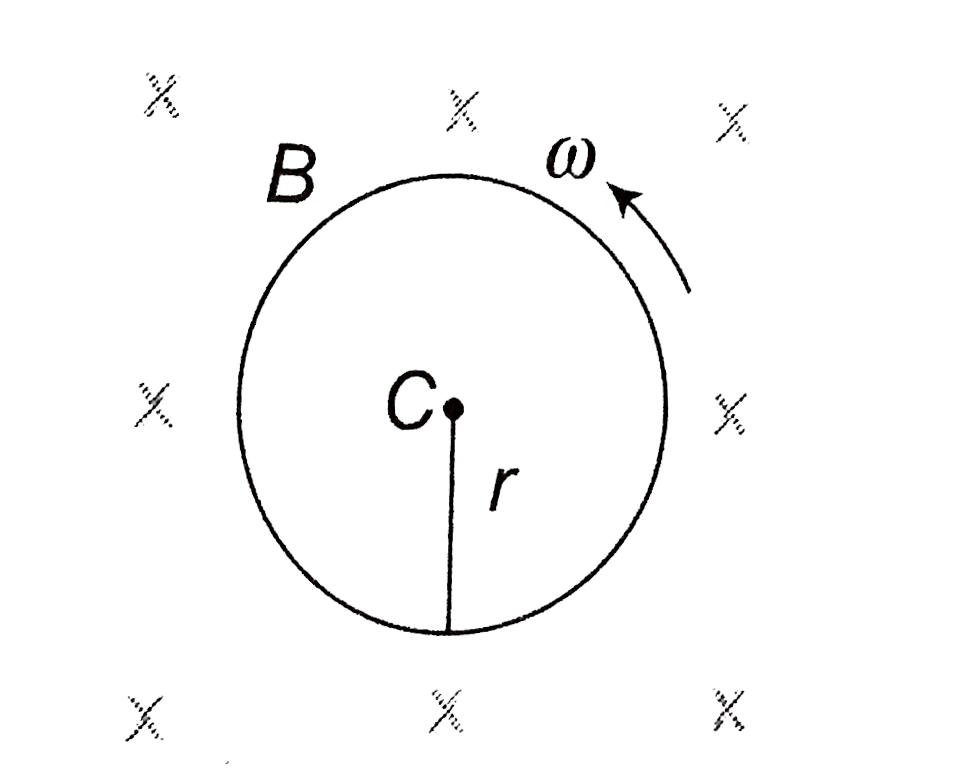
View SolutionA conducting disc of radius `r` spins about its axis an angular velocity `omega` . There is a uniform magnetic field of magnitude `B` perpendicular to the plane of the disc `C` is the centre of the disc.
(i) No emf is induced in the disc
(ii) The potential difference between `C` and the rim is `1//2Br^(2)omega`
`C` is at a higher potential than the rim
(iv) Current flows between `C` and the rim
View SolutionA flat coil, `C`, of `n` turns, area `A` and resistance `R`, is placed in a uniform magnetic field of magnitude `B`. The plane of the coil is initially perpendicular to `B`. The coil,is rotated by an angle `theta` about a diameter and charge of amount `Q` flows through it. Choose the correct alternatives.
View SolutionThe `SI` unit of inductance, the henry can be written as
View Solution
All Questions questions and complete solutions for chapter ELECTROMAGNETISM of D MUKHERJEE PHYSICS (HINGLISH) of Class 12