Complete Solutions to Example of chapter WAVE OPTICS of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | WAVE OPTICS |
| DC PANDEY NEET | Example |
| Number Of Questions | 57 |
| Academic Year | 2023-24 |
A wave front is represented by the plane `y=5-x` Find the direction of propagation of wave.
View SolutionA plane wavefront incident on a reflecting surface at an angle of `30^(@)` with horizontal. Find the angle of reflected wavefront with horizontal
View SolutionA plane wavefront incident on a reflecting surface at and angle of `30^(@)` with vertical. Find the angle (acute) of reflected wave with vertical.
View SolutionA plane wavefront is incident at an angle of `37^(@)` with horizontal a boundary of refractive medium from air `(mu=1)` to a medium of refractive index `mu=3/2`. Find the angle of refracted wavefront with horizontal
View SolutionA plane wavefront of yellow light with wavelength `0.5mum` in air suffers refraction in a medium in which velocity of light is `2xx10^(8)ms^(-1)` them, the wavelength of the light associated with a wavefront in the medium would be
View SolutionTwo sources of intensity `I` and 4I are used in an interference experiment. Find the intensity at a point where the waves from two sources superimpose with a phase difference of (a) zero, (b) `pi//2`, (c ) `pi` and (d) ratio of maximum and minimum intensity.
View SolutionTwo sources with intensity `I_(0)` and `4I_(0)` respectively, interfere at a point in a medium. Find the ratio of
(i) maximum and minimum possible intensities,
(ii) ratio of amplitudes
View SolutionTwo incoherent sources of light emitting light of intensity `I_(0)` and `3I_(0)` interfere in a medium. Calculate, the resultant intensity at any point.
View SolutionIn a YDSE green light of wavelength 500 nm is used. Where will be the second bright fringe be formed for a set up in which separation between slits is 4 mm and the screen is placed 1 m from the slits?
View SolutionBichromatic light is used in YDSE having wavelengths `lambda_(1)=400nm` and `lambda_(2)=700nm` Find minimum order of `lambda_(1)` which overlaps with `lambda_(2)`
View SolutionYoung's double slit experiment is carried out using microwaves of
wavelength `lambda=3cm`. Distance between the slits is `d= 5cm` and the distance
between the plane of slits and the screen is `D=100cm`.
(a) Find total number of maxima and
(b) their positions on the screen.
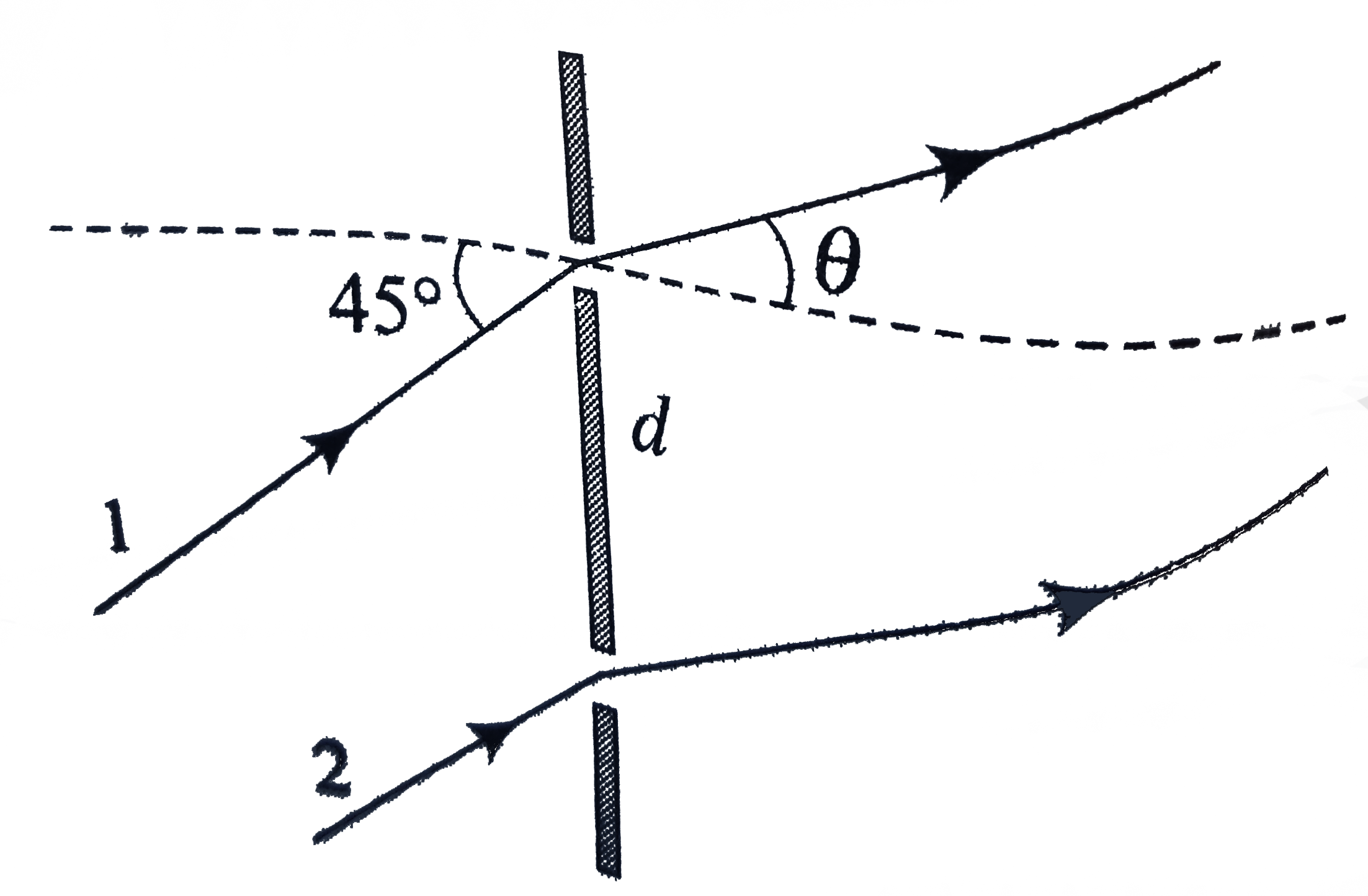
View SolutionDistance between the slit shown in figure is `d = 20 lambda`, where `lambda` is the wavelength of light used. Find the angle `theta` where
a. central maxima (where path difference is zero) is obtained, and
b. third-order maxima is obtained.
View SolutionIn YDSE, the two slits are separated by 0.1 mm and they are
0.5 m from the screen. The wavelength of light used is 5000 Å. Find the distance
between 7th maxima and 11 th mimima on the upper side of screen.
View SolutionIn YDSE, the slits are seperated by `0.28` mm and the screen is placed 1.4 m away. The distance between the first dark fringe and fourth bright fringe is obtained to be `0.6` cm Determine the wavelength of the light used in the experiment.
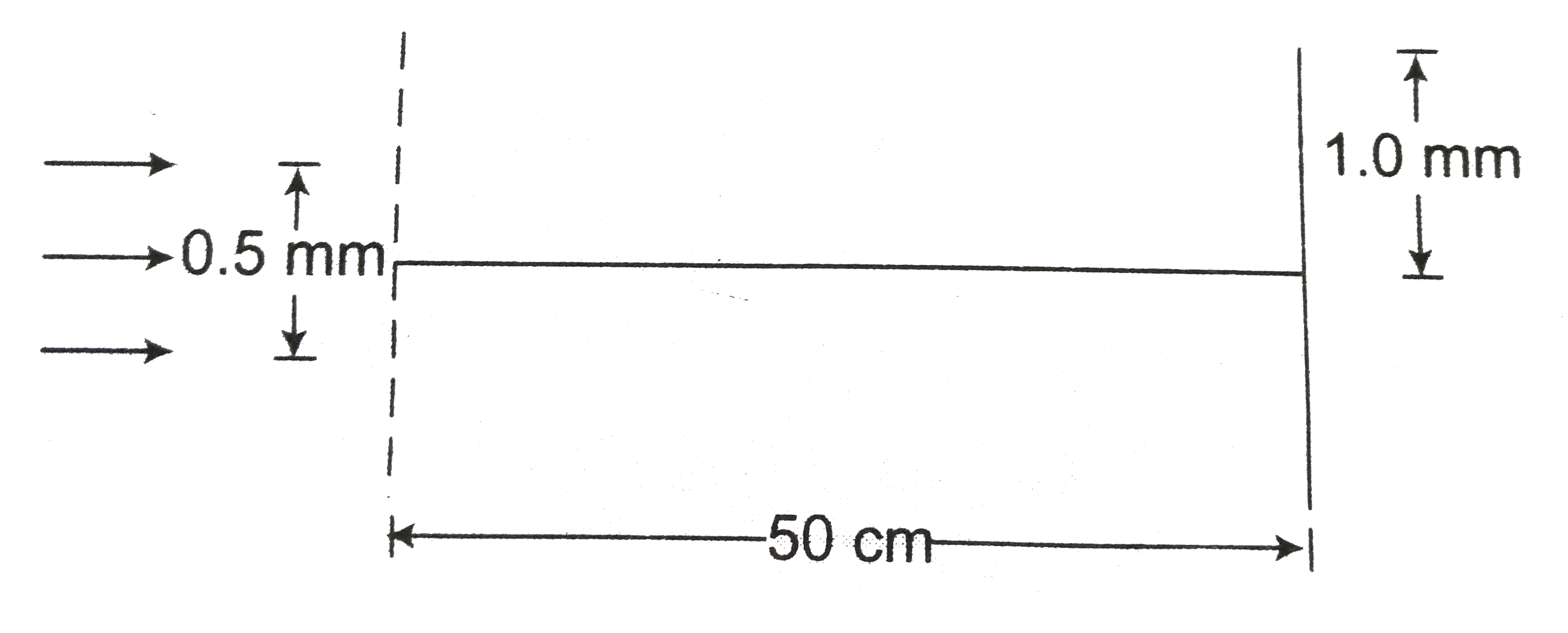
View SolutionWhite coherent light `(400 nm-700 nm)` is sent through the slits of a YDSE. `D=0.5 mm`, D=50 cm. There is a hole in the screen at a point `1.0 mm` away (along the width of the fringes) from the central line.
(a) Which wavelength will be absent in the light coming from the hole?
(b) Which wavelength(s) will have a strong intensity?
View SolutionTwo slits in YDSE are placed 2 millimetre from each other. Interference pattern is observedon a screen placed 2 m from the plane of slits. What is the fringe width for a light of wavelength 400 nm?
View SolutionIn young's double slit experiment with monochromic light, fringes are obtained on a screen placed at some distance from the plane of slits. If the screen is moved by 5 cm towards the slits, then the change in fringe width is `30mum` if the distance between the slits is 1mm, then calculate wavelength of the light used.
View SolutionFringe width in a particular YDSE is measured to be `beta` What will be the fringe width, if wavelength of the light is doubled, separation between the slits is halved and separation between the screen and slits is tripled ?
View SolutionWhose fringe width will be larger, the one for red light or the one for yellow light, all other things be the same?
View SolutionTwo slits in YDSE are placed 1 mm from each other. Interference pattern is observed on a screen placed 1m from the plane of slits. What is the angular fringe width for a light of wavelength 400 nm
View SolutionTwo slits are separated by 0.320 mm. A beam of 500 nm light strikes the slits, producing an interference pattern. Determine the number of maxima observed in the angular range `- 30.0^(@) lt theta lt 30.0^(@)`.
View SolutionIn Young's double slit experiment separation between slits is 1mm, distance of screen from slits is 2m. If wavelength of incident light is 500 nm. Determine
(i) fringe width
(ii) angular fringe width
(iii) distance between 4 th bright fringe and 3rd dark fringe
(iii) If whole arrangement is immersed in water `(mu_(w)=4//3)`, new angular fringe width.
View SolutionTwo coherent sources each emitting light of intensity `I_(0)` Interfere, in a medium at a point, where phase different between them is `(2pi)/3`. Then, the resultant intensity at that point would be.
View SolutionIn a Young's double slit set up using monochromatic light of wavelength `lambda` the intensity of light at a point, where path difference is `2lambda` is found to be `I_(0)` What will be the intensity at a point when path different is `lambda//3` ?
View SolutionMaximum intensity in YDSE is `I_0`. Find the intensity at a
point on the screen where
(a) The phase difference between the two interfering beams is `pi/3.`
(b) the path difference between them is `lambda/4`.
View SolutionIn a Young's double slits set up, the wavelength of light used is 546nm. The distance of screen from slits is 1m. The slits separation is 0.3 mm.
(a) Compare the intensity at a point P distant 10mm from the central fringe where the intensity is `I_0`.
(b) Find the number of bright fringes between P and the central fringe.
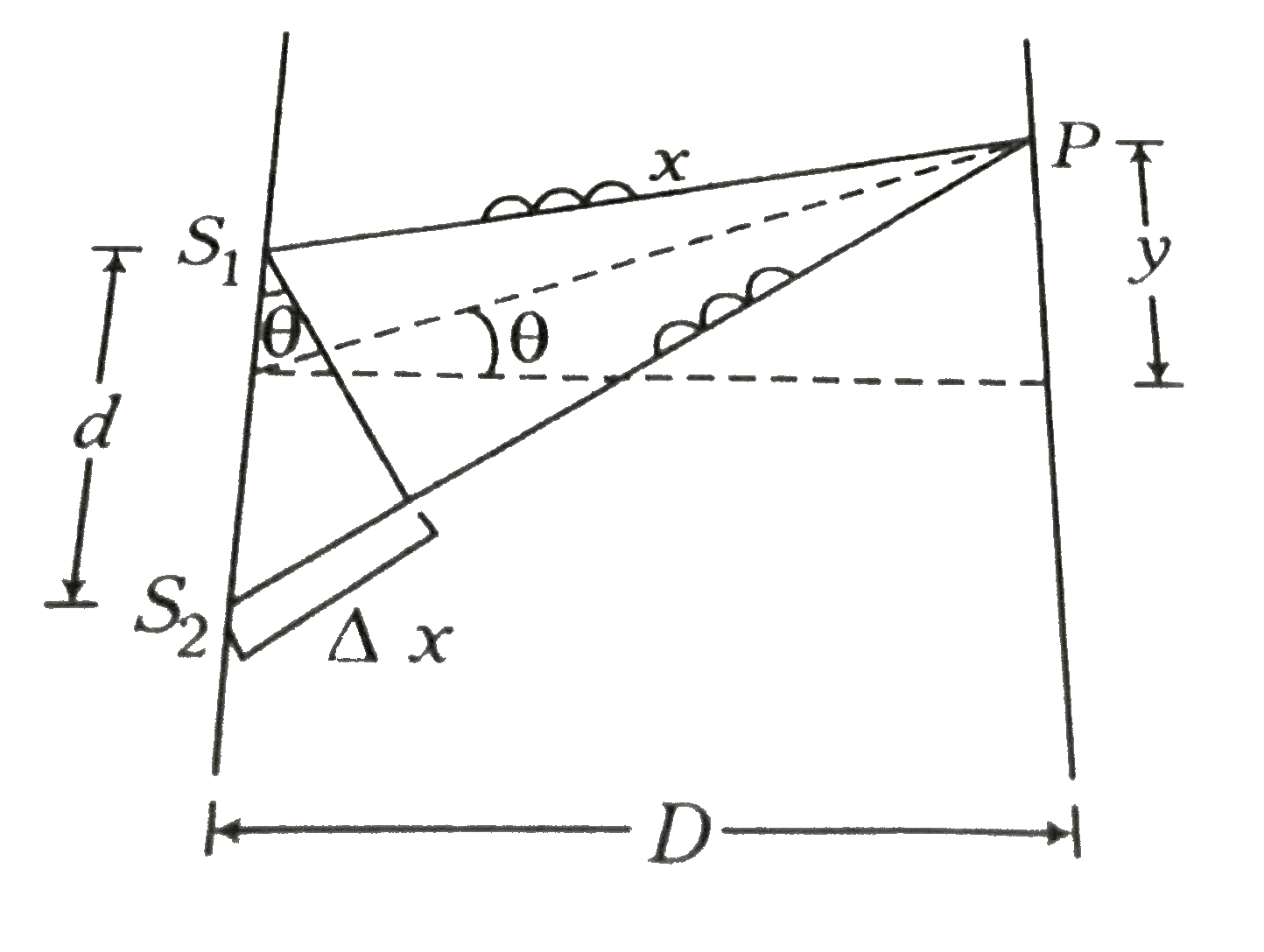
View SolutionConsider an interference arrangement similar to YDSE. Slits `S_(1)` and `S_(2)` are illuminated with coherent microwave sources each of frequency 2 MHz. The sources are synchronised to have zero phase difference. The slits are separated by distance d= 75m. The intensity `I_((theta))` is measured as a function of `theta` where `theta` is defined as shown in figure. if `I_(0)` is maximum intensity then calculated `I_((theta))` for `(i) theta= 0^(@), (ii) theta=pi//6 and (iii) theta = pi//2`
View SolutionLight travels in a mica sheet of refractive index `1.4` and length 10 cm.Find the optical path equivalent to length of the sheet.
View SolutionInterference fringes are produced by a double slit arrangement
and a piece of plane parallel glass of refractive index 1.5 is interposed in one of
the interfering beam. If the fringes are displaced through 30 fringe widths for
light of wavelength `6xx 10^(-5) cm`, find the thickness of the plate.
View SolutionIn YDSE using monochromatic light, the fringe pattern shifts by a certain distance on the screen when a mica sheet of refractive index `1.5` and thickness 2 microns is introduced in the path of one of the interfering waves. The mica sheet is then removed and the distance between the plane of slits and the screen is doubled. It is found that the distance between successive maxima (or minima) now is the same as the observed fringe shift upon the introduction of the mica sheet. Calculate the wavelength of the light.
View SolutionIn YDSE, find the thickness of a glass slab `(mu=1.5)` which
should be placed in front of the upper slit `S_1` so that the central maximum now
lies at a point where 5th bright fringe was lying earlier (before inserting the
slab). Wavelength of light used is `5000 Å`.
View SolutionIn a double slit pattern `(lambda = 6000Å)`, the first order and tenth order maxima fall at 12.50 mm and 14.55mm from a particular reference point. If `lambda` is changed to 5500Å, find the position of zero order and tenth order fringes, other arrangements remaining the same.
View SolutionA double - slit apparatus is immersed in a liquid of refractive index `1.33` it has slit separation of 1mm and distance between the plane of slits and screen `1.33` m the slits are illuminated by a parallel beam of light whose wavelength in air is 800 nm.
(i) Calculate the fringe width.
(ii) One of the slits of apparatus is covered by a thin glass sheet of refractive index `1.53` Find the smallest thickness of the sheet to bring the adjacent minima on the axis.
View SolutionA monochromatic light of `lambda=500 nm` is incident on two identical slits separated by a distance of `5xx10^(-4)m`. The interference pattern is seen on a screen placed at a distance of `1 m` from the plane of slits. A thin glass plate of thickness `1.5xx10^(-6)m` and refractive index `mu=1.5` is placed between one of the slits and the screen. Find the intensity at the centre of the screen if the intensity is `I_(0)` in the absence of the plate. Also find the lateral shift of the central maxima and number of fringes crossed through centre.
View SolutionIn a double slit pattern `(lambda = 6000Å)`, the first order and tenth order maxima fall at 12.50 mm and 14.55mm from a particular reference point. If `lambda` is changed to 5500Å, find the position of zero order and tenth order fringes, other arrangements remaining the same.
View SolutionCalculate the minimum thickness of a soap bubble film `(mu=1.33)`that results in constructive interference in the reflected light if the film is illuminated with light whose wavelength in free space is `lambda=600nm`.
View SolutionIn solar cells, a silicon solar cell `(mu = 3.5)` is coated with a thin film
of silicon monoxide `SiO (mu = 1.45)` to minimize reflective losses from the surface.
Determine the minimum thickness of SiO that produces the least reflection at a
wavelength of 550nm, near the centre of the visible spectrum. Assume
approximately normal incidence .
View SolutionA parallel beam of white light falls on a thin film whose refractive index is equal to `4//3` . The angle of incidence `i=53^(@)` What must be the minimum film thickness, if the reflected light is to be coloured yellow `(lambda "of yellow" =0.6mum)` most intensity ? `(tan 53^(@)=4//3)`
View SolutionLight source of wavelength 400 nm used in a Lloyd's mirror, lies 1 mm above the plane mirror. Find the distance of first minima above the mirror. Distance of the screen from the plane of the sources is 2 m.
View SolutionA screen is placed `50 cm` from a single slit, which is illuminated with `6000 Å` light. If the distance between the first and third minima in the diffraction pattern is `3.00 mm`, what is the width of the slit ?
View SolutionA beam of light of wavelength 400 nm falls on a narrow slit and the resulting diffraction pattern is observed on a screen 2 m away from the slit. It is observed that 2nd order minima occurs at a distance of 2 mm from the position of central maxima. Find the width of hte slit.
View SolutionA beam of light of wavelength 600 nm from a distance source falls on a single slit 2 mm wide and the resulting diffraction pattern is observed on a screen 2m away. What is the distance between the first dark fringes on either side of central bright fringe ?
View SolutionA slit of width d is illuminated by red light of wavelength `6500Å` For what value of d will the first maximum fall atangle of diffractive of `30^(@)`
View SolutionA beam of light of wavelength 600 nm from a distant source falls on a single slit 1.0 mm wide and the resulting diffraction pattern is observed on a screen 2 m away What is the distance between the second bright fringe on either side of the central bright fringe ?
View SolutionA screen is placed 50cm from a single slit, which is illuminated with 6000Å light. If distance between the first and third minima in the diffraction pattern is 3.0 mm, what is the width of the slit?
View SolutionA beam of light of wavelength 600 nm from a distance source falls on a single slit 1 mm wide and resulting diffraction pattern is observed on a screen 2m away. What is the distance between the first bright fringes on either side of the central bright fringe ?
View SolutionIn a single slit diffraction experiment first minima for `lambda_1 = 660nm` coincides with first maxima for wavelength `lambda_2`. Calculate the value of `lambda_2`.
View SolutionA lens of focal length `1m` forms Fraunhofer diffraction pattern of a single slit of width `0.04 cm` in its focal plane. The incident light contains two wavwlength `lambda_(1)` and `lambda_(2)`. It is found that the fourth minimum crresponding to `lambda_(1)` and the fifth minimum corresponding to `lambda_(2)` occur at the same point `0.5 cm` from the central maximum. Compute `lambda_(1)` and `lambda_(2)`
View SolutionLight of wavelength 580 nm is incident on a slit having a width of `0.300` nm The viewing screen is 2.00 m from the slit. Find the positions of the first dark fringe and the width of the central bright fringe.
View SolutionA parallel beam of monochromatic light of wavelength 450 m passes through a long slit of width 0.2 mm. find the angular divergence in which most of the light is diffracted.
View SolutionAngular width of central maximum in the Fraunhoffer diffraction pattern of a slit is measured. The slit is illuminated by light of wavelength `6000Å`. When the slit is illuminated by light of another wavelength, the angular width decreases by `30%`. Calculate the wavelength of this light. The same decrease in the angular width of central maximum is obtained when the original apparatus is immersed in a liquid. Find the refractive index of the liquid.
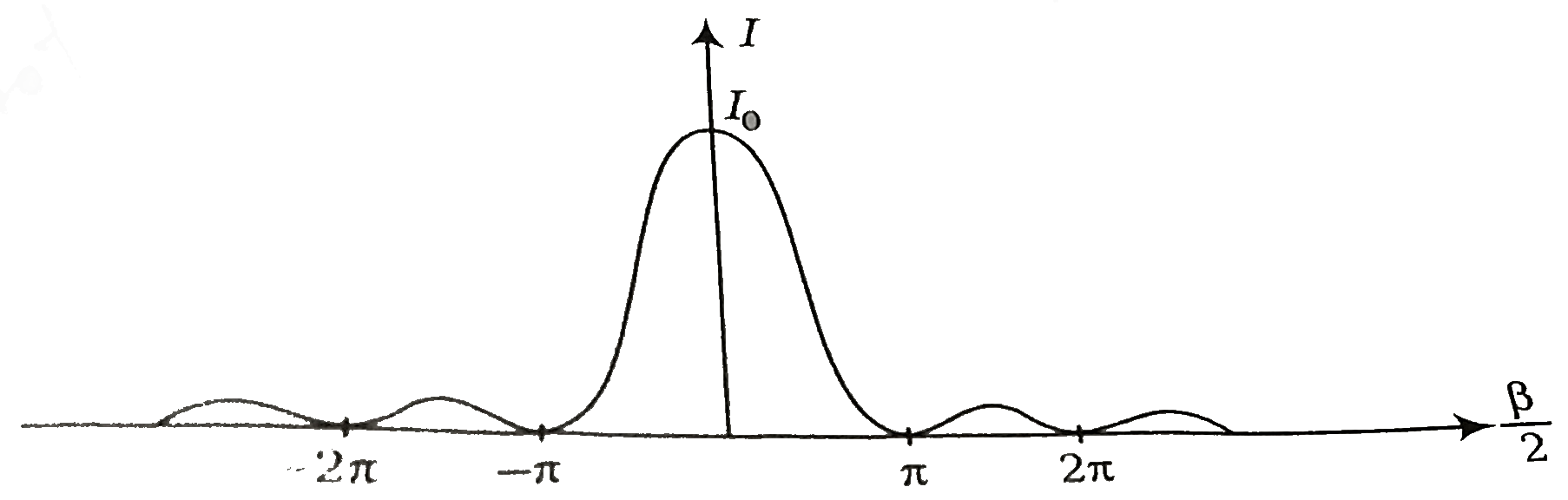
View SolutionFind the ratio of the intensities of the secondary maxima to the intensity of the central maximum for the single slit Fraunhofer diffraction pattern.
View SolutionFor what distance is ray optics a good approximation when the aperture is `2 mm` wide and wavelength is `600 nm` ?
View SolutionEstimate how large can be the aperture opening to work with laws of ray optics using a monochromatic light of wavelength 450 nm, to a distance of around 20 m.
View SolutionA diffraction grating has `1.26xx10^(4)` rulings uniformly spaced over width w=25.4 mm. It is illuminated at normal incidence by blue light of wavelength 450 nm At what angles to the central axis do the second order maxima occur
View SolutionTwo polaroids are oriented with their planes perpendicular to incident light and transmission axis making an angle of `30^(@)` with each other. What fraction of incident unpolarised light transmitted?
View SolutionWhen light of particular wavelength falls on a plane surface at an angle of incidence `60^(@)` then the reflected light becomes completely plane polarised Find the refractive index of surface material and the angle of refraction through it.
View Solution
Example questions and complete solutions for chapter WAVE OPTICS of DC PANDEY PHYSICS (HINGLISH) of Class 12
All Exercises from DC PANDEY NEET Class 12 PHYSICS WAVE OPTICS
- Assertion reason
- Check point
- Example
- For JEE Advanced B. More than one option is correct
- For JEE Advanced C. Comprehension Type Questions. Passage (I)
- For JEE Advanced C. Comprehension Type Questions. Passage (II)
- For JEE Advanced C. Comprehension Type Questions. Passage (III)
- For JEE Advanced D. Matrix Matching type Questions.
- For JEE Advanced E. Integer Type Questions
- For JEE Advanced Only one option is correct
- For JEE main (Only one option is Correct )
- match column
- medical entrances
- taking it together