Complete Solutions to physics of chapter LAWS OF MOTION of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | LAWS OF MOTION |
| DISHA | physics |
| Number Of Questions | 85 |
| Academic Year | 2023-24 |
A boy standing on a weighing machine observes his weight as 200 N. When he suddenly jumpes upwards, his friend notices that the reading increased to 400 N. The acceleration by which the boy jumped will be-
View SolutionA force of `(6hati+8hatj)` N acted on a body of mass 10 kg. The displacement after 10 sec, if it starts from rest, will be -
View SolutionA boat of mass 1000 kg is moving with a velocity of 5 `m//s.` A person of mass 60 kg jumps into the boat. The velocity of the boat with the person will be -
View SolutionA disc of mass 10 gm is kept horizontally in air by firing bullets of mass 5 g each at the rate of `10//s.` If the bullets rebound with same speed. The velocity with which the bullets are fired is -
View SolutionA fire man has to carry an injured person of mass 40 kg from the top of a building with the help of the rope which can withstand a load of 100 kg. The acceleration of the fireman if his mass is 80 kg, will be-
View SolutionA body of mass 0.02 kg falls from a height of 5 metre into a pile of sand. The body penetrates the sand a distance of 5 cm before stoping. What force has the sand exerted on the body ?
View SolutionThe magnitude of the force (in newtons) acting on a body varies with time t (in micro seconds) as shown in the fig AB, BC and CD are straight line segments. The magnitude of the total impulse of the force on the body from `t= 4 mus` to `t=16 mus` is ….Ns
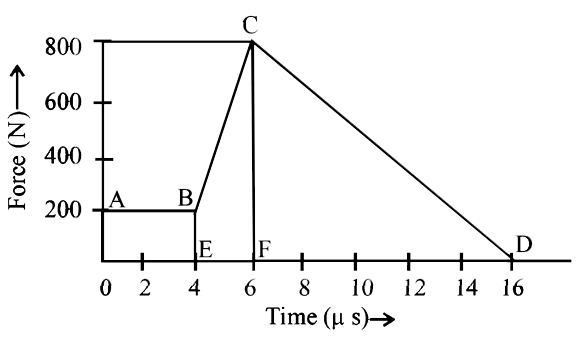
View SolutionThe total mass of an elevator with a 80 kg man in it is 1000 kg. This elevator moving upward with a speed of 8 `m//sec,` is brought to rest over a distance of 16m. The tension T in the cables supporting the elevator and the force exerted on the man by the elevator floor will respectively be-
View SolutionIn the arrangement shown in the Fig, the ends P and Q of an unstretchable string move downwards with uniform speed U. Pulleys A and B are fixed.
Mass M moves upwards with a speed
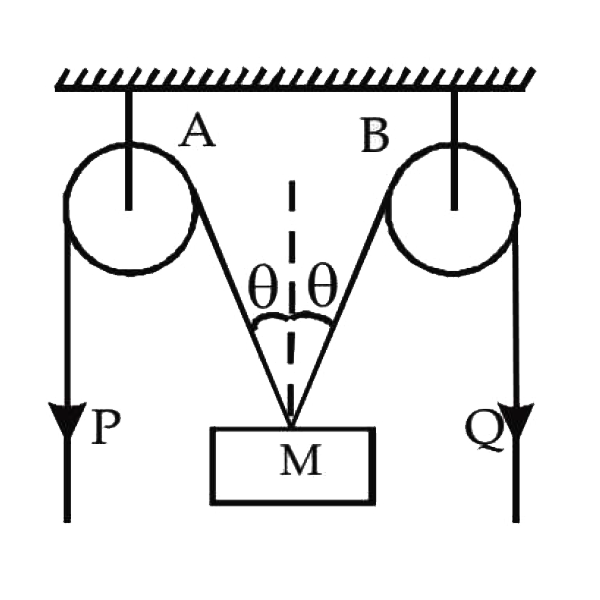
View Solution0An engine of mass` 5 xx 104` kg pulls a coach of mass`4xx10kg.` Suppose that there is a resistance of 1 N per 100 kg acting on both coach and engine, and that the driving
View SolutionA body whose mass 6 kg is acted upon by two forces
`(8hati+10hatj)` N and `(4hati+8hatj)`N. The accelerartion produced will be `("in" m//s^(2))-
View Solution2A car of 1000 kg moving with a velocity of `18 km//hr` is stopped by the brake force of 1000 N. The distance covered by it before coming to rest is -
View SolutionA block of metal weighing 2kg is resting on a frictionless plane. It is struck by a jet releasing water at a rate of `1kg s^(-1)` and at a speed of `5ms^(_1)`. The initial acceleration of the block is
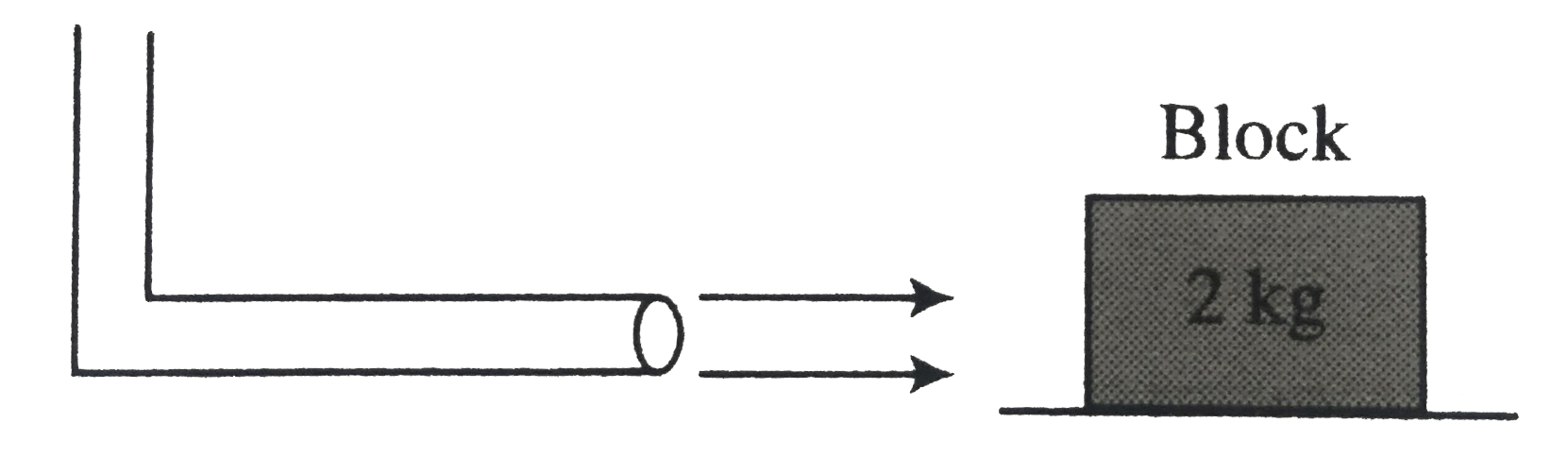
View Solution4A man fires the bullets of mass m each with the velocity v with the help of machine gun, if he fires n bullets every sec, the reaction force per second on the man will be -
View Solution5A body of mass 15 kg moving with a velocity of 10 `m//s` is to be stopped by a resistive force in 15 sec, the force will be -
View Solution6A cricket ball of mass 250 gm moving with a velocity of 24 m/s is hit by a bat so that it acquires a velocity of 28 m`// s` in the opposite direction. The force acting on the ball, if the contact time is `1//100` of a second, will be -
View SolutionA force of 2 N is applied on a particle for 2 sec, the change in momentum will be
View SolutionA body of mass 2 kg is moving along x-direction with a velocity of 2 `m//sec.` If a force of 4 N is applied on it along y-direction for 1 sec, the final velocity of particle willbe-
View Solutioncricket ball of mass 150 g is moving with a velocity of `12m//sec` and is hit by a bat so that the ball is turned back with a velocity of `20 m//sec,` the force on the ball acts for 0.01 sec, then the average force exerted by the bat on the ball will b
View Solution0A body of mass 20 kg moving with a velocity of 3 `m//s,` rebounds on a wall with same velocity. The impulse on the body is -
View Solution1A mass of 60 kg is on the floor of a lift moving down. The lift moves at first with an acceleration of 3 `3m//sec^(2),` then with constant velocity and finally with a retardation of `3m//sec^(2),` Choose the correct options related to possible reactions exerted by the lift on the body in each part of the
(1) 408 N (2)500 N (3) 768 N (4) 508
View SolutionA mass of 10 kg is hung to a spring balance in lift. If the lift is moving with an acceleration `g//3 `in upward & downward directions, choose the correct options related to the reading of the spring balance.
View SolutionChoose the correct option
View SolutionPseudo force is an imaginary force which is recognised only by a non-inertial observer to explain the physical situation according to newton’s laws. Magnitude of pseudo force Fp is equal to the product of the mass m of the object and the acceleration a of the frame of reference. The direction of the force is opposite to the direction of acceleration,`F_(p)=-ma`
spring weighing machine inside a stationary lift reads 50 kg when a man stand on it. What would happen to the scale reading if the lift is moving upward with (i) constant velocity (ii) constant acceleratioin
View SolutionA 25 kg lift is supported by a cable. The acceleration of the lift when the tension in the cable is 175 N, will be -
View SolutionA body is suspended by a string from the ceiling of an elevator. It is observed that the tension in the string is doubled when the elevator is accelerated. The acceleration will be
View SolutionSTATEMENT-1: A cloth covers a table. Some dishes are kept on it. The cloth can be pulled out without dislodging the dishes from the table.
STATEMENT-2: For every action there is an equal and opposite reaction.
View SolutionStatement-1 : If the net external force on the body is zero then its acceleration is zero. Statement-2 : Acceleration does not depend on force
View SolutionStatement-1 : The slope of momentum versus time graph give us the acceleration. Statement-2 : Force is given by the rate of change of momentum
View SolutionA block of mass M is pulled along a horizontal frictionless surface by a rope of mass m. If a force P is applied at the free end of the rope, the force exerted by the rope on the block will be
View SolutionA body of mass 50 kg is pulled by a rope of length 8 m on a surface by a force of 108N applied at the other end. The force that is acting on 50 kg mass, if the linear density of rope is 0.5 kg/m will be -
View SolutionA rope of length 15 m and linear density 2 kg/m is lying length wise on a horizontal smooth table. It is pulled by a force of 25 N. The tension in the rope at the point 7 m away from the point of application, will be -
View SolutionA force of 100 N acts in the direction as shown in figure on a block of mass 10 kg resting on a smooth horizontal table. The speed acquired by the block after it has .moved a distance of 10 m, will be - (in m/sec) `("g"=10"m/sec"^(2))`

View SolutionIn the above example, the velocity after 2 sec will be - (in m/sec)
View SolutionTwo blocks of mass m = 1 kg and M = 2 kg are in contact on a frictionless table. A horizontal force F(= 3N) is applied to m. The force of contact between the blocks, will be-
View SolutionA force produces an acceleration of `5" m/s"^(2)` in a body and same force an acceleration of `15 "m/s"^(2)` in another body. The acceleration produced by the same force when applied to the combination of two bodies will be -
View SolutionWhat is the tension in a rod of length length L and mass M at a distance y from`F_(1)` when the rod is acted on by two unequal force `F_(1)` and `F_(2) ( lt F_(1))` as shown in.
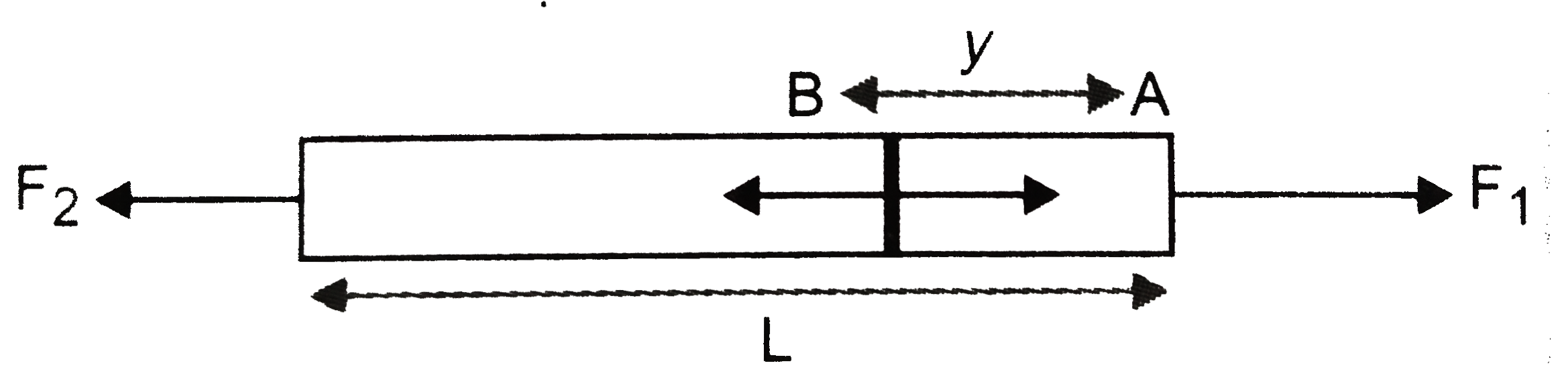
View SolutionA force produces acceleration `1 , 1/2 , 1/3,1/4 , ......... ("all in m/ s"^(2))`, applied separetly to n bodies. If these bodies are combined to form single one, then the acceleration of the system will be, if same force is taken into account.
View SolutionTwo blocks of masses 6 kg and 4 kg connected by a rope of mass 2 kg are resting on frictionless floor as shown in fig. If a constant force of 60 N is applied to 6 kg block, tension in the rope at A, B, and C will respectively be -
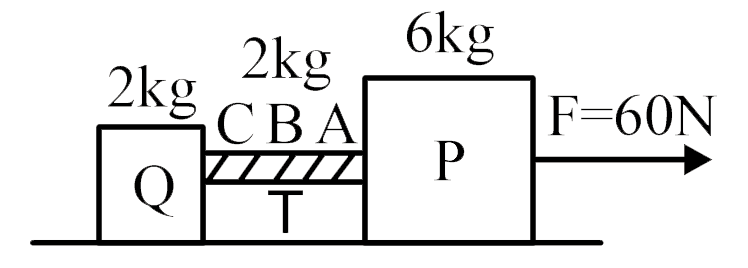
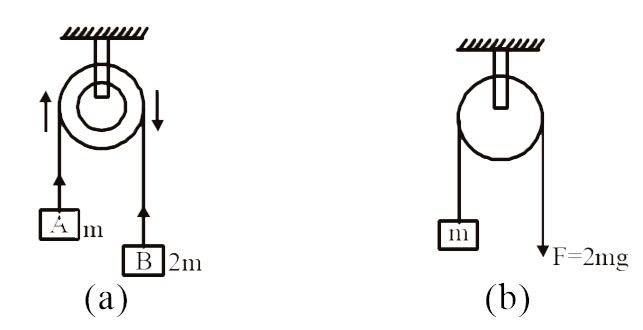
View SolutionThe pulley arrangements of fig (a) and (b) are identical. The mass of the rope is negligible. In (a) the mass m is lifted up by attaching a mass 2 m to the other end of the rope. In (b) m is lifted up by pulling the other end of the rope with a constant downward force F = 2 mg. Which of the following is correct?
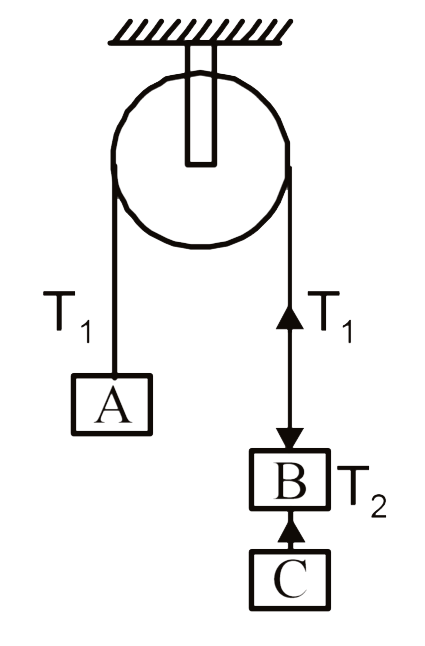
View SolutionThree equal weights of mass m each are hanging on a string passing over a fixed pulley as shown in fig. The tensions in the string connecting weights A to B and B to C will respectively be -
View SolutionIn the situation shown in figure, both the pulleys and the strings are light and all the surfaces are frictionless. The acceleration of mass M, tension in the string PQ and force exerted by the clamp on the pulley, will respectively be -
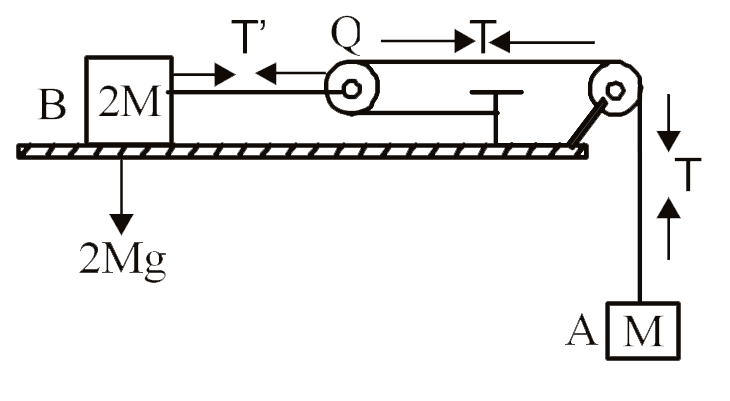
View SolutionA body of mass 50kg resting on a smooth inclined plane is connected by a massless inextensible string passing over a smooth pulley, at the top of the inclined plane have another mass of 40 kg as shown in the figure. The distance through which 50 kg mass fall in 4 sec will be - (The angle of the inclined plane is `30^(@)`)
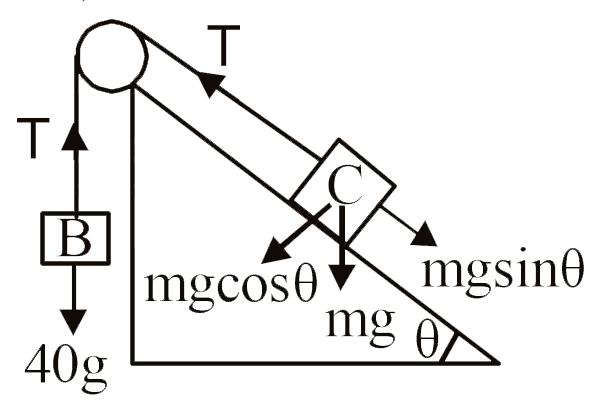
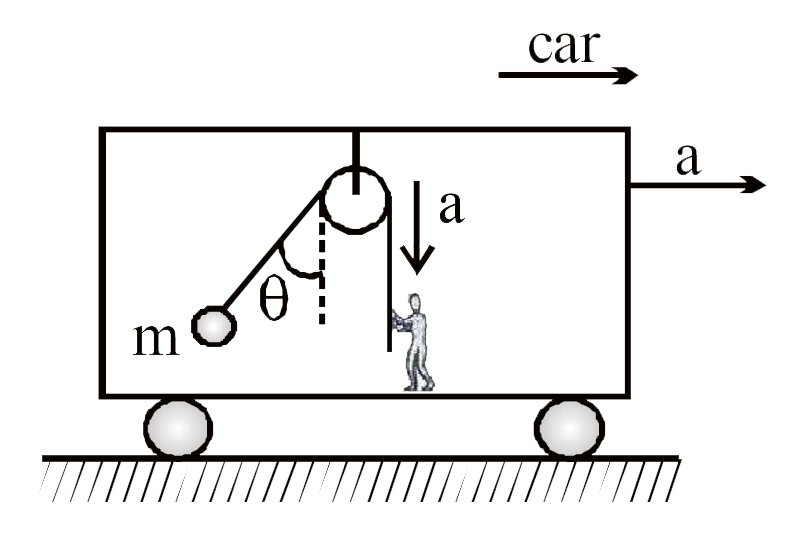
View SolutionA bob is hanging over a pulley inside a car through a string. The second end of the string is in the hand of a person standing in the car. The car is moving with constant acceleration 'a' directed horizontally as shown in figure. Other end of the string is pulled with constant acceleration ‘a’ vertically. The tension in the string is –
View SolutionIn the fig shown, the velocity of each particle at the end of 4 sec will be -`

View SolutionIn the above example, the height ascended or descended, as the case may be, during that time i.e. 4 sec will be -
View SolutionIn the above question, if at the end of 4 sec, the string be cut, the position of each particle in next 2 seconds will respectively be -
View SolutionConsider the double Atwood’s machine as shown in the figure. What is acceleration of the masses ?`
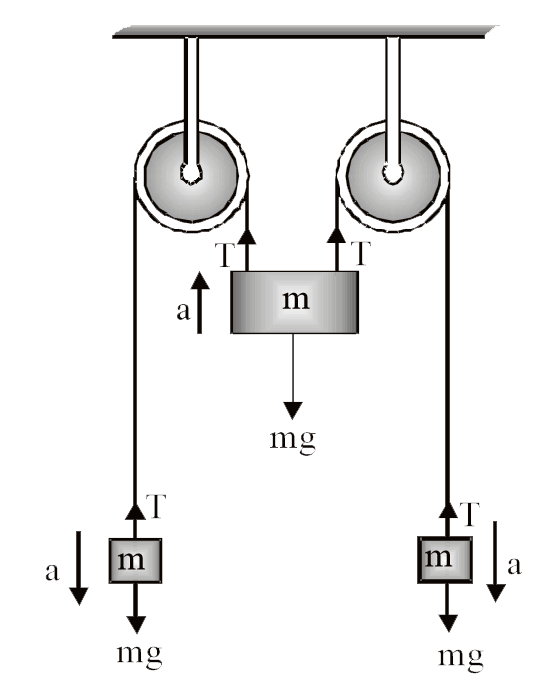
View SolutionIn above question, what is the tension in each string ?
View SolutionChoose the correct options-
(1) Inertia ` prop` mass
(2) `1 " newton"=10^(5)" dyne"`
(3) Thrust on rocket `overset rightarrow"F"=(Delta"M")/(Delta"t")" "overset rightarrow"V"-"M"oversetrightarrow"g"`
(4) Apparent weight of a body in the accelerated lifts is W=m (g+a).
View SolutionChoose the correct statements-
(1) For equilibrium of a body under the action of concurrent forces `oversetrightarrow"F"_(1)+oversetrightarrow"F"_(2)+oversetrightarrow"F"_(3)+.......oversetrightarrow"F"_("n")=0`
(2) If the downward acceleration of the lift is a = g, then the body will experience weightlessness.
(3) If the downward acceleration of the body is a gt g, then the body will rise up to the ceiling of lift
(4) If the downward acceleration of the lift is a gt g, then the body will experience weightlessness.
View SolutionA bead of mass m is attached to one end of a spring of natural length R and spring constant `K=((sqrt(3)+1)mg)/(R )`. The other end of the spring is fixed at a point A on a smooth vertical ring of radius R as shown in fig. The normal reaction at B just after it is released to move is
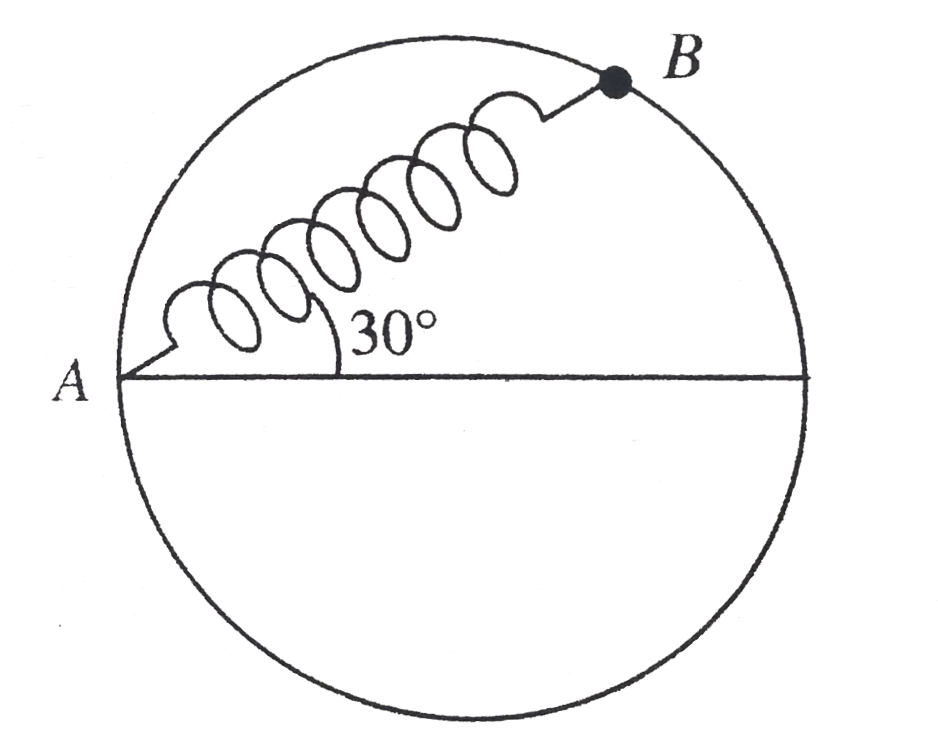
View SolutionA bead of mass m is attached to one end of a spring of natural length R and spring constant `K=((sqrt(3)+1)mg)/(R )`. The other end of the spring is fixed at a point A on a smooth vertical ring of radius R as shown in fig. The normal reaction at B just after it is released to move is

View SolutionA Bead of mass `m` is attached to one end of a spring of natural length `'R'` and spring cosntant `'k=((sqrt3+1)mg)/R'`. The other end of the spring is fixed at point `'A'` on a smooth vertical ring of radius `'R'` as shown
View SolutionStatement I: In fig., ground is smooth and masses of both the block are differents. The net force acting on each of the block is not same.
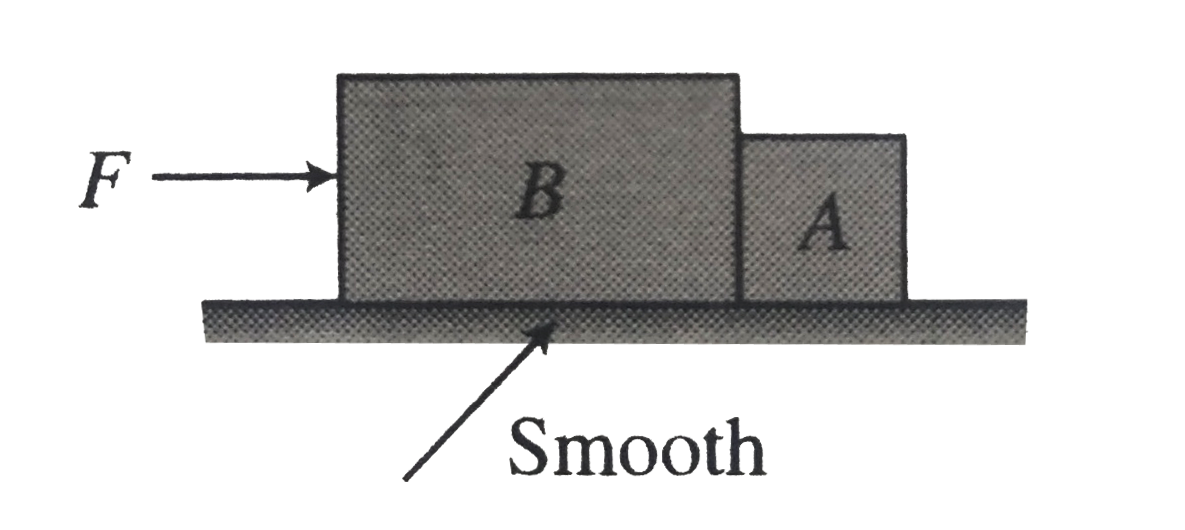
Statement II: Acceleration of both will be different.
View SolutionStatement I: Block A is moving on the horizontal surface towards right under action of force F. All surfaces are smooth. At the instant shown the force exerted by block A on block B is equal to net force on block B.

Statement II: From Newton's third law, the force exerted by block A on B is equal in magnitude to force exerted by block B on A.
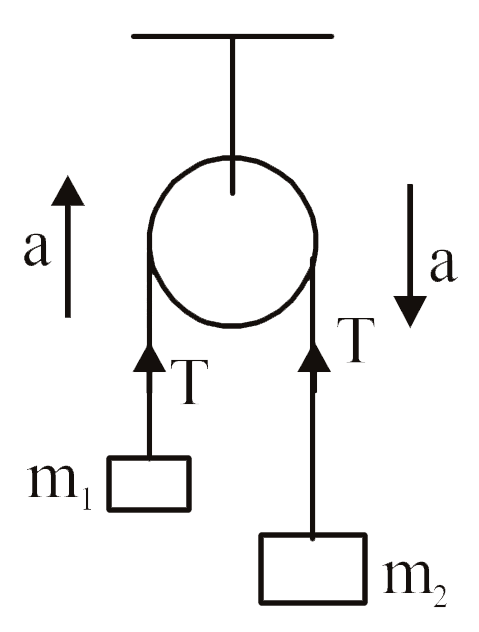
View SolutionStatement– 1 : In the given fig. `"a"=(("m"_(2)-"m"_(1))/("m"_(1)+"m"_(2)))"g"`
Statement– 2 : In the given fig., `"T"=("m"_(1)+"m"_(2))/(2"m"_(1)"m"_(2))`
View SolutionThe body of mass 400 g slides on a rough horizontal surface. If the frictional force is 3.0 N, find (a). the angle made by the contact force on the body with the vertical and (b). the magnitude of the contact force. Take `g=10 m/s^2`
View SolutionThe body of mass 400 g slides on a rough horizontal surface. If the frictional force is 3.0 N, find (a). the angle made by the contact force on the body with the vertical and (b). the magnitude of the contact force. Take `g=10 m/s^2`
View SolutionThe coefficient of static friction between a block of mass m and an incline is `mu_s=03`, (a). What can be the maximum angle `theta` of the incline with the horizontal so that the block does not slip on the plane? (b). If the incline makes an angle `theta/2` with the horizontal, find the frictional force on the block.
View SolutionThe coefficient of static friction between the two blocks shown in figure is `mu` and the table is smooth. What maximum horizontal forced F can be applied to he block of mass M so that the block move together?

View SolutionBlock A weighs `4 N` and block weigh `8 N` The coefficient of kinetic friction is `0.25` for all surface find the force `F` to slide `B` at a constant speed when (a)`A` rest on `B` and moves with it (b) `A` is held at rest and (c )`A and B` are connected by a light cord passing over a smooth putting as shown in fig 7.31 (a - c) restively.
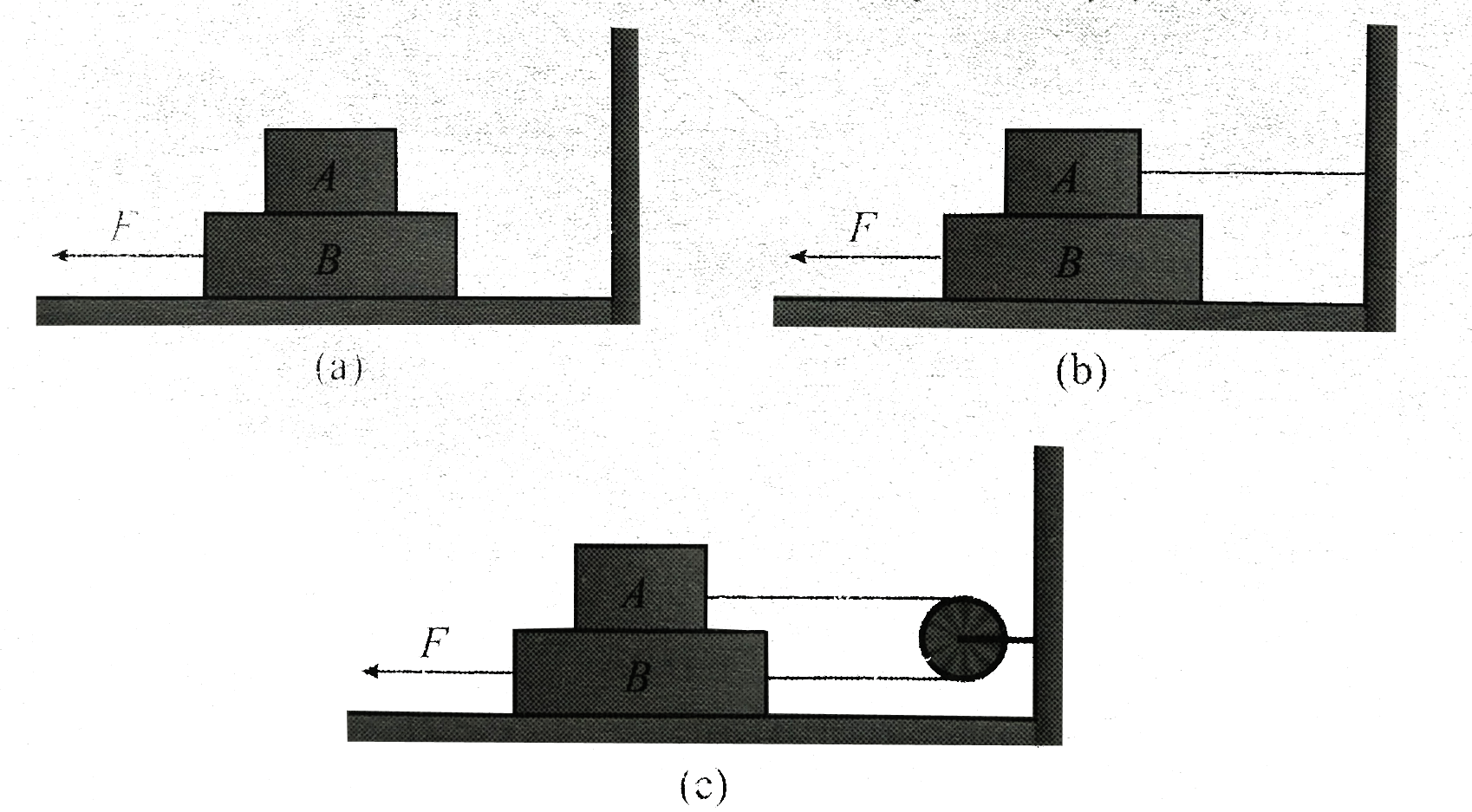
View SolutionBlock A weighs `4 N` and block weigh `8 N` The coefficient of kinetic friction is `0.25` for all surface find the force `F` to slide `B` at a constant speed when (a)`A` rest on `B` and moves with it (b) `A` is held at rest and (c )`A and B` are connected by a light cord passing over a smooth putting as shown in fig 7.31 (a - c) restively.

View SolutionBlock `A` weight `4N` and block `B` weighs `8N` The coefficient of kinetic friction is 0.25 for all surfaces. Find the force `F` to slide `B` at a constant speed when (a) A rests on `B` and moves with it (b) A is held at rest and `(c)` A and `B` are connected by a light cord passing over a smooth pulley as shown in and respectively .
 .
.
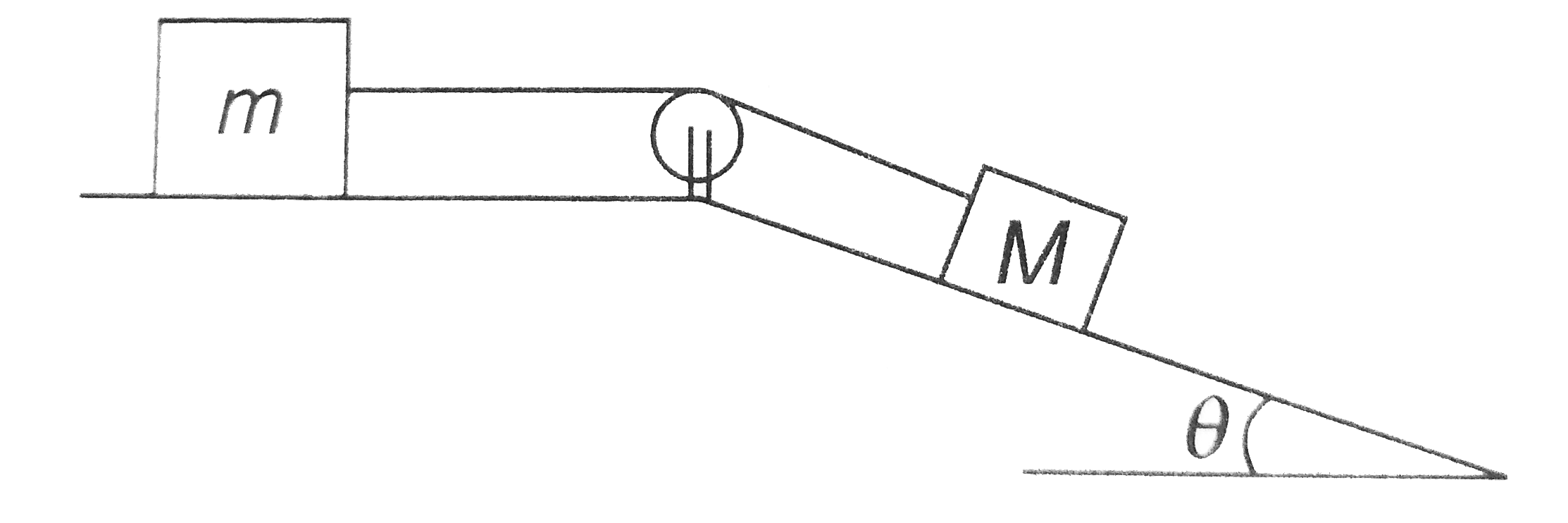
View SolutionFind the maximum value of (M/m) in the situation shown in figure so that the system remains at rest. Friction coefficient of both the contacts is `mu` , string is massless an pulley is friction less.
View SolutionA block placed on a horizotnal surface is being pushed by a force F making an angle `theta` with the vertical. If the friction coefficient is `mu`. How much force is needed to get the block just started. Discuss the situation when `tan thetaltmu`.
View SolutionAssuming the length of a chain to be L and coefficient of static friction `mu`. Compute the maximum length of the chain which can be held outside a table without sliding.
View SolutionIf the coefficient of friction between an insect and bowl is `mu` and the radius of the bowl is r, find the maximum height to which the insect can crawl in the bowl.
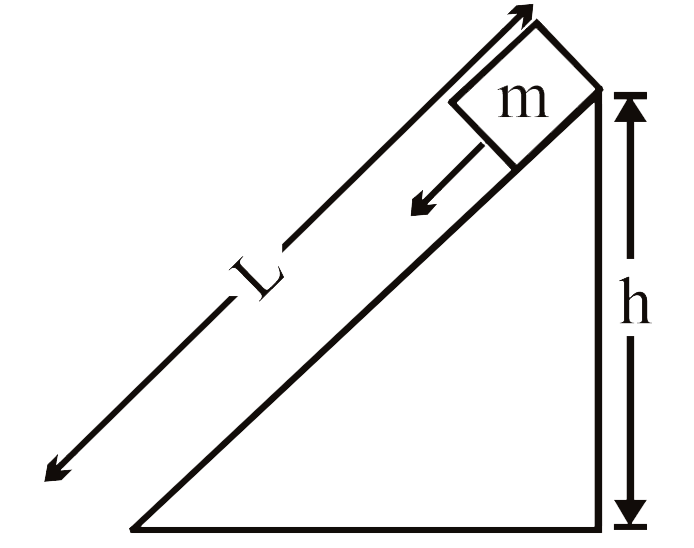
View SolutionA body of mass m is released from the top of a rough inclined plane as shown in figure. If the frictional force be F, then body will reach the bottom with a velocity
View SolutionA block of mass 2 kg is placed on the floor. The coefficient of static friction is 0.4.A force F of 2.5 N is applied on the block, as shown. Calculate the force of friction between the block and the floor. `("g = 9.8 ms"^(–2))`
View SolutionA block is kept on a horizontal table. The stable is undergoing simple harmonic motion of frequency `3Hz` in a horizontal plane. The coefficient of static friciton between block and the table surface is `0.72.` find the maximum amplitude of the table at which the block does not slip on the surface.
View SolutionTwo cars of unequal masses use similar tyres. If they are moving at the same initial speed, the minimum stopping distance
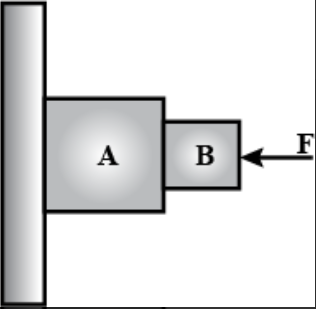
View SolutionConsider the situation shown in figure. The wall is smooth but the surfces of A and B in contact are rough. The friction on B due to A in equilibrium.
View SolutionA block is placed on a rough floor and a horizontal force F is applied on it.The force of friction f by the floor on the block is measured for different values of F and a graph is plotted between them.
View SolutionThe contact force exerted by a body A on another body B is equal to the normal force between the bodies. We concude that
View SolutionIt is easier to pull a body than to push, because -
View SolutionA block of metal is lying on the floor of a bus. The maximum acceleration which can be given to the bus so that the block may remain at rest, will be -
View SolutionChoose the correct statements –
(1) Kinetic friction is lesser than limiting friction.
(2) In rolling the surfaces at contact do not rub each other.
(3) If a body is at rest and no pulling force is acting on it, force of friction on it is zero.
(4) Kinetic friction is greater than limiting friction.
View SolutionChoose the correct statements –
(1) Force of friction is partically independent of microscopic area of surface in contact and relative velocity between them. (if it is not high)
(2) Normally with increase in smoothness friction decreases. But if the surface area are made too smooth by polishing and cleaning the bonding force of adhesion will increase and so the friction will increase resulting in 'Cold welding'
(3) Friction is a non conservative force, i.e. work done against friction is path dependent.
(4) Force of fricton depends on area
View SolutionChoose the correct options –
(1) Friction always opposes the motion
(2) Friction may opposes the motion
(3) If the applied force is increased the force of static friction remains constant.
(4) If the applied force is increased the force of static friction also increases upto limiting friction.
View SolutionA block of mass `1kg `is placed on a rough horizontal surface. A spring is attached to the block whose other end is joined to a rigid wall, as shown in the figure. A horizontal force is applied on the block so that it remains at rest while the spring is elongated by `x(x ge (mumg)/(k))`. Let `F_(max)` and `F_(mi n)` be the maximum and minimum values of force `F` for which the block remains in equilibrium. For a particular `x, F_(max)-F_(mi n)=2N`. Also shown is the variation of `F_(max)+F_(mi n)` versus `x`, the elongation of the spring.
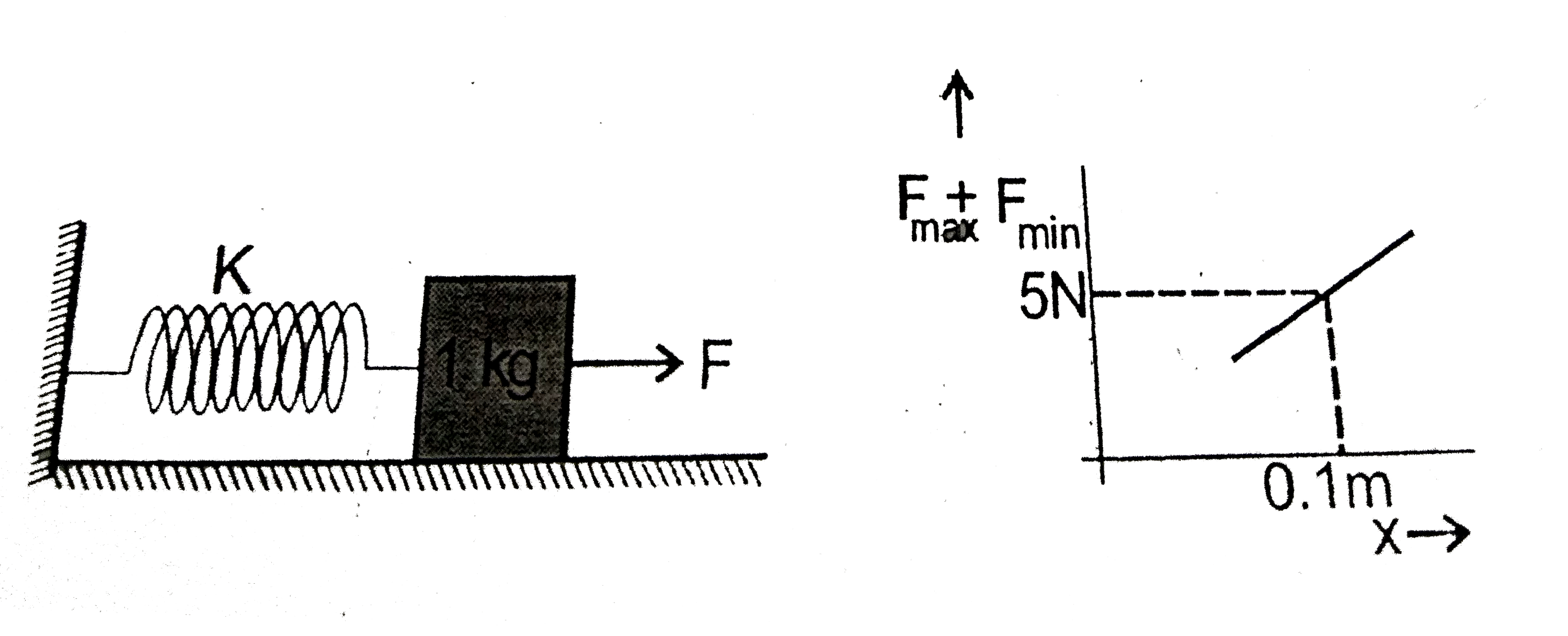
The coefficient of friction between the block and the horizontal surface is `:`
View SolutionA block of mass `1kg `is placed on a rough horizontal surface. A spring is attached to the block whose other end is joined to a rigid wall, as shown in the figure. A horizontal force is applied on the block so that it remains at rest while the spring is elongated by `x(xge(mumg)/(k))`. Let `F_(max)` and `F_(mi n)` be the maximum and minimum values of force `F` for which the block remains in equilibrium. For a particular `x, F_(max)-F_(mi n)=2N`. Also shown is the variation of `F_(max)+F_(mi n)` versus `x`, the elongation of the spring.
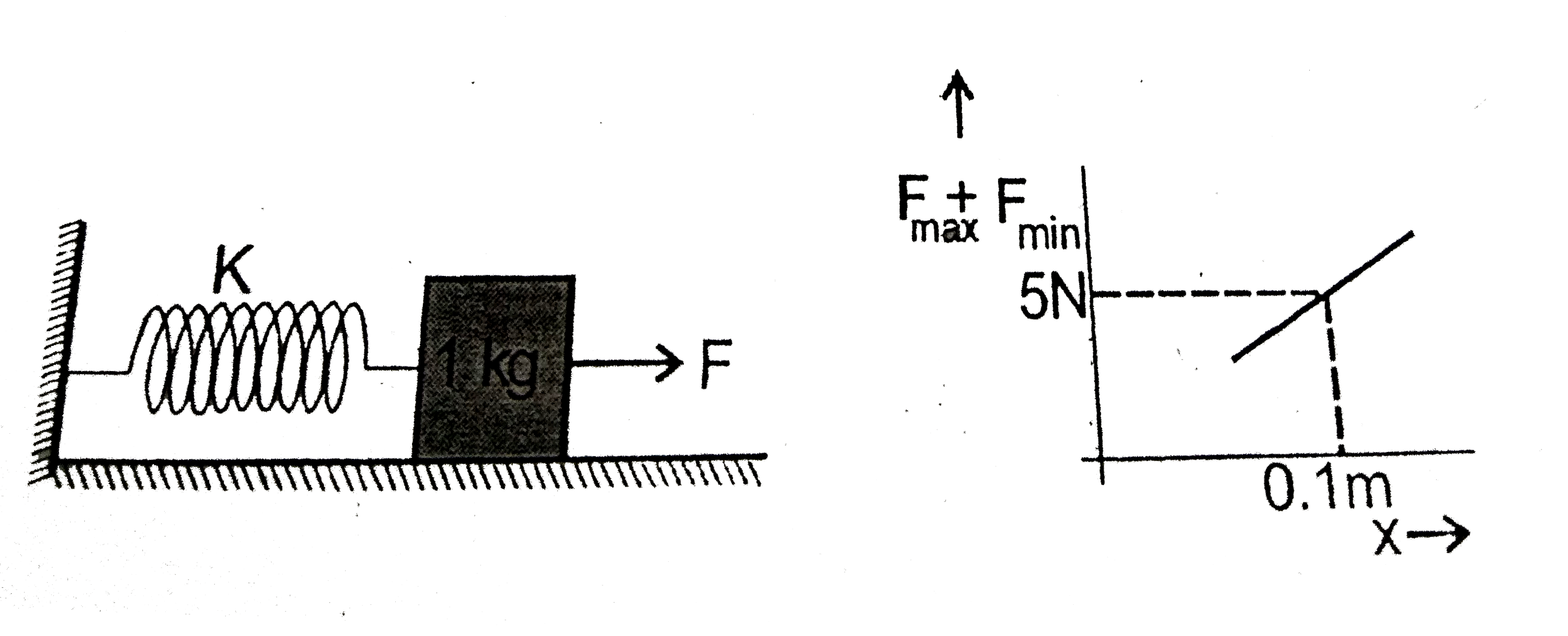
The spring constant of the spring is `:`
View SolutionA block of mass `1kg `is placed on a rough horizontal surface. A spring is attached to the block whose other end is joined to a rigid wall, as shown in the figure. A horizontal force is applied on the block so that it remains at rest while the spring is elongated by `x(x ge (mumg)/(k))`. Let `F_(max)` and `F_(mi n)` be the maximum and minimum values of force `F` for which the block remains in equilibrium. For a particular `x, F_(max)-F_(mi n)=2N`. Also shown is the variation of `F_(max)+F_(mi n)` versus `x`, the elongation of the spring.
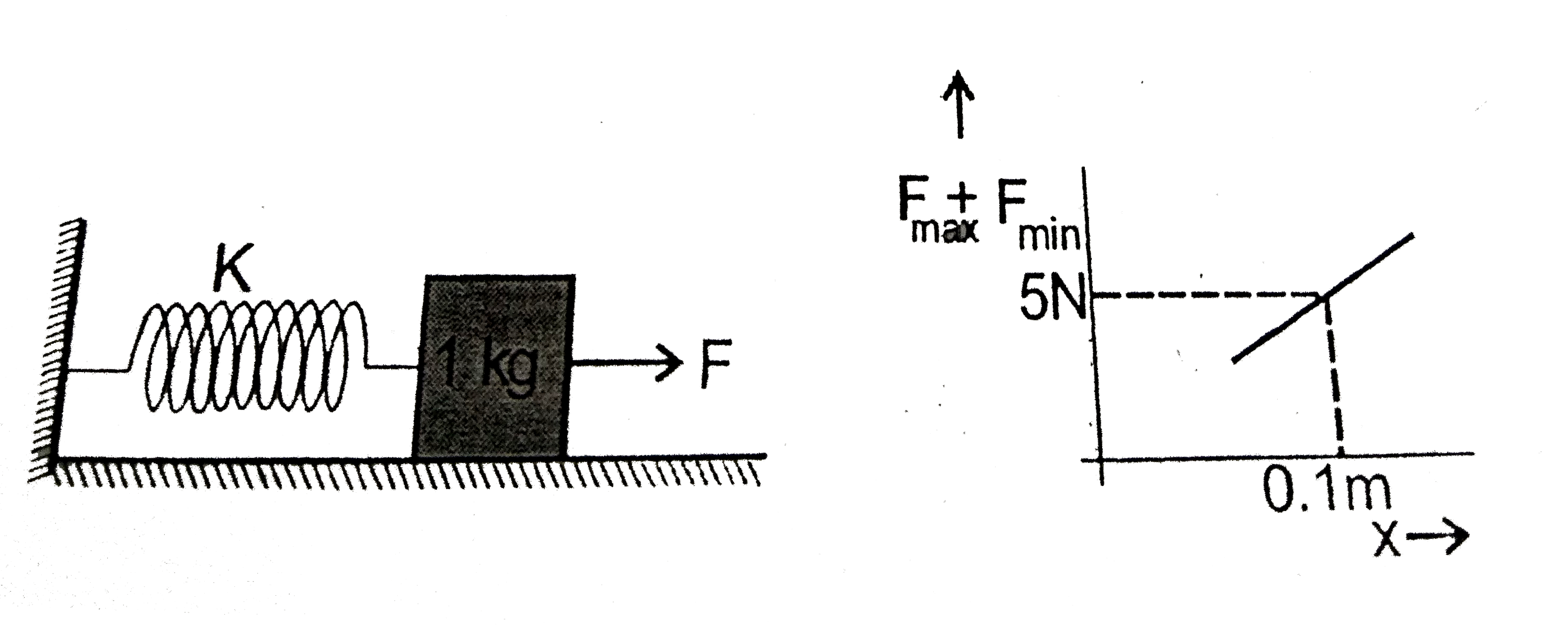
The value of `F_(mi n)`, if `x=3cm` is `:`
View SolutionA solid sphere and a hollow sphere of same mass M and same radius R are released from the top of a rough inclined plane. Friction coefficient is same for both the bodies. If both bodies perform imperfect rolling, then
Statement - 1 : Work done by friction for the motion of bodies from top of incline to the bottom will be same for both the bodies.
Statement - 2 : Force of friction will be same for both the bodies.
View SolutionStatement - 1 : Maximum value of friction force between two surfaces is `mu xx" normal reaction"`.
where `mu` = coefficient of friction between surfaces.
Statement - 2 : Friction force between surfaces of two bodies is always less than or equal to externally applied force.
View Solution
physics questions and complete solutions for chapter LAWS OF MOTION of DISHA PHYSICS (HINGLISH) of Class 12