Complete Solutions to Unsolved Numerical Problems for Preparation of NSE, INPhO & IPhO of chapter WAVES of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | WAVES |
| PHYSICS GALAXY | Unsolved Numerical Problems for Preparation of NSE, INPhO & IPhO |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
Write down the equation of a wave travelling in the negative direaction along x-axis with an amplitude `0.01 m`, a frequency `550 Hz` and a speed `330 m//s`.
View SolutionCalculate the velocity of sound in a gas in which two waves of wavelength 1 m and `1.01 m` produce `20` beats in `6` seconds.
View SolutionThe speed of sound in hydrogen is `1270 ms^(-1)` at temperature T. the speed at the same T in a mixture of oxygen and hydrogen mixed in a volume ratio 1:4 will be ?
View SolutionWhen a train is approaching the observer, the frequency of the whistle is 100 cps. When it has passed observer, it is 50 cps. Calculate the frequncy when the observer moves with the train.
View SolutionThe speed of longitudinal wave is 100 times, then the speed of transverse wave in a brass wire. What is the stress in wire ? TheYoung's modulus of brass Is `1.0 xx 10^(11)N//m^(2)`
View SolutionA copper wire is held at the two ends by rigid supports. At `30^(@)C`, the wire is just taut with negligible tension. Find the speed of transverse waves in the wire at `10^(@)C`. Given `alpha=(1.7xx10^(-5))^(@)C^(-1)`, `Y=1.4xx10^(11)Nm^(-2)` and `rho=9xx10^(3)kgm^(-3).`
View SolutionA train approaching a hill at a speed of `40 km//hr` sounds a whistle of frequency `580 Hz` when it is at a distance of `1km` from a hill. A wind with a speed of `40km//hr` is blowing in the direction of motion of the train Find
(i) the frequency of the whistle as heard by an observer on the hill,
(ii) the distance from the hill at which the echo from the hill is heard by the driver and its frequency.
(Velocity of sound in air `= 1, 200 km//hr`)
View SolutionA train approaching a hill at a speed of `40 km//hr` sounds a whistle of frequency `580 Hz` when it is at a distance of `1km` from a hill. A wind with a speed of `40km//hr` is blowing in the direction of motion of the train Find
(i) the frequency of the whistle as heard by an observer on the hill,
(ii) the distance from the hill at which the echo from the hill is heard by the driver and its frequency.
(Velocity of sound in air `= 1, 200 km//hr`)
View SolutionA wave of frequency `500 Hz` has a phase velocity of `350 m//s`. (a) How far apart are two points `60^(@)` out of phase? (b) What is the phase difference between two displacement at a certain point at times `10^(-3) s` apart?
View SolutionA wave of frequency 500 Hz has a phase velocity.of 350 m/s
(ii) What is the phase difference between two displacements at a certain point at time `10^(-3)`s apart?
View SolutionThe velocity of sound in air at `14^@ C` is `340 ms^(-1)`.What will it be when the pressure is doubled and temperature is raised to `157.5^@ C`?
View SolutionA steel wire of length `1m`, mass `0.1kg` and uniform cross-sectional area `10^(-6)m^(2)` is rigidly fixed at both ends. The temperature of the wire is lowered by `20^(@)C`. If transverse waves are set up by plucking the string in the middle.Calculate the frequency of the fundamental mode of vibration.
Given for steel `Y = 2 xx 10^(11)N//m^(2)`
`alpha = 1.21 xx 10^(-5) per ^(@)C`
View SolutionThe equation `y = A sin 2pi(500t - x//lambda)` represents a wave. Speed of wave is 360 m/s. Calculate
(a) wavelength of wave (b) distance between two points which are `pi//3` out of phase.
View SolutionWhich of the following represents (a) a progressive wave and (b) a stationary wave ?
(a)`y = 2 cos 5 x sin 9t,`
(b) `y = 2sqrt(x -vt),`
(c) `y = sin(5x - 0.5t) + 4 cos(5x - 0.5 t)`
(d) `y=cos x sint + cos 2x * sin 2t`. If progressive , and its velocity .
View SolutionThe velocity of sound in hydrogen is `1270 m s^(-1)` at `0^(@)C` and the frequency of a fork is `335 Hz`. Find the distance travelled by sound in hydrogen at `0^(@)C` and `30^(@)C` in the time in which the fork completes `71` vibrations.
View SolutionAt what temperature is the velocity of sound in nitrogen equal to its velocity in oxygen at `20^(@)` C? The atomic weights of oxygen and nitrogen are in the ratio `16 :14`
View SolutionCalculate the velocity of sound in a mixture of two gases obtained by mixing `V_(1)` and `V_(2)` volumes of them if the velocity of sound in them be `C_(1)` and `C_(2)`. The atomicity of the gases is the same.
View SolutionCalculate the velocity of sound in a mixture of two gases obtained by mixing `m_(1)` and `m_(2)` of them if the velocity of sound in them be `C_(1)` and `C_(2)`. The atomicity of the two gases is the same.
View SolutionCalculate the frequency of a ntoe emitted by a wire 20cm in length when stretched by a weight 8 kg, If 2m of the wore is found to weight 4g. Also calculate the velocity of transverse waves along the string
View SolutionIn the spectrum of light of a luminous heavenly body the wavelength of a spectral line is measured to be `4747 Å` while actual wavelength of the line is `4700 Å`. The relative velocity of the heavenly body with respect to earth will be (velocity of light is `3 xx 10^(8) m//s`)
View SolutionA wire 50cm long vibrates 100 times per second .If the length is shortened to 30 cm and the stretching force is quadrupled, what will be the frequency ?
View SolutionA sensitive microphone with its receiving surface turned towards a long vertical wall is placed at a distance of 2 m from the wall. A strong source of sound of `500 Hz` is placed between the wall and microphone on the line perpendicular to the wall and passing through the position of microphone. Find the position of the source where no sound will be heard in the microphone. (Velocity of sound in air `=350 m s^(-1)`)
View SolutionA tuning fork of frequency 341 Hz is vibrated just over a tube of length 1m. Water is being poured gradually in the tube. What height of water colume will be required for resonance?Speed of sound in air is 341 m `//` s.
View SolutionA man sets his watch by the noon-whistle of a factory at a distance of `1.5` kilometers. By how many seconds is his watch slower than the clock of the factory? (Velocity of sound in air is `332 m s^(-1)`)
View SolutionTwo tuning frok when sounded together give 4 beats per second.One is in unison with a length of 96 cm of a sonometer wire under a certain tension and the with 97 cm of the same wire under tension.Find the frequencies of the forks
View SolutionA bridges is placed under the string the of a monochord at a point near the middle and it is found that the parts produce 3 beats per second when the streching force is 8 kg.if the load be then increased to 12 kg , determene the ratio of beating of the two parts of the string
View SolutionA whistle emitting a sound of frequency `440 Hz` is tied to a string of `1.5m` length and rotated with an angular velocity of `20rad s^(-1)` in the horizontal plane. Calculate the range of frequencies heard by an observer stationed at a large distance from the whistle.
View SolutionAn under-water swimmer sends a sound signal to the surface. If it produces 5 beats per second when compared with the fundamental tone of a pipe of 20 cm length closed at one end, what is the wavelength of sound in water ? `(v_(air) = 360 m/s and v_(water) = 1500 m/s)`
View SolutionOne end of a string of length 120 cm is tied to a peg and other is attached to a weightless ring that can slide along a frictionless vertical rod fixed at a distance slightly greater then 120 cm .Find the three longest possible waveleghts
View SolutionThe minimum intensity of audibility of a source is `10^(-12) "watt"//m^(2)`. If the frequency of the note is 1000 Hz, calculate the amplitude of vibrations of air particles. Density of air `= 1.293 kg m^(-3)` and velocity of sound `=340 ms^(-1)`
`["Hint " a = sqrt((I)/(2pi^(2)n^(2)rhoC)) ]`
View SolutionA plane wave `zeta= A cos (omega t-kx)` propagates in the reference frame S. find the equation of this wave in a refreence frame S' moving in the +ve direction of x-axis with a constant velocity V relative to S.
View SolutionThe intensity of a sound wave 20 m away from the sound source is `3 xx 10^(-9) W//m^(2)`. Find the intensity of the wave 32m away from the source, if the half thickness for sound of this frequency is 120m.
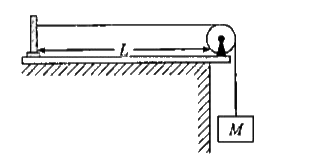
View SolutionA load of 20 kg is suspended by a steel wire as shown in figure-6.109. Velocity of wave when rubbed with a resined cloth along the length is 20 times the velocity of the wave in the same string when it is plucked. Find the area of cross - section of the wire if Y for streel is `19.6 xx 10^(10) N//m^(2)` and `g = 9.8 m//s^(2)`
View SolutionA policemen blows a whistle of frequency 330 Hz as a car speeds and passed him with avelocity 18 km per hour. Find the change in frequency as heard by the driver of the car just as he passes the police man (Velocity of sound = 320 m//s)
View SolutionA wire ofuniform cross-section is suspended vertically from a fixed point , with a load attached at the lower end. Show that the change in frequency related to the altered frequency of the wire due to rise in temperature is approx ` (1)/(2) alpha `t, Where `alpha` is the linear coefficient of expansion of the wire and `t^(@)C` is small rise in temperature.
View SolutionStanding waves are produced by superposition of two waves
`y_(1) = 0.05 sin(3pi t - 2x),`
`y_(2) = 0.05 six(3pi t +2x)`
where x and y are measured in metre and t in second. Find the amplitude of the particle at x = 0.5m.
View SolutionA ship steams towards a hill in the sea and sounds its siren and the echo is heard after 6 s. The siren is sounded again 3 minutes later after the first sounding and the echo is heard after 4 s. If the velocity of the ship is 6.87 kmph, calculate the velocity of sound in air.
View SolutionA source of sonic oscillations with frequency and a receiver are located on the same normal to the wall. Both the source and the receiver are stationary and the wall recedes from source with velocity u. Find the beat frequency registered by the receiver. The velocity of sound is equal to v.
View SolutionAn organ pipe 17 cm long open at one end radiates a tone of frequency 1.5 kHZ at temperature `16^(@)C`. What harmonic is this ? What is the fundamental frequency of these oscillations velocity of sound at `NTP = 330 m//s`.
View SolutionA string `25cm` long and having a mass of `2.5 gm` is under tension. A pipe closed at one end is `40cm` long. When the string is set vibrating in its first overtone and the air in the pipe in its fundamental frequency, `8` beats per second are heard. It is observed that decreasing the tension in the string decreases beat frequency. If the speed of sound in air is `320m//s`, find the tension in the string.
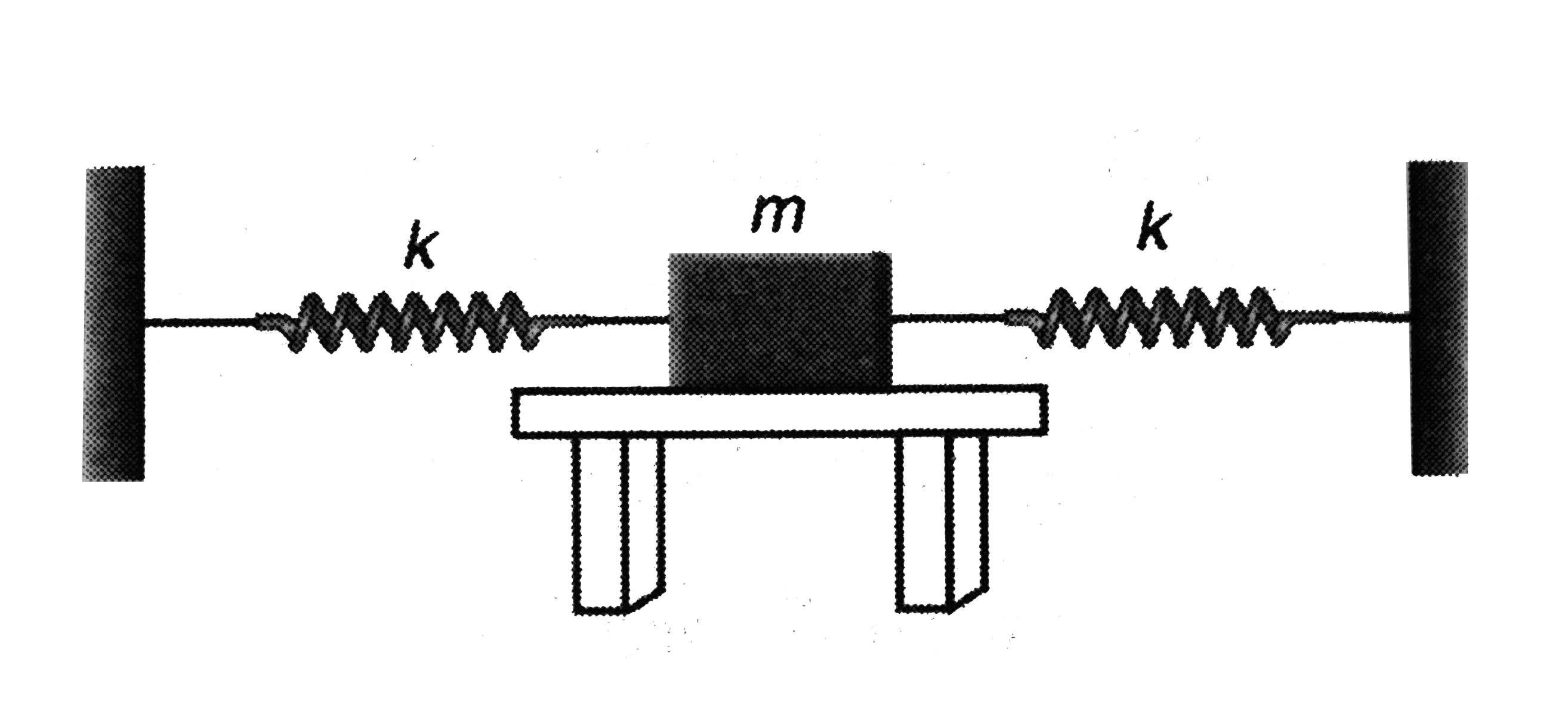
View SolutionOne end of each of two identical springs, each of force constant `0.5N//m` are attached on the opposite sides the a wooden block of mass `0.01kg`. The other ends of the spring are connected to separate rigid supports such that the springs are unstrtched and are collinear in a horizontal plane. To the wooden piece is fixed a pointer which touches a vertically moving plane paper. The wooden piece kept on a smooth horizontal table is now displaced by `0.02m` along the line of springs and released. If the speed of paper is `0.1m//s`, find the equation of the path traced by the pointer on the paper and the distance between two consecutive maximum on this path.
View SolutionA note of frequency 300 Hz has an intensity of 1 microwatt per square metre. What is the amplitude of the air vibrations caused by this sound ? (Density of air `= 1.293 kg m^(-3)` and velocity of sound in air `= 332 ms^(-1)`)
View SolutionA source of sound with natural frequency `v_(0)` moves uniformly along a straight line separated from a stationary observer by a distance l . The velocity of the source is equal to `eta` fraction of velocityof sound. Find the frequency of sound received by the observer at the moment when the source gets closest to him and also find the distance between the source and the observer at the moment, when the observer receives a frequency `v = v_(0)`
View SolutionA wire when stretched by the weight of a solid,' gives a fundamental )frequaicy `v_(1)` when the solid is immersed in water it gives a frequency v, and when immersed in liquid it gives a frequency of `v_(2)` Calculate the specific gravityofthe solidand that ofthe liquid.
View SolutionTwo wires of different mass densities are soldered together end to end and are then stretched undera tension F (the tension is same in both the wires). The way e speed in the second wire is three times that in the first wire. When a harmonic wave is travelling in the first wire, it is reflected at thejunction of the wires, the reflected wave has half the am plitude of the incident wave
(a) If the amplitude of incident wave is A, what are the amplitudes of the reflected and transmitted waves ?
(b) Assuming no loss in wire, what fiaction of the incident power is reflected at thejunction and what fraction is transmitted ?
(c) Show that the displacement just to the left of the junction equals that just to the right ofthe junction.
View SolutionCalculate the velocity of sound in a mixture of oxygen, nitrogen and argon at `0^(@)` when their masses are in the ratio 2:7: 1. The molecular weights of gases are 32, 2S and 40 respectively.
View SolutionA non-uniform wire of length `l` and mass `M` has a variable linear mass density given by `mu = kx`, where `x` is distance from one end of wire and `k` is a constant. Find the time taken by a pulse starting at one end to reach the other end when the tension in the wire is `T`.
View SolutionMicrowaves which travel with the speed of light are reflected from a distant aeroplane approaching the waye source radar. It is found that when the reflected waves are beat against• the wave sradiated from the source, the beat frequency is 990 Hz. If the micro waves are 0.1 m in wavelength, what is the approaching speed of the aeroplane.
View SolutionA man walks towards a cliff while beating a drum at the rate of 5 beats per second till the echo of beating disappears completely. He walks at the rate of 8 kilometres per hour. Calculate the distance of the man from the cliff in the beginning if he walked for 5 mintes. (Velocity of sound in air `=350 m s^(-1)` )
View SolutionA tunnel leading straight through a hill greatly amplifies tones at 135 and 138 Hz. Find the shortest length of the tunnel if velocity of sound in air is 330 m/s.
View SolutionA smoked plate falls vertically under gravity. A tuning fork traces wave on it. It is found that the lengths of two conscutive grounps of 10 waves are 5.143 and 6.64 cm respectively. What is the frequecny of the fork ?
View SolutionA sonometer wire under tension of 64 N vibrating in its fundamental mode is in resonance with a vibrating tuning fork. The vibrating portion of the sonometer wire has a length of 10 cm and mass of 1 kg. The vibrating tuning fork is now moved away from the vibrating wire with a constant speed and an observer standing near the sonometer hears one beat per second. Calculate the speed with which the tuning fork is moved, if the speed of sound in air is 300 m/s.
View SolutionSources Separated by 20 m vibrate according to equations `y_(1) = 0.06 sin pi t` metre and `y_(2) = 0.02 sin pi t` metre. They send out waves along a rod at speed 3 m/s. What is the equation of motion of a particle 12 m from the first source and 8 metre from the second ?
View SolutionA wire of density `9000 kg//m^(3)` is stretched between two clamps `100 cm` apart while subjected to an extension od `0.05 cm`. What is the lowest frequency of transverse vibrations in the wire , assuming Young's modulus of the material to be `9 xx 10^(10) N//m^(2)`?
View SolutionOrdinary cotton thread, 200cm of which weight 1g, is used in Melde's experiment It is attached at one to a vibrator of frequency 100 Hz and at the order to a pan weights 6g.What length of the srting will vibrate in 4 loops in the longitudinal arrangement if 10g weight is put on the pan ?
View SolutionA heavy ball is suspended from the ceiling of a motor car through a light string. A transverse pulse travels at a speed of `60 cm s^-1` on the string when the car is at rest and `62 cm s^-1` when the car accelerates on a horizontal road. Find the acceleration of the car. Take `g = 10 ms^-2`
View SolutionThe difference between the apparent frequency of a source of sound as perceived by an observer during its approach and recession is 2% of the natural frequency of the source. Then the speed of the source will be
View SolutionTwo wires are kept tight between the same pair of supports. The tensions in the wires are in the ratio 2 : 1, the radii are in the ratio 3 : 1 and the densities are in the ratio 1 : 2. Find the ratio of their fundamental frequencies.
View SolutionThree metal rods are located relative to each other shown in figure - 6.101, where `L_(1) + L_(2) = L_(3)` Values of density and Young's modulus of the three materials are:
`(rho_(1)) = 2.7 xx 10^(3)kg//m^(3)` `y_(1) = 7 xx 10^(10)Pa,`
`(rho_(1)) = 11.3 xx 10^(3)kg//m^(3)` `Y_(2) = 1.6 xx 10^(10) pa,`
`(rho_(1)) = 8.8 xx 10^(3)kg//m^(3)` `Y_(3) = 11 xx 10^(10) pa` If `L_(3) = 1.5m` What musht the ratio `L_(1)//L_(_(2))` be if a sound wave is to travel the length of rods 1 and 2 in the same time as required to travel the length of rod 3 ?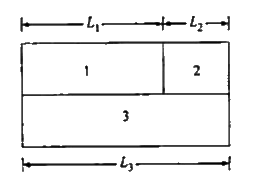
View SolutionThree sound waves of frequencies `320, 344` and `280` are produced simultaneously. Find the number of beats per second, assuming the human ear's resolution is 10 beats per second.
View SolutionA source of sound with frequency `256 Hz` is moving with a velocity `V` towards a wall and an observer is stationary between the source and the wall. When the observer is between the source and the wall he will hear beats.
View SolutionA siren emitting a sound of frequency `1000 H_(Z)` moves away from you towards a cliff at a speed of `10 m//s` .
(a) What is the frequency of the sound you hear coming directly from the sirven ?
(b) What is the frequency of sounds you hear reflected off the cliffb ?
(c) What beat frequency would you hear ? Take the speed of sound in air as `330 m//s` .
View Solution`S_(1)` and `S_(2)` are two loudspeakers with the same frequency of 165 Hz and acoustic output `1.2 xx 10 ^(-3)` and `1.8 xx 10^(-3)` watts respectively/ They vibrate in the same phase. P is a point at a distance 4m from `S_(1)` and 3m from `S_(2)`
How are the phase of the two waves arriving at P related?
View Solution`S_(1)` and `S_(2)` are two loudspeakers with the same frequency of 165 Hz and acoustic output `1.2 xx 10 ^(-3)` and `1.8 xx 10^(-3)` watts respectively/ They vibrate in the same phase. P is a point at a distance 4m from `S_(1)` and 3m from `S_(2)`
What is the intensity of sound at P if `S_(1)` is turned off `(S_(2))` on)
View Solution`S_(1)` and `S_(2)` are two loudspeakers with the same frequency of 165 Hz and acoustic output `1.2 xx 10 ^(-3)` and `1.8 xx 10^(-3)` watts respectively/ They vibrate in the same phase. P is a point at a distance 4m from `S_(1)` and 3m from `S_(2)`
What is the intensity of sound at P if `S_(2)` is turned off?
View Solution`S_(1)` and `S_(2)` are two loudspeakers with the same frequency of 165 Hz and acoustic output `1.2 xx 10 ^(-3)` and `1.8 xx 10^(-3)` watts respectively/ They vibrate in the same phase. P is a point at a distance 4m from `S_(1)` and 3m from `S_(2)`
What is the intensity at P with `S_(1)` and `S_(2)` on?
View SolutionA stretched sonometer wire gives 2 beats per second with a tuning frok when its length is 14.3 cm and also when its length is 14.5 cm.What is the frequency of the tuning frok ?
View SolutionThe sounding rod of a dust tube apparatus is made of brass and is 160 cm long. The distance between adjacent nodes in the wave tube was 11.35 cm. Calculate the Young's modulus of the rod assuming that velocity of sound in air at room temperature is `350 ms^(-1)` and density o fbrass 900 kg m^(-3)`
View SolutionIf at `t = 0`, a travelling wave pulse on a string is described by the function.
`y = (6)/(x^(2) + 3)`
What will be the waves function representing the pulse at time `t`, if the pulse is propagating along positive x-axis with speed `4m//s`?
View SolutionA receiver and a source of sonic oscillation of frequency n = 2000 Hz are located on the x - axis. The source swings harmonically along that axis with a circular frequency `omega` and an amplitude a = 50 cm. At what value of `omega` will the frequency band width registered by the stationary receiver be equal to `Deltan = 200 Hz? The velocity of sound is equal to v = 340 m/s
View SolutionCalculate the velocity of sound in air saturated with moisture at `25^(@)C` and `745` mm pressure. The saturation pressure at `25^(@)C` is `23.76 mm` of mercury and the velocity of sound at `0^(@)C` in dry is `332 m s^(-1)`.
[Hint: See example 4.]
View SolutionTwo long strings A and B, each having linear mass density `1.2 xx 10 ^-2 kg m^-1`, are stretched by different tensions 4.8 N and 7.5 N respectively and are kept parallel to each other with their left ends at x = 0. Wave pulses are produced on the strings at the left ends at t = 0 on string A and at t = 20 ms on string B. When and where will the pulse on B overtake that on A ?
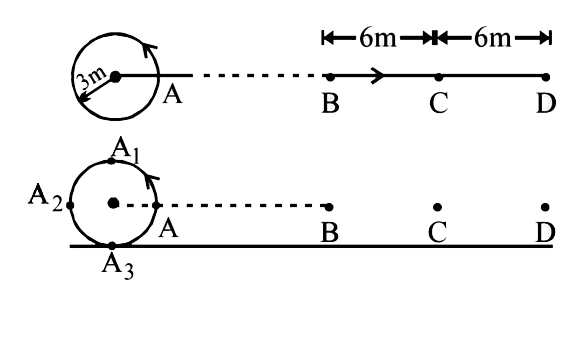
View SolutionA source of sound is moving along a circular orbit of radius `3 meter` with an angular velocity of `10 rad//s`. A sound detector located far away from the source is executing linear simple harmonic motion along the line `BD` with an amplitude `BC = CD = 6 meters`. The frequency of oscillation of the detector is `(5)/(pi)` per second. The source is at the point `A` when the detector is at the point `B`. If the source emits a continuous sound wave of frequency `340 Hz`, Find the maximum and the minimum frequencies recorded by the detector.
View SolutionA vibrator makes 150 cm of a arting vibrate in 6 loops in the longitudinal arrangement when it is stretched by 15 g. The entire length of the string is then weighed and is found to weigh 500mg.What is the frequency of the vibrator ? Wjat is the sidtance beween two nodes?
View SolutionFind the radius vector defining the position of a point source of spherical waves if that source is known to be located on the straight line between the points with radius vector `r_(1)` and `r_(2)` at which the oscillation amplitudes of particles of the medium are equal to `a_(1)` and `a_(2)`. The damping of the wave is negligible, the medium is homogeneous.
View SolutionA sonometer wire of length `1.5m` is made of steel. The tension in it produces an elastic strain of `1%`. What is the fundamental frequency of steel if density and elasticity of steel are `7.7 xx 10^(3) kg//m^(3)` and `2.2 xx 10^(11) N//m^(2)` respectively ?
View SolutionFifty-six tuning forks are arranged in order of increasing frequencies so that each fork gives 4 beats per second with the next one. The last fork gives the octave of the first. Find the frequency of the first.
View SolutionTwo plane sonic waves of same frequency are travelling in a homogeneous medium. Loudness recorded by a moving detector in the medium varies from `L_(1) = 30 db " to" L_(2) = 43 . 9794 ` dB . Calculate intensity of the two waves.
Give `log_(1) 2 = 0 . 30103 `
View SolutionA column of air and a tuning fork produce 4 beats per second when sounded together. The tuning fork gives the lower note. The temperature of air is `15^(@)C`. When the temperature falls to `10^(@)C`, the two produce 3 beats per second. Find the frequency of the fork.
View SolutionA source of sonic oscillations with frequency `v_(0)=100Hz` moves at right angles to the wall with a velocity `u=0.17 m//s`. Two stationary receivers `R_(1)` and `R_(2)` are located on a straight line, coinciding with the trajectory of the source , in the following succession :` R_(1)-` source `-R_(2)-` wall. Which receiver registers the beatings and what is the beat frequency ? The velocity of sound is equal to `upsilon= 340 m//s`.
View SolutionA `200 Hz` wave with amplitude `1 mm` travels on a long string of linear mass density `6 g//m` keep under a tension of `60 N`.
(a) Find the average power transmitted across a given point on the string.
(b) Find the total energy associated with the wave in a `2.0 m` long portion of the string.
View SolutionA `200 Hz` wave with amplitude `1 mm` travels on a long string of linear mass density `6 g//m` keep under a tension of `60 N`.
(a) Find the average power transmitted across a given point on the string.
(b) Find the total energy associated with the wave in a `2.0 m` long portion of the string.
View SolutionThe temperature of air varies with height linearly from `T_(1)` at the earth's surface to `T_(2)` at a height h. Calculate the time t needed for a sound wave produced at a heights to reach the earth's surface. The velocity ofsound near the earth's surface is C.
View SolutionThe shape of a wave propagating in the positive x or negative x-direction is given `y=1/sqrt(1+x^(2))` at t=0 and `y=1/sqrt(2-2x+x^(2))` at t=1s where x and y are in meters the shape the wave disturbance does not change during propagation find the velocity of the wave
View SolutionA wire of density `9000 kg//m^(3)` is stretched between two clamps `100 cm` apart while subjected to an extension od `0.05 cm`. What is the lowest frequency of transverse vibrations in the wire , assuming Young's modulus of the material to be `9 xx 10^(10) N//m^(2)`?
View SolutionThe following equations represent transverse waves :
`z_(1) = A cos(kx - omegat)`,
`z_(2) = A cos (kx + omegat)`, `z_(3) = A cos (ky - omegat)`
Identify the combination (s) of the waves which will produce (i) standing wave(s), (ii) a wave travelling in the direction making an angle of `45^(@)` with the positive `x` and positive `y` axes. In each case, find the positions at which the resultant is always zero.
View SolutionA wire of length l is kept just taut horizontally between two walls.A mass m hanging from its mid point depresses it by `delta`.Calculte the time in which a pulse set up at one end will reach the other end . The mass of the wire per unit length of it is `mu`
View SolutionWavelength of two notes in ai rare `80//195` m and `80//193` m. Each note produces five beats per second with a third note of a fixed frequency. Calculate the velocity of sound in air.
View SolutionA fork and a monochord string 100 cm long give 4 beats per second.The string is made shorter without any change of tension untill it is in unsion the frok.If its length is now 99 cm, what is the frequency if the frok ?
View SolutionA 40 cm wire having a mass of 3.2 g is stretched between two fixed supports 40.05 cm apart. In its fundamental mode, the wire vibrates at 220 Hz. If the area of cross section of the wire is `1.0 mm^2`, find its Young modulus.
View SolutionA stationary observer receives sonic oscillations from two tuning forks one of which approaches and the other recedes with the same velocity. As this takes place, the observer hears the beats of frequency `f = 2.0 H_(Z)`. Find the velocity of each tuning fork if their oscillation frequency is `f_(o) = 680 H_(Z)` and the velocity of sound in air is `upsilon = 340 m//s`.
View SolutionA sonometer wire under tension of 64 N vibrating in its fundamental mode is in resonance with a vibrating tuning fork. The vibrating portion of the sonometer wire has a length of 10 cm and mass of 1 kg. The vibrating tuning fork is now moved away from the vibrating wire with a constant speed and an observer standing near the sonometer hears one beat per second. Calculate the speed with which the tuning fork is moved, if the speed of sound in air is 300 m/s.
View Solutionthe linear density of a wire under tension t varies linearly from `mu_(1)` to `mu_(20`.Calculate the time that a pulse would need to pass one to the other.The length of the wire is l
View SolutionIn a sonometer wire, the tension is maintained by suspending a 50.7 kg mass from the free end of the wire. The suspended mass has a volume of 0.0075 m 3. The fundamental frequency of the wire is 260 Hz . If the suspended mass is completely submerged in water, the fundamental frequency will become (take `g = 10 ms^(-2)`) [
View SolutionCalculate the velocity of sound in air on a day when temperature is `30^(@)C`, pressure `0.74 m` of mercury and relative humidity `60%`. Velocity of sound at NTP `=330 m s^(-1)`. Saturated vapour pressure at `30^(@)C=0.032 m` of mercury.
`[["Hint: Relative Humidity "=("Vapour pressure at "30^(@)C)/("Saturated vapour pressure at "30^(@)C)],[C_(m)=C_(d) sqrt(P/(P-0.385f))]]`
View SolutionIf a loop of chain is spunat high speed, it will roll like a hoop without collapsing. Consider a chain oflinear mass density p that `mu` is rollingwithoutslipping at a high speed `v_(0)`
(a) Show that the tension in the chain is `F = muv_(0)^(2)`
(b) If the chain rolls over a small hump, a transverse wave pulse will be generated in the chain. At whatspeedwill it travel alongthe chain ?
(c)How far around the loop (in degrees) will a transverse pulse travelin the time the hoop rolls through one complete revolution ?
View SolutionIn a pipe closed at both ends the maximum amplitude of vibration is 5 mm and the amplitude of vibration at a distance 5 cm from one end is 4.33 mm. The length of the pipe is 120cm. To what mode of vibration does it correspond ? What is the frequency of the note emitted by the pipe ? Velocity of sound in the gas enclosed in the pipe 336 m/s.
View SolutionA 2.00 m-long rope, having a mass of 80 g, is fixed at one end and is tied to a light string at the other end. The tension in the string is 256 N. (a) Find the frequencies of the fundamental and the first two overtones. (b) Find the wavelength in the fundamental and the first two overtones.
View SolutionCalculate the velocity of sound in a medium where change in pressure and volume takes place according to the law `p=alpha/V^(2)` where `alpha` is a constant. Treat the medium as an ideal gas and assume `rho` as its normal density.
View SolutionA 2 m string is fixed at one end and is vibrating in its third harmonic with amplitude 3 cm and frequency 100Hz
(a) Write an expression for the kinetic energy of a segment of a segment of the string of length dx at a point x at some time l. At what time is its kinetic energy maximum? What is the shape of the string at this time?
Find the maximum kinedic energy of the string by intergrating your expression for part (a) over the total length of the string.
View Solution
Unsolved Numerical Problems for Preparation of NSE, INPhO & IPhO questions and complete solutions for chapter WAVES of GK PUBLICATIONS PHYSICS (HINGLISH) of Class 12
All Exercises from PHYSICS GALAXY Class 12 PHYSICS WAVES
- Advance MCQs with One or More Options Correct
- Conceptual MCQs Single Option Correct
- Discussion Question
- Illustrative
- Numerical MCQs Single Options Correct
- Practice Exercise 6.1
- Practice Exercise 6.2
- Practice Exercise 6.3
- Practice Exercise 6.4
- Practice Exercise 6.5
- Practice Exercise 6.6
- Practice Exercise 6.7
- Practice Exercise 6.8
- Unsolved Numerical Problems for Preparation of NSE, INPhO & IPhO