Complete Solutions to EXAMPLE of chapter CIRCLE of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | CIRCLE |
| SELINA PUBLICATION | EXAMPLE |
| Number Of Questions | 15 |
| Academic Year | 2023-24 |
In the figure, given alongside, CD is a diameter which meets the chord AB at E, such that AE = BE = 4 cm. If CE is 3 cm, find the radius of the circle.
View Solutionएक आयत की लम्बाई x, 5 cm / min की दर से घट रही है और चौड़ाई y, 4 cm / min कि दर से बढ़ रही है जब x=8 cm और y= 6 cm है तब आयत के (a) परिमाप (b) क्षेत्रफल की परिवर्तन की दर ज्ञात कीजिए
View SolutionChords AB and CD of a circle are parallel to each other and lie on opposite sides of the centre of the circle. If AB = 36 cm, CD = 48 cm and the distance between the chords is 42 cm, find the radius of the circle.
View SolutionChords AB and CD of a circle with centre O, intersect at a point E. If OE bisects angle AED, prove that chord AB = chord CD.
View SolutionBC is an equilateral triangle. A circle is drawn with centre A so that it cuts AB and AC at points M and N respectively. Prove that BN = CM
View SolutionProve that the line joining the mid-points of two parallel chords of a circle passes through the centre.
View SolutionAB and CD are two equal chords of a circle with centre O. If AB and CD, on being produced, meet at a point P outside the circle, prove that :

PA = PC
View SolutionAB and CD are two equal chords of a circle with centre O. If AB and CD, on being produced, meet at a point P outside the circle, prove that :
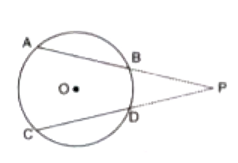
PB = PD
View SolutionTwo circles with centres A and B intersect each other at points P and Q. Prove that the centre-line AB bisects the common chord PQ perpendicularly.
View SolutionTwo circles with centres O and O' intersect each other at points P and Q. The straight line APB is parallel to centre-line OO'. Prove that : OO' =`1/2` AB

View SolutionOut of two unequal chords of a circle, the bigger chord is closer to the centre of the circle. Prove it.
View SolutionThree boys A, B and C are standing on the circumference of a circle with radius 10 cm and centre at point O. If the distance between A and B = distance between B and C = 12 cm, find the distance between A and C.

View SolutionIn the given figure, O is the centre of a circle, AB is a side of regular octagon and AC is a side of regular hexagon. Find :

`angleAOB`
View SolutionIn the given figure, O is the centre of a circle, AB is a side of regular octagon and AC is a side of regular hexagon. Find :

`angleAOC`
View SolutionIn the given figure, O is the centre of a circle, AB is a side of regular octagon and AC is a side of regular hexagon. Find :

`angleBOC`.
View Solution
EXAMPLE questions and complete solutions for chapter CIRCLE of ICSE of Class 9