Complete Solutions to EXERCISE 17(C) of chapter CIRCLE of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | CIRCLE |
| SELINA PUBLICATION | EXERCISE 17(C) |
| Number Of Questions | 29 |
| Academic Year | 2023-24 |
In the given figure, an equilateral triangle ABC is inscribed in a circle with centre O. Find :
`angleBOC`
View SolutionIn the given figure, an equilateral triangle ABC is inscribed in a circle with centre O. Find :
`angleOBC`
View SolutionIn the given figure, a square is inscribed in a circle with centre O. Find :
`angleBOC` . Is BD a diameter of the circle
View SolutionIn the given figure, a square is inscribed in a circle with centre O. Find :
`angleOCB` . Is BD a diameter of the circle
View SolutionIn the given figure, a square is inscribed in a circle with centre O. Find :
`angleCOD` . Is BD a diameter of the circle
View SolutionIn the given figure, a square is inscribed in a circle with centre O. Find :
`angleBOD` .Is BD a diameter of the circle
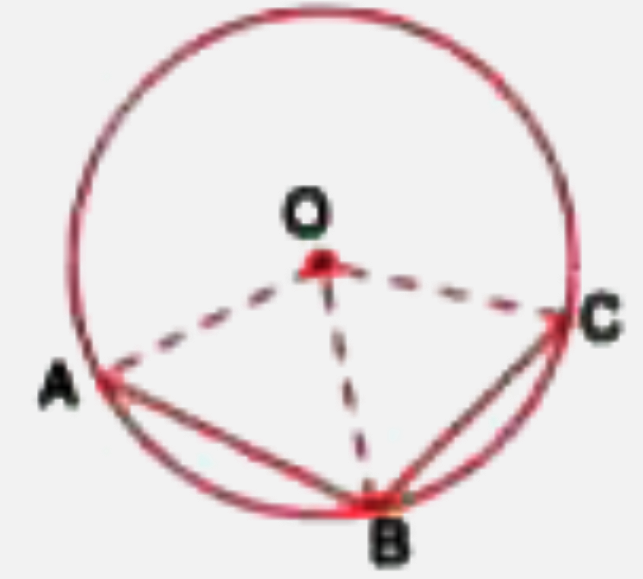
View SolutionIn the given figure, AB is a side of regular pentagon and BC is a side of regula hexagon.
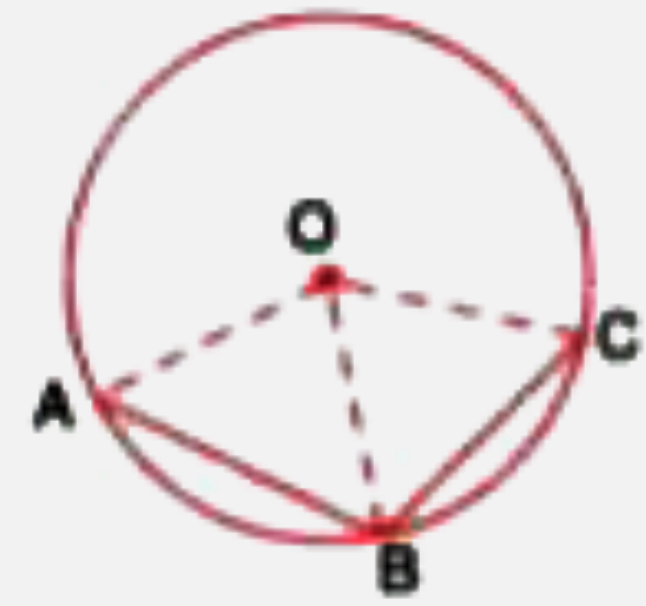
`angleAOB`
View SolutionIn the given figure, AB is a side of regular pentagon and BC is a side of regula hexagon.

`angleBOC`
View SolutionIn the given figure, AB is a side of regular pentagon and BC is a side of regular hexagon.
`angleAOC`
View SolutionIn the given figure, AB is a side of regular pentagon and BC is a side of regula hexagon.
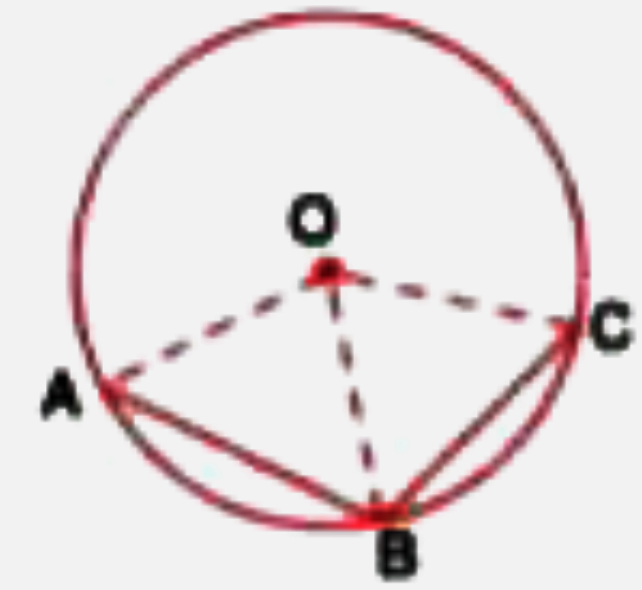
`angleOBA`
View SolutionIn the given figure, AB is a side of regular pentagon and BC is a side of regula hexagon.

`angleOBC`
View SolutionIn the given figure, AB is a side of regular pentagon and BC is a side of regula hexagon.

`angleABC`
View SolutionIn the given figure, arc AB and arc BC are equal in length. If `angleAOB = 48^(@)`, find

`angleBOC`
View SolutionIn the given figure, arc AB and arc BC are equal in length. If `angleAOB = 48^(@)`, find

`angleOBC`
View SolutionIn the given figure, arc AB and arc BC are equal in length. If `angleAOB = 48^(@)`, find

`angleAOC`
View SolutionIn the given figure, arc AB and arc BC are equal in length. If `angleAOB = 48^(@)`, find

`angleOAC`
View SolutionIn the given figure, the lengths of arcs AB and BC are in the ratio 3 : 2. If `angleAOB = 96^(@)`, find:

`angleBOC`
View SolutionIn the given figure, the lengths of arcs AB and BC are in the ratio 3 : 2. If `angleAOB = 96^(@)`, find:

`angleABC`
View SolutionIn the given figure, AB = BC = DC and `angleAOB = 50^(@)`
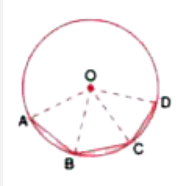
`angleAOC` =?
View SolutionIn the given figure, AB = BC = DC and `angleAOB = 50^(@)`

`angleAOD`
View SolutionIn the given figure, AB = BC = DC and `angleAOB = 50^(@)`

`angleBOD`
View SolutionIn the given figure, AB = BC = DC and `angleAOB = 50^(@)`

`angleOAC`
View SolutionIn the given figure, AB = BC = DC and `angleAOB = 50^(@)`

`angleODA`
View SolutionIn the given figure, AB is a side of a regular hexagon and AC is a side of a regular eight sided polygon. Find :
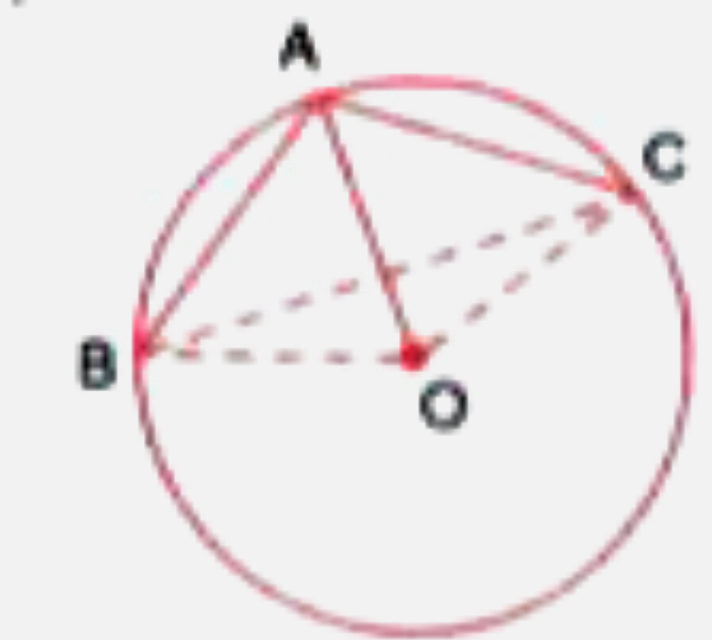
`angleAOB`
View SolutionIn the given figure, AB is a side of a regular hexagon and AC is a side of a regular eight sided polygon. Find :

`angleAOC`
View SolutionIn the given figure, AB is a side of a regular hexagon and AC is a side of a regular eight sided polygon. Find :

`angleBOC`
View SolutionIn the given figure, AB is a side of a regular hexagon and AC is a side of a regular eight sided polygon. Find :

`angleOBC`
View SolutionIn the given figure, O is the centre of the circle and the length of arc AB is twice the length of arc BC.
if `angleAOB =100^(@)` find `angleBOC`
View SolutionIn the given figure, O is the centre of the circle and the length of arc AB is twice the length of arc BC.
if `angleAOB =100^(@)` find

`angleOAC`
View Solution
EXERCISE 17(C) questions and complete solutions for chapter CIRCLE of ICSE of Class 9