Complete Solutions to EXERCISE 17(B) of chapter CIRCLE of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | CIRCLE |
| SELINA PUBLICATION | EXERCISE 17(B) |
| Number Of Questions | 15 |
| Academic Year | 2023-24 |
The figure shows two concentric circles and AD is a chord of larger circle.
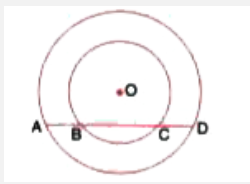
Prove that : AB = CD.
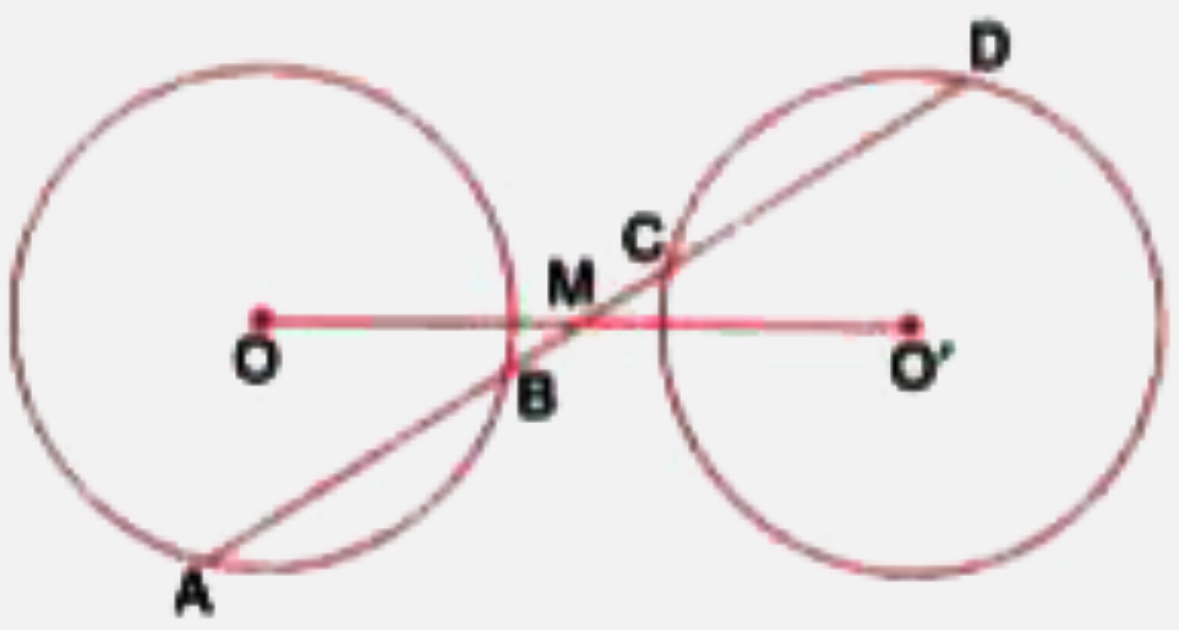
View SolutionA straight line is drawn cutting two equal circles and passing through the mid-point M of the line joining their centres O and O'.
 >
>
Prove that the chords AB and CD, which are intercepted by the two circles, are equal.
View SolutionM and N are the mid-points of two equal chords AB and CD respectively of a circle with centre O. Prove that :
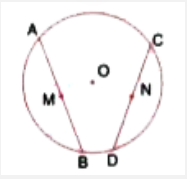
(i) `angleBMN = angleDNM`
View SolutionM and N are the mid-points of two equal chords AB and CD respectively of a circle with centre O. Prove that :
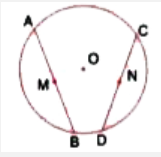
`angleAMN = angleCNM`.
View SolutionIn the following figure, P and Q are the points of intersection of two circles with centres O and O'. If straight lines APB and CQD are parallel to OO', prove that
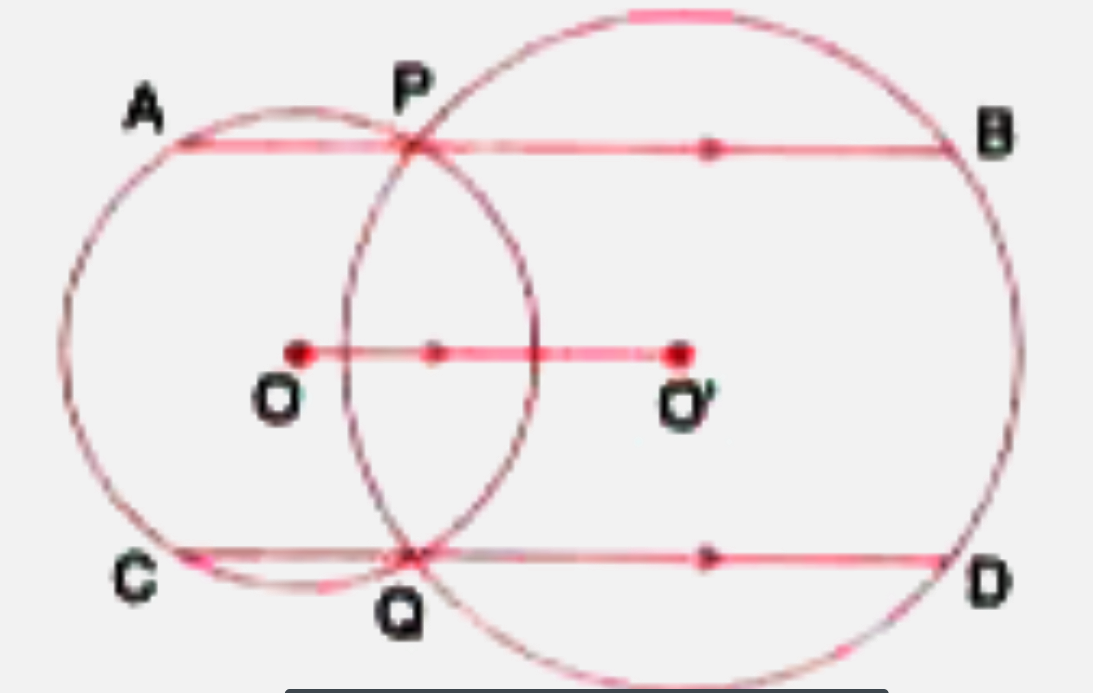
OO'=`1/2AB`=`1/2CD`
View SolutionIn the following figure, P and Q are the points of intersection of two circles with centres O and O'. If straight lines APB and CQD are parallel to OO', prove that

AB=CD
View SolutionTwo equal chords AB and CD of a circle with centre O, intersect each other at point P inside the circle. Prove that

AP=CP
View SolutionTwo equal chords AB and CD of a circle with centre O, intersect each other at point P inside the circle. Prove that

BP=DP
View SolutionIn the following figure, OABC is a square. A circle is drawn with O as centre which meets OC at P and OA at Q. Prove that :

`triangle OPA = triangle OQC`,
View SolutionIn the following figure, OABC is a square. A circle is drawn with O as centre which meets OC at P and OA at Q. Prove that :

`triangle BPC = triangle BQA`.
View SolutionThe length of common chord of two intersecting circles is 30 cm. If the diameters of these two circles be 50 cm and 34 cm, calculate the distance between their centres.
View SolutionThe line joining the mid-points of two chords of a circle passes through its centre. Prove that the chords are parallel.
View SolutionIn the following figure, the line ABCD is perpendicular to PQ, where P and Q are the centres of the circles. Show that :

AB=CD
View SolutionIn the following figure, the line ABCD is perpendicular to PQ, where P and Q are the centres of the circles. Show that :
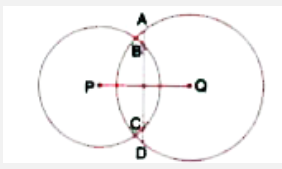
AC=BD
View SolutionAB and CD are two equal chords of a circle with centre O which intersect each other at right angle at point P. If OM `bot` AB and ON `bot` CD, show that OMPN is a square.
View Solution
EXERCISE 17(B) questions and complete solutions for chapter CIRCLE of ICSE of Class 9