Complete Solutions to Introduction To Euclid Geometry of chapter INTRODUCTION TO EUCLID GEOMETRY of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | INTRODUCTION TO EUCLID GEOMETRY |
| NCERT EXEMPLAR | Introduction To Euclid Geometry |
| Number Of Questions | 48 |
| Academic Year | 2023-24 |
The three steps from solids to point are
View SolutionThe number of dimension ,a solid has
View Solutionthe number of dimension,a surface has
View SolutionThe number of dimension,a point has
View SolutionEuclid divided his famous treatise 'The Element' into
View SolutionThe total number of propositions in 'The Element' are
View SolutionBoundaries of solids are
View SolutionBoundaries of surfaces are curves.
View SolutionIn Indus valley Civilisation (about 3000 BC), the bricks used for construction work were having dimensions in the ratio
View SolutionA pyramid is a solids figure, the base of which is
View SolutionThe side faces of a pyarmid are
View SolutionIt is known that if `x+y=10`, then `x+y+z=10+z`. The Euclid's axiom that illustrates this statement is
View SolutionIn ancient India, the shapes of altars used for household rituals were
View SolutionThe number of interwoven isosceles triangles in Sriyantra (in the Atharvaveda) is
View SolutionGreek's emphasised on
View SolutionIn ancient India, altars with combination of shapes like rectangles, triangles and trapeziums were used for
View SolutionEuclid belongs to the country
View SolutionThales belongs to the country
View SolutionPythagoras was a student of
View SolutionWhich of the following needs a proof ?
View SolutionEuclid stated that all right angles are equal to each other in the form of
View SolutionLines are parallel, if they do not intersect' is stated in the form of
View SolutionEuclidean geometry is valid only for curved surfaces.
View SolutionThe boundaries of the solids are curves.
View SolutionThe edges of a surface are curves.
View SolutionThe things which are double of the same thing are equal to one another.
View SolutionIf a quantity B is a part of another quantity A, then A can be written as the sum of B and some third quanrity C.
View SolutionState True or False
The statements that are proved are called axioms.
View Solution"For every line L and for every point P not lying on a given line L, there exists a unique line m passing through P and parallel to L" is known as playfair 's axiom.
View SolutionTwo distinct intersecting lines cannot be parallel to the same line.
View SolutionAttempt to prove Euclid 's fifth postulate using the other postulates and axioms led to the discovery of several other geometries.
View SolutionTwo salesmen make equal sales during the month of August. In September, each salesman doubles his sale of the month of August. Compare their sales in September.
View SolutionIt is known that `x+y=10` and that `x=z`. Show that `z+y=10`.
View SolutionLook at the adjoining figure. Show that length AH gt sum of lengths of AB + BC + CD
View SolutionIn the adjoining figure, if AB = BC and BX =BY, then show that AX=CY
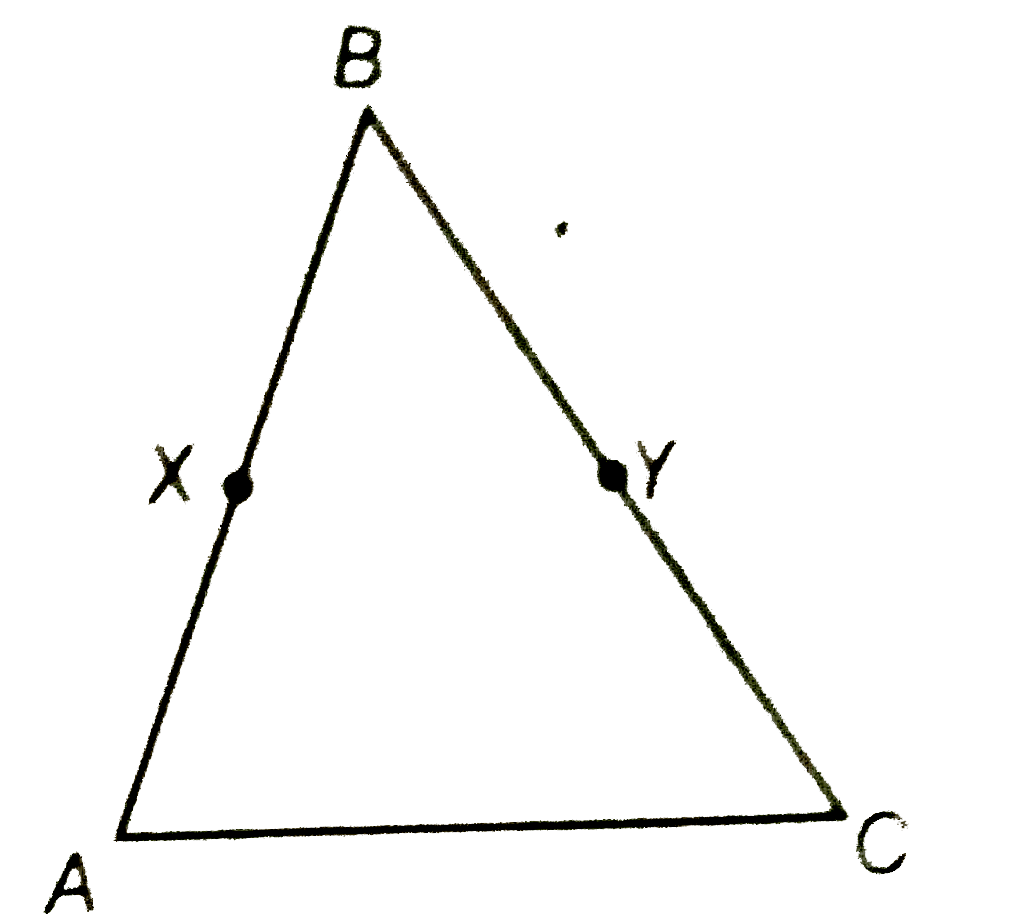
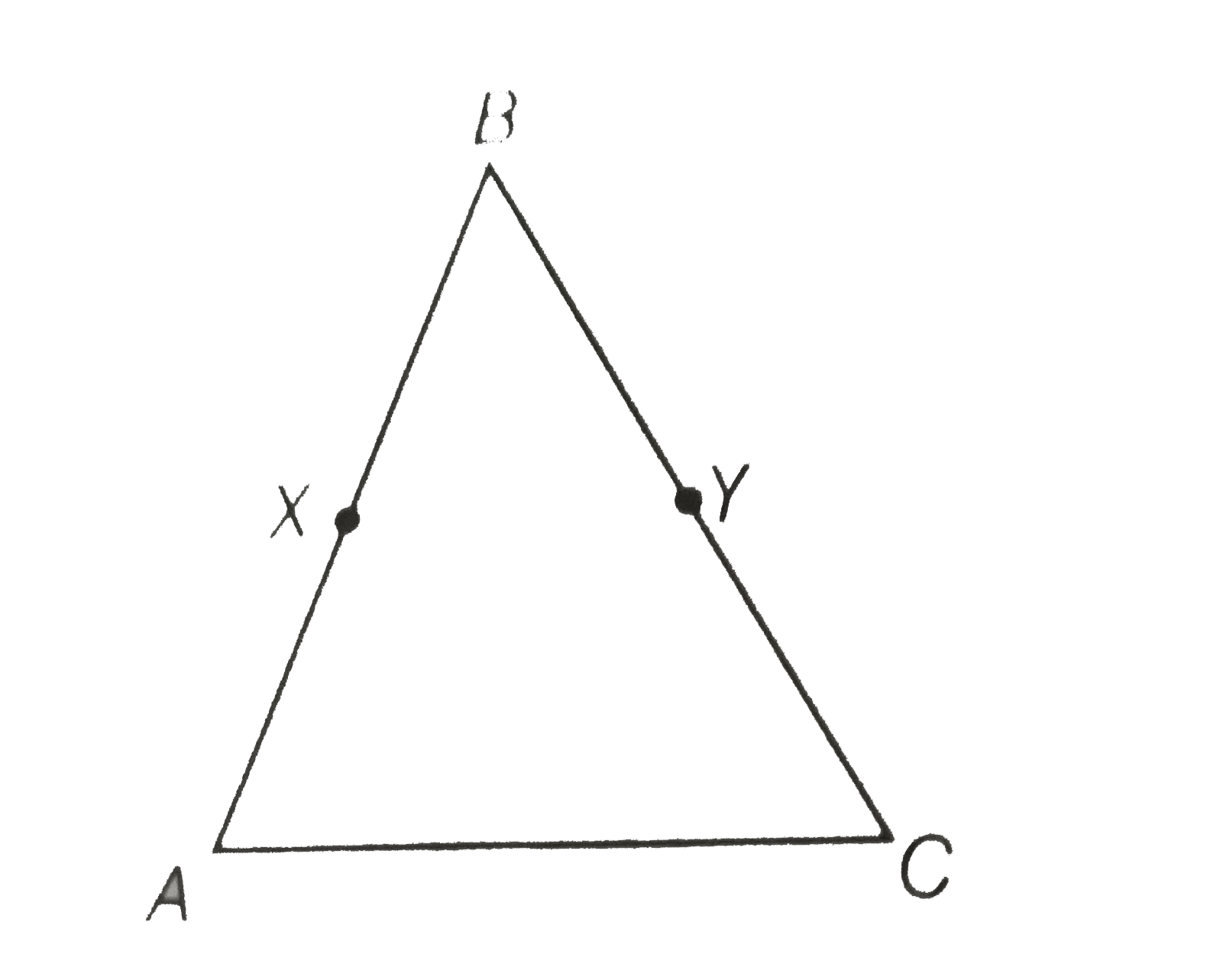
View SolutionIn the adjoining figure , we have X and Y are the mid - point of AC and BC and AX = CY . Show that AC =BC.
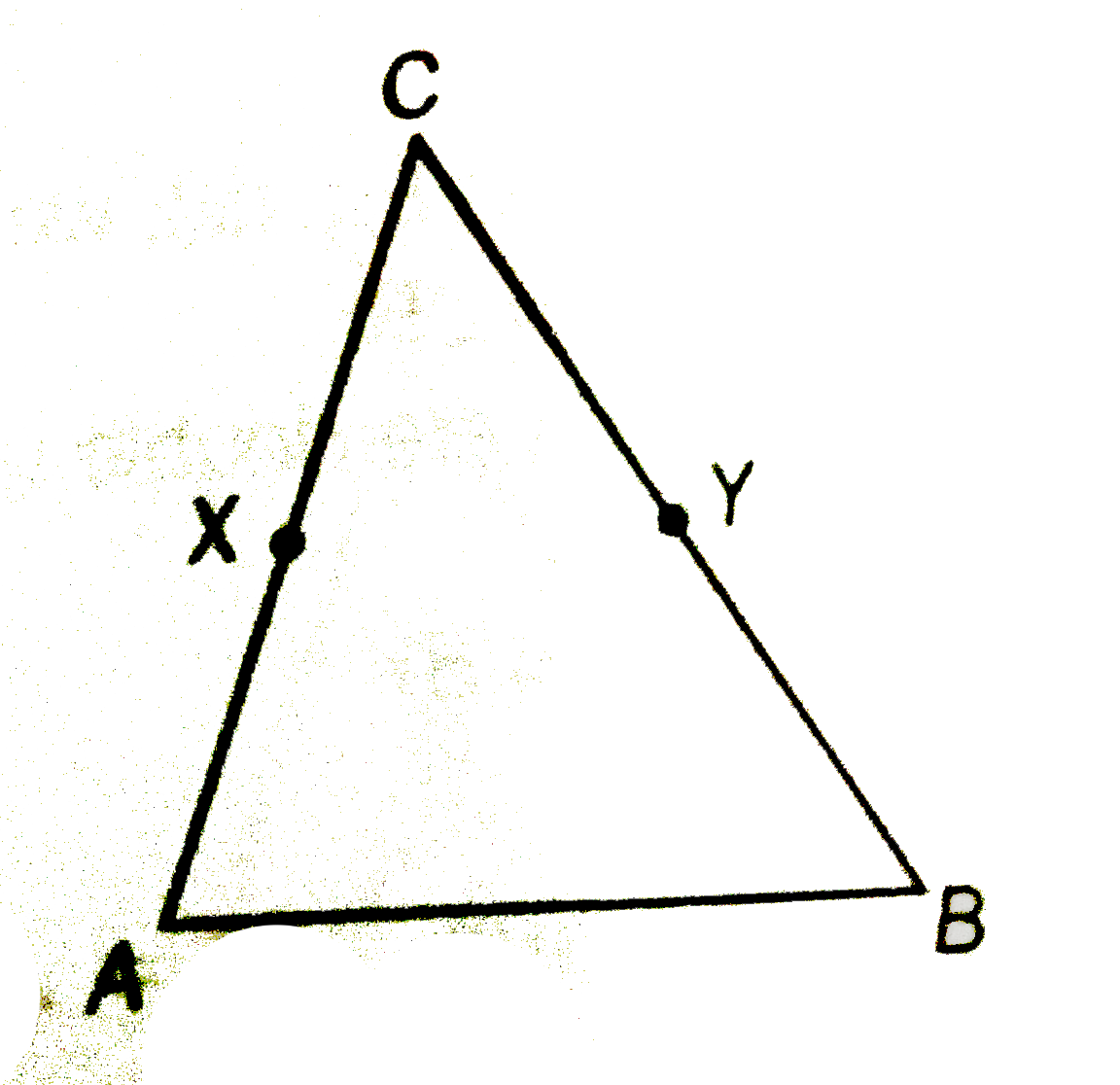
View SolutionIn the adjoining figure , if `BX=(1)/(2)AB,BY=(1)/(2)BCand AB=BC`, then show that BX =BY.
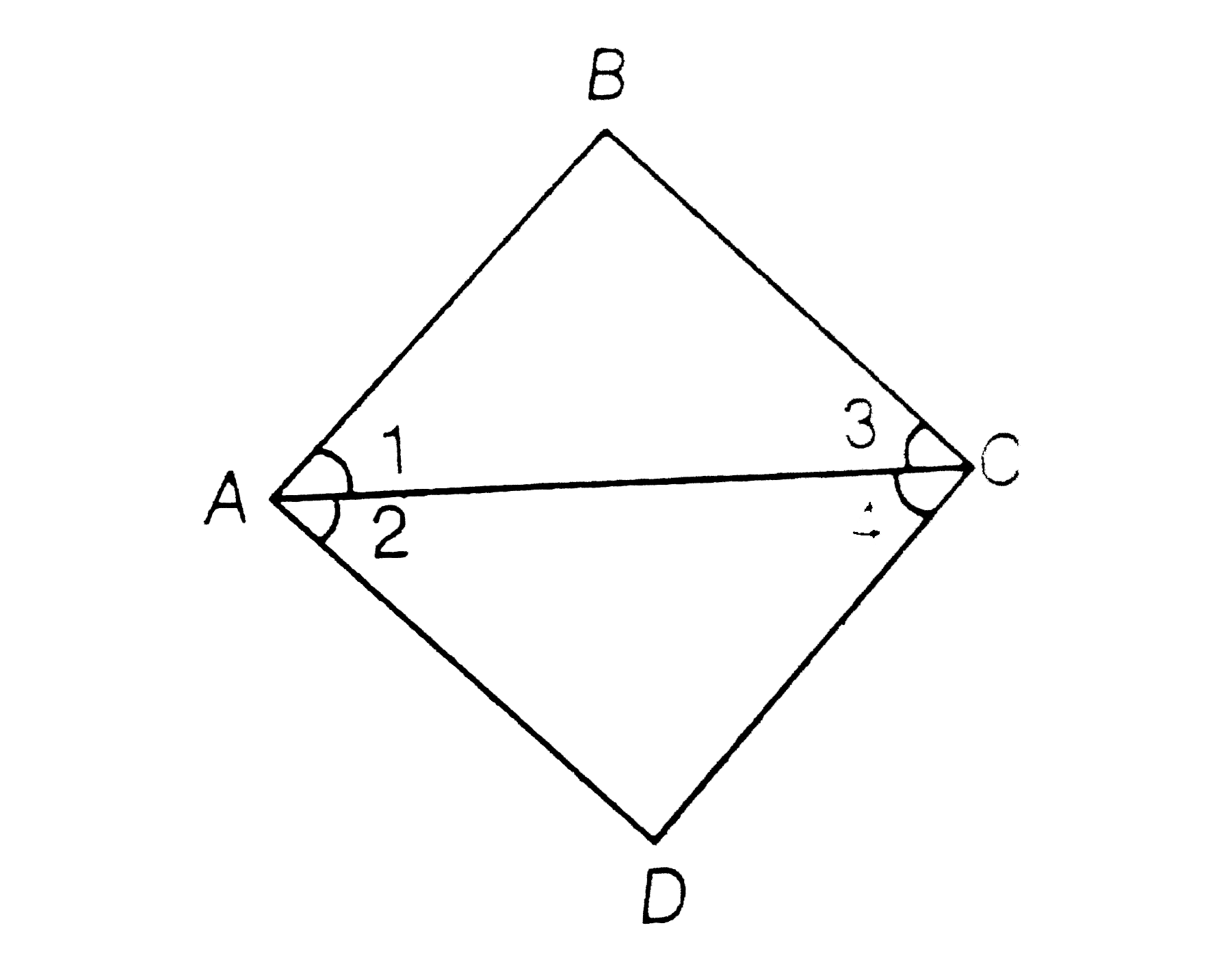
View SolutionIn the adjoining figure, we have `angle1=angle2` and `angle 2 =angle3.` Show that `angle1=angle3.`

View SolutionIn the adjoining figure, we have `angle1=angle3 and angle2=angle4`. Show that `angleA=angleC`.
View SolutionIn the ajoining figure , we have`angleABC=angleACBand angle3=angle4`. Show that BD=DC.
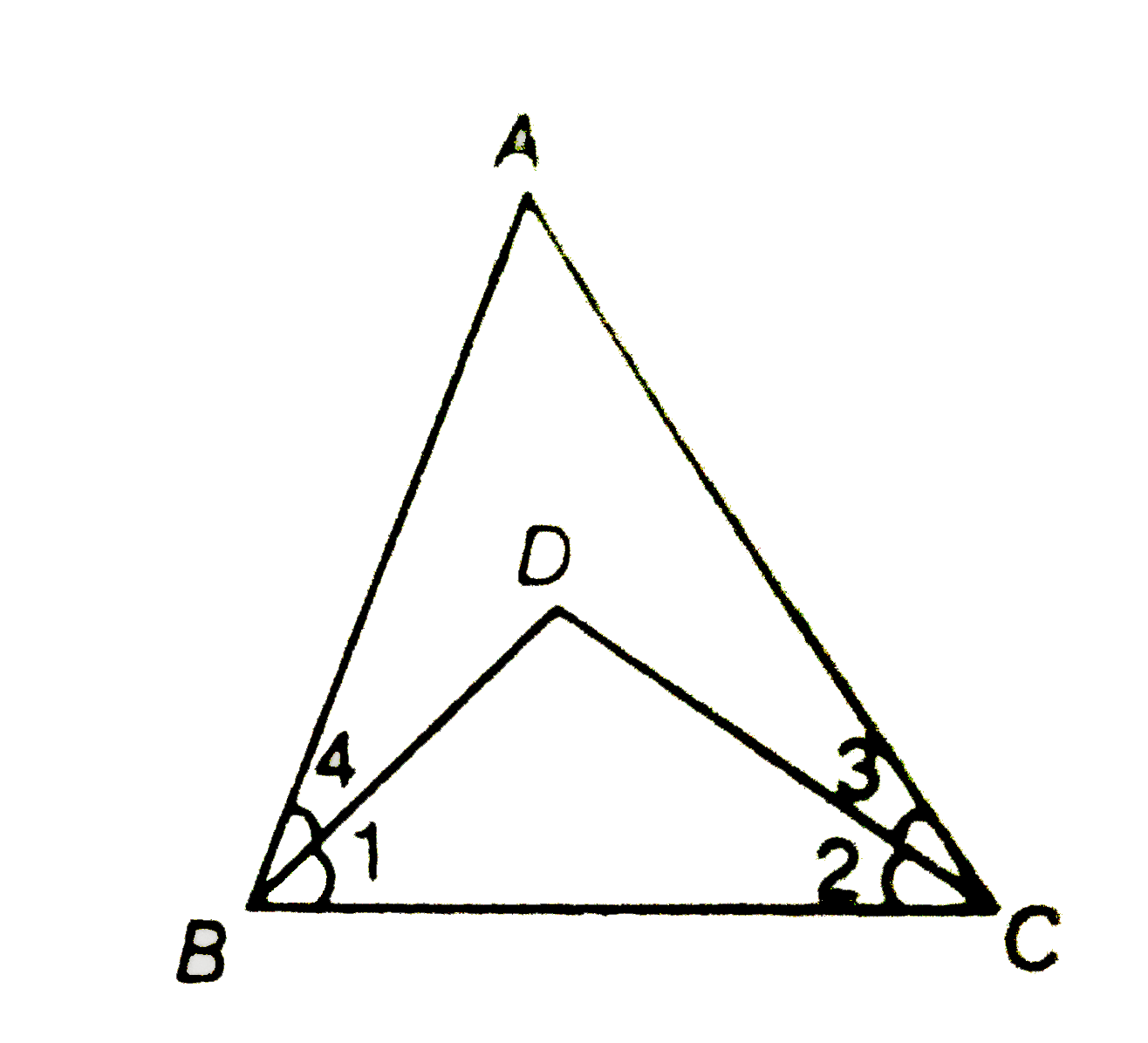
View SolutionIn the adjoining figure , we have AC=DC and CB = CE. Show that AB=DE.
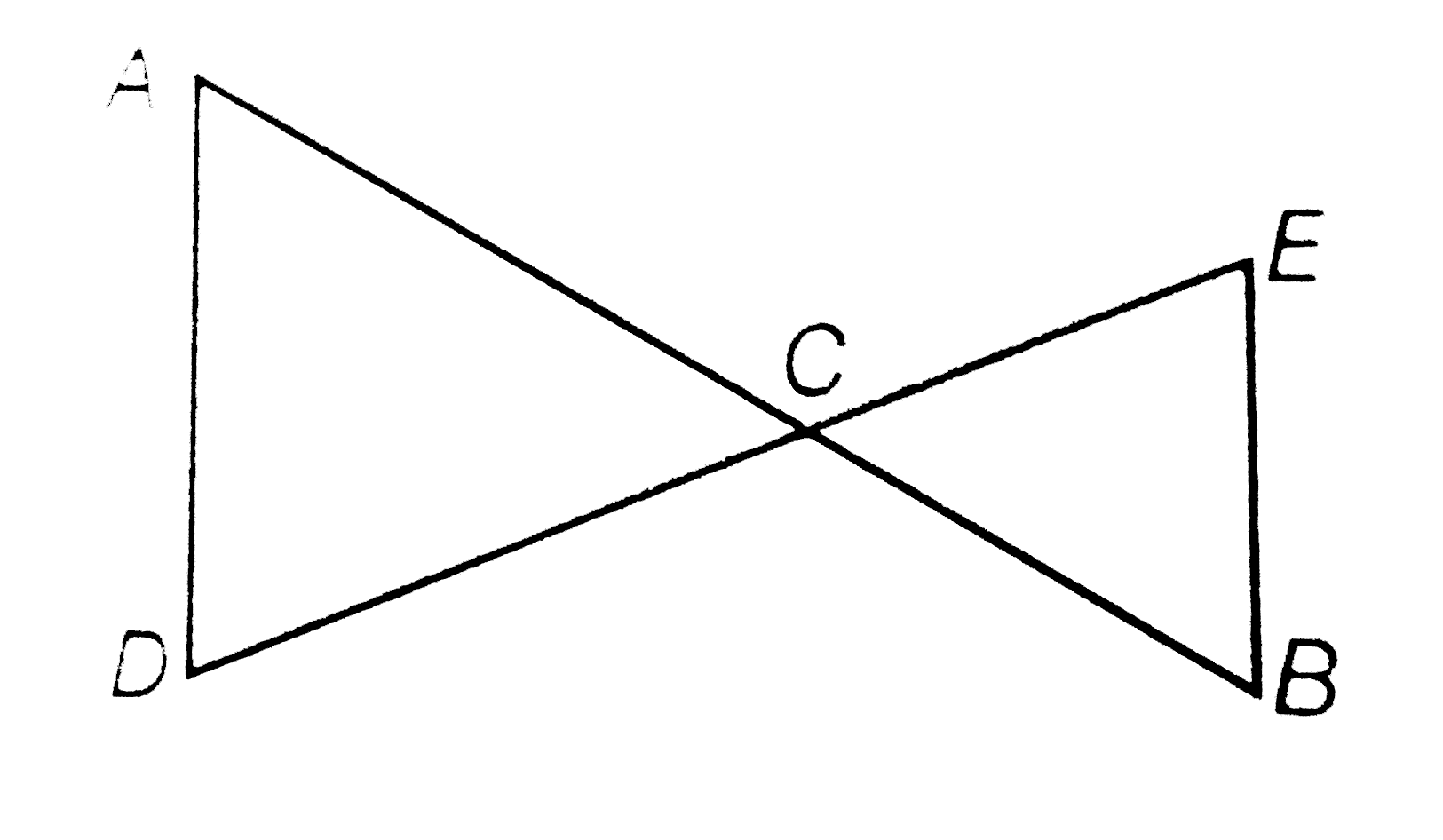
View SolutionIn the adjoining figure, `OX =1/2XY, PX =1/2XZ `and OX =PX. Show that XY= XZ
View SolutionIn the adjoining figure
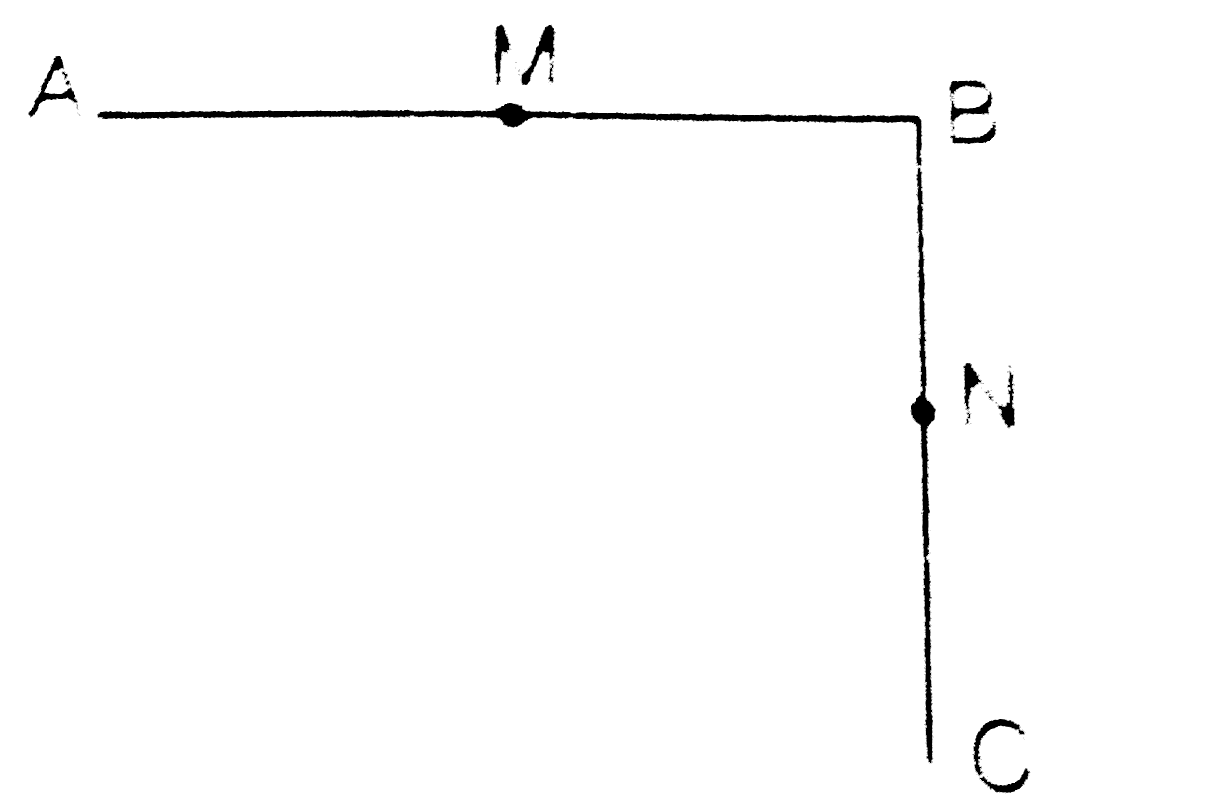
(i) AB=BC, M is the mid -point of AB and N is the mid- point of BC. Show that AM=NC.
(ii) BM=BN,M is the mid- point of AB and N is the mid - point of BC. Show that AB=BC.
View SolutionRead the following statements:
An equilateral triangle is a polygon made up of three line segments out of which two line segments are equal to the third - one and all its angles are `60^(@)` each.
Define the terms used in this definition which you feel necessary. Are there any undefined terms in this ? Can you justify that all sides and all angles are equal in a equilateral triangle.
View SolutionStudy the following statements
" Two intersecting lines cannot be perpendicular to the same line " Check whether it is an equivalent version to the Euclid's fifth postulate.
View SolutionRead the following statements which are taken as axioms
(i) If a transversal intersects two parallel lines, then corresponding angles are not necessarily equal.
(ii) If a transversal intersect two parallel lines, then alternate interior angles are equal.
Is this system of axioms consistent ? Justify your answer.
View SolutionRead the following two statements which are taken as axiom:
(i) If two lines intersect each other , then the vertically opposite angles are not equal.
(ii) If a ray stands on a line , then the sum of two adjacent angles, so formed is equal to `180^(@)`.
Is this system of axioms consistent ? Justify your answer.
View SolutionRead the following axioms
(i) Things which are equal to the same thing are equal to one another.
(ii) If equals are added to equals, the wholes are equal.
(iii) Things which are double of the same things are equal to one another.
Check whether the given system of axioms is consistent or inconsistent.
View Solution
Introduction To Euclid Geometry questions and complete solutions for chapter INTRODUCTION TO EUCLID GEOMETRY of NCERT MATHS (HINGLISH) of Class 9