SECTION-B (NUMERICAL FROM TEXTUAL EXERCISE) from KUMAR PRAKASHAN KENDRA PHYSICS (GUJRATI ENGLISH) OSCILLATIONS for Class 11
| Class 11 | PHYSICS |
| Chapter | OSCILLATIONS |
| KUMAR PRAKASHAN KENDRA PHYSICS (GUJRATI ENGLISH) | SECTION-B (NUMERICAL FROM TEXTUAL EXERCISE) |
| Medium | English |
| Academic Year | 2023-2024 |
Question 9
Which of the following functions of time represent (a) simple harmonic, (b) periodic but not simple harmonic, and (c) non-periodic motion? Give period for each case of periodic motion (ω is any positive constant):
(a) sinωt−cosωt
(b) sin3ωt
(c) 3cos(π/4−2ωt)
(d) cosωt+cos3ωt+cos5ωt
(e) exp(−ω2t2)
(f) 1+ωt+ω2t2View SolutionQuestion 12
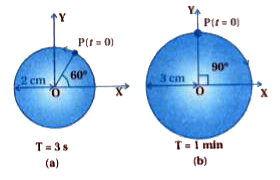
Figures depicts two circular motions. The radius of the circle, the period of revolution, the initial position and the sense of revolution are indicated on the figures. Obtain the simple harmonic motions of the x-projection of the radius vector of the rotating particle P in each case.
 View Solution
View SolutionQuestion 20
Two identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in Fig. 14.14. Show that when the mass is displaced from its equilibrium position on either side, it executes a simple harmonic motion. Find the period of oscillations.
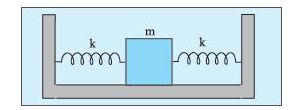 View Solution
View SolutionQuestion 21
A block whose mass is 1kg is fastened to a spring. The spring has a spring constant of 50Nm−1. The block is pulled to a distance x=10 cm from its equilibrium position at x=0 on a frictionless surface from rest at t=0. Calculate the kinetic, potential and total energies of the block when it is 5 cm away from the mean position.View SolutionQuestion 22
A block whose mass is 2kg is fastened to a spring. The spring has a spring constant of 100Nm−1. The block is pulled to a distance x=10 cm from its equilibrium position at x=0 on a frictionless surface from rest at t=0. Calculate the kinetic, potential and total energies of the block when it is 5 cm away from the mean position.View SolutionQuestion 23
A block whose mass is 1kg is fastened to a spring. The spring has a spring constant of 50Nm−1. The block is pulled to a distance x=10 cm from its equilibrium position at x=0 on a frictionless surface from rest at t=0. Calculate the kinetic, potential and total energies of the block when it is 7.07cm away from the mean position.View SolutionQuestion 25
A 5 kg collar is attached to a spring of spring constant 500 N m−1. It slides without friction over a horizontal rod. The collar is displaced from its equilibrium position by 10.0 cm and released. Calculate
(a) the period of oscillation,
(b) the maximum speed and
(c) maximum acceleration of the collar.View SolutionQuestion 26
A 5 kg collar is attached to a spring of spring constant 500 N m−1. It slides without friction over a horizontal rod. The collar is displaced from its equilibrium position by 10.0 cm and released. Calculate
(a) the period of oscillation,
(b) the maximum speed and
(c) maximum acceleration of the collar.View SolutionQuestion 50
Which of the following functions of time represent (a) simple harmonic, (b) periodic but not simple harmonic, and (c) non-periodic motion? Give period for each case of periodic motion (ω is any positive constant):
(a) sinωt−cosωt
(b) sin3ωt
(c) 3cos(π/4−2ωt)
(d) cosωt+cos3ωt+cos5ωt
(e) exp(−ω2t2)
(f) 1+ωt+ω2t2View SolutionQuestion 51
Which of the following functions of time represent (a) simple harmonic, (b) periodic but not simple harmonic, and (c) non-periodic motion? Give period for each case of periodic motion (ω is any positive constant):
(a) sinωt−cosωt
(b) sin3ωt
(c) 3cos(π/4−2ωt)
(d) cosωt+cos3ωt+cos5ωt
(e) exp(−ω2t2)
(f) 1+ωt+ω2t2View SolutionQuestion 52
Which of the following functions of time represent (a) simple harmonic, (b) periodic but not simple harmonic, and (c) non-periodic motion? Give period for each case of periodic motion (ω is any positive constant):
(a) sinωt−cosωt
(b) sin3ωt
(c) 3cos(π/4−2ωt)
(d) cosωt+cos3ωt+cos5ωt
(e) exp(−ω2t2)
(f) 1+ωt+ω2t2View SolutionQuestion 53
Which of the following functions of time represent (a) simple harmonic, (b) periodic but not simple harmonic, and (c) non-periodic motion? Give period for each case of periodic motion (ω is any positive constant):
(a) sinωt−cosωt
(b) sin3ωt
(c) 3cos(π/4−2ωt)
(d) cosωt+cos3ωt+cos5ωt
(e) exp(−ω2t2)
(f) 1+ωt+ω2t2View SolutionQuestion 54
Which of the following functions of time represent (a) simple harmonic, (b) periodic but not simple harmonic, and (c) non-periodic motion? Give period for each case of periodic motion (ω is any positive constant):
(a) sinωt−cosωt
(b) sin3ωt
(c) 3cos(π/4−2ωt)
(d) cosωt+cos3ωt+cos5ωt
(e) exp(−ω2t2)
(f) 1+ωt+ω2t2View SolutionQuestion 55
Which of the following functions of time represent (a) simple harmonic, (b) periodic but not simple harmonic, and (c) non-periodic motion? Give period for each case of periodic motion (ω is any positive constant):
(a) sinωt−cosωt
(b) sin3ωt
(c) 3cos(π/4−2ωt)
(d) cosωt+cos3ωt+cos5ωt
(e) exp(−ω2t2)
(f) 1+ωt+ω2t2View SolutionQuestion 66
The motion of a particle executing simple harmonic motion is described by the displacement function,
x(t)=Acos(ωt+ϕ).
If the initial (t = 0) position of the particle is 1 cm and its initial velocity is ω cm/s, what are its amplitude and initial phase angle ? The angular frequency of the particle is πs−1. If instead of the cosine function, we choose the sine function to describe the SHM : x=Bsin(ωt+α), what are the amplitude and initial phase of the particle with the above initial conditions.View SolutionQuestion 68
A spring having with a spring constant 1200Nm−1 is mounted on a horizontal table as shown in Fig. 14.24. A mass of 3 kg is attached to the free end of the spring. The mass is then pulled sideways to a distance of 2.0 cm and released.
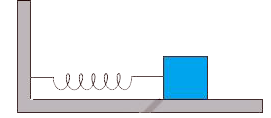
Determine (i) the frequency of oscillations, (ii) maximum acceleration of the mass, and (iii) the maximum speed of the mass.View SolutionQuestion 69
A spring having with a spring constant 1200Nm−1 is mounted on a horizontal table as shown in Fig. 14.24. A mass of 3 kg is attached to the free end of the spring. The mass is then pulled sideways to a distance of 2.0 cm and released.

Determine (i) the frequency of oscillations, (ii) maximum acceleration of the mass, and (iii) the maximum speed of the mass.View SolutionQuestion 70
A spring having with a spring constant 1200Nm−1 is mounted on a horizontal table as shown in Fig. 14.24. A mass of 3 kg is attached to the free end of the spring. The mass is then pulled sideways to a distance of 2.0 cm and released.

Determine (i) the frequency of oscillations, (ii) maximum acceleration of the mass, and (iii) the maximum speed of the mass.View SolutionQuestion 71
In Exercise 9, let us take the position of mass when the spring is unstretched as x=0, and the direction from left to right as the positive direction of x-axis. Give x as a function of time t for the oscillating mass if at the moment we start the stopwatch (t=0), the mass is at the mean position.View SolutionQuestion 72
In Exercise 9, let us take the position of mass when the spring is unstretched as x=0, and the direction from left to right as the positive direction of x-axis. Give x as a function of time t for the oscillating mass if at the moment we start the stopwatch (t=0), the mass is at the maximum streatched position.View SolutionQuestion 73
In Exercise 9, let us take the position of mass when the spring is unstretched as x=0, and the direction from left to right as the positive direction of x-axis. Give x as a function of time t for the oscillating mass if at the moment we start the stopwatch (t=0), the mass is at the maximum compressed position.
In what way to these functions for SHM differ from each other, in frequency, in amplitude or the initial phase?View SolutionQuestion 74
Figures 14.25 correspond to two circular motions. The radius of the circle, the period of revolution, the initial position, and the sense of revolution (i.e. clockwise or anti-clockwise) are indicated on each figure.
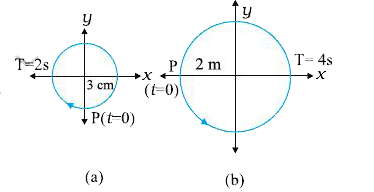
Obtain the corresponding simple harmonic motions of the x-projection of the radius vector of the revolving particle P, in each case.View SolutionQuestion 75
Plot the corresponding reference circle for each of the following simple harmonic motions. Indicate the initial (t =0) position of the particle, the radius of the circle, and the angular speed of the rotating particle. For simplicity, the sense of rotation may be fixed to be anticlockwise in every case: (x is in cm and t is in s).View SolutionQuestion 76
Plot the corresponding reference circle for each of the following simple harmonic motions. Indicate the initial (t =0) position of the particle, the radius of the circle, and the angular speed of the rotating particle. For simplicity, the sense of rotation may be fixed to be anticlockwise in every case: (x is in cm and t is in s).View SolutionQuestion 77
Plot the corresponding reference circle for each of the following simple harmonic motions. Indicate the initial (t =0) position of the particle, the radius of the circle, and the angular speed of the rotating particle. For simplicity, the sense of rotation may be fixed to be anticlockwise in every case: (x is in cm and t is in s).View SolutionQuestion 78
Plot the corresponding reference circle for each of the following simple harmonic motions. Indicate the initial (t =0) position of the particle, the radius of the circle, and the angular speed of the rotating particle. For simplicity, the sense of rotation may be fixed to be anticlockwise in every case: (x is in cm and t is in s).View SolutionQuestion 79
Figure 14.26 (a) shows a spring of force constant k clamped rigidly at one end and a mass m attached to its free end. A force F applied at the free end stretches the spring. Figure 14.26 (b) shows the same spring with both ends free and attached to a mass m at either end. Each end of the spring in Fig. 14.26(b) is stretched by the same force F.

What is the maximum extension of the spring in the two cases ? View SolutionQuestion 83
Answer the following question :
The motion of a simple pendulum is approximately simple harmonic for small angle oscillations. For larger angles of oscillation, a more involved analysis shows that T is greater than 2π√lg, Think of a qualitative argument to appreciate this result.View SolutionQuestion 86
A simple pendulum of length l and having a bob of mass M is suspended in a car. The car is moving on a circular track of radius R with a uniform speed v. If the pendulum makes small oscillations in a radial direction about its equilibrium position, what will be its time period ?View SolutionQuestion 87
A cylindrical piece of cork of density of base area A and height h floats in a liquid of density pl. The cork is depressed slightly and then released. Show that the cork oscillates up and down simple harmonically with a period
T=2π√hpp1g
where p is the density of cork. (Ignore damping due to viscosity of the liquid).View SolutionQuestion 88
One end of a U-tube containing mercury is connected to a suction pump and the other end to atmosphere. A small pressure difference is maintained between the two columns. Show that, when the suction pump is removed, the column of mercury in the U-tube executes simple harmonic motion.View SolutionQuestion 89
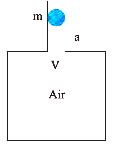
An air chamber of volume V has a neck area of cross section a into which a ball of mass m just fits and can move up and down without any friction (Fig.14.27). Show that when the ball is pressed down a little and released , it executes SHM. Obtain an expression for the time period of oscillations assuming pressure-volume variations of air to be isothermal [see Fig. 14.27].
 View Solution
View SolutionQuestion 90
You are riding in an automobile of mass 3000 kg. Assuming that you are examining the oscillation characteristics of its suspension system. The suspension sags 15 cm when the entire automobile is placed on it. Also, the amplitude of oscillation decreases by 50% during one complete oscillation. Estimate the values of the spring constant k.View SolutionQuestion 91
You are riding in an automobile of mass 3000 kg. Assuming that you are examining the oscillation characteristics of its suspension system. The suspension sags 15 cm when the entire automobile is placed on it. Also, the amplitude of oscillation decreases by 50% during one complete oscillation. Estimate the values of the damping constant b for the spring and shock absorbar system of one wheel, assuming that each wheel supports 750 kg.View SolutionQuestion 93
A circular disc of mass 10 kg is suspended by a wire attached to its centre. The wire is twisted by rotating the disc and released. The period of torsional oscillations is found to be 1.5s. The radius of the disc is 15 cm. Determine the torsional spring constant of the wire. (Torsional spring constant α is defined by the relation J=−αθ, where J is the restoring couple and θ the angle of twist).View SolutionQuestion 97
A mass attached to a spring is free to oscillate, with angular velocity ω, in a horizontal plane without friction or damping. It is pulled to a distance x0 and pushed towards the centre with a velocity v0 at time t=0. Determine the amplitude of the resulting oscillaters ω,x0 and v0. [Hint : Start with the equation x=acos(ωt+θ) and note that the initial velocity is negative.]View SolutionQuestion 103
A particle executes SHM on a straight line path. The amplitude of oscillation is 2 cm. When the displacement of the particle from the mean position is 1 cm, the magnitude of its acceleration is equal to that of its velocity. Find the time period, maximum velocity and maximum acceleration of SHM.View SolutionQuestion 104
A rectangular pipe having cross-sectional area A is closed at one end and at its other end a block having same cross-section is placed so that the system is airtight. In the equilibrium position of the block, the pressure and volume of air enclosed in the pipe and P and V respectively. Prove that the block performs SHM when it is given a small displacement 'x' inward and released. Also find the period of this SHM. Assume the walls to be frictionless and compression of air to be isothermal.View Solution
All Exercises from KUMAR PRAKASHAN KENDRA PHYSICS (GUJRATI ENGLISH) Class 11 PHYSICS OSCILLATIONS
- SECTION-A (QUESTIONS- ANSWERS)
- SECTION-A (TRY YOURSELF)
- SECTION-B (ADDITIONAL EXERCISE)
- SECTION-B (NUMERICAL FROM DARPAN BASED ON TEXTBOOK)
- SECTION-B (NUMERICAL FROM TEXTUAL EXERCISE)
- SECTION-B (NUMERICAL FROM TEXTUAL ILLUSTRATIONS)
- SECTION-C (OBJECTIVE QUESTIONS ASSERTION OR REASION)
- SECTION-C (OBJECTIVE QUESTIONS FILL IN THE BLANK)
- SECTION-C (OBJECTIVE QUESTIONS MATCHING)
- SECTION-C (OBJECTIVE QUESTIONS TRUE OR FALSE)
- SECTION-C (OBJECTIVE QUESTIONS)
- SECTION-D (NCERT EXEMPLAR SOLUTION LONG ANSWER QUESTIONS)
- SECTION-D (NCERT EXEMPLAR SOLUTION MCQS MORE THAN ONE OPTIONS)
- SECTION-D (NCERT EXEMPLAR SOLUTION MCQS)
- SECTION-D (NCERT EXEMPLAR SOLUTION SHORT ANSWER QUESTIONS)
- SECTION-D (NCERT EXEMPLAR SOLUTION VERY SHORT ANSWER QUESTIONS)
- SECTION-E (MCQS ASKED IN GUJARAT BOARD AND COMPETITIVE EXAMS)
- SECTION-F (QUESTIONS FROM MODULE SIMPLE QUESTIONS)
- QUESTION PAPER (SECTION - A)
- QUESTION PAPER (SECTION - B)
- QUESTION PAPER (SECTION - C)
- QUESTION PAPER (SECTION - D)