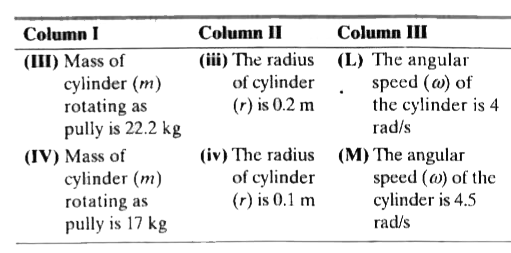
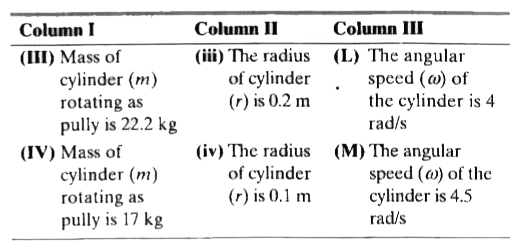
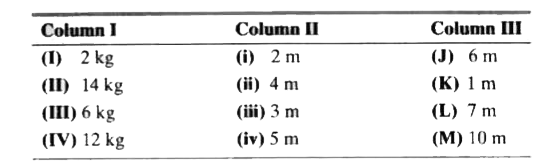
PRACTICE QUESTIONS (Matrix-Match) from RESNICK AND HALLIDAY PHYSICS (HINGLISH) RIGID BODY DYNAMICS-II for Class 12
| Class 12 | PHYSICS |
| Chapter | RIGID BODY DYNAMICS-II |
| RESNICK AND HALLIDAY PHYSICS (HINGLISH) | PRACTICE QUESTIONS (Matrix-Match) |
| Medium | English |
| Academic Year | 2023-2024 |
Question 3
A pulley os radius 2m is rotated about its axis by a force F=(20t−5t2) newton (where t is measured in seconds) applied tangentially. If the moment of inertia of the pulley about its axis of rotation is 10kgm2 the number of rotaitons made by the pulley before its direction of motion is reversed, is:View SolutionQuestion 14
Two disks are rotating about the same axis. Disk A has a moment of inertia of 3.4kg−m2 and an angular velocity of +72rad/s. Disk B is rotating with an angular velocity of −9.8rad/s. The two disks are then linked together without the aid of any external torques, so that they rotate as a single unit with an angular velocity of −2.4rad/s. The axis of rotation for this unit is the same as that for the separate disks. What is the moment of inertia of disk B?View SolutionQuestion 16
A solid disk rotates in the horizontal plane at an angular velocity of 0.067rad/s with respect to an axis perpendicular to the disk at its center. The moment of inertia of the disk is 0.10kg.m2. From above, sand is dropped straight down onto this rotating disk, so that a thin uniform ring of sand is formed at a distance of 0.40 m from the axis. The sand in the ring has a mass of 0.50 kg. After all the sand is in place, what is the angular velocity of the disk?View SolutionQuestion 17
A flat uniform circular disk (radius = 2.00 m, mass=1.00×102 kg) is initially stationary. The disk is free to rotate in the horizontal plane about a frictionless axis perpendic ular to the center of the disk. A 40.0-kg person, standing 1.25 m from the axis, begins to run on the disk in a circular path and has a tangential speed of 2.00m/s relative to the ground. Find the resulting angular speed of the disk (in rad/s) and describe the direction of the rotation.View SolutionQuestion 18
A thin uniform rod is rotating at an angular velocity of 7.0rad/s about an axis that is perpendicular to the rod at its center. As the drawing indicates, the rod is hinged at two places, one-quarter of the length from each end. Without the aid of external torques, the rod suddenly assumes a u shape, with the arms of the u parallel to the rotation axis. What is the angular velocity of the rotating u?View SolutionQuestion 19
A small 0.500 kg object moves on a frictionless horizontal table in a circular path of radius 1.00 m. The angular speed is 6.28rad/s. The object is attached to a string of negligible mass that passes through a small hole in the table at the center of the circle. Someone under the table begins to pull the string downward to make the circle smaller. If the string will toler ate a tension of no more than 105 N, what is the radius of the smallest possible circle on which the object can move?View SolutionQuestion 20
One end of a thin rod is attached to a pivot, about which it can rotate without friction. Air resistance is absent. The rod has a length of 0.80 m and is uniform. It is hanging vertically straight downward. The end of the rod nearest the floor is given a linear speed v0, so that the rod begins to rotate upward about the pivot. What must be the value of v0 such that the rod comes to a momentary halt in a straight-up orientation, exactly opposite to its initial orientation?View SolutionQuestion 21
A marble and a cube are placed at the top of a ramp. Starting from rest at the same height, the marble rolls and the cube slides (no kinetic friction) down the ramp. Determine the ratio of the center-of-mass speed of the cube to the center-of-mass speed of the marble at the bottom of the ramp.View SolutionQuestion 22
A particle of mass 2 kg is on a smooth horizontal table and moves in a circular path of radius 0.6 m. The height of the table from the ground is 0.8 m. If the angular speed of the particle is 12 rad s−1 , the magnitude of its angular momentum about a point on the ground right under the centre of the circle is View SolutionQuestion 23
Two thin rectangular sheets (0.20m×0.40m) are identical. In the first sheet the axis of rotation lies along the 0.20-m side, and in the second it lies along the 0.40-m side. The same torque is applied to each sheet. The first sheet, starting from rest, reaches its final angular velocity in 8.0 s. How long does it take for the second sheet, starting from rest, to reach the same angular velocity?View SolutionQuestion 24
A disk in xy plane, initially at rest, of mass 1 kg and radius 10 m, with center at origin, can only rotate about its axis, that is, about z-axis due to a fixed axle through its center. A force →F=(3ˆi−4ˆj+ˆk) N starts acting on it at the point (3ˆi+5ˆj) m. The work done by the force in the first one second isView SolutionQuestion 25
A solid cylinder and a thin-walled hollow cylinder have the same mass and radius. They are rolling horizontally toward the bottom of an incline. The center of mass of each has the same translational speed. The cylinders roll up the incline and reach their highest points. Calculate the ratio of the distances (SSolid/Shollow) along the incline through which each center of mass moves.View SolutionQuestion 26
A thin rod has a length of 0.25 m and rotates in a circle on a frictionless tabletop. The axis is perpendicular to the length of the rod at one of its ends. The rod has an angular velocity of 0.32rad/s and a moment of inertia of 1.1×10−3kg.m2. A bug standing on the axis decides to crawl out to the other end of the rod. When the bug (mass=4.2×10−3kg) gets where it is going, what is the angular velocity of the rod?View SolutionQuestion 27
A small 0.500 kg object moves on a frictionless horizontal table in a circular path of radius 1.00 m. The angular speed is 6.28rad/s. The object is attached to a string of negligible mass that passes through a small hole in the table at the center of the circle. Someone under the table begins to pull the string downward to make the circle smaller. If the string will tolerate a tension of no more than 105 N, what is the radius of the smallest possible circle on which the object can move?View SolutionQuestion 31
Two discs A and B are mounted coaxiallay on a vertical axle. The discs have moments of inertia I and 2 I respectively about the common axis. Disc A is imparted an initial angular velocity 2ω using the entire potential energy of a spring compressed by a distance x1 Disc B is imparted an angular velocity ω by a spring having the same spring constant and compressed by a distance x2 Both the discs rotate in the clockwise direction.
The ratio x1/x2 isView SolutionQuestion 32
Two discs A and B are mounted coaxiallay on a vertical axle. The discs have moments of inertia I and 2 I respectively about the common axis. Disc A is imparted an initial angular velocity 2ω using the entire potential energy of a spring compressed by a distance x1 Disc B is imparted an angular velocity ω by a spring having the same spring constant and compressed by a distance x2 Both the discs rotate in the clockwise direction.
When disc B is brought in contact with disc A, they acquire a common angular velocity in time t. The average frictional torque on one disc by the other during this period is View SolutionQuestion 33
Two discs A and B are mounted coaxially ona vertical axle. The discs have moments of inertia l and 2l respectively about the common axis. Disc A is imparted an initial angular velocity 2ω using the centre potential energy of a spring compressed by a distance x1. Disc B is imparted angular velocity ω by a spring having the same spring constant and compressed by a distance x2. Both the disc rotate in the clockwise direction.
The loss of kinetic energy the above process is -View SolutionQuestion 41
A uniform circular disc of mass 50kg and radius 0.4 m is rotating with an angular velocity of 10rads−1 about its own axis, which is vertical. Two uniform circular rings, each of mass 6.25 kg and radius 0.2 m, are gently placed symmetrically on the disc in such a manner that they are touching each other along the axis of the disc and are horizontal. Assume that the friction is large enough such that the rings are at rest relative to the disc and the system rotates about the original axis. The new angular velocity (∈reds−1) of the system isView SolutionQuestion 42
A binary star consists of two stars A(mass2.2Ms) and B (mass11Ms) where Ms is the mass of the sun, they are separted by distane d and are rotating about their center of mass, which is stationary. The ratio of the total angular momentum of the binary to the angular momentum of star B about the centre of mass isView Solution