A rigid rod of mass m and length L is suspended from two identical springs of negligible mass as shown in the diagram above. The upper ends of the springs are fixed in place and the springs stretch a distance d under the weight of the suspended rod.a. Determine the spring constant k of each spring in terms of the other given quantities and fundamental constants.As shown above the upper end of the springs are connected by a circuit branch containing a battery of emf ε and a switch S so that a complete circuit is formed with the metal rod and springs. The circuit has a total resistance R represented by the resistor in the diagram. The rod is in a uniform magnetic field directed perpendicular to the page. The upper ends of the springs remain fixed in place and the switch S is closed. When the system comes to equilibrium the rod has been lowered an additional distance Δd. b. With reference to the coordinate system shown above on the right what is the direction of the magnetic field? c. Determine the magnitude of the magnetic field in terms of m L d Δd ε R and fundamental constants. d. When the switch is suddenly opened the rod oscillates. For these oscillations determine the following quantities in terms of d Δd and fundamental constants: i. The period ii. The maximum speed of the rod
a Using hookes law. 2Fsp = mg 2kΔx = mg k = mg/2db From the battery we can see that + current flows to the right through the rod. In order to move the rod down a distance Δd the magnetic force must act down. Based on the RHR the B field would have to act out of the page +z.c The extra spring stretch must be balanced by the magnetic force. Fspextra = FbkΔd = BIL … mg/2d Δd = BIL Now substitute ε = IR for I and we get B → B = mgRΔd / εLdduse 2k for k since there are two springsii Set the equilibrium position at Δx = d as zero spring energy to use the turn horizontal trick. This is the maximum speed location and we now set the kinetic energy here to the spring energy and the Δd stretch position.
Related Solutions
A body of mass is suspended from a massless spring of natural length . It stretches the spring through a vertical distance . The potential energy of the stretched spring is
View SolutionWhen a mass M hangs from a spring of length l, it stretches the spring by a distance x. Now the spring is cut in two parts of length l/3 and 2l/3, and the two springs thus formed are connected to a straight rod of mass M which is horizontal in the configuration shown in figure. Find the stretch in each of the spring.
View SolutionWhen a mass M hangs from a spring of length l, it stretches the spring by a distance x. Now the spring is cut in two parts of length l//3"and" 2l//3 , and two springs thus formed are connected to a straight rod of mass M which is horizontal in the configuration shown in figure . Find the stretch in each of the spring
View SolutionWhen a mass M hangs from a spring of length l, it stretches the spring by a distance x. Now the spring is cut in two parts of length l/3 and 2l/3, and the two springs thus formed are connected to a straight rod of mass M which is horizontal in the configuration shown in figure. Find the stretch in each of the spring.
View SolutionA mass M is suspended by two springs of force constants K_(1) and K_(2) respectively as shown in the diagram. The total elongation (stretch) of the two springs is
View SolutionA rod of mass 'M' and length' L' is hinged about one end and a particle of mass 'M' is attached to its other end If it is kept horizontal with the help of a spring of spring constant 'K' as shown in fig. find the extension in spring :-
View SolutionAn ideal spring with spring constant k is suspended from the ceiling, and a block of mass M is attached to its lower end. If the mass is released initially when the spring is not stretched, then the maximum extension in the spring is:
View SolutionAn ideal spring having force constant k is suspended from the rigid support and a block of mass M is attached to its lowe end. The mass is released from the natural length of the spring. Then the maximum exiension in the spring is
View SolutionA spring of spring constant 'k' is used to suspend a mass 'm' at its free end while the other end of the spring is rigidly fixed.
If the mass is slightly depressed and released, then name the motion of the mass.
View SolutionIn the figure shown a uniform conducing rod of mass m and length l is suspended invertical plane by two conducing springs of spring constant k each. Upper end of springs are connected to each other by a capacitor of capacitance C. A uniform horizontal magnetic field perpendicular to plane of springs in space initially rod is in equillibrium. If the rod is pulled down and released, it performs SHM. (Assume resistance of springs and rod are negligible)
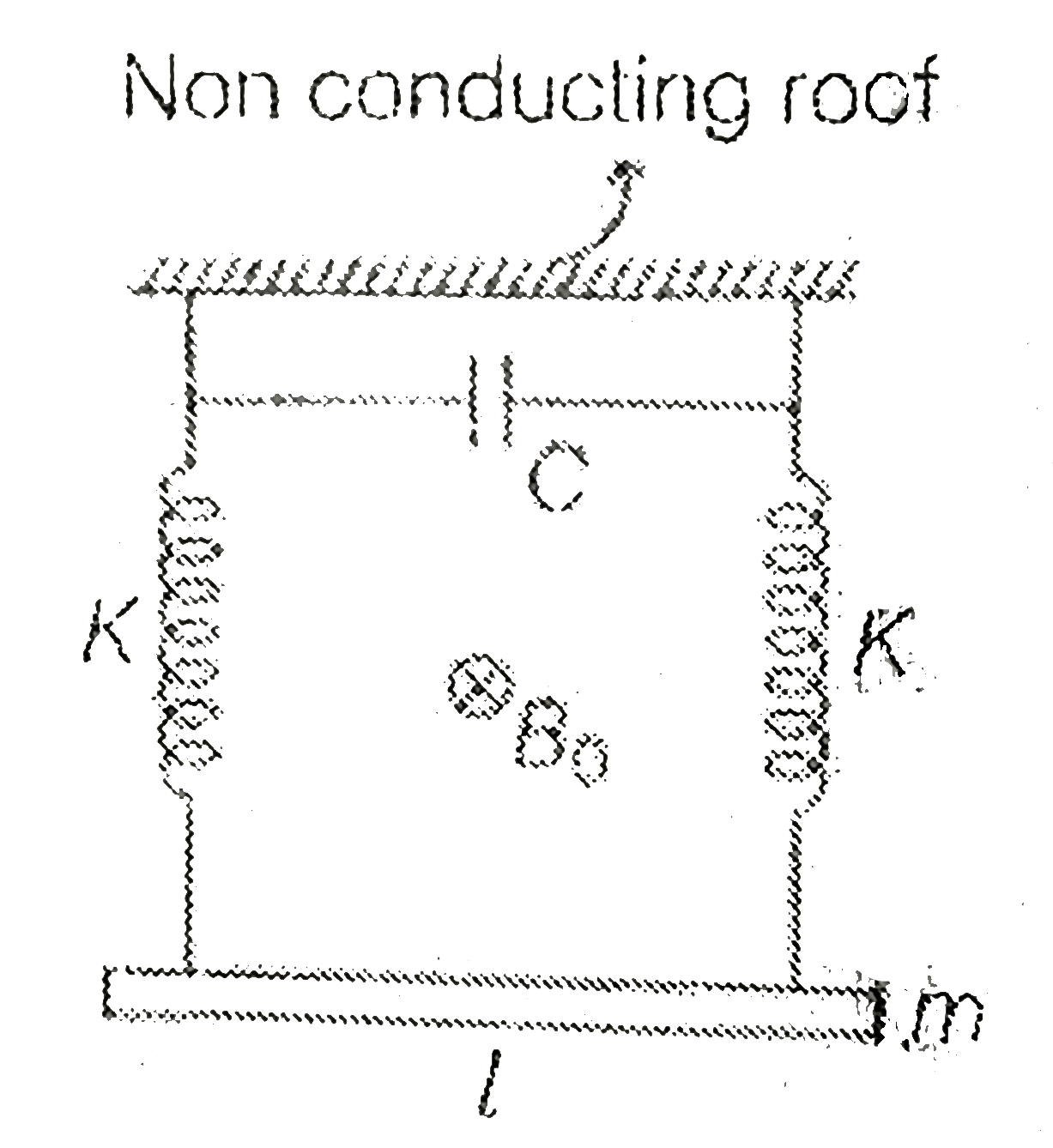
Choose the correct options from the followng:
View SolutionIn the figure shown a uniform conducing rod of mass m and length l is suspended invertical plane by two conducing springs of spring constant k each. Upper end of springs are connected to each other by a capacitor of capacitance C. A uniform horizontal magnetic field perpendicular to plane of springs in space initially rod is in equillibrium. If the rod is pulled down and released, it performs SHM. (Assume resistance of springs and rod are negligible)
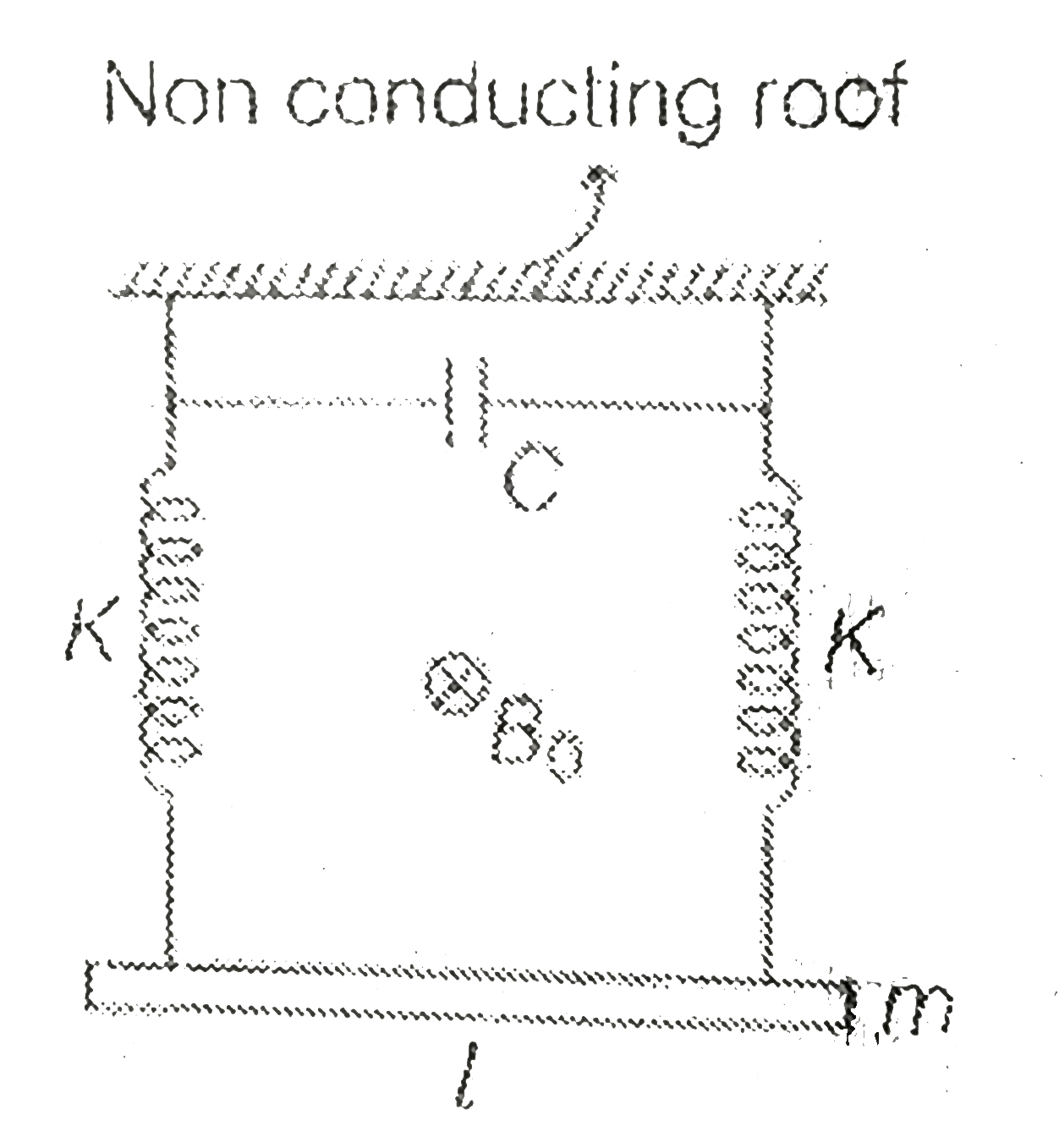
Find the period of oscillation of rod.
View SolutionIn the figure shown a uniform conducing rod of mass m and length l is suspended invertical plane by two conducing springs of spring constant k each. Upper end of springs are connected to each other by a capacitor of capacitance C. A uniform horizontal magnetic field perpendicular to plane of springs in space initially rod is in equillibrium. If the rod is pulled down and released, it performs SHM. (Assume resistance of springs and rod are negligible)

Find the period of oscillation of rod.
View SolutionTwo equal masses are attached to the two ends of a spring of spring constant k. The masses are pulled out symmetrically to stretch the spring by a length x over its natural length. The work done by the spring on each mass is
View SolutionTwo equal masses are attached to the two ends of a spring of spring constant . The masses are pulled a part symmetrically to stretch the spring by a length over its natural length. The work done by the spring on each mass is.
View SolutionTwo equal masses are attached to the two ends of a spring of spring constant . The masses are pulled a part symmetrically to stretch the spring by a length over its natural length. The work done by the spring on each mass is.
View SolutionTwo equal masses are attached to the two ends of a spring of spring constant k. The masses are pulled out symmetrically to stretch the spring by a length x over its natural length. The work done by the spring one each mass is
View SolutionTwo equal masses are attached to the two ends of a spring of spring constant k. The masses are pulled out symmetrically to stretch the spring by a length x over its natural length. The work done by the spring one each mass is
View SolutionAn ideal spring of spring constant k, is suspended from the ceiling of a room and a blok of mass m is fastened to its lower end. If the block is released when the spring is un-stretched, then then maximum extension in the spring is :
View SolutionAn ideal spring of spring constant k, is suspended from the ceiling of a room and a blok of mass m is fastened to its lower end. If the block is released when the spring is un-stretched, then then maximum extension in the spring is :
View SolutionAn ideal spring of spring constant k, is suspended from the ceiling of a room and a block of mass M is fastened to its lower end. If the block is released when the spring is un-stretched, then the maximum extension in the spring is
View Solution