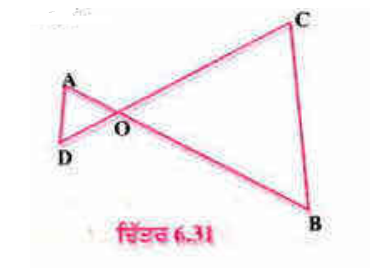
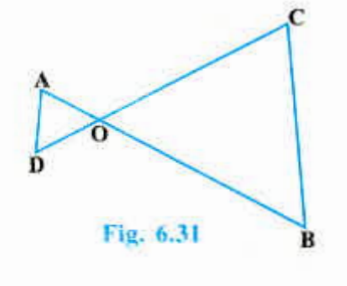
In Fig. 6.31 OA . OB = OC . OD.Show that ∠A =∠C and ∠B =∠D.
OA . OB = OC . OD GivenSo OA/OC=OD/OB .... 1Also we have ∠AOD =∠COB Vertically opposite angles ...... 2Therefore from 1 and 2 ΔAOD ~ ΔCOB SAS similarity criterionSo ∠A = ∠C and ∠D =∠BCorresponding angles of similar triangles
Related Solutions
ਚਿੱਤਰ 6.31 ਵਿੱਚ, OA . OB = OC . OD. ਹੈ। ਦਿਖਾਉ ਕਿ ਅਤੇ ਹੈ।
View Solutionபடத்தில் OA OB =OC OD .OD .A =C மற்றும் B =D எனக் காட்டுக ?
View SolutionIn Fig, OA . OB = OC . OD. Show that and .
View SolutionOABC is a parallelogram such that , then the value is
View SolutionIf OAB is a tetrahedron with edges and are unit vectors along bisectors of
respectively and , then :
View SolutionIf OAB is a tetrahedron with edges and are unit vectors along bisectors of
respectively and , then :
View SolutionIf OAB is a tetrahedron with edges and are unit vectors along bisectors of
respectively and , then :
View Solutionवक्तव्य । चित्रानुसार सदिशों OA, OB एवं OC का परिणामी है। R वृत्त की त्रिज्या है।
वक्तव्य || के अनुदिश है तथा के अनुदिश है।

View SolutionThe most appropriate I-H curve for a paramagnetic substances is
(a) OA
(b) OB
(c) OC
(d) OD

View SolutionIn figure OA. OB= OC. OD
Show that
View SolutionIf OA=vec a;OB=vec b;OC=2a+3b,OD=a-2, the length of OA is three xx the length of OB and OA is perpendicular to DB, then (BD xx AC).(OD xx OC) is
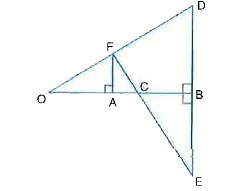
View SolutionIn the Fig. given below, OB is the perpendicular bisector of the line segment DE, and FE intersects OB at the point C. Prove that
View SolutionOABC is a tetrahedron such that OA=OB=OC=k and each of the edges OA,OB and OC is inclined at an angle theta with the other two the range of theta is
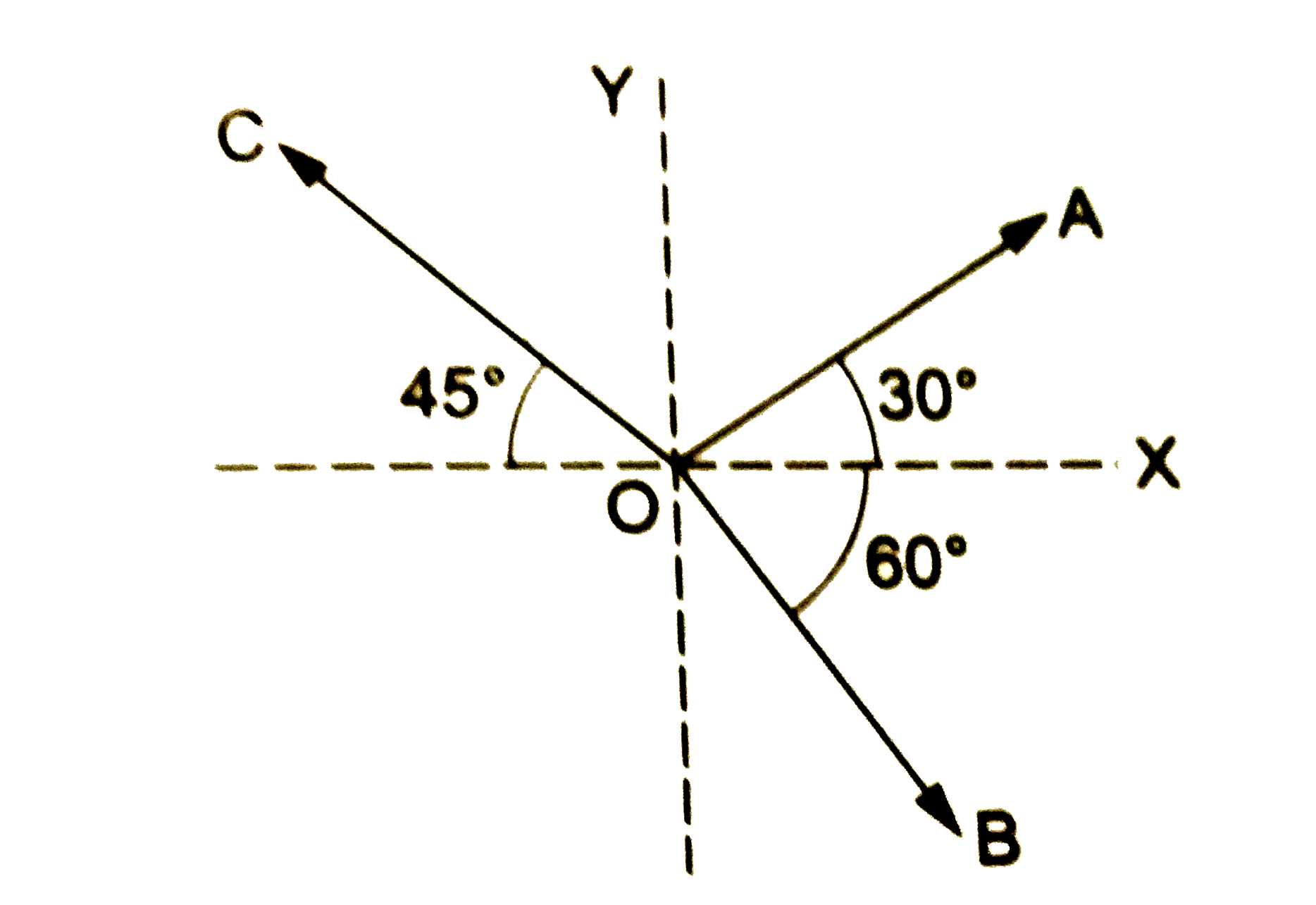
View SolutionThe magnitude of vectors in figure are equal. Find the direction of .
 .
.
View SolutionThe magnitude of vectors in figure are equal. Find the direction of .
 .
.
View SolutionThe magnitude of vectors in figure are equal. Find the direction of .
 .
.
View Solutionदिखाए गए वेक्टरो तथा के परिमाण बराबर है। वेक्टर की दिशा बताएँ।
View SolutionIn Fig. 4.126, . Prove that and . (FIGURE)
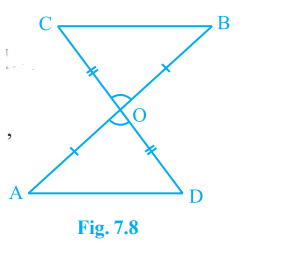
View SolutionIn Fig. 7.8, OA = OB and OD = OC. Show that (i) and (ii) AD || BC.
View Solutiona and b are unit vectors along OA, OB and OC bisects the angle AOB. The unit vector along OC is
View Solution