A physical pendulum is positioned so that its centre of gravity is above the suspension point. From that position the pendulum started moving toward the stable equilibrium and passed it with an angular velocity ω. Neglecting the friction find the period of small oscillations of the pendulum.
Let l = distance between the C.G.C of the pendulum and its point of suspension o originally the pendulum is in inverted position and its C.G. is above o. when it falls to the normal stable position of equilibrium its C.G.has fallen by a distance 2l. In the equilibrium position the total energy is equal to K.E. = 1/2Iω2 and we have from energy conservation :Angular frequency of oscillation for a physical pendulum is given by ω20 = mgl/I
Related Solutions
A physical pendulum is positioned so that its centre of gravity is above the suspension point. When the pendulum is realsed it passes the point of stable equilibrium with an angular velocity . The period of small oscollations of the pendulum is
View SolutionA physical pendulum is positioned so that its centre of gravity is above the suspension point. When the pendulum is realsed it passes the point of stable equilibrium with an angular velocity omega . The period of small oscollations of the pendulum is
View SolutionA particle pendulum is positioned such that is centre of gravity is vertically above suspension point. From that position the pendulum started moving tward the stable equilibroum and passed it with an angular velocity . By neglecting the friction at point of suspension, the period of small oscillations of the pendulum is
View SolutionWhen the point of suspension of a pendulum is moved. Its period of oscillation :
View SolutionTime period of a simple pendulum is 2s and it can go to and fro from equilibrium position at a maximum distance of 6cm. If at the start of the motion the pendulum is in the position of maximum displacement towards the right of the equilibrium position, then write the displacement equation of the pendulum.
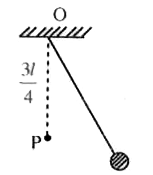
View SolutionA pendulum has time period T for small oscillations. An obstacle P is situated below the point of suspension O at a distance . The pendulum is released from rest. Throughout the motion the moving string makes small angle with vertical. Find the time after which the pendulum returns back to its initial position.
 View Solution
View SolutionAssertion :- For a physical pendulum if distance of point of suspension from centre of mass increases time period first decreases then increases.
Reason :- For a physical pendulum there is some distance from centre of mass at which frequency of oscillation is maximum.View SolutionA simple pendulum has time period . Charges are now fixed at the point of suspension of the pendulum and on the bob. If the pendulum continues to oscillate, its time period will now be
View SolutionA pendulum has time period T for small oscillations. An obstacle P is situated below the point of suspension O at a distance (3l)/4 . The pendulum is released from rest. Throughout the motion, the moving string makes small angle with vertical. Time after which the pendulum returns back to its initial position is
View SolutionA pendulum has time period T for small oscillations. An obstacle P is situated below the point of suspension O at a distance (3l)/4 . The pendulum is released from rest. Throughout the motion, the moving string makes small angle with vertical. Time after which the pendulum returns back to its initial position is
View SolutionA pendulum is displaced to an angle from its equilibrium position, then it will pass through its mean position with a velocity v equal to
View SolutionA pendulum is displaced to an angle theta from its equilibrium position, then it will pass through its mean position with a velocity v equal to
View Solutionएक सरल लोलक का दोलनकाल 2 सेकण्ड है तथा यह सम्य स्थिति से अधिक -से -अधिक 5 सेमी इधर -उधर जा सकता है यदि गति के प्रारम्भ होने के समय लोलक सम्य स्थिति के दाहिनी ओर अधिकतम विस्थापन की स्थिति में हो तो लोलक ला विस्थापन समीकरण लिखिए
View Solutionएक सरल लोलक के धात्विक गोलक पर ऋणावेश है तथा लोलक का आवर्तकाल T है | यदि इसे धनावेशित धात्विक प्लेट के ऊपर दोलित किया जाये तो इसका आवर्तकाल होगा
View SolutionA simple pendulum of mass m and charge +q is suspended vertically by a massless thread of length l. At the point of suspension, a point charge +q is also fixed. If the pendulum is displaced slightly from equilibrium position, its time period will be
View SolutionA second pendulum is shifted 4 cm away from its equilibrium position and then released. After 2s the pendulum is 3 cm away from its position of equilibrium. What will be the position of the pendulum after another 2 s?
View Solutionलम्बाई l के सरल लोलक को क्षैतिज स्थिति से छोड़ने पर दोलन करते समय उसके धागे में तनाव ज्ञात कीजिए जबकि लोलक क्षैतिज स्थिति में है ।
View Solutionएक सरल लोलक में एक धातु का लोलक इस्तेमाल किया गया है जो ऋणावेशित है। यह एक धनावेशित प्लेट के ऊपर दोलन करता है तो इसका आवर्तका
View SolutionA neutral pendulum oscillates in a uniform electric field as shown in figure. If a positive charge is given to the pendulum, then its time period
View SolutionA neutral pendulum oscillates in a uniform electric field as shown in figure. If a positive charge is given to the pendulum, then its time period
View Solution
