P is a point on the bisector of an angle ∠ABC. If the line through P parallel to AB meets BC at Q prove that triangle BPQ is isosceles.
Given that P is a point on the bisector of an angle ΔABC and PQ||AB.We have to prove that ΔBPQ is isoscelesSince BP is bisector of ∠ABC⇒ ∠ABP=∠PBC ......1
Related Solutions
P is a point on the bisector of an angle angleABC . If the line through P parallel to AB meets BC at Q. prove that triangle BPQ is isosceles.
View Solutionis a point on the bisector of an angle If the line through parallel to meets at , prove that triangle is isosceles.
View Solutionis a point on the bisector of .If the line through , parallel to meet at ,prove that is an isosceles triangle.
View SolutionP is a point on the bisector of .If the line through P,parallel to BA meet at Q ,prove that BPO is an isosceles triangle.
View SolutionIn triangle ABC, D is mid-point of AB and P is any point on BC. If CQ parallel to PD meets AB at Q, prove that:
View SolutionIn the figure, triangle ABC ~ triangle BPQ . If the line AB = BC and P is the midpoint of BC, then A(triangle ABC) : A(triangle BPQ) =
View SolutionIn triangle ABC, P is the mid-point of side BC. A line through P and parallel to CA meets AB at point Q and a line through Q and a line through Q and parallel to BC meets median AP at point R. Prove that :
.View Solutionis a point on the bisector of an angle If the line through parallel to meets at , prove that triangle is isosceles.
View Solutionकोण के समद्विभाजक पर स्थित एक बिन्दु P है | यदि P से AB से के समान्तर एक रेखा BC को Q पर काटती है, तो सिध्द करो कि PBQ त्रिभुज समद्विबाहु है |
View SolutionIn triangle ABC, P is the mid-point of side BC. A line through P and parallel to CA meets AB at point Q and a line through Q and parallel to BC meets median AP at point R. Prove that :
View SolutionIn triangleABC,D is the mid-point of AB and P is point on BC. If CQ||PD meets AB in Q then prove that : ar(BPQ) = 1/2 ar(ABC)
View SolutionAn isosceles triangle ABC, with AB = AC, circumscribes a circle, touching BC at P, AC at Q and AB at R. Prove that the contact point P bisects BC.
View SolutionIn the given figure, in triangle ABC, D is the mid-point cf AB and P is any point on BC. If CQ || PD meets AB in Q and area of triangle ABC = 30 cm2, then, area of triangle BPQ is
View SolutionThe bisector of of an isosceles triangle with meets the circumcircle of at as shown in Figure. If produced meet at . prove that
View SolutionIn ABC, the bisectors of B and C intersect at I. A line drawn through I and parallel to BC intersects AB at P and AC at Q. Prove that PQ = BP + CQ.
View SolutionIn ABC, the bisectors of B and C intersect at I. A line drawn through I and parallel to BC intersects AB at P and AC at Q. Prove that PQ = BP + CQ.
View SolutionIn the attached figure, Delta ABC D is the mid point of side AB and P is any point on side BC. CQ || PD intersects AB at Q. prove area Delta BPQ = (1)/(2) Area Delta ABC
View SolutionIn the parallelogram , the line drawn through point on , parallel to meets at . The line through , parallel to meets .
Prove that
.
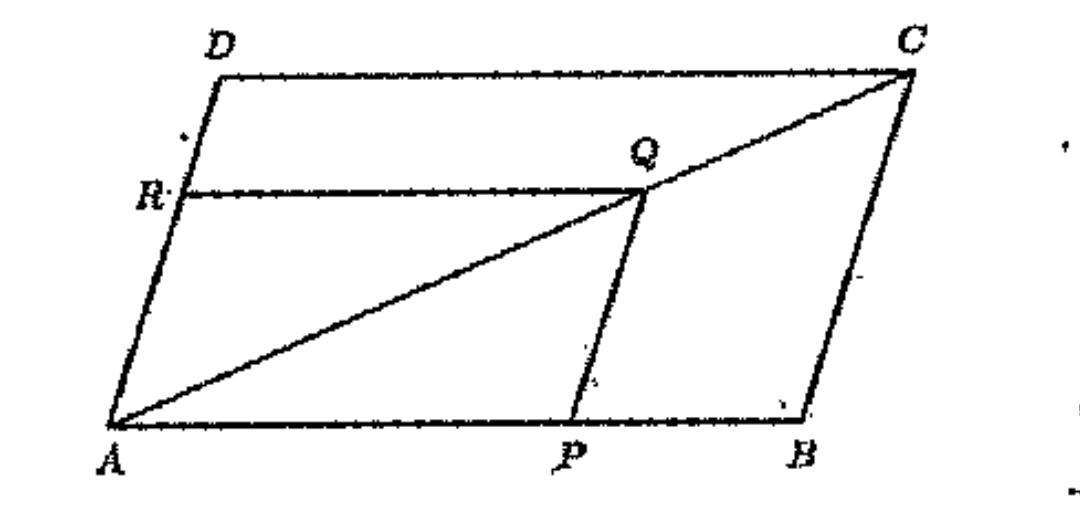.View Solutionबगल की आकृति में ,D भुजा AB का मध्य बिंदु है| P ,BC पर कोई बिंदु है | CQ ,PD के समांतर ही तथा भुजा AB को Q पर प्रतिच्छेद करती है|
सिद्ध कीजिए की
View Solutionबिंदु P और Q क्रमश : त्रिभुज ABC की भुजा AB और BC पर स्थित हैं, त्रिभुज B पर समकोण है
View Solution