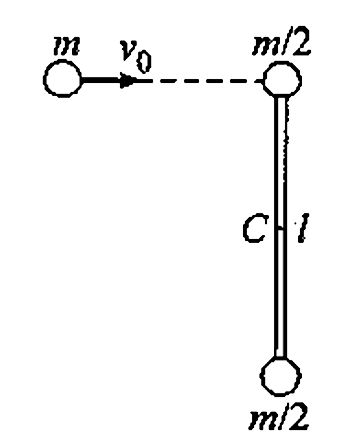
A ball of mass m moving with velocity vo experiences a head-on elastic collision with one of the spheres of a stationary rigid dumbbell as whown in Fig. 1.50. The mass of each sphere equals m/2 and the distance between them is l. Disregarding the size of the spheres find the proper angular momentum M of the dumbbell after the collision i.e. the angular momentum in the reference frame moving translationally and fixed to the dumbbells centre of inertia.
From conservation of linear momentum along the direction of the incident ball for the system consists with colliding ball and phheremv0=mv+m/2v1 1 where v and v1 are the velocities of ball and sphere 1 respectively after collision. Remember that the collision is head on. As the collision is perfectly elastic from the definition of co-efficient of restitution
Related Solutions
A ball of mass m moving with velocity v_0 experiences a head-on elastic collision with one of the spheres of a stationary rigid dumbbell as whown in figure. The mass of each sphere equals m//2 , and the distance between them is l . Disregarding the size of the spheres, find the proper angular momentum overset~M of the dumbbell after the collision, i.e. the angular momentum in the reference frame moving translationally and fixed to the dumbbell's centre of inertia.
View SolutionA ball ofmass m moving with velocity experiences a head on elastic collision with one of the spheres of as tationary rigid dumbbell as shown in figure-5.149. The mass of each sphere equals m2, and the distance between them is l.Disregarding the size of the spheres, find the angular momentum of the dumbbell in the reference frame moving translationally and fixed to the centre of mass of the dumbbell.
View SolutionA sphere of mass M,, moving with a velocity V0 collides head on with a stationary sphere of mass M. The collision is elastic. V1 and V2 are respectively their velocities immediately after collision.
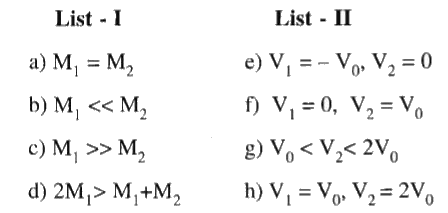
View SolutionA sphere of mass m moving with a constant velocity v collides with a stationary sphere of the same mass. If the coefficient of reposition is, then the ratio of the velocities of the two spheres after the collision will be.
View Solutionm द्रव्यमान का एक गोला नियत वेग से गतिशील है तथा समान द्रव्यमान के अन्य स्थिर गोले पर टकराता है। यदि e प्रत्यास्थता गुणांक है, तब संघट्ट के बाद गोले के वेग का अनुपात होगा
View SolutionA moving sphere of mass m suffer a perfect elastic collision (not head on) with equally massive stationary sphere. After collision both fly off at angle theta , value of which is :
View SolutionA moving sphere of mass m suffer a perfect elastic collision (not head on) with equally massive stationary sphere. After collision both fly off at angle , value of which is :
View SolutionA uniform solid sphere of mass m, radius R moving with velocity is rolling without slipping on a frictionless surface vertical wall. Ratio of magnitude of angular momentum of the sphere and after the collision about its bottommost point is
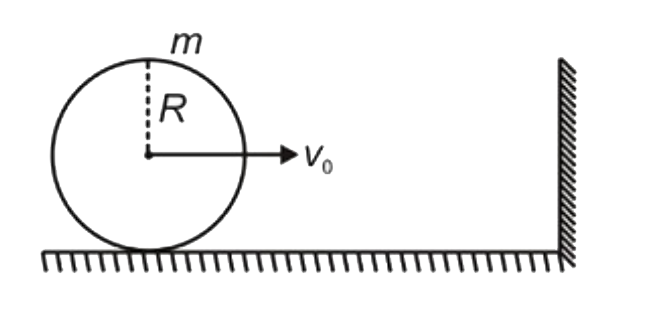
View SolutionA uniform solid sphere of mass m, radius R moving with velocity is rolling without slipping on a frictionless surface vertical wall. Ratio of magnitude of angular momentum of the sphere and after the collision about its bottommost point is

View SolutionWhen two spheres of equal masses undergo glancing elastic collision with one of them at rest after collision they will move
View SolutionWhen two spheres of equal masses undergo glancing elastic collision with one of them at rest after collision they will move
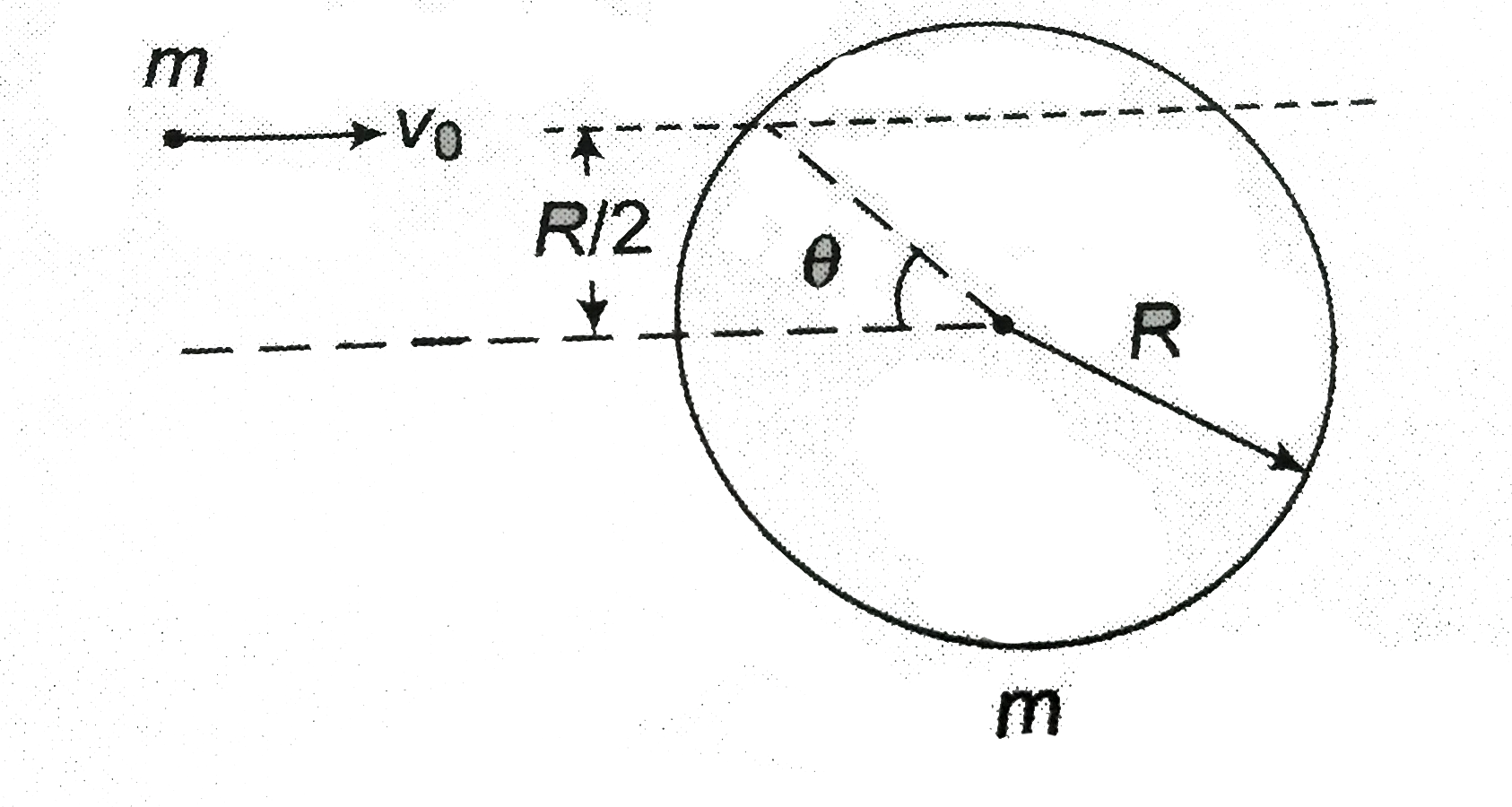
View SolutionA particle of mass moving with velocity collides with sphere of same mass at rest , as shown. If the surface of contact is smooth and the collision is elastic, find the velocities of particle and sphere after the collision.
View SolutionA sphere moving with speed and rotating With angular velocity makes a head-on elastic collision with an identical stationary sphere . There is no friction between the surfaces of and . Choose the conrrect alternative(s). Disregard gravity.
View SolutionA sphere moving with speed and rotating With angular velocity makes a head-on elastic collision with an identical stationary sphere . There is no friction between the surfaces of and . Choose the conrrect alternative(s). Disregard gravity.
View SolutionA sphere A moving with a speed u and rotating with an angular velocity omega , makes a head-on elastic collision with an identical stationary sphere B. There is no friction between the surfaces of a and B. Disregard gravity.
View SolutionA sphere of mass M moving with velocity w collides elastically with another of mass m at rest. After collision their final velocities are V and v. The value of vis:
View SolutionStatement I: If a sphere of mass m moving with speed u undergoes a perfectly elastic head-on collision with another sphere of heavier mass M at rest ( M gt m ), then direction of velocity of sphere of mass m is reversed due to collision (no external force acts on system of two spheres). Statement II: During a collision of spheres of unequal masses, the heavier mass exerts more force on the lighter mass in comparison to the force which lighter mass exerts on the heavier one,
View SolutionStatement I: If a sphere of mass m moving with speed u undergoes a perfectly elastic head-on collision with another sphere of heavier mass M at rest ( M gt m ), then direction of velocity of sphere of mass m is reversed due to collision (no external force acts on system of two spheres). Statement II: During a collision of spheres of unequal masses, the heavier mass exerts more force on the lighter mass in comparison to the force which lighter mass exerts on the heavier one,
View SolutionStatement I: If a sphere of mass moving with speed undergoes a perfectly elastic head-on collision with another sphere of heavier mass at rest (), then direction of velocity of sphere of mass is reversed due to collision (no external force acts on system of two spheres).
Statement II: During a collision of spheres of unequal masses, the heavier mass exerts more force on the lighter mass in comparison to the force which lighter mass exerts on the heavier one,
View SolutionA ball 'A' of mass 'm' moving along positive x-direction with kinetic energy K and linear momentum "p" undergoes elastic head on collision with a stationary ball 'B' of mass M . After the collision, the ball A moves along negative x-direction with kinetic energy K//9 , the final linear momentum of the ball B is
View Solution