A homogeneous block with a mass hangs on three vertical wires of equal length arranged symmetrically. Find the tension of the wires if the middle wire is of steel and the other two are of copper. All the wires have the same cross section. Consider the modulus of elasticity of steel to be double than of copper.
Solving these two equations we get
Related Solutions
A homogeneous block with a mass hangs on three vertical wires of equal length arranged symmetrically . Find the tensions of the wires if the middle wire is of steel and the other two are of copper . All the wires have the same cross - section . Consider the modulus of elasticity of steel to be double than that of copper .
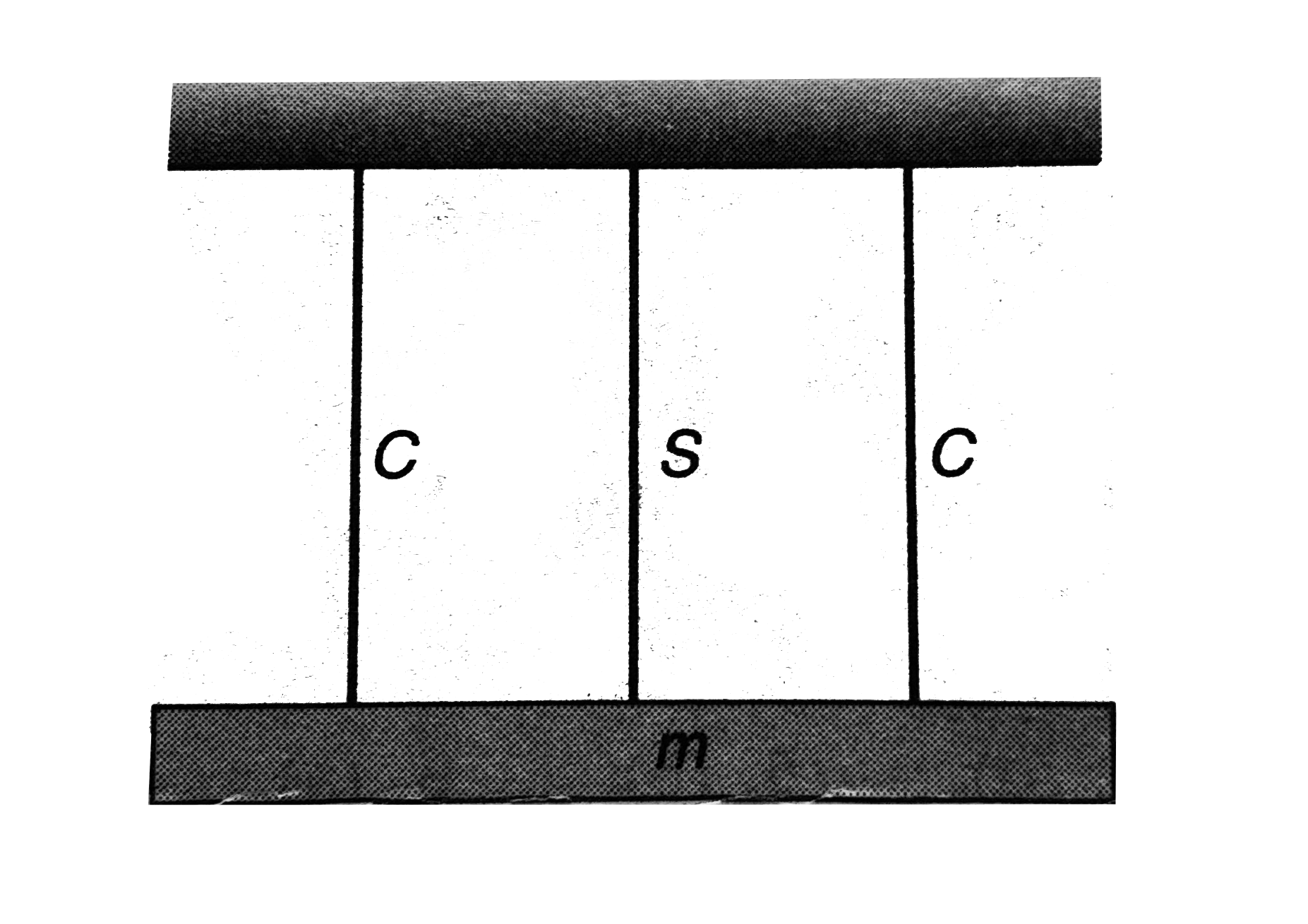
View SolutionA homogeneous block with a mass hangs on three vertical wires of equal length arranged symmetrically . Find the tensions of the wires if the middle wire is of steel and the other two are of copper . All the wires have the same cross - section . Consider the modulus of elasticity of steel to be double than that of copper .

View SolutionA heavy plank of mass 100 kg hangs on three vertical wires of equal length arranged symmetrically (see figure). All the wires have the same cross - section. The middle wire is of steel and the other two are of copper . The modulus of elasticity of steel is assumed to be double that copper. Determine the tensions in the wire. (use g=9.8ms^-2 )
View SolutionA heavy plank of mass 100 kg hangs on three vertical wires of equal length arranged symmetrically (see figure). All the wires have the same cross - section. The middle wire is of steel and the other two are of copper . The modulus of elasticity of steel is assumed to be double that copper. Determine the tensions in the wire. (use g=9.8ms^-2 )
View SolutionTwo wires of same radius and length are subjected to the same load. One wire is of steel and the other is of copper. It the Young's modulus of steel is twice that of copper, the ratio of elastic energy stored per unit volume in steel to that of copper wire is
View SolutionTwo wires of same radius and legth are subjected to the same load . One wire is of steel an the other is of copper . If the young modulus of steel is twice that of copper find the ratio of elastic energy stored per unit volume in steel to that of copper .
View SolutionTwo wires of equal cross section but one made of steel and the other of copper, are joined end to end. When the combination is kept under tension, the elongations in the two wires are found to be equal. Find the ratio of the lengths of the two wires. Young modulus of steel and that of copper
View SolutionTwo wires of equal cross section but one made of steel and the other of copper, are joined end to end. When the combination is kept under tension, the elongations in the two wires are found to be equal. Find the ratio of the lengths of the two wires. Young modulus of steel and that of copper
View SolutionTwo wires of equal cross section but one made of steel and the other of copper, are joined end to end. When the combination is kept under tension, the elongations in the two wires are found to be equal. Find the ratio of the lengths of the two wires. Young modulus of steel and that of copper
View SolutionTwo wire of same radius and lengh the are subjected to the same load. One wire is of steel and the other is of copper. If the Young's modulus of steel is twice that of copper, the ratio the energy stored per unit volume in steel to that of copper wire is
View SolutionTwo wires, out of copper and the other of steel, are of same length and cross section. They are welded together to from a long wire. On suspending a weight at its one end, increment in length is found to be 3 cms. If Young's modulus of steel is double that of copper the increment in steel wire will be
View SolutionThe radius of a copper wire is twice that of a steel wire. One end of the copper wire is joined with one end of the steel wire and the same tensile force is applied on them . If the length of the copper wire increases by 1% , what will be the percentage increase in the length of the steel wire? Given Young's modulus of steel is twice that of copper.
View SolutionIn figure the upper wire is made of steel and the lower of copper. The wires have equal cross section. Find the ratio of the longitudinals strains developed in the two wires.

View SolutionIn figure the upper wire is made of steel and the lower of copper. The wires have equal cross section. Find the ratio of the longitudinal strains developed in the two wires.

View SolutionIn figure the upper wire is made of steel and the lower of copper. The wires have equal cross section. Find the ratio of the longitudinal strains developed in the two wires.

View SolutionA copper wire and a steel wire of the same length and same cross-section are joined end to end to form a composite wire. The composite wire is hung form a rigid support and a load is suspended form the other end. If the increses in length of the composite wire is . then the increases in lenghts of steel and copper wires are
View SolutionA steel wire and a copper wire of equal length and equal cross- sectional area are joined end to end and the combination is subjected to a tension. Find the ratio of copper/steel (a) the stresses developed . In the two wires, (b) the strains developed. (Y of steel = 2xx10^(11) N//m^(2) )
View SolutionA steel wire and a copper wire of equal length and equal cross- sectional area are joined end to end and the combination is subjected to a tension. Find the ratio of copper/steel (a) the stresses developed . In the two wires, (b) the strains developed. (Y of steel = 2xx10^(11) N//m^(2) )
View SolutionM द्रव्यमान की कोई दुढ़ छड़ तीन तारों, जिनमें प्रत्येक की लंबाई । है, पर सममित रूप से टिकी है। इनमें दोनों सिरों वाले तार कॉपर के तथा मध्य वाला तार आयरन का है। यदि प्रत्येक में तनाव समान रहता है, तो इन तारों के व्यासों का अनुपात बराबर है
View SolutionTwo copper wires A and B have the same mass. The resistance of wire A is 0.5 Omega and the length of wire B is double that of wire A. Find the resistance of wire B.
View Solution