Two wires of equal cross section but one made of steel and the other of copper, are joined end to end. When the combination is kept under tension, the elongations in the two wires are found to be equal. Find the ratio of the lengths of the two wires. Young modulus of steel =2.0×1011Nm−2 and that of copper =1.1×1011Nm−2Solution in Tamil
As the cross sections of the wires are equal and same tension exists in both the stresses developed are equal. Lelt the origiN/Al lengths of the steel wire and the copper wire be LsandLc respectively and the elongation in each wire be l.
lLs=stress2.0x1011nm−2............i
andlLc=stress1.1×101Nm−2............ii
Dividing ii by i
LsLc=2.01.1=20:11
Topper's Solved these Questions
- BOOK - HC VERMACHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Question For short Anser18videos
SOME MECHANICAL PROPERTIES OF MATTER
- BOOK - HC VERMACHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Objective-129videos
SOME MECHANICAL PROPERTIES OF MATTER
- BOOK - HC VERMACHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Exercises32videos
SOME MECHANICAL PROPERTIES OF MATTER
- BOOK - HC VERMACHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercises58videos
SIMPLE HARMONIC MOTION
- BOOK - HC VERMACHAPTER - SOUND WAVESEXERCISE - All Questions139videos
SOUND WAVES
Similar Questions
The ratio stress/strain remains constant for small deformation of a metal wire. When the deformation is made larger, will this ratio increase or decrease ?
09:54View SolutionA rope 1 cm in diameter breaks if the tension in it exceeds 500 N. The maximum tension that may be given to a similar rope of diameter 2 cm is
05:59View SolutionA load of 10 kg is suspended by a metal wire 3 m long and having a cross sectional area 4mm2. Find a. the stress b. the strain and c. the elongation. Young modulus of the metal is2.0×1011Nm−2
06:56View SolutionA particle executes simple harmonic motion with an amplitude of 10 cm and time period 6s. At t=0 it is at position x=5 cm going towards positive x-direction. Write the equation for the displacement x at time t. Find the magnitude of the acceleration of the particle at t=4s.
02:37View SolutionA wave of wavelength 0.60 cm is produced in air and it travels at a speed of 300 ms^-1. Will it be audible?
04:32View SolutionA steel wire and a copper wire of equal length and equal cross sectionasl area area joined end to end and the combination is subjected to as tension. Find the ratio of as. The stresses developed in the two wire and b. the strains developed. Y of steel =2×1011Nm−2. Y o copper =1.3×1011Nm−2.
03:54View SolutionIn figure the upper wire is made of steel and the lower of copper. The wires have equal cross section. Find the ratio of the longitudinals strains developed in the two wires.
 04:37View Solution
04:37View SolutionA copper wire of cross sectional asrea 0.01 cm is under a tension of 20 N. Find the decrease in the cross sectional area. Young modulus of copper =1.1×1011Nm−2 and Poisson ratio 0.32.
07:09View SolutionA steel wire and a copper wire of equal length and equal cross- sectional area are joined end to end and the combination is subjected to a tension. Find the ratio of copper/steel (a) the stresses developed . In the two wires, (b) the strains developed. (Y of steel = 2xx10^(11) N//m^(2) )
05:20View SolutionA homogeneous block with a mass m hangs on three vertical wires of equal length arranged symmetrically . Find the tensions of the wires if the middle wire is of steel and the other two are of copper . All the wires have the same cross - section . Consider the modulus of elasticity of steel to be double than that of copper .
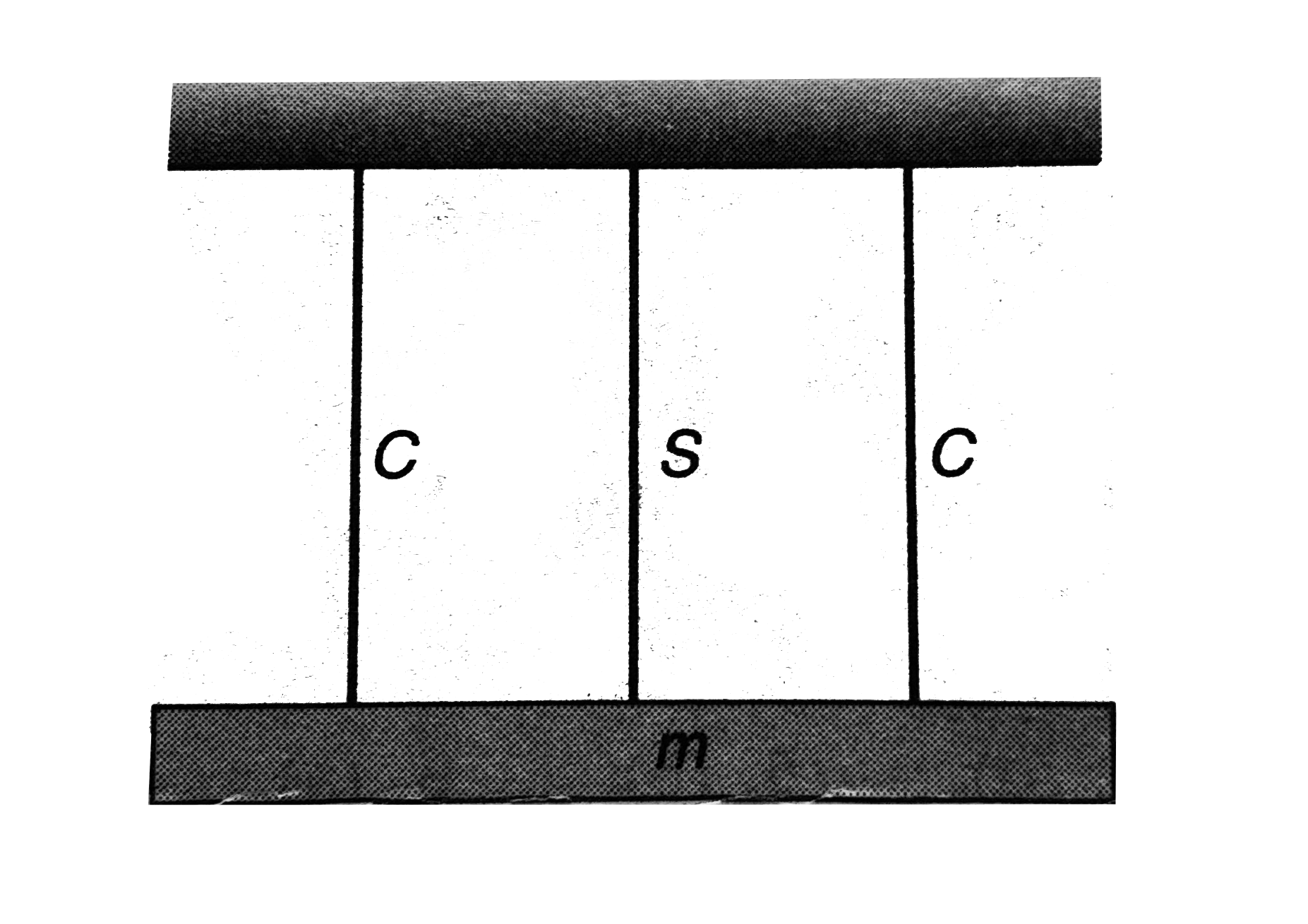 02:30View Solution
02:30View SolutionA copper wire of length 2.2m and a steel wire of length 1.6m, both of diameter 3.0mm are connected end to end. When stretched by a force, the elongation in length 0.50 mm is produced in the copper wire. The streching force is (Ycopper=1.1×1011Nm−2,
Ysteel=2.0×1011Nm−2)02:35View SolutionTwo wires of equal cross-section but one made of steel and the other of copper are joined end to end. When the cobination is kept under tension, the elongations in the two wires are found to be equal elongations in the two wire are found to be equal. What is the ratio of the lengths of the two wires? (Given, Young's modulus of steel = 2 xx 10^(11) Nm^(-2) and young's modulus of copper = 1.1 xx 10^(11) Nm^(-2) )
04:32View SolutionTwo wires, out of copper and the other of steel, are of same length and cross section. They are welded together to from a long wire. On suspending a weight at its one end, increment in length is found to be 3 cms. If Young's modulus of steel is double that of copper the increment in steel wire will be
03:06View SolutionThe radius of a copper wire is twice that of a steel wire. One end of the copper wire is joined with one end of the steel wire and the same tensile force is applied on them . If the length of the copper wire increases by 1% , what will be the percentage increase in the length of the steel wire? Given Young's modulus of steel is twice that of copper.
02:52View SolutionA steel wire and a copper wire of equal length and equal cross sectional area are joined end to end and the combination is subjected to a tension. Find the ratio of a. The stresses developed in the two wire and b. the strains developed. Y of steel =2×1011Nm−2. Y of copper =1.3×1011Nm−2.
05:28View SolutionIn figure the upper wire is made of steel and the lower of copper. The wires have equal cross section. Find the ratio of the longitudinal strains developed in the two wires.
 04:03View Solution
04:03View Solution
