A solid cylinder of mass m and radius r starts rolling down an inclined plane of inclination $θ$ . Friction is enough to prevent slipping. Find the speed of its centre of mass when its centre of mass has fallen a height $h$ .

Video Solution

Text Solution

Verified by Experts

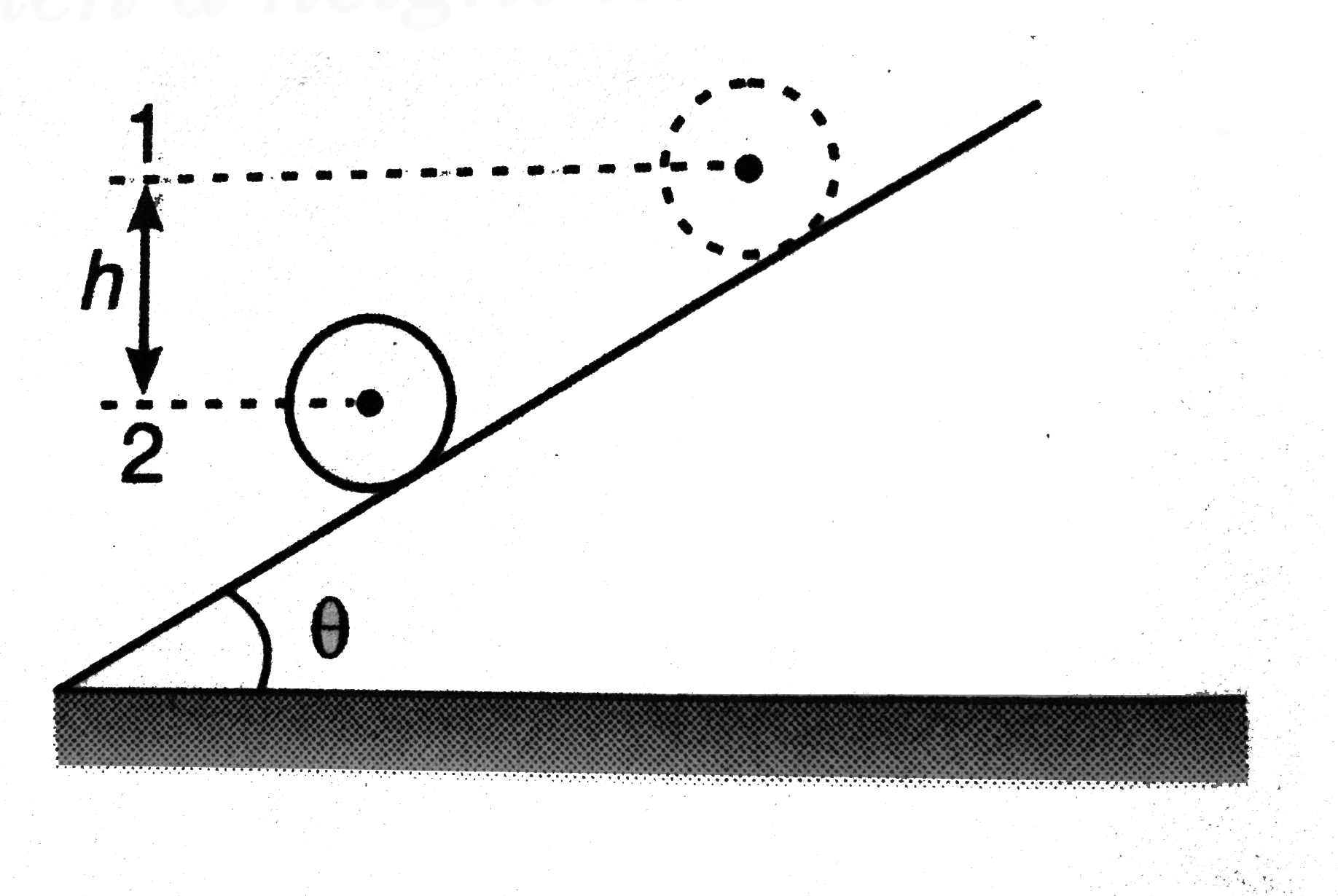
Considering the two shown position of the cylinder. As it does not slip hence total mechanical energy will be conserved.
energy at position 1 is $E_{1} = m g h$
Energy at position 2 is $E_{2} = \frac{1}{2} m v_{C O M}^{2} + \frac{1}{2} I_{C O M} ω^{2}$
$\frac{v_{C O M}}{r} = ω$ and $I_{C O M} = \frac{m r^{2}}{2}$
$\Rightarrow E_{2} = \frac{3}{4} m v_{C O M}^{2}$
From conservation of energy $E_{1} = E_{2}$ or $m g h = \frac{3}{4} m v_{C O M}^{2}$
$\Rightarrow v_{C O M} = \sqrt{\frac{4}{3} g h}$

|

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One or More
A solid cylinder of mass $m$ and radius $r$ is rolling on a rough inclined plane of inclination $θ$ . The coefficient of friction between the cylinder and incline is $μ$ . Then.
Africtional force is always $μ m g cos θ$
Bfriction is a dissipative force
Cby decreasing $θ$ , frictional force decreases
Dfrictiona opposes translation and supports rotation.
Question 2 - Select One
A solid cylinder of mass M and radius R rolls without slipping down on inclined plane of length L and height h what is the speed of its centre of mass when the cylinder reaches its bottom?
A $\sqrt{\frac{4}{3} g h}$
B $\sqrt{4 g h}$
C $\sqrt{2 g h}$
D $\sqrt{\frac{3}{4} g h}$
Question 3 - Select One
A solid cylinder of mass M and radius R rolls without slipping down on inclined plane of length L and height h what is the speed of its centre of mass when the cylinder reaches its bottom?
A $\sqrt{\frac{4}{3} g h}$
B $\sqrt{4 g h}$
C $\sqrt{2 g h}$
D $\sqrt{\frac{3}{4} g h}$

DC PANDEY-ROTATIONAL MECHANICS-Solved Examples

Home
Ask Doubt
Profile