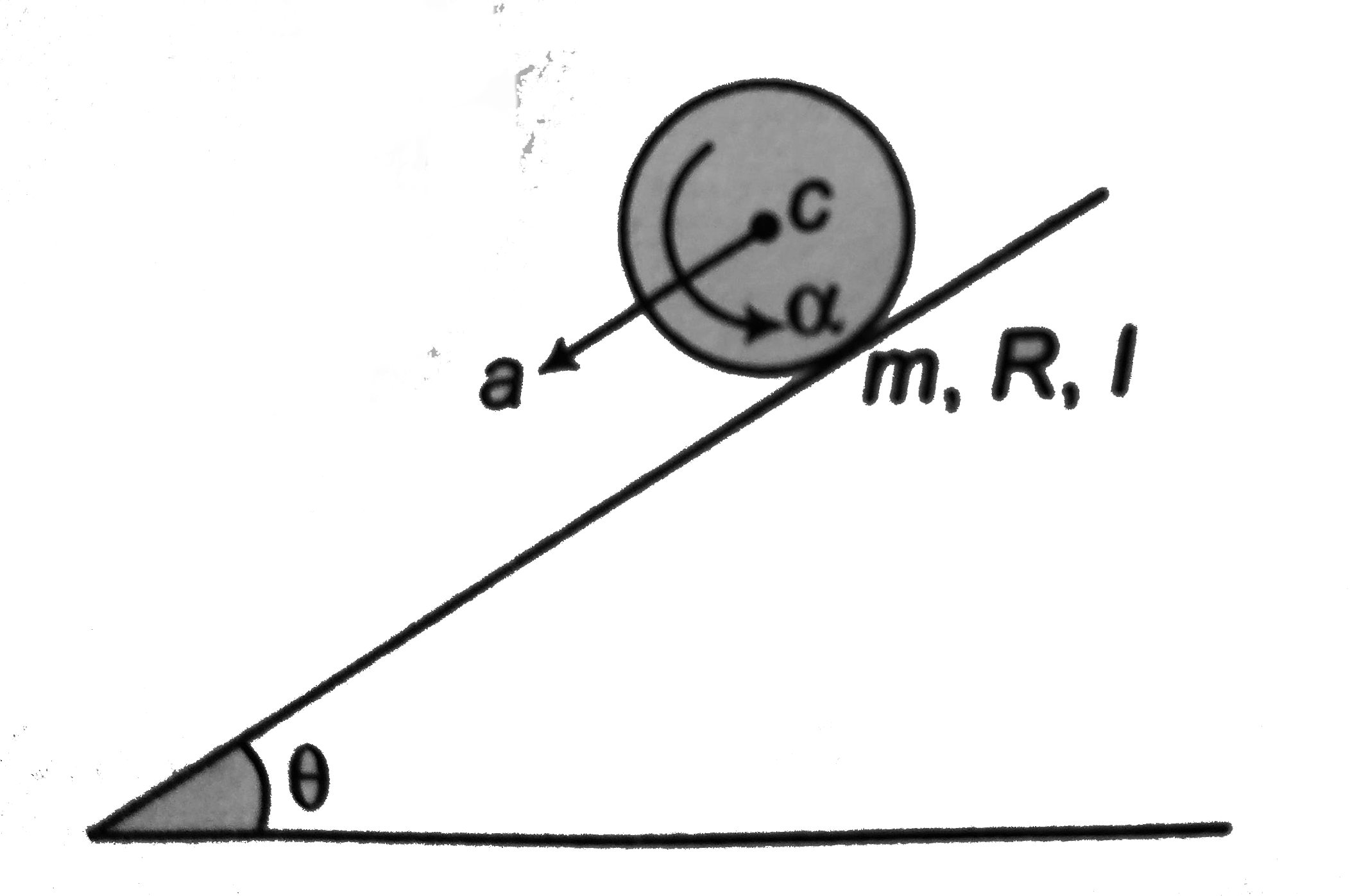
A body of mass $m$ , radius $R$ and moment of inertia $I$ (about an axis passing through the centre of mass and perpendicular to plane of motion) is released from rest over a sufficiently rough ground (to provide accelerated pure rolling) find linear acceleration of the body.

Video Solution

Text Solution

Verified by Experts

Let linear acceleration is $a$ and angular acceleration $α$ for accelerated pure rolling
$α = \frac{a}{R}$
After time t, displacement of centre of mass along the plane $s = \frac{1}{2} a t^{2}$
$∴$ height fallen by centre of mass along the plane $s = \frac{1}{2} a t^{2}$
$∴$ height fallen by centre of mass
$h = (s) (sin θ) = \frac{1}{2} a t^{2} sin θ$
Linear velocity $v = a t$
angular velocity $ω = α t = \frac{a t}{R}$
From energy conservation principle, decrease in potential energy $=$ increase in translational and rotaional kinetic energy
or $m g h = \frac{1}{2} m v^{2} + \frac{1}{2} I ω^{2}$
Substituting the value we have
$m g (\frac{1}{2} a t^{2} sin θ) = \frac{1}{2} m {(a t)}^{2} + \frac{1}{2} I {(\frac{a t}{R})}^{2}$
Solving this equation we get $a = \frac{g sin θ}{1 + \frac{I}{m R^{2}}}$

Updated on:21/07/2023

Moment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
A $1 / 2 M R^{2}$
B $M R^{2}$
C $1 / 4 M R^{2}$
D $3 / 4 M R^{2}$
Moment of inertia of a uniform quarter disc of radius $R$ and mass $M$ about an axis through its centre of mass and perpendicular to its plane is $:$
A $\frac{M R^{2}}{2} - M {(\frac{4 R}{3 π})}^{2}$
B $\frac{M R^{2}}{2} - M {(\sqrt{2} \frac{4 R}{3 π})}^{2}$
C $\frac{M R^{2}}{2} + M {(\frac{4 R}{3 π})}^{2}$
D $\frac{M R^{2}}{2} + M {(\sqrt{2} \frac{4 R}{3 π})}^{2}$
For the same total mass, which of the following will have the largest moment of inertia about an axis passing through the centre of mass and perpendicular to the plane of the body
Aa disc of radius R
Ba ring of radius R
Ca square lamina of side 2 R
Dfour rods forming a square of side 2R