Three concentric, thin, spherical, metallic shells have radii 1, 2, and 4 cm and they are held at potentials 10,0 and 40V respectively. Taking the origin at the common centre, calculate the following:
(a) Potential at r = 1.25 cm
(b) Potential at r = 2.5 cm
(c) Electric field at r =1.25 cm
The correct Answer is:A, B
Let q1:q2 and q3 be the respective charges. Then
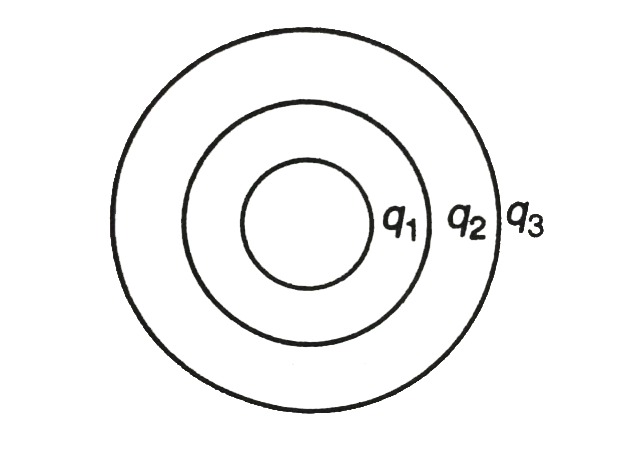
=10=9×10910−2[q11+q22+q34]
=0(9xx10^9)/10^-2[q_1/2+q_2/2+q_3/4]<br>and40=(9xx10^9)/10^-2[q_1/4+q_2/4+q_3/4]<br>solv∈gtheseequationswe≥t<br>q_1=+200/9x10^-12C,q_2=-200xx10^-12andq_3=3200/9xx10^-2C<br>a.Atr=1.25cm<br>V=(9xx10^9)/10^-2[((200/9)x10^-12)/1.25(200xx10^-12)/2+((3200/9)x10^-12)4]<br>=6V<br>b.Potentialatr=2.5 cm<br>V=(9xx10^9)/10^-2[((200/9)xx10^I-12)/2.5-(200xx10^-12)/2.5+((3200/9)xx10^-12)/4]<br>=16V<br>c.E≤ctricfieldatr=1.25 cm will be due to charge q1 only
∴E=14πε0.q1r2 ltbr> =⎛⎜
⎜⎝9×109×(2009)×10−12(1.25×10−2)2
=1.28×103Vm
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Check point 1.1
10 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Check point 1.2
15 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Subjective
15 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEYCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - All Questions
120 Videos
Similar Questions
Two concentric shells have masses M and m and their radii are R and r, respectively, where R>r. What is the gravitational potential at their common centre?
View SolutionThree concentric charged metallic spherical shells A, B and C have radii a,b and c, charge densities σ,−σ and σ and potentials VA,VB and VC respectively. Then which of the following relations is correct?
View Solution
Knowledge Check
Two concentric shells have masses M and m and their radii are R and r, respectively, where R>r. What is the gravitational potential at their common centre?
A−GMRB−GMrC−G[MR−mr]D−G[MR+mr]Three concentric charged metallic spherical shells A, B and C have radii a,b and c, charge densities σ,−σ and σ and potentials VA,VB and VC respectively. Then which of the following relations is correct?
 AVA=(a+b+c)σε0BVB=([(a2/b)−b+c])σε0CVC=[(a2+b2)/c+c]σε0DVA=VB=VC=(a−b+c)σε0
AVA=(a+b+c)σε0BVB=([(a2/b)−b+c])σε0CVC=[(a2+b2)/c+c]σε0DVA=VB=VC=(a−b+c)σε0Two concentric spherical shells of radii R and 2R have charges Q and 2Q as shown in figure

If we draw a graph between potential V and distance r from the centre, the graph will be likeA B
B C
C D
D
There are two concentric conducting spherical shells of radii 10cm and 20cm. The outer shell is charged to a potential of 50esu while inner shell is earthed. The charge on outer shell is
View SolutionThere are two concentric conducting spherical shells of radii 10 cm and 20 cm. The outer shell is charged to a potential of 50esu while inner shell is earthed. The charge on outer shell is
View SolutionThree concentric conducting spherical shells of radii R,2R carry charges Q, -2Q and 3Q, respectively. Find the electric potential at r = R and at r = 3 R, where r is the radii distance from the centre.

View SolutionThree conducting concentric spherical shells of radii R, 2R and 3R carry some charge on them. The potential at the centre is 50 V and that of middle and outer shell is 20 V and 10 V respectively. Find the potential of the inner Shell.
View SolutionTwo concentric spherical shells of radii R and 2R have charges Q and 2Q as shown in figure

If we draw a graph between potential V and distance r from the centre, the graph will be like
View Solution
