There are two concentric conducting spherical shells of radii 10cm and 20cm. The outer shell is charged to a potential of 50esu while inner shell is earthed. The charge on outer shell is
1000 esu
2000 esu
5 esu
1500 esu
To find the charge on the outer conducting spherical shell, we can follow these steps:
1. Understand the Configuration:
- We have two concentric spherical shells. The inner shell has a radius of r1=10cm and is earthed (grounded), meaning its potential is zero.
- The outer shell has a radius of r2=20cm and is charged to a potential of V=50ESU.
2. Use the Concept of Induction:
- Since the inner shell is earthed, it will have an induced charge on its surface due to the presence of the outer shell's charge. Let the charge on the outer shell be Q.
- The inner shell will have an induced charge of −Q on its surface.
3. Calculate the Potential:
- The potential V at the surface of the outer shell (radius r2) due to its own charge Q can be expressed as:
V=kQr2
- The potential at the inner shell (radius r1) due to the induced charge −Q is:
V′=k(−Q)r1
4. Set Up the Equation:
- Since the inner shell is earthed, the total potential at the inner shell must be zero:
V+V′=0
- Substituting the expressions for V and V′:
kQ20−kQ10=0
5. Simplify the Equation:
- Rearranging gives:
kQ20=kQ10
- This simplifies to:
Q20−2Q20=0⟹−Q20=0
- Therefore, we can express Q in terms of the total potential:
Q20=5020⟹Q=−25ESU
6. Total Charge on the Outer Shell:
- The total charge on the outer shell is the sum of the charge Q and the induced charge −Q:
Qtotal=Q+(−Q)=Q+Q=50ESU
Final Answer:
The charge on the outer shell is 50ESU.
- We have two concentric spherical shells. The inner shell has a radius of r1=10cm and is earthed (grounded), meaning its potential is zero.
- The outer shell has a radius of r2=20cm and is charged to a potential of V=50ESU.
- Since the inner shell is earthed, it will have an induced charge on its surface due to the presence of the outer shell's charge. Let the charge on the outer shell be Q.
- The inner shell will have an induced charge of −Q on its surface.
- The potential V at the surface of the outer shell (radius r2) due to its own charge Q can be expressed as:
V=kQr2
- The potential at the inner shell (radius r1) due to the induced charge −Q is:
V′=k(−Q)r1
- Since the inner shell is earthed, the total potential at the inner shell must be zero:
V+V′=0
- Substituting the expressions for V and V′:
kQ20−kQ10=0
- Rearranging gives:
kQ20=kQ10
- This simplifies to:
Q20−2Q20=0⟹−Q20=0
- Therefore, we can express Q in terms of the total potential:
Q20=5020⟹Q=−25ESU
- The total charge on the outer shell is the sum of the charge Q and the induced charge −Q:
Qtotal=Q+(−Q)=Q+Q=50ESU
The charge on the outer shell is 50ESU.
Topper's Solved these Questions
- TEST 1BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - TEST 1EXERCISE - EXERCISE
21 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Try Yourself
63 Videos
- TEST 2BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - TEST 2EXERCISE - Exercise
308 Videos
Similar Questions
The potential of outer shell is
View SolutionThere are two concentric hollow conducting spherical shells of radii r and R(R>r). The charge on the outer shell is Q. What charge should be given to the inner shell, so that the potential at a point P , at a distance 2R from the common centre is zero ?
View SolutionConsider two concentric spherical metal shells of radii r1 and r2(r2>r1). If the outer shell has a charge q and the inner one is grounded, then the charge on the inner shell is
View SolutionTwo concentric conducting spherical shells of radii a1anda2(a2>a1) are charged to potentials ϕ1 and ϕ2, respectively. Find the charge on the inner shell.
View SolutionConsider two concentric spherical metal shells of radii 'a' and b>a .The outer shell has charge Q but the inner shell has no charge.Now the inner shell is grounded ,This means that the inner shell will come at zero potential and that electric fields lines leave the outer shell and end on the inner shell.(a) Find the charge on the inner shell
Find the potential on outer sphere.
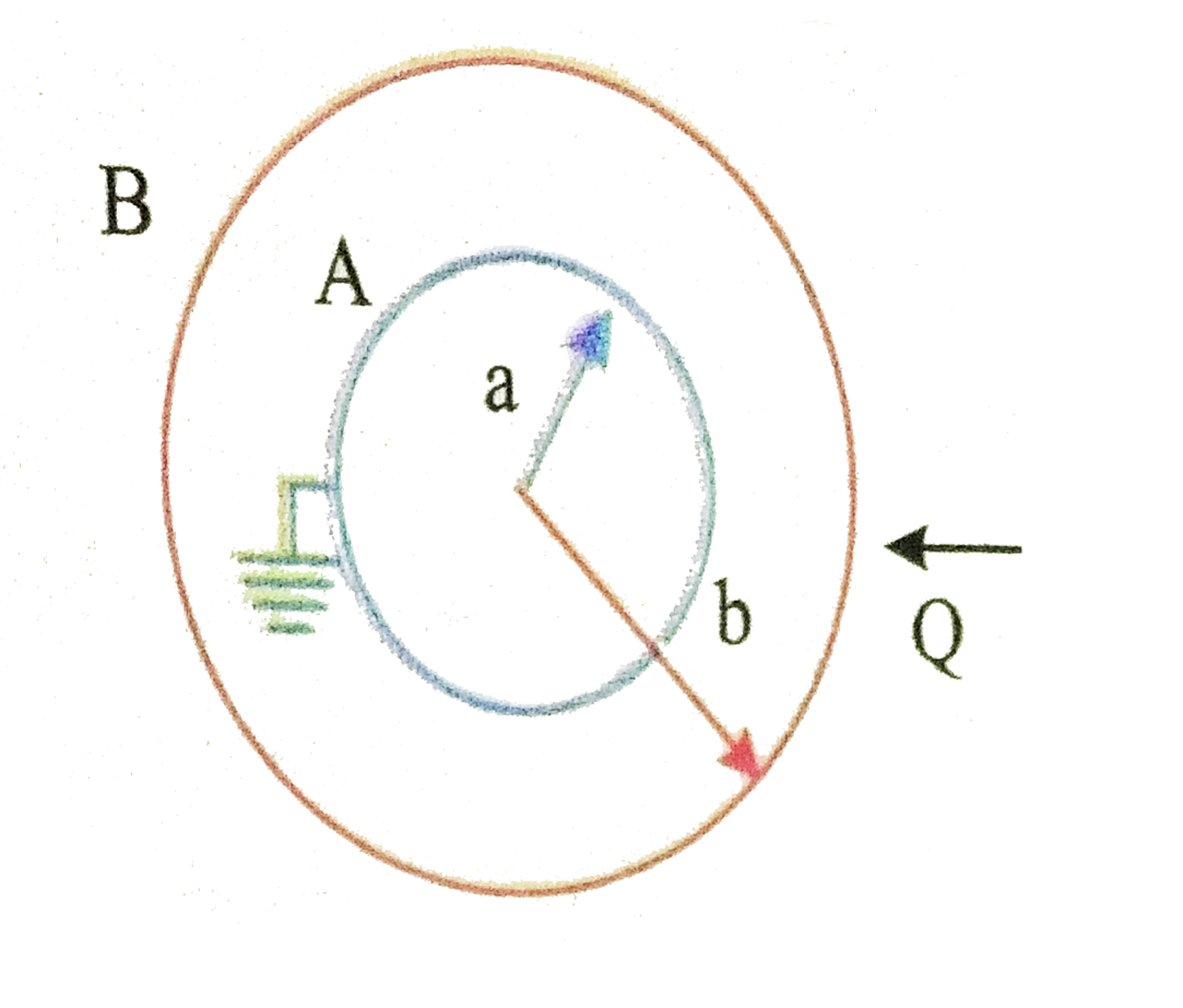
View Solutionthere are two concentric metallic shells of radii R and 2R, if outer shell is given charge q1 , then charge on surface of inner shell will be
View SolutionThere are two concentric conducting spherical shells of radius rA and rB respectively (rB=2rA). If the inner shell is given a charge Q and outer shell is given a charge 2Q, then the potential difference between the surfaces of the shells will be K=14πε0
View SolutionThere are two concentric metal shells of radii r1 and r2(>r1). If initially, the outer shell has a charge q and the inner shell is having zero charge and then inner shell is grounded. Find :
(i) Charge on the inner surface of outer shell.
(ii) Final charges on each sphere.
(iii) Charge flown through wire in the ground.
View SolutionFigure shows two conducting thin concentric shells of radii r and 3r. The outer shell carries charge q and the inner shell is neutral . The amount of charge that flows from the inner shell to the earth after the key K is closed is equal to (1/n)th of the charge on the outer shell. What is the value of n ?
 .
.
View SolutionThere are three conducting and concentricspherical shells of radii R,2R and 3R. The charge on inner and outer most shells are q and 3q while middle shell is earthed. Find the ratio of number of field lines emitted by outermost shell and inner shell.
View Solution
