An ideal diatomic gas is caused to pass throguh a cycle shown on the P−V diagram in figure, where V2=3.00V1. If P1,V1,and T1 specify the state 1, then the temperature of the state 3 is
(T1/3)1
(T1/3)4
(T1)(1/3)0.4
(T1/3)2
(T1/3)0.4
Topper's Solved these Questions
- BOOK - CENGAGE CHEMISTRYCHAPTER - THERMODYNAMICSEXERCISE - Ex 6.227videos
THERMODYNAMICS
- BOOK - CENGAGE CHEMISTRYCHAPTER - THERMODYNAMICSEXERCISE - Ex 6.327videos
THERMODYNAMICS
- BOOK - CENGAGE CHEMISTRYCHAPTER - THERMODYNAMICSEXERCISE - Archives (Subjective)23videos
THERMODYNAMICS
- BOOK - CENGAGE CHEMISTRYCHAPTER - STOICHIOMETRYEXERCISE - Archives Subjective33videos
STOICHIOMETRY
Similar Questions
The enthalpy changes for the following reactions at 298k and 1atm are given below:
a. CH3COOH(l)+2O2(g)→2CO2(g)+2H2O(l),ΔH=−874kJ
b. CH3CH2OH(l)+3O2(g)→2CO2(g)+3H2O(l),ΔH=−1363kJ
Calculate the internal enegry changes for these recaitons.05:14View SolutionA mixture of hydrocarbons containing acetylene and ethane, when burned under controlled consitions produced 16.20g of water and 139.7kcal of heat. Given the molar composition of the mixture. Standard heats of combustion for acetylene and ethane are, respectively, −310.6 and −373.8kcal.
03:49View SolutionThe enthalpies for the following reactions (ΔHΘ) at 25∘C are given below.
a. 12H2(g)+12O2(g)→OH(g)
ΔH=10.06kcal
b. H2(g)→2H(g),ΔH=104.18kcal
c. O2(g)→2O(g),ΔH=118.32kcal
Calculate the O−H bond energy in the hydroxyl radical.View SolutionIn the analysis of 0.1 of sample of feldspar, 0.118 g of mixture of NaCl and KCl is obtained which on treatment with AgNO3 gives 0.2451 of AgCl. Calculate the precentage of Na2O and K2O in feldspar.
View SolutionFor an ideal gas, an illustratio of three different paths A(B+C) and (D+E) from an initial state P1,V1,T1 to a final state P2,V2,T1 is shown in the given figure.
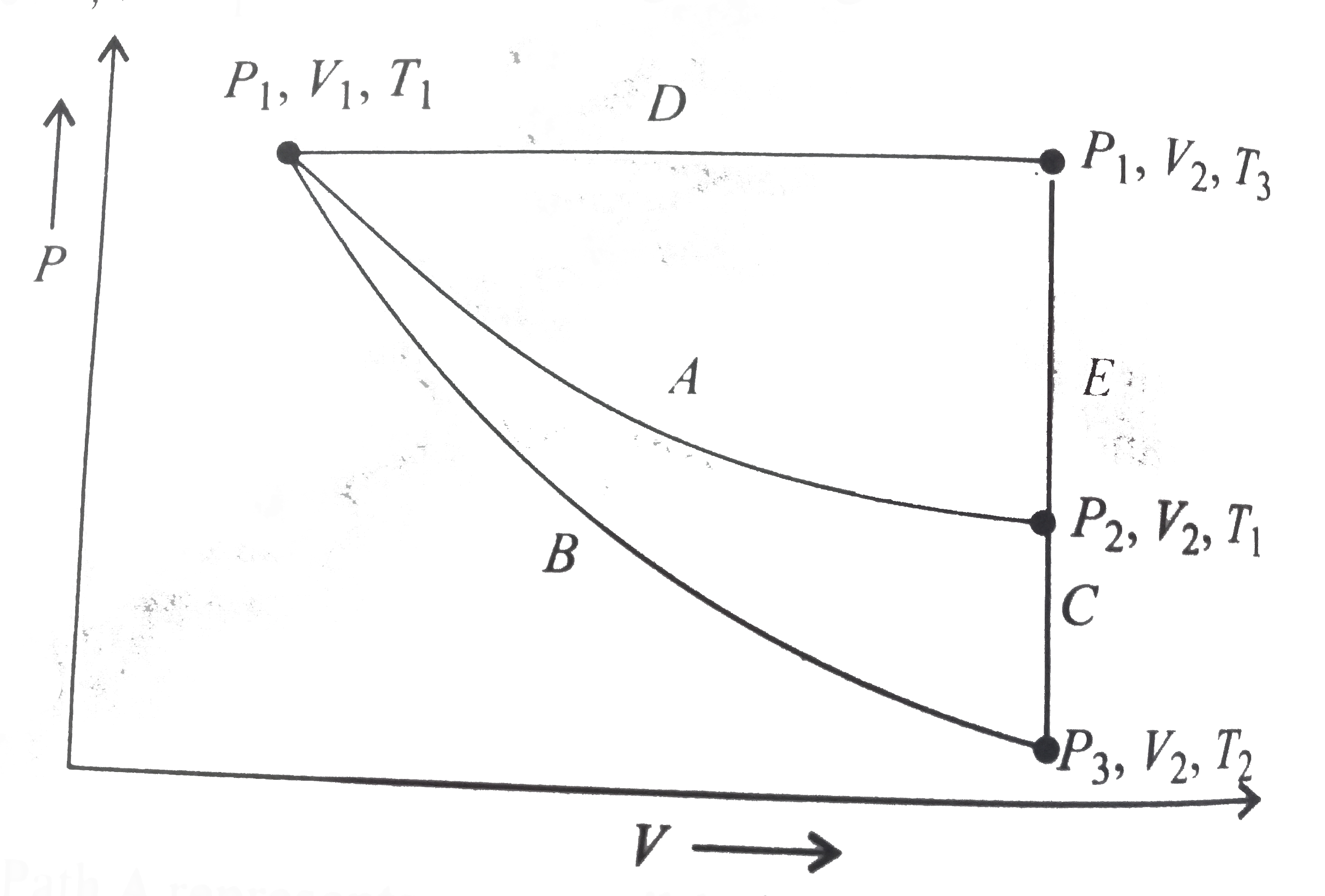
Path Arepresents a reversible isothermal expansion form P1,V1 to P2,V2, Path (B+C) represents a reversible adiabatic expansion (B) from P1,V1,T1→P3,V2,T2 followed by reversible heating the gas at constant volume (C)from P3,V2,T2 to P2,V2,T1. Path (D+E) represents a reversible expansion at constant pressure P1(D) from P1,V1,T1 to P1,V2,T3 followed by a reversible cooling at constant volume V2(E) from P1,V2,T3→P2,V2,T1.
What is qrev, for path (A)?01:51View SolutionFor an ideal gas, an illustratio of three different paths A(B+C) and (D+E) from an initial state P1,V1,T1 to a final state P2,V2,T1 is shown in the given figure.
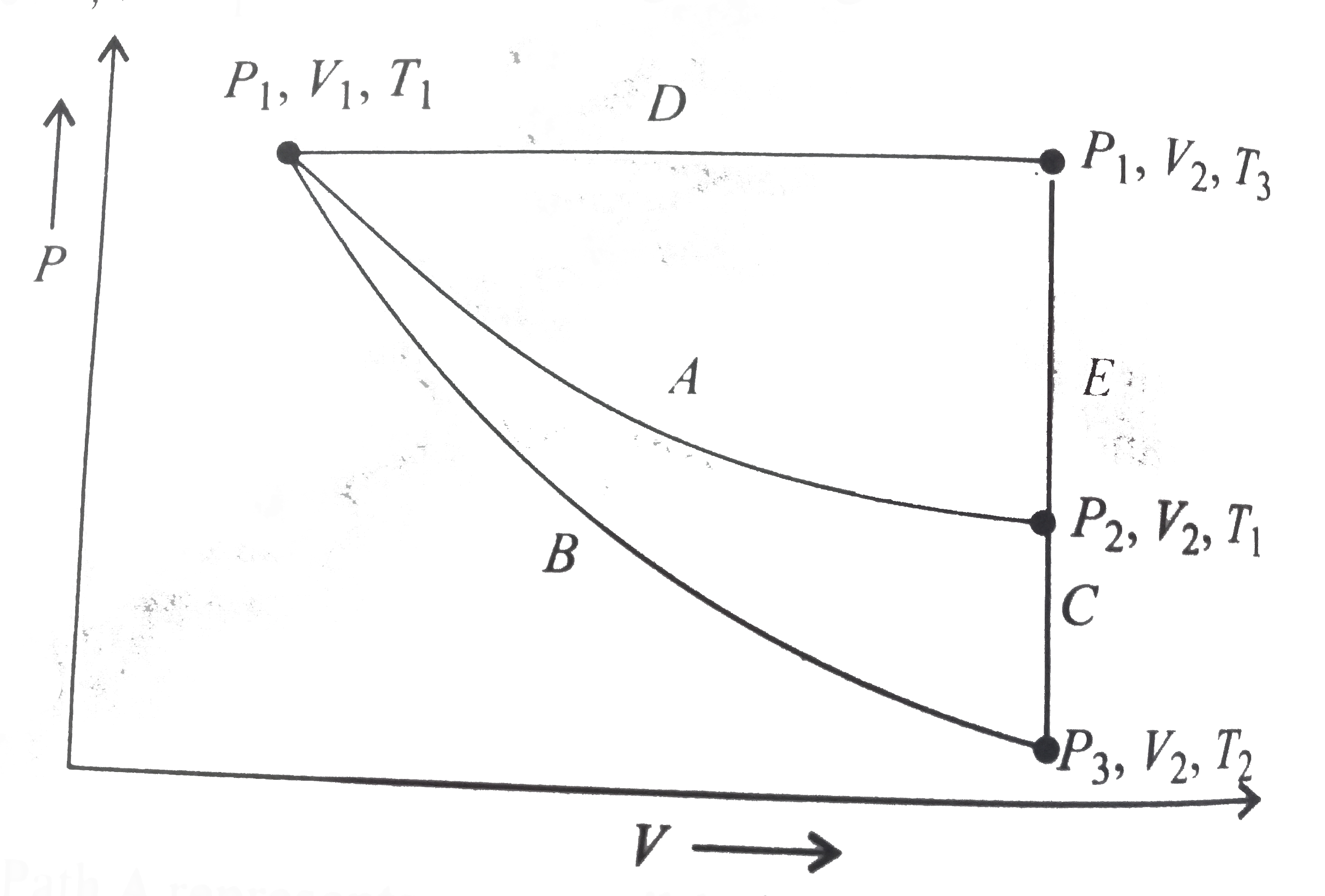
Path Arepresents a reversible isothermal expansion form P1,V1 to P2,V2, Path (B+C) represents a reversible adiabatic expansion (B) from P1,V1,T1→P3,V2,T2 followed by reversible heating the gas at constant volume (C)from P3,V2,T2 to P2,V2,T1. Path (D+E) represents a reversible expansion at constant pressure P1(D) from P1,V1,T1 to P1,V2,T3 followed by a reversible cooling at constant volume V2(E) from P1,V2,T3→P2,V2,T1.
What is ΔS for path A?03:52View SolutionAn ideal gasa undergoes through folowing cyclic procees. 1-2 : Reversible adialbatic comparession from P_(1)V_(1)T_(1)"to"P_(2)V_(2)T_(2) 2-3 : Reversible isochoric heating from P_(2)V_(2)T_(2)" to " P_(3)V_(3)T_(3) 3-4 Reversible adialbatic expansion from P_(3)V_(3)T_(3)" to " P_(4)V_(4)T_(4) 4-1 Reversible isochoric cooling from P_(4)V_(4)T_(4)" to " P_(1)V_(1)T_(1) Eefficiencyof the cycle is :
View SolutionFor an ideal monoatomic gas, an illustration of three different paths A,(B+C) and (D+E) from an initial state P1,V1,T1 to a final state P2,V2,T1 is shown in the given figure .
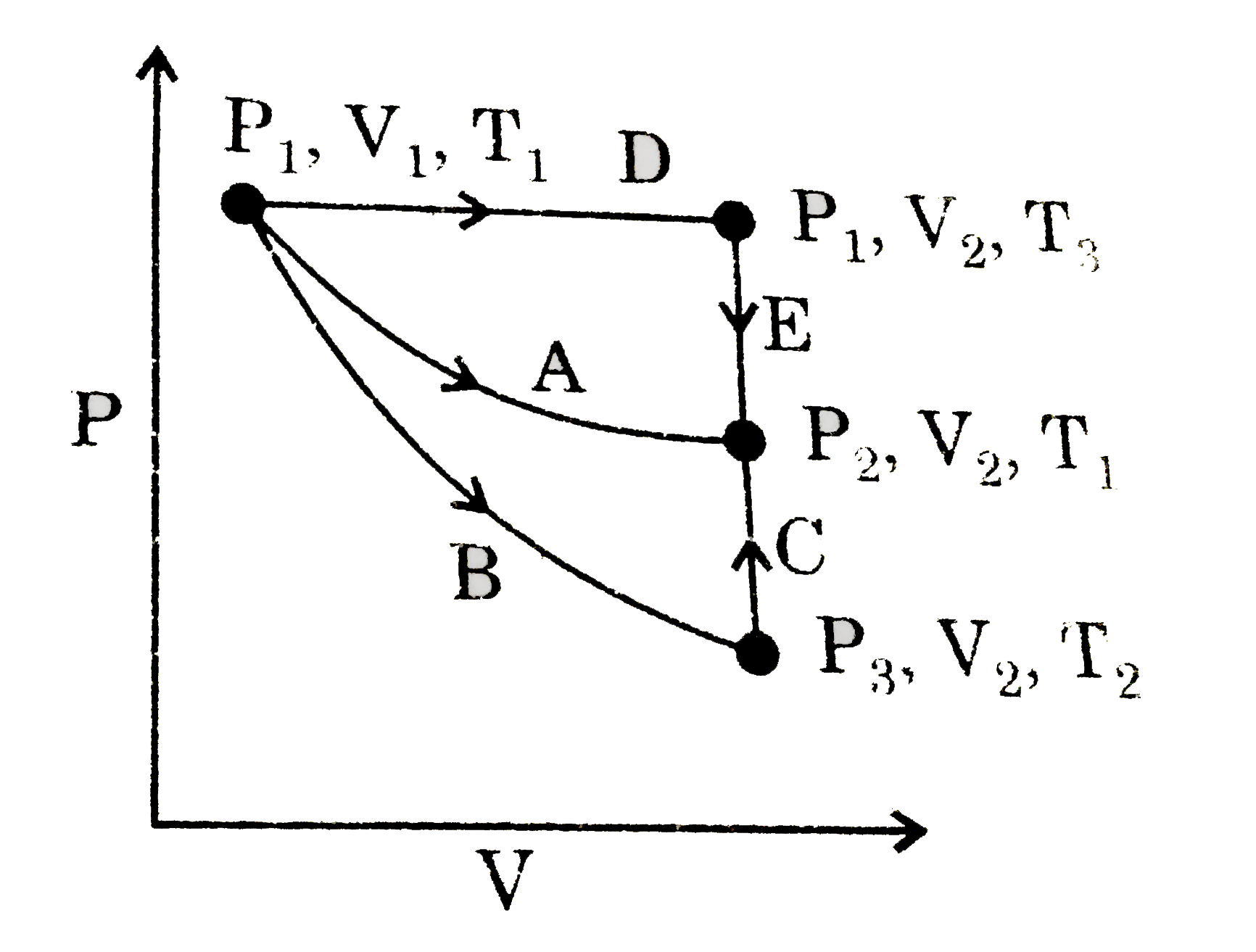
Path A represents a reversible isothermal from P1V1 to P2,V2, path (B+C) represent a reversible adiabatic expansion (B) from P1,V1,T1 to P3,V2,T2 followed by reversible heating of the gas at constant volume (C) from P3,V2,T2 to P2,V2,T1. Path (D+E) represents a reversible expansion at constant pressure P1(D) from P1,V1,T1 to P1,V2,T3 followed by a reversible cooling at constant volume V2(E) form P1,V2,T3 to P2,V2,T1
What is ΔS for (D+E)?05:56View SolutionAn ideal gas passes from one equllibrium state (P1,V1,T1,N) to anoter equilibrium state (2P1,3V1,T2,N) Then
01:51View Solutionयदि रुद्धोष्म परिवर्तन में गैस की अवस्था P1,V1,T1 सेP2,V2,T2 तक परिवर्तित हो जाती है , तो निम्न में से कौन - सा संबंधसत्य है -
04:02View SolutionAn ideal gas expands from state (P_(1), V_(1)) to state (P_(2), V_(2)) , where P_(2) = 2P_(1) and V_(2) = 2V_(1) . The path of the gas is expressed by the following relation, P = P_(1) [1 + ((V - V_(1))/(V_(1)))^(2)]
01:54View SolutionEstablish gas equation, (P_(1)V_(1))/(T_(1))=(P_(2)V_(2))/(T_(2)) .
View SolutionAn ideal gas passes from one equllibrium state (P1,V1,T1,N) to anoter equilibrium state (2P1,3V1,T2,N) Then
05:16View Solutionunderset(P_(1), V_(1), T_(1))("State") A overset("Rev")rarr underset(P_(2), V_(2), T_(2))("State B") : If P_(1) = P_(2) then
04:01View SolutionA gas with CP/CV= goes from an initial state (P1,V1,T1) to a final state (P2,V2,T2) through an adiabatic process. The work done by the gas is
a)nR(T1−T2)γ−1
b)P1V1−P2V2γ−1
c) nRγ(T1−T2)02:12View Solution

