If a line segment joining two points subtends equal angles at two other points lying on the sae side of the line segment; the four points are concyclic.

Video Solution

Text Solution

Verified by Experts

Proof $:$ There is one and only one circle that passes through three non-collinear points.
$∴$ A circle passes through points P,S and Q. Let us assume that the fourth point R does not lie on the circle.

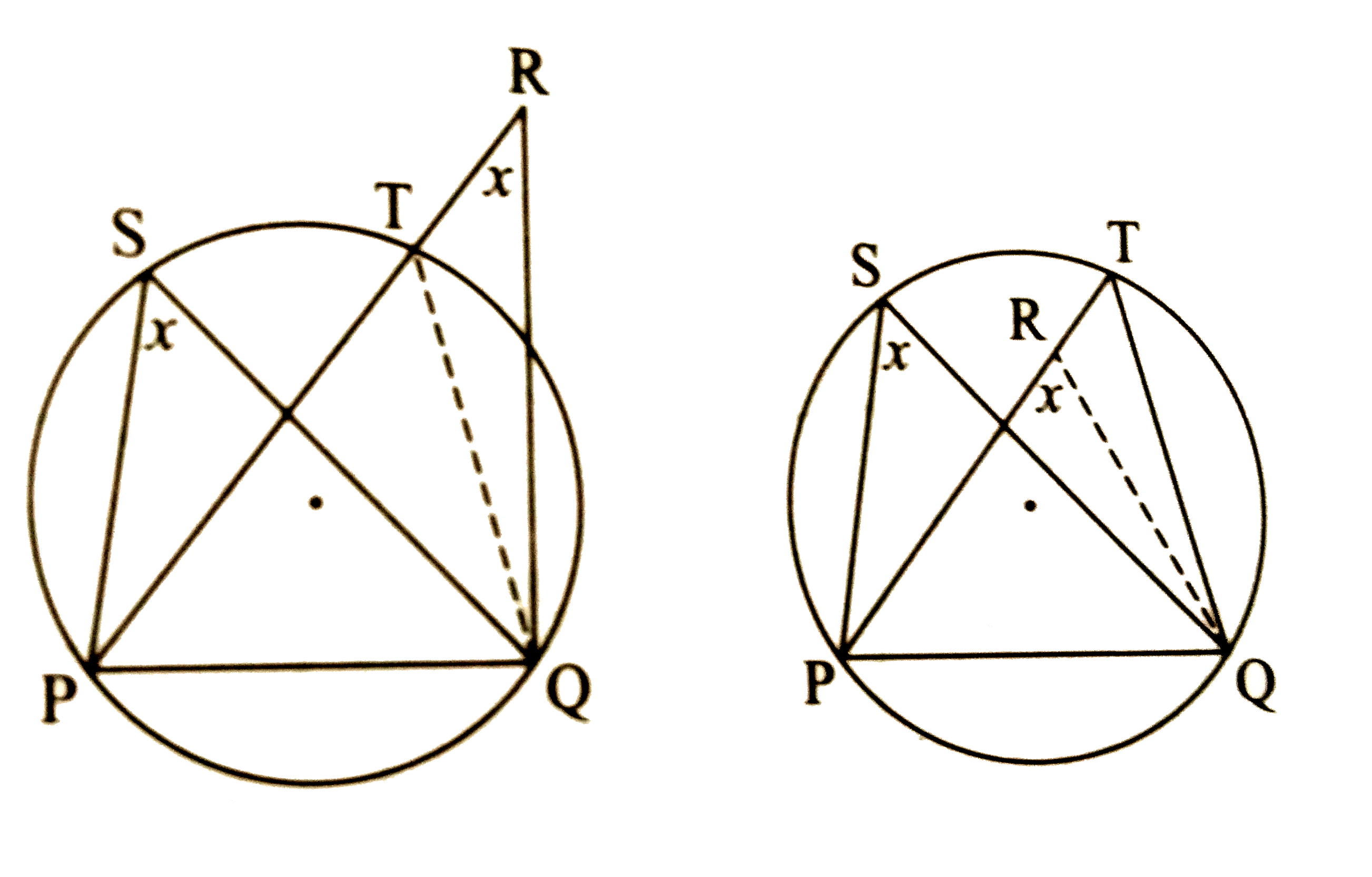
$∴$ It lie either in the interior or in the exterior of the circle.
Let us assume that point R lies in the exterior of the circle. Let seg PR intersect the circle at point T. Draw seg QT.
$∠ P S Q ≅ P R Q$ ...(Given ) ...(1)
$∠ P S Q ≅ ∠ P T Q$ ....(Angles inscribed in the same arc are congruent ) ....(2)
$∴ ∠ P Q R ≅ ∠ P T Q$ ....[From (1) and (2) ] ...(3)
$∠ P T Q$ is an exterior angle of $Δ T R Q$
$∴ ∠ P T Q > ∠ T R Q$ ...(Exterior angle theorem )
i.e., $∠ P T Q > ∠ P R Q$ ...(P-T-R)
This contradicts (3)
$∴$ Our assumption is false
$∴$ Point P cannot lie outside the circle.
Let us assume that point R lies in the interior of the circle.
$∠ P S Q ≅ ∠ P T Q$ ....(4) ....(Angles inscribed in the same arc are congruent )
But $∠ P S Q ≅ ∠ P R Q$ ....(Given ) ...(5)
$∴$ From (4) and (5)
$∠ P T Q ≅ ∠ P R Q$ ....(6)
$∠ P R Q$ is an exterior angle of $Δ R T Q$ ,
$∠ P R Q > ∠ R T Q$ ...(Exterior angle theorem )
i.e., $∠ P R Q > ∠ P T Q$ ....(P-R-T)
This contradicts (6)
$∴$ Point R cannot lie inside the cicle,
$∴$ Point R lies on the circle.
$∴$ Points P,S,R and Q are concyclic .
Note $:$ The above theorem is the converse of 'Angles inscribed in the same arc are congruent '.

Updated on:21/07/2023

If the line segment joining the points A(a,b) and B(c,d) subtends an angle $θ$ at the origin, then $cos θ$ is equal to
A $\frac{a b + c d}{\sqrt{(a^{2} + b^{2}) (c^{2} + d^{2})}}$
B $\frac{a c + b d}{\sqrt{(a^{2} + b^{2}) (c^{2} + d^{2})}}$
C $\frac{a c - b d}{\sqrt{(a^{2} + b^{2}) (c^{2} + d^{2})}}$
D $\frac{a d + b c}{\sqrt{(a^{2} + c^{2}) (b^{2} + d^{2})}}$