Theorem of internal division of chords.
Suppose two chords of a circle intersect each other in the interior of the circle, then the product of the lengths of the two segments of one chord is equal to the product of the lengths of the two segments of the other chord.

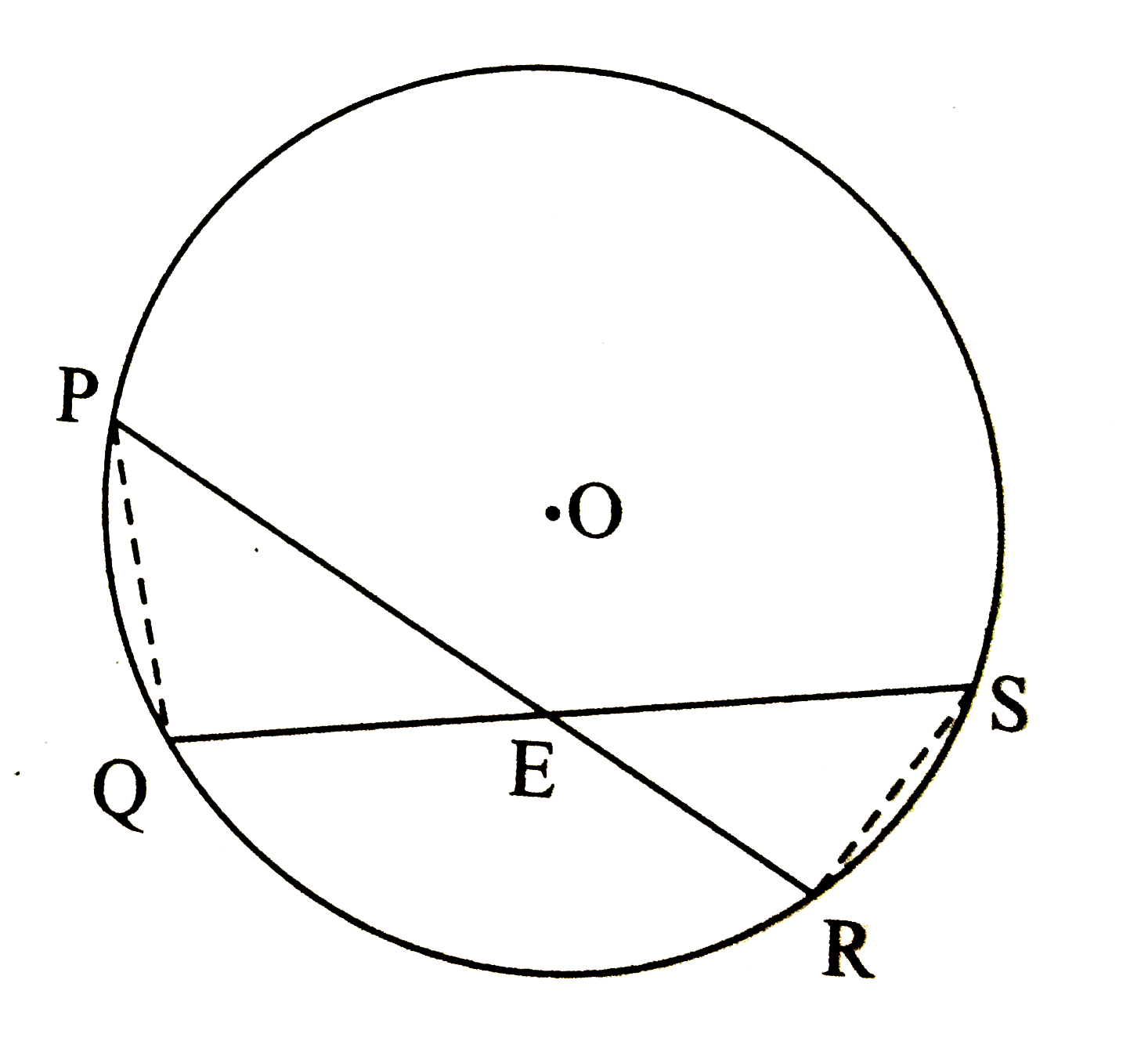
Given $:$ (1) A circle with centre O .
(2) chords PR and QS intersect at point E inside the circle.
To prove $:$ PE $\times$ ER = QE xx ES $< b r > C o n s t r u c t i o n$ : Draw seg PQ and seg RS

Video Solution

Text Solution

Verified by Experts

Proof $:$ $∠ P Q S ≅ ∠ S R P$ ....(Angles inscribed in the same arc ar congruent )
i.e., $∠ P Q E ≅ ∠ S R E$ ….(P-E-R and Q-E-S ) ….(1)
In $Δ P Q E$ and $Δ S R E$ ,
$∠ P Q E ≅ ∠ S R E$ ….[From (1) ]
$∠ P E Q ≅ ∠ S E R$ .....(Vertically opposite angles )
$∴ Δ P Q E ~ Δ S R E$ .....(AA test of similarity )
$∴ \frac{P E}{S E} = \frac{Q E}{R E}$ ......(Corresponding sides of similar triangles are in proportion )
$∴ P E \times E R = Q E \times S E$ .

Updated on:21/07/2023

Two circles with same radius r intersect each other and one passes through the centre of the other. Then the length of the common chord is
Ar
B $\sqrt{3}$ r
C $\frac{\sqrt{3}}{2}$ r
D $\sqrt{5}$ r
Two circles with same radius $r$ intersect each other and one passes through the centre of the other. Then the length of the common chord is
A $r$
B $\sqrt{3}$ r
C $\frac{\sqrt{3}}{2}$ r
D $\sqrt{5}$ r
The length of a chord of a circle is equal to the radius of the circle. The angle which this chord subtends in the major segment of the circle is equal to :
A $30^{\circ}$
B $45^{\circ}$
C $60^{\circ}$
D $90^{\circ}$