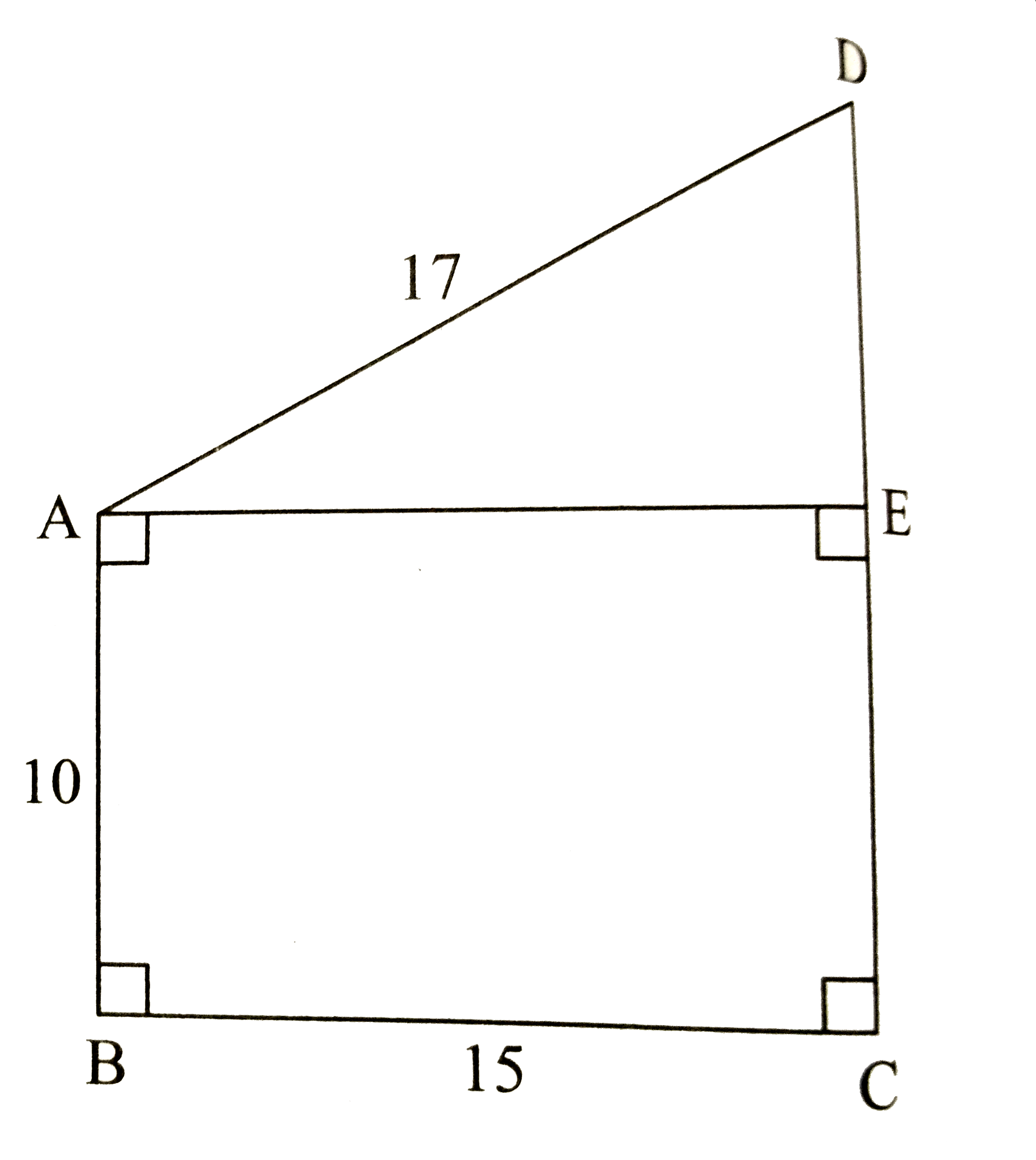
In the figure, AD=17, AB=10, BC=15. ∠ABC=∠BCD=90∘ seg AE⊥ side CD then find the length of (i)AE (ii)DE (iii)DC.
Video Solution
Text Solution
In □ABCE,
∠ABC=∠BCE=∠CEA=90∘…….(Given)
∴∠EAB=90∘…….(Remaining angle of a quadrilateral)
∴SquareABCE is rectangle.
(i)∴AE=BC……(Opposite sides of rectangle are equal)
∴AE=15
(ii) In ΔDEA,
∠DEA=90∘
∴ by Pythagoras theorem,
AD2=AE2+ED2
∴172=152+ED2
∴ED2=172−152
∴ED2=289−225
∴ED2=64
∴ED=8
(iii)CE=AB .......(Opposite sides of rectangle are equal)
∴CE=10
CD=CE+ED.......(C−E−D)
∴CD=10+8
∴CD=18
|
Topper's Solved these Questions
- PYTHAGORAS THEOREMBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PYTHAGORAS THEOREMEXERCISE - 3.5 (4 mark each)
4 Videos
- PYTHAGORAS THEOREMBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PYTHAGORAS THEOREMEXERCISE - Assignment 3.1
8 Videos
- PYTHAGORAS THEOREMBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PYTHAGORAS THEOREMEXERCISE - 3.3 (2 mark each)
8 Videos
- PROBABILITY BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PROBABILITY EXERCISE - ASSIGNEMENT 5.4
12 Videos
- QUADRATIC EQUATION BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUADRATIC EQUATION EXERCISE - CHALLENGIN QUESTIONS
2 Videos
Similar Questions
Knowledge Check
In the given figure, AB⊥AD,BC⊥BD and AD = 9 cm, BC = 8 cm and CD= 17 cm. Find the area of quadrilateral ABCD
 A112 cm2B114 cm2C119 cm2D117 cm2
A112 cm2B114 cm2C119 cm2D117 cm2
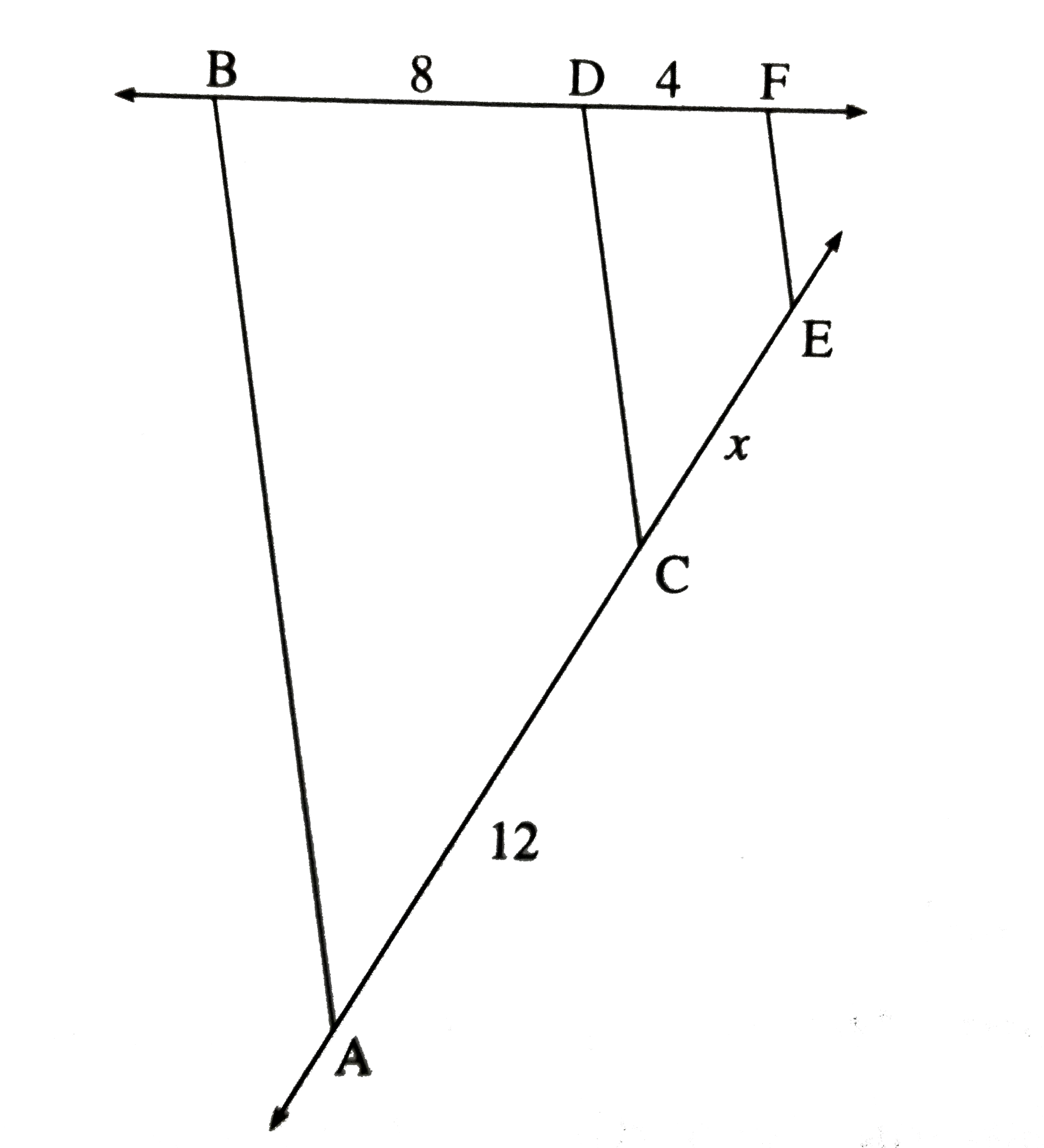
In the figure, seg AB∣∣ seg CD∣∣ seg EF AC=1.5, BD=10, DF=6 then AE=?
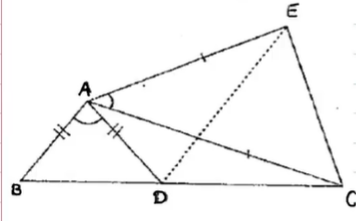
View SolutionIn Figure, AC=AE, AB=AD and ∠BAD=∠EAC. Prove that BC=DE
View SolutionIn the figure, DE∣∣BCandAE:EC=3:7. Find
View SolutionIn the following figure, AD=DB and DE∣∣BC. Then AE=EC (True/ False)
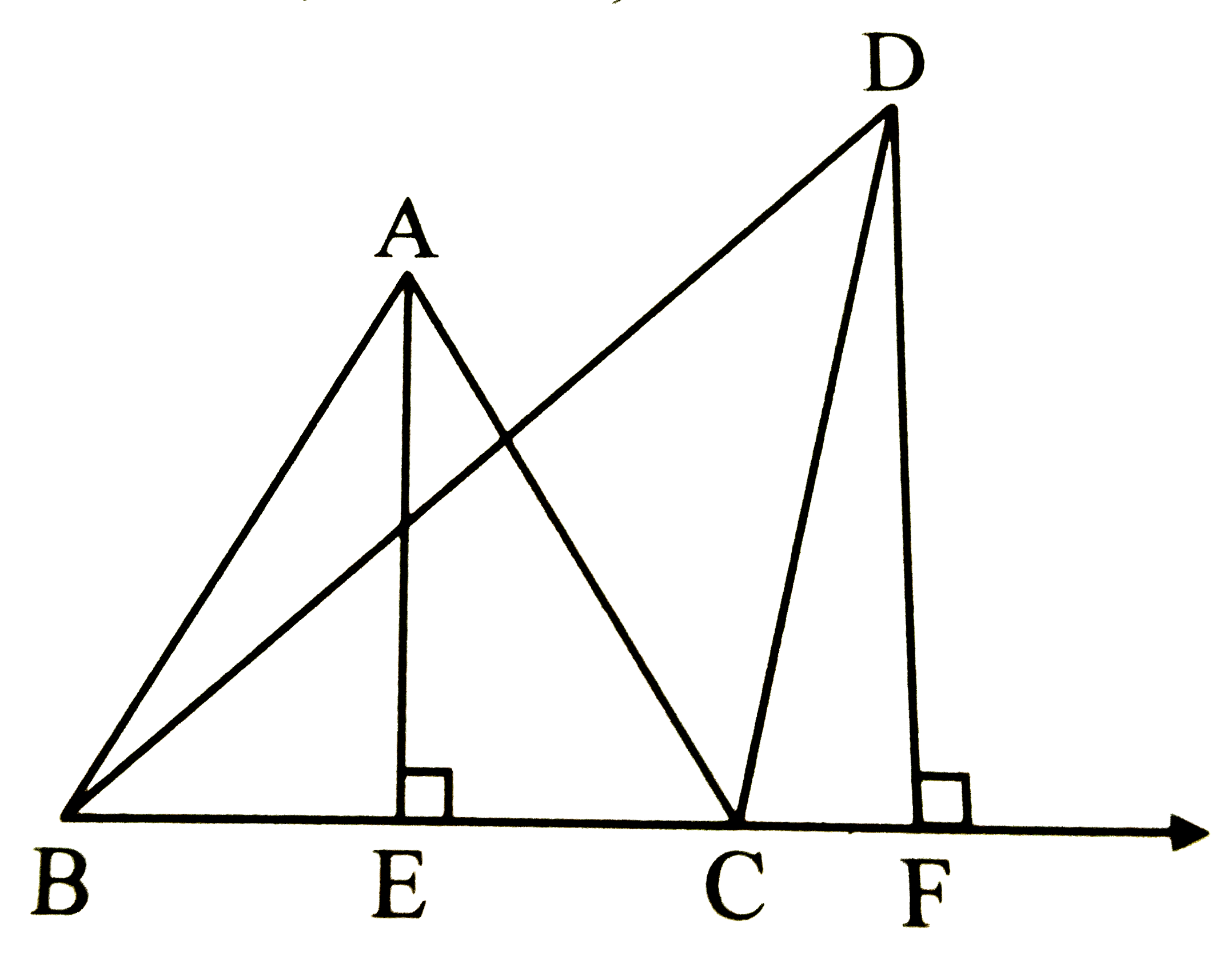
View SolutionIn given figure, seg AE ⊥ seg BC, seg DF ⊥ line BC,
AE = 4, DF = 6, then find A(ΔABC)A(ΔDBC).
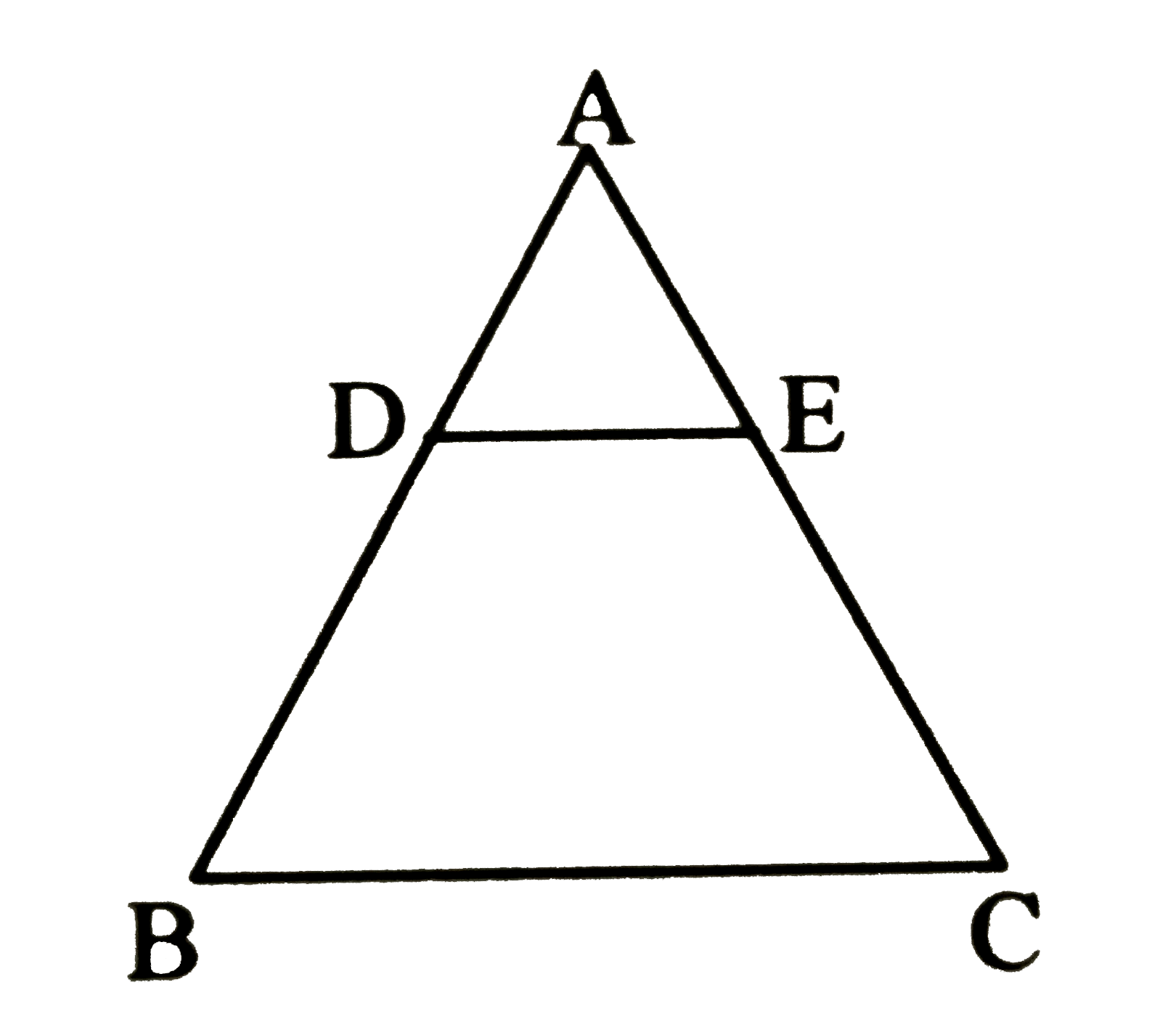
View SolutionIn the given figure, DE || BC. If AB = 12 cm and AD = 3cm, then AE : EC =
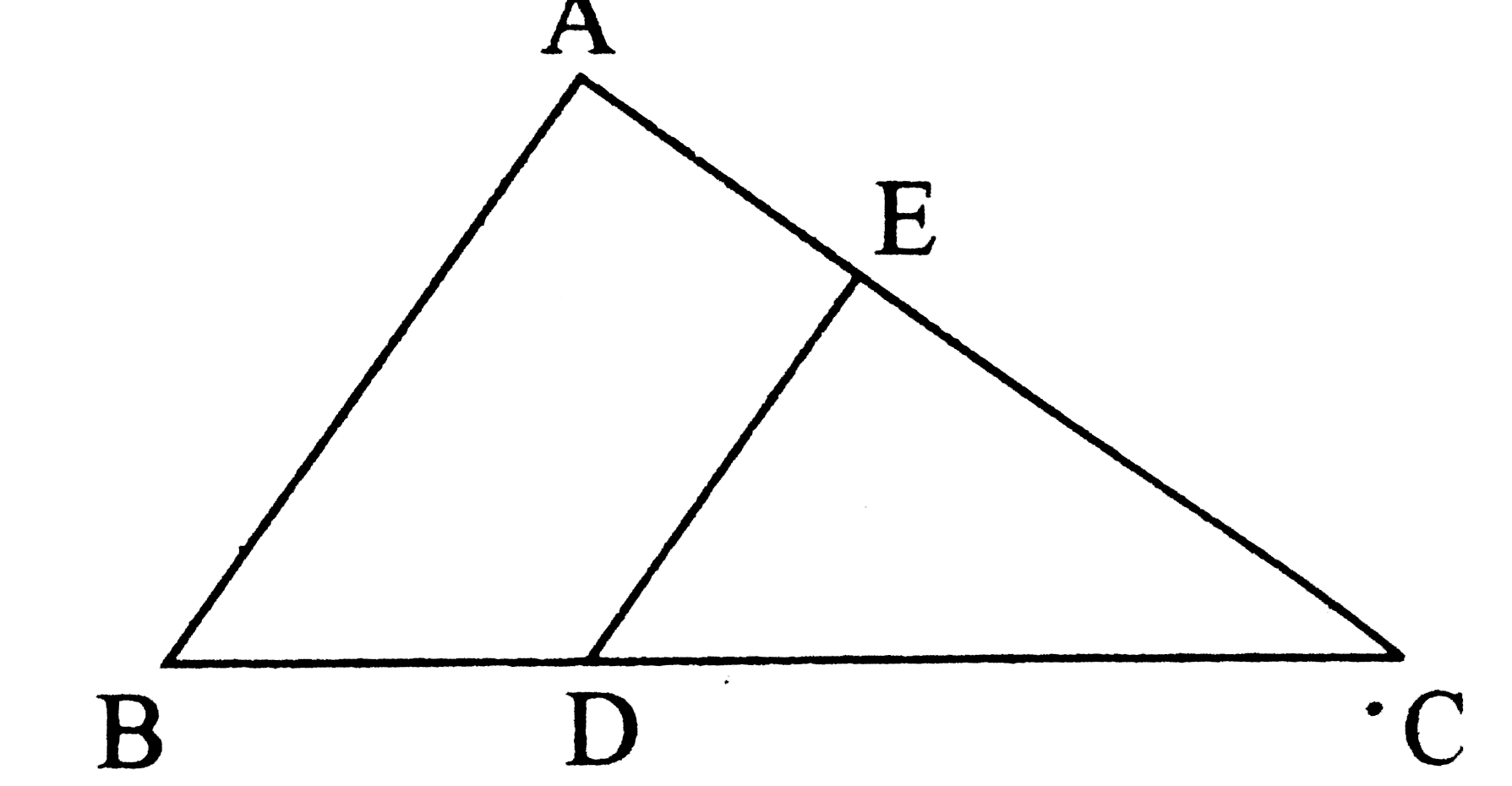
View SolutionIn the figure, seg DE∣∣ side AB. DC=2BD, A(ΔCDE)=20cm2. Find A(□ABDE).
View Solution