Ray BA is tangent at point A . Ray BD is secant intersecting the circle at points C and D then
BA2=□×□
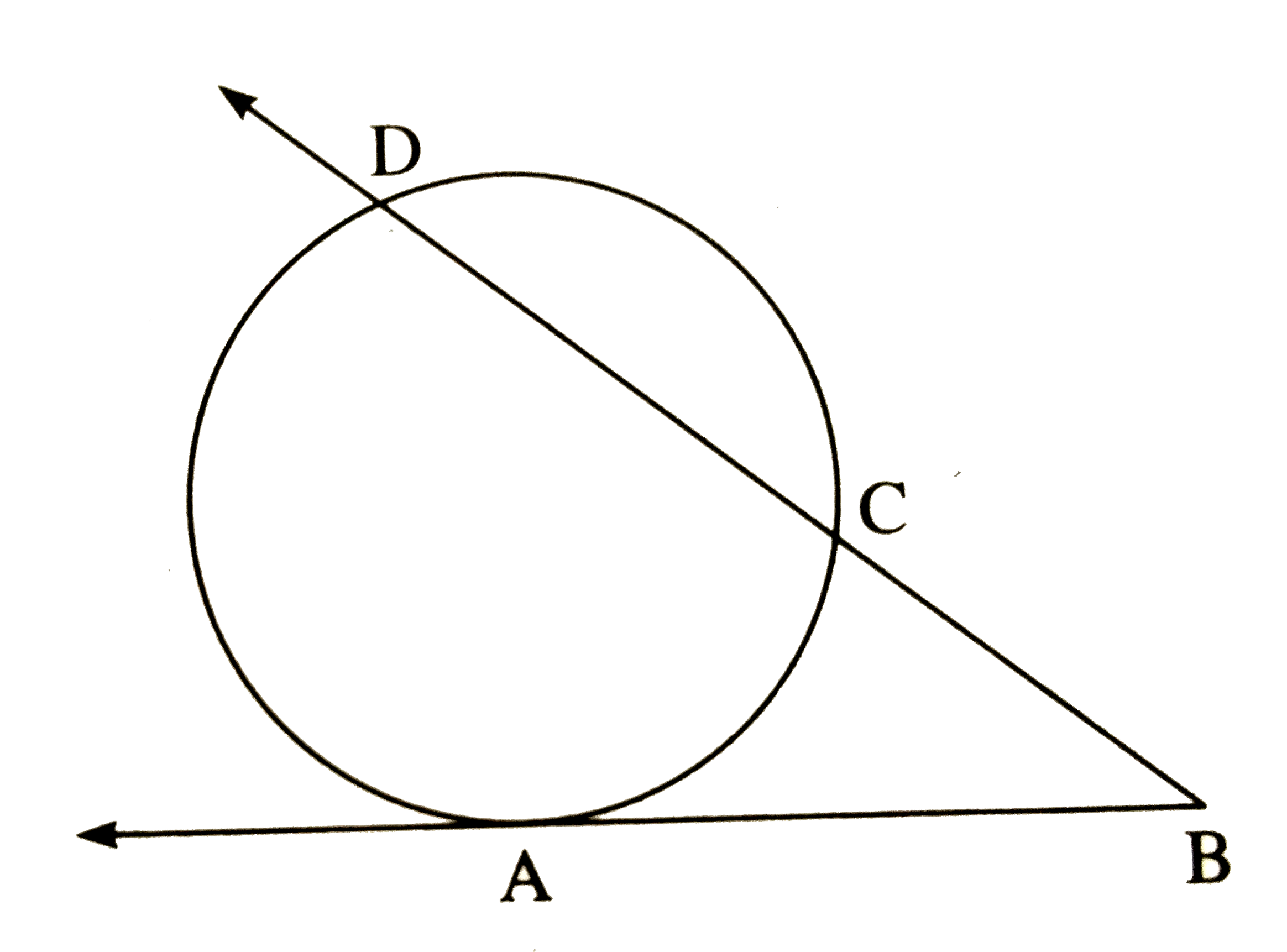
The correct Answer is:BA2=BC×BD
Step by step video & image solution for Ray BA is tangent at point A . Ray BD is secant intersecting the circle at points C and D then BA^(2) = square xx square by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CIRCLEBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - ASSIGNMENT 4.3
11 Videos
- CIRCLEBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - ASSIGNMENT 4.4
12 Videos
- CIRCLEBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - ASSIGNMENT 4.1
10 Videos
- CHALLENGING QUESTIONSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CHALLENGING QUESTIONSEXERCISE - SECTION 3 (MODEL QUESTION PAPER FOR PRACTICE ) Solve any one of the following subquestions :
1 Videos
- COORDINATE GEOMETRY BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - COORDINATE GEOMETRY EXERCISE - Assignment 6.5
13 Videos
Similar Questions
Given : Line ET is tangent to the circle at point E. Line EAB is secant intersecting at point A and B.
To Prove :ET2=EA×EB
Construction : Draw seg BT and Seg AT
Complete the proof by filling the boxes.

View SolutionIn the figure, ray PT touches the circle at point T and line PAB is secent intersecting the circle at points A and B, then

View Solution
Knowledge Check
P is a point outside a circle and is 13 cm away from its centre. A secant drawn from the point P intersects the circle at points A and B in such a way that PA=9 cm and AB=7 cm. The radius of the circle is
A5.5 cmB5 cmC4 cmD4.5 cm
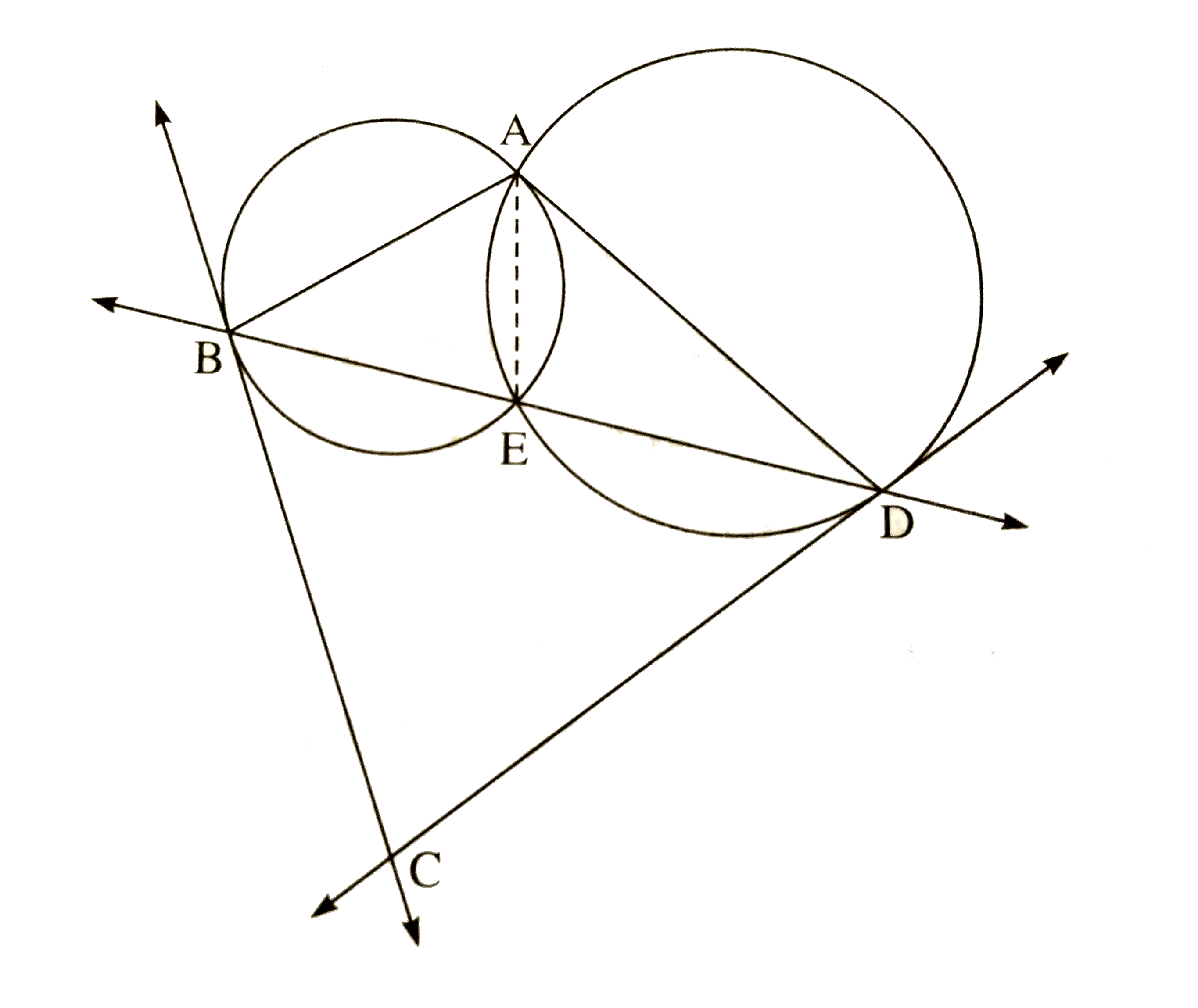
In figure, two circles intersect each other at points A and E. Their common secant through E intersects the circles at points B and D. The tangents of the circles at points B and D intersect each other at point C. Prove that □ ABCD is cyclic.
View SolutionIn the figure, a tangent segment PA touches the circle at point A and secant PBC intersects the circle at points B and C. If AP=15 cm and BP=10, then find BC.
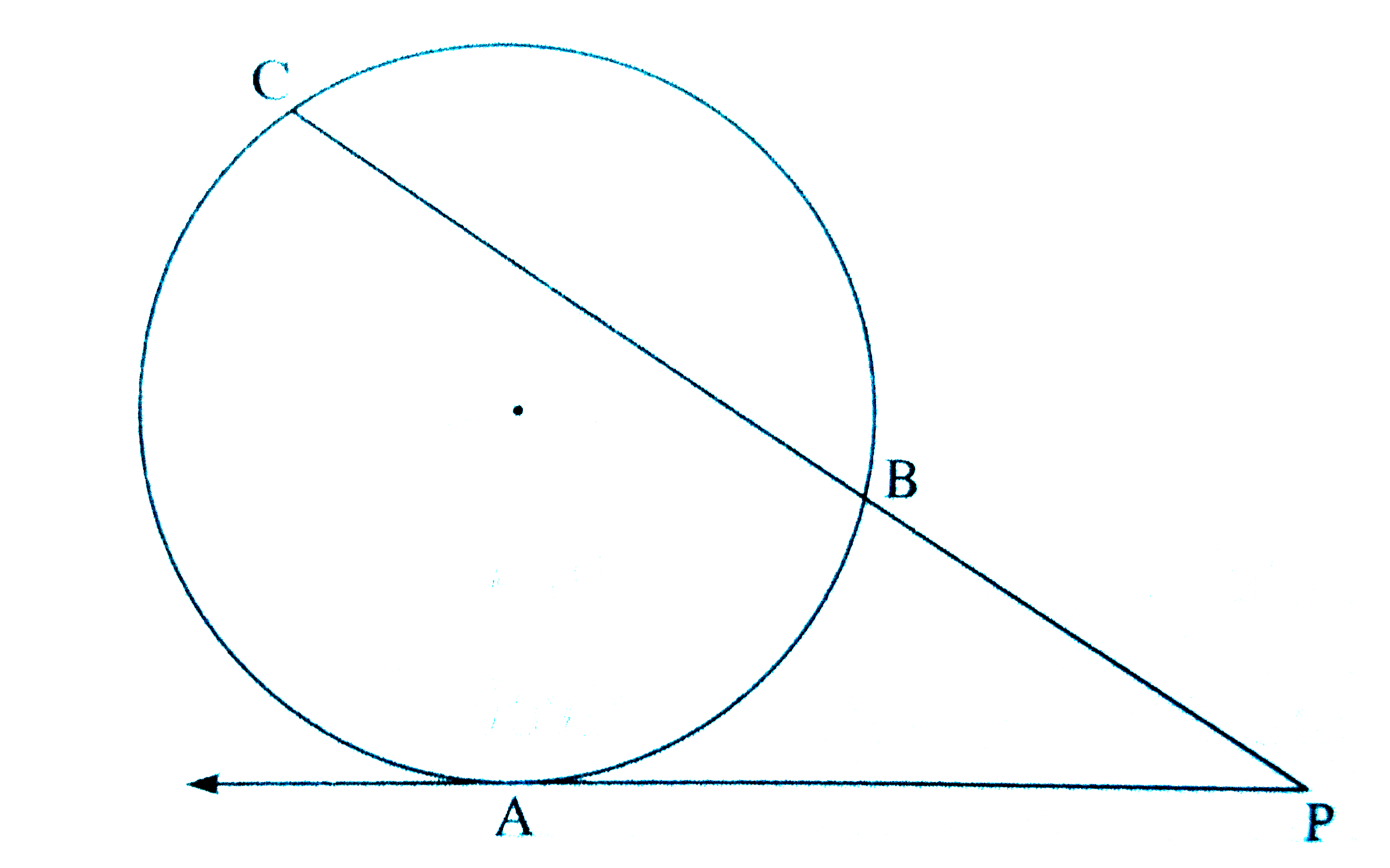
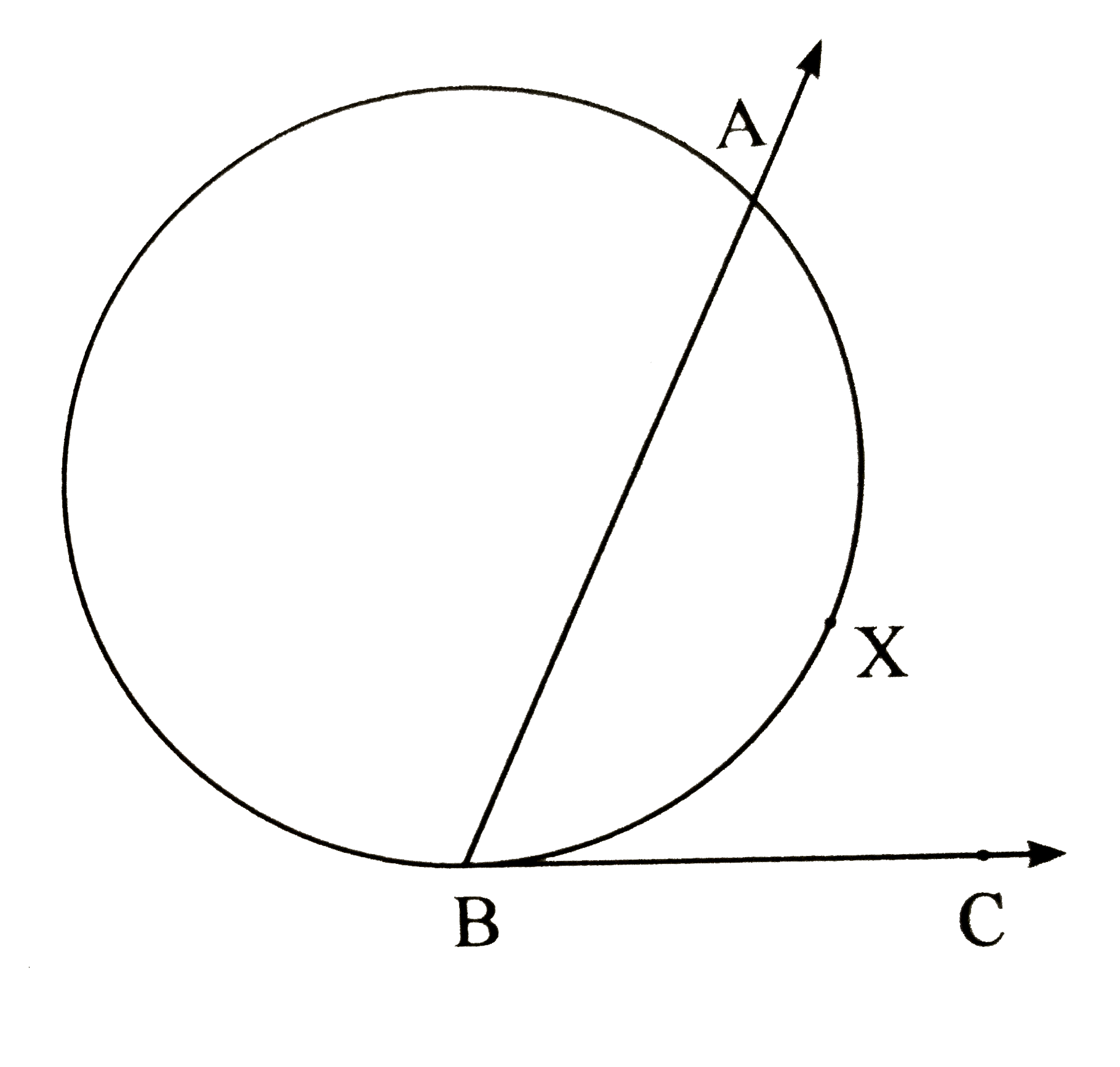
View SolutionIn the figure, ray BC is tangent at point B and ray BA is secant. ∠ABC intercepts are AXB if m(arc AXB ) =130∘ then find the measure of ∠ABC.
View SolutionTangent Secant Theorem
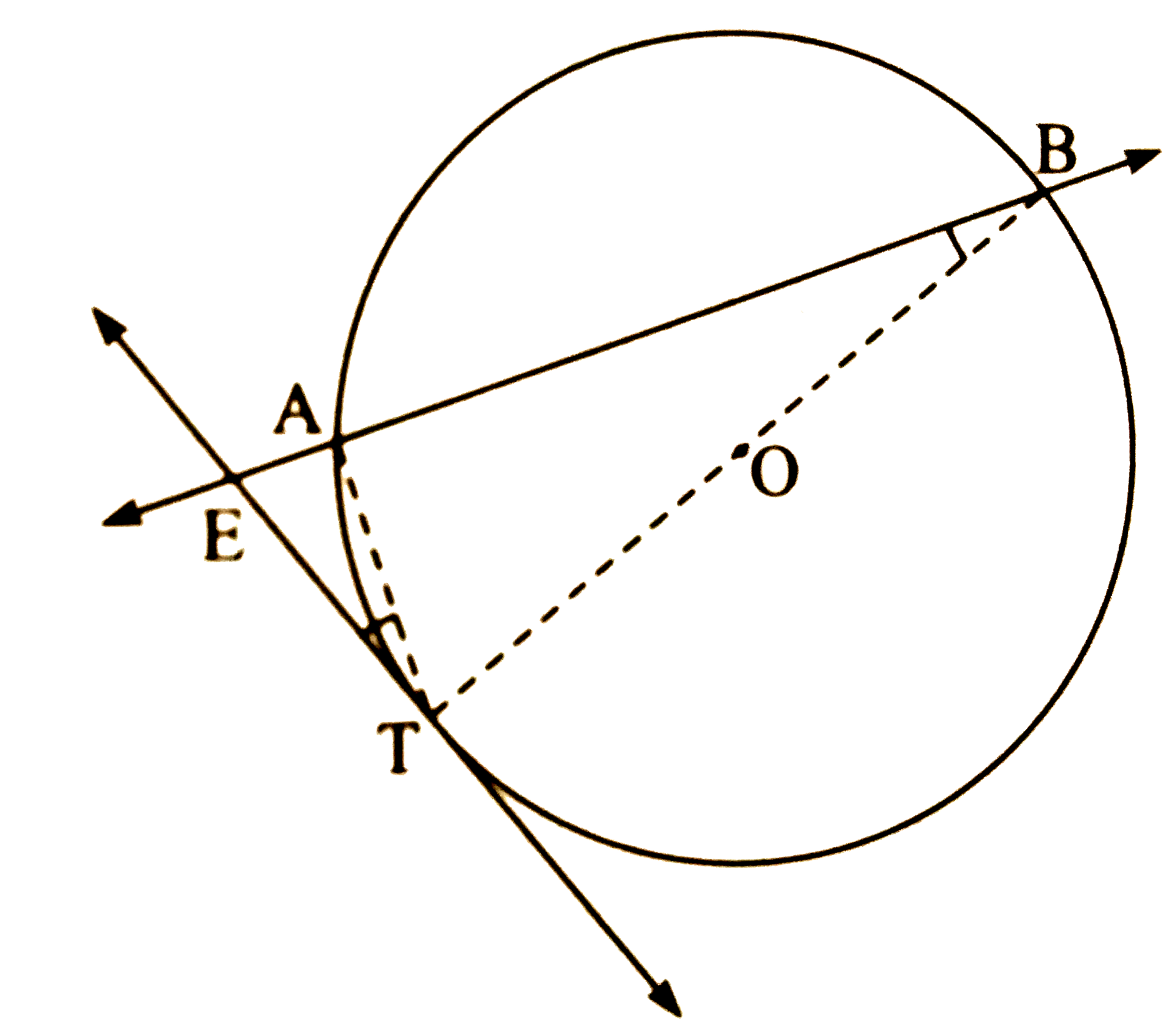
Point E is in the exterior of a circle. A secant through E intersects the circle at points A and B, and a tangent through E touches the circle at point T, then EA×EB=ET2.
Given : (1) A circle with centre O
(2) Tangent ET touches the circle at pointT
(3) Secant EAB intersects the circle at points A and B .
To prove : EA×EB=ET2
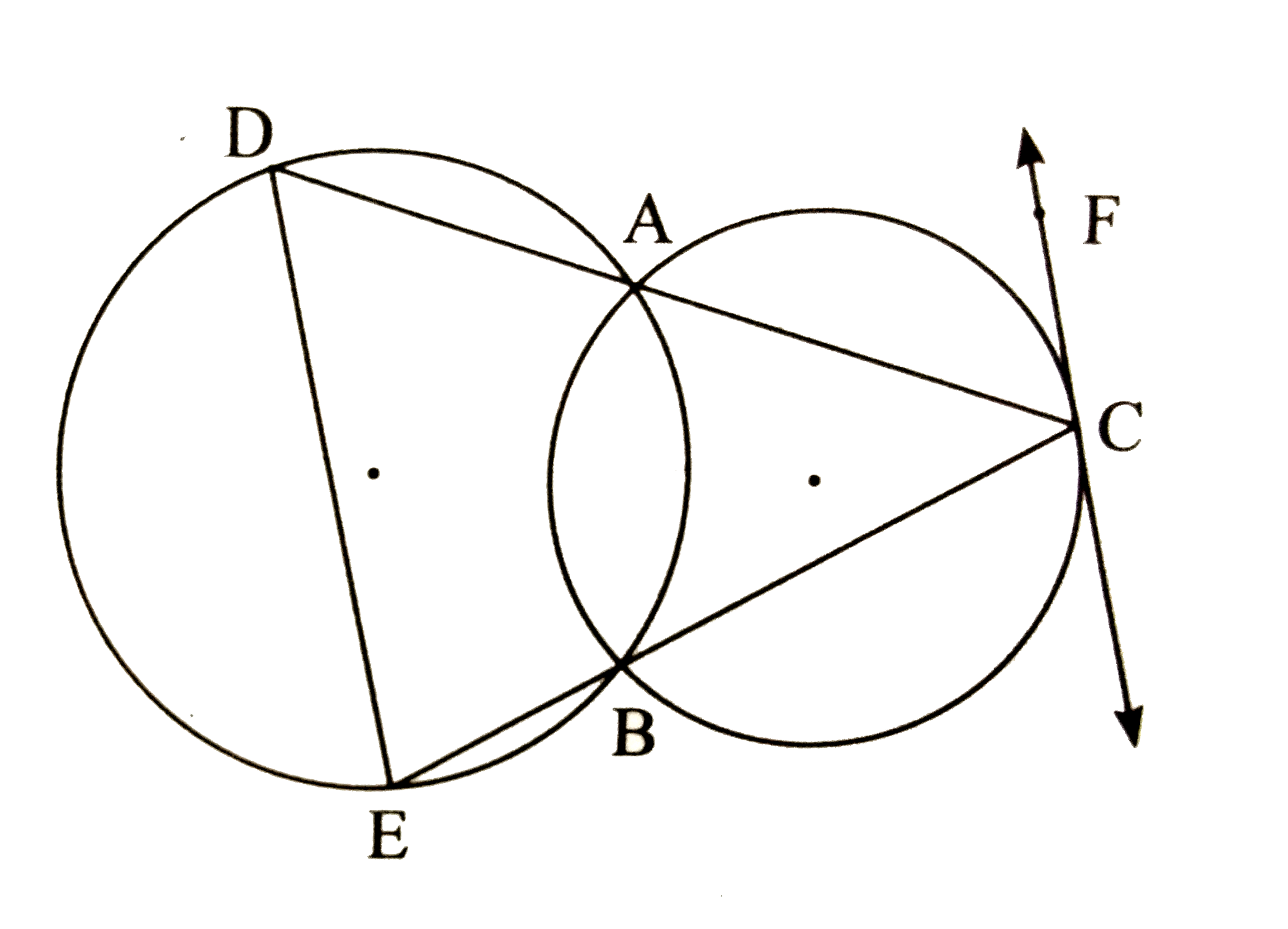
View SolutionIn the figure, two circles intersect each other at points A and B . C is a point on the smaller circle. Secant CA and secant CB of the smaller circle intersect the bigger circle at points D and E respectively then prove : seg DE ∣∣ line CF
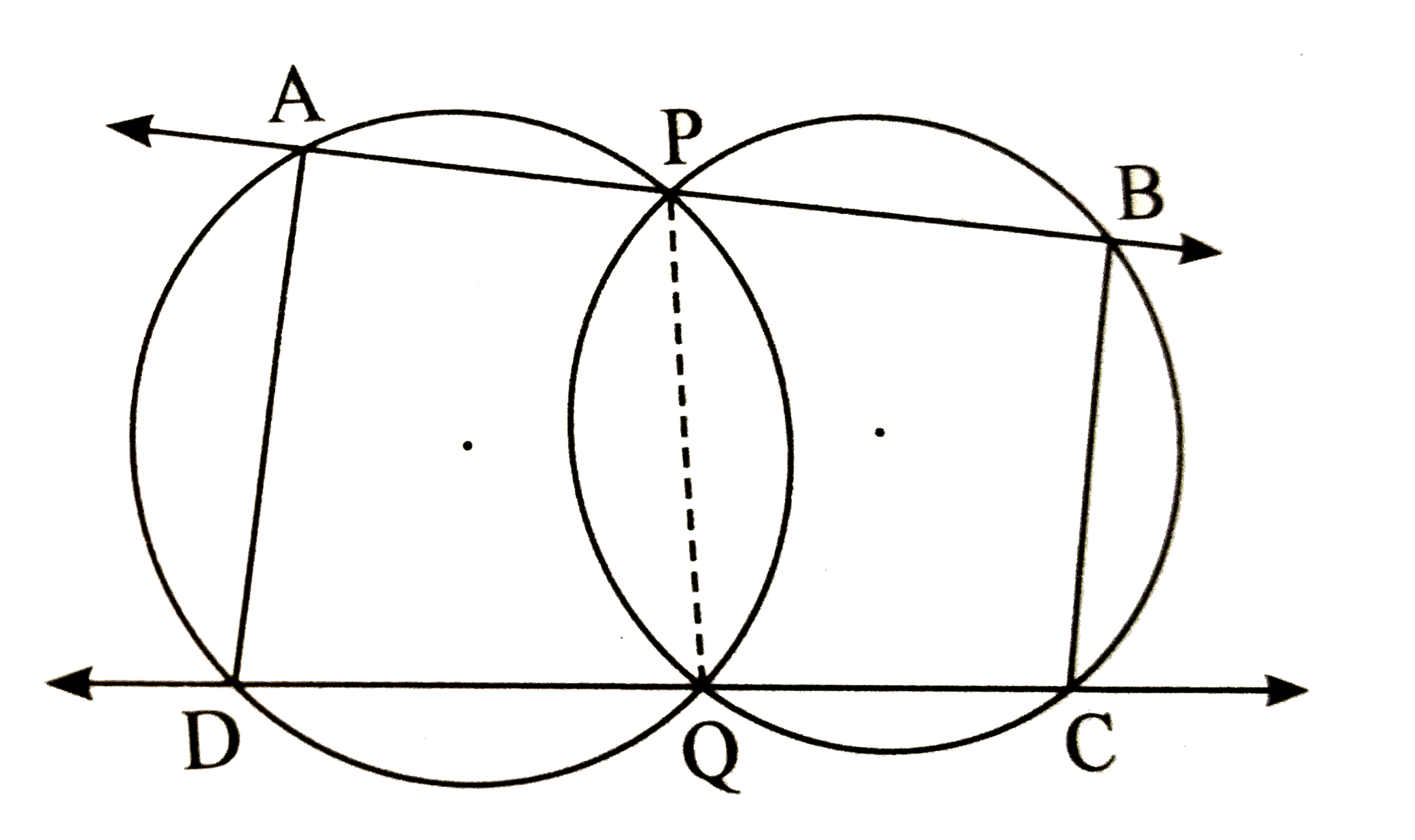
View SolutionTwo circles intersect each other at point P and Q. Secants drawn through p and Q intersect the circles at points A,B and D,C
Prove that :∠ADC+∠BCD=180∘
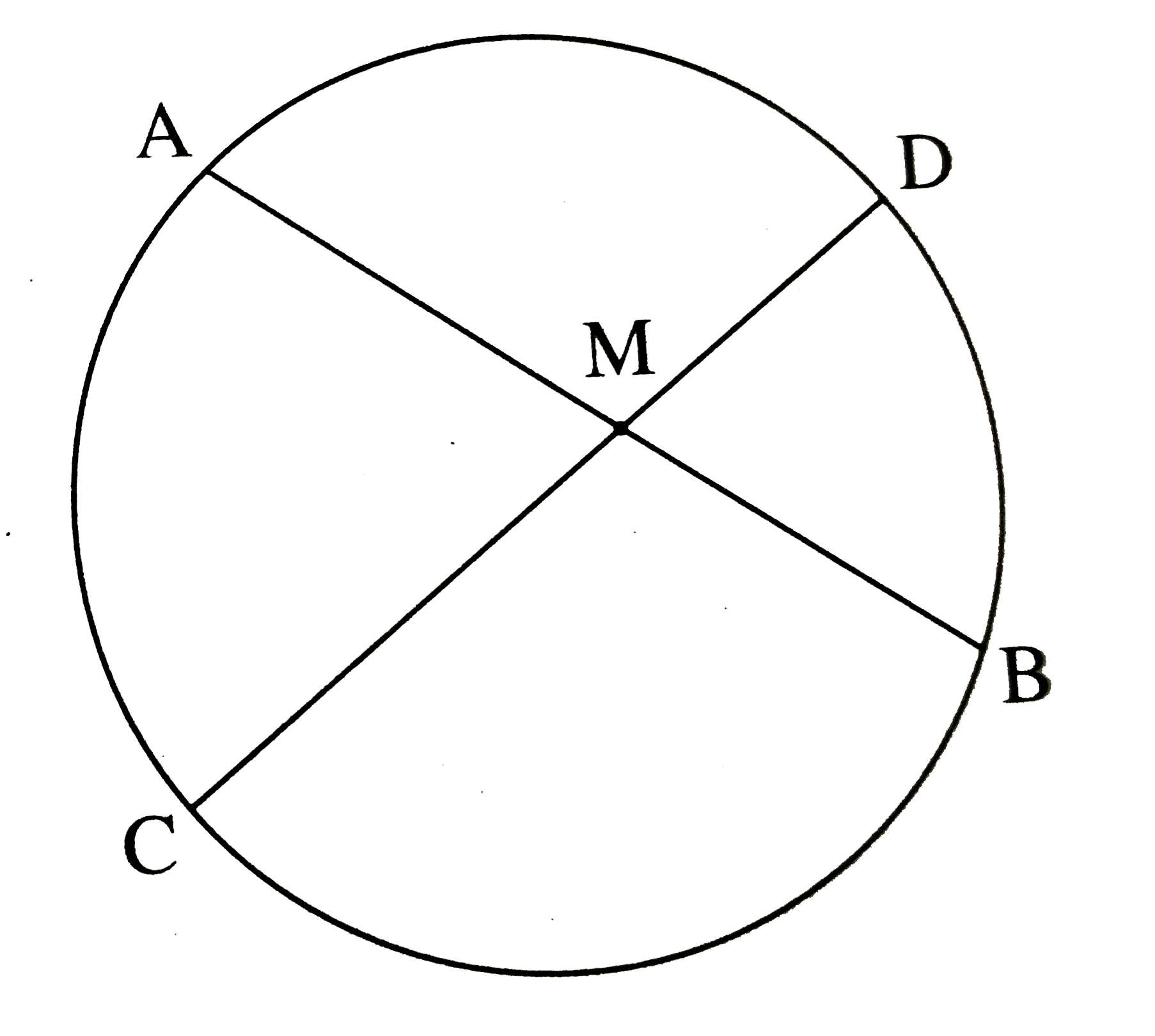
View SolutionChords AB and CD intersect at point M inside the circle then theorem of internal division of chords.
AM×BM=□×□
View Solution
