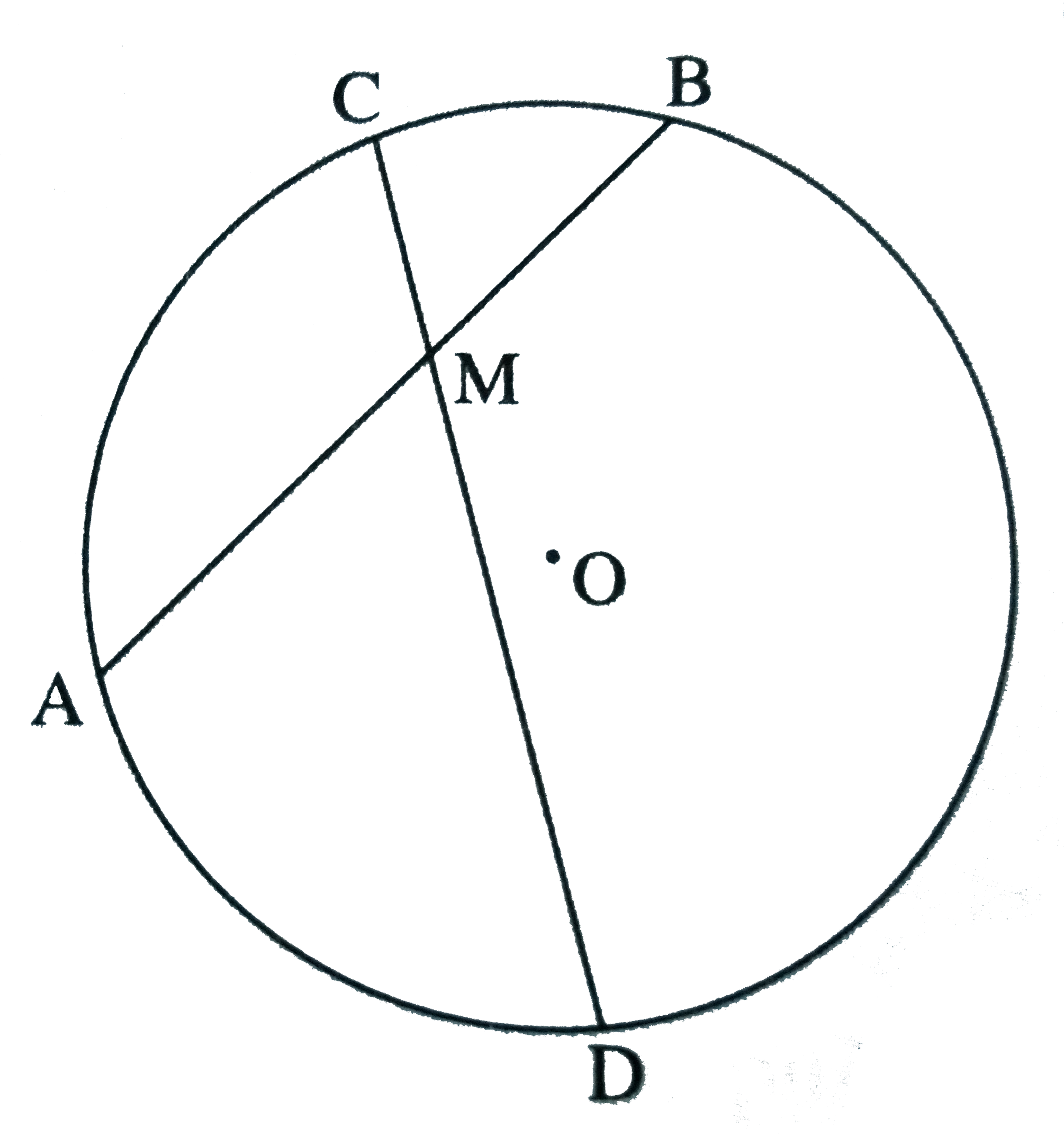
In the figure , chords PQ and RS intersect at point T outside the circle then by theorem of external division of chords,
PT×□=ST×□

The correct Answer is:PT×QT=ST×RT
Step by step video & image solution for In the figure , chords PQ and RS intersect at point T outside the circle then by theorem of external division of chords, PT xx square = ST xx square by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CIRCLEBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - ASSIGNMENT 4.3
11 Videos
- CIRCLEBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - ASSIGNMENT 4.4
12 Videos
- CIRCLEBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - ASSIGNMENT 4.1
10 Videos
- CHALLENGING QUESTIONSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CHALLENGING QUESTIONSEXERCISE - SECTION 3 (MODEL QUESTION PAPER FOR PRACTICE ) Solve any one of the following subquestions :
1 Videos
- COORDINATE GEOMETRY BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - COORDINATE GEOMETRY EXERCISE - Assignment 6.5
13 Videos
Similar Questions
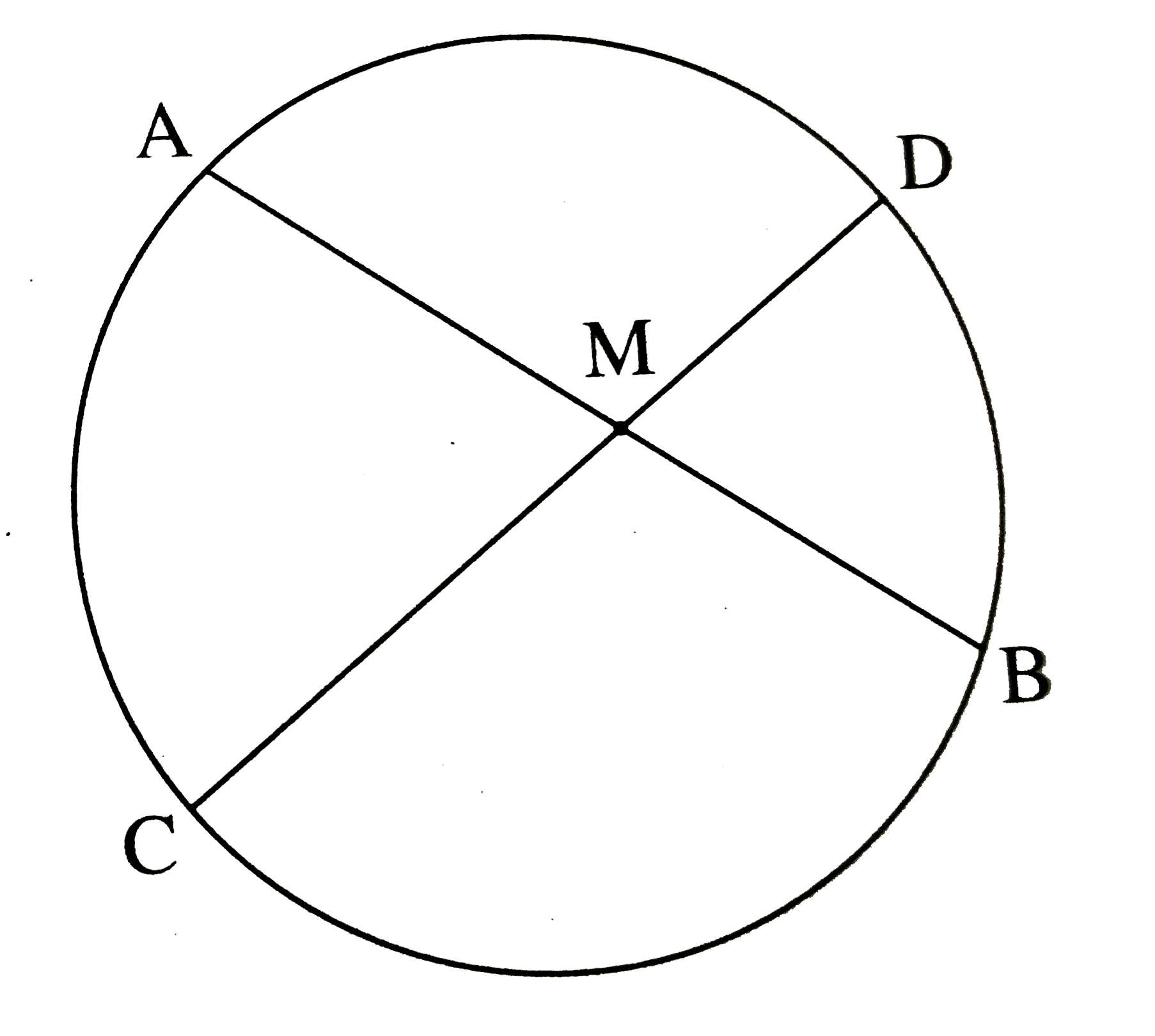
Chords AB and CD intersect at point M inside the circle then theorem of internal division of chords.
AM×BM=□×□
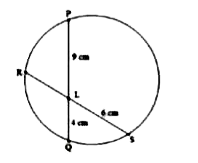
View SolutionIn the given figure, chords PQ and RS intersect each other at point L Find the length of RL.
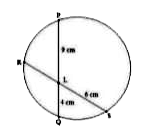
View SolutionIn the given figure, chords PQ and RS intersect each other at point L. Find the lenth of RL.
View SolutionIn the figure, chord AB and chord CD intersect at point M inside the circle such that AM=4, BM=3, CM=2, then CD=………
View SolutionIf two chords of a circle intersect inside or outside the circle when produced ; the rectangle formed by the two segments of one chord is equal in area to the rectangle formed by the two segments of the other chord.
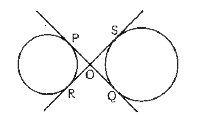
View SolutionIn the figure, PQ and RS are the common tangents to two circles intersecting at O.
Prove that PQ=RS
View SolutionTheorem of internal division of chords.
Suppose two chords of a circle intersect each other in the interior of the circle, then the product of the lengths of the two segments of one chord is equal to the product of the lengths of the two segments of the other chord.
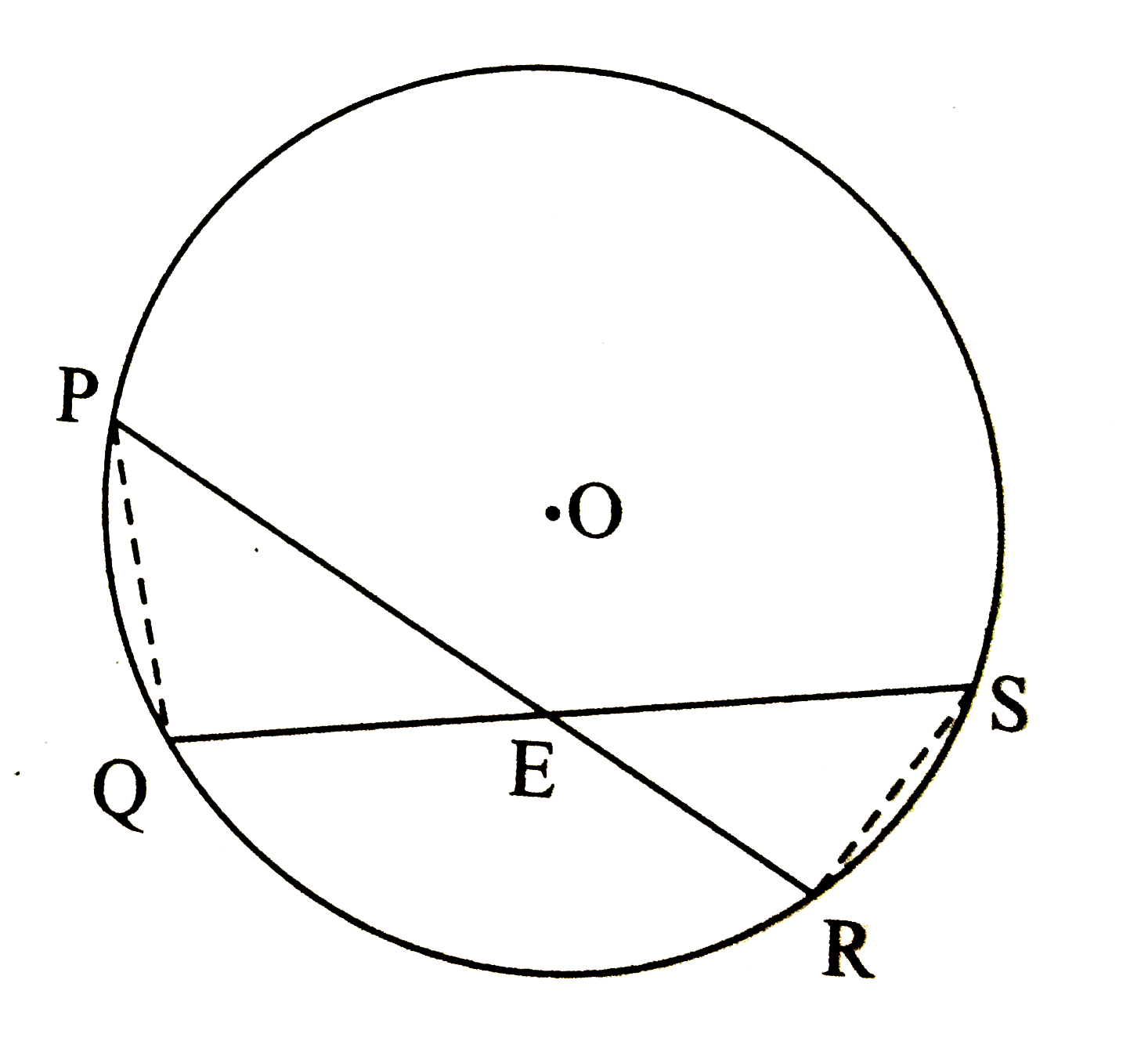
Given : (1) A circle with centre O .
(2) chords PR and QS intersect at point E inside the circle.
To prove : PE × ER = QE xx ES <br>Construction: Draw seg PQ and seg RS
View SolutionIn a circle, two chords PQ and RS bisect each other. Then PRQR is _____________.
View SolutionIn the figure, chords PQ and RS intersect at T as shown. If ∠PSR=42∘ and m(arc SQ)=42∘, then ∠STQ=…….
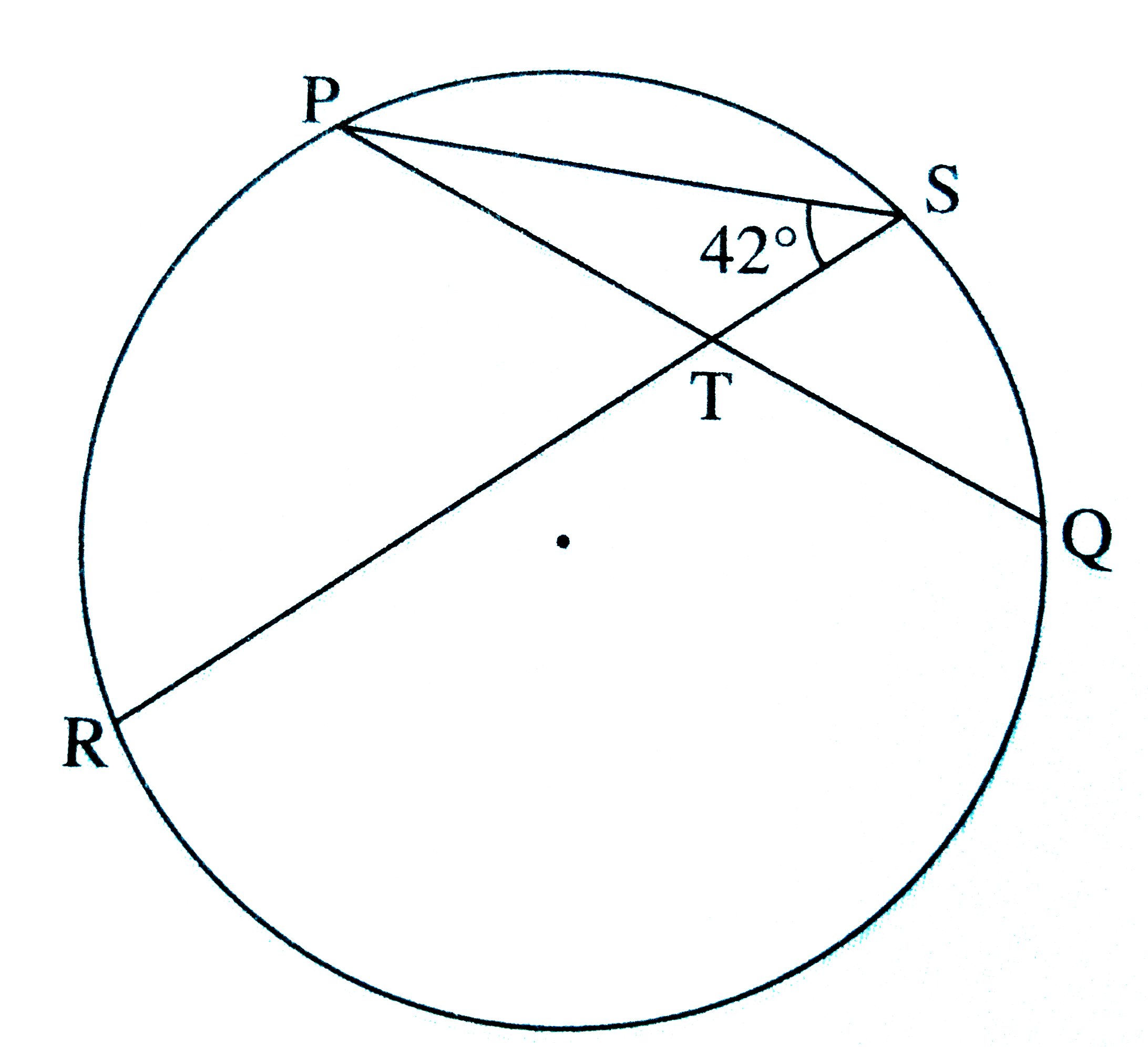
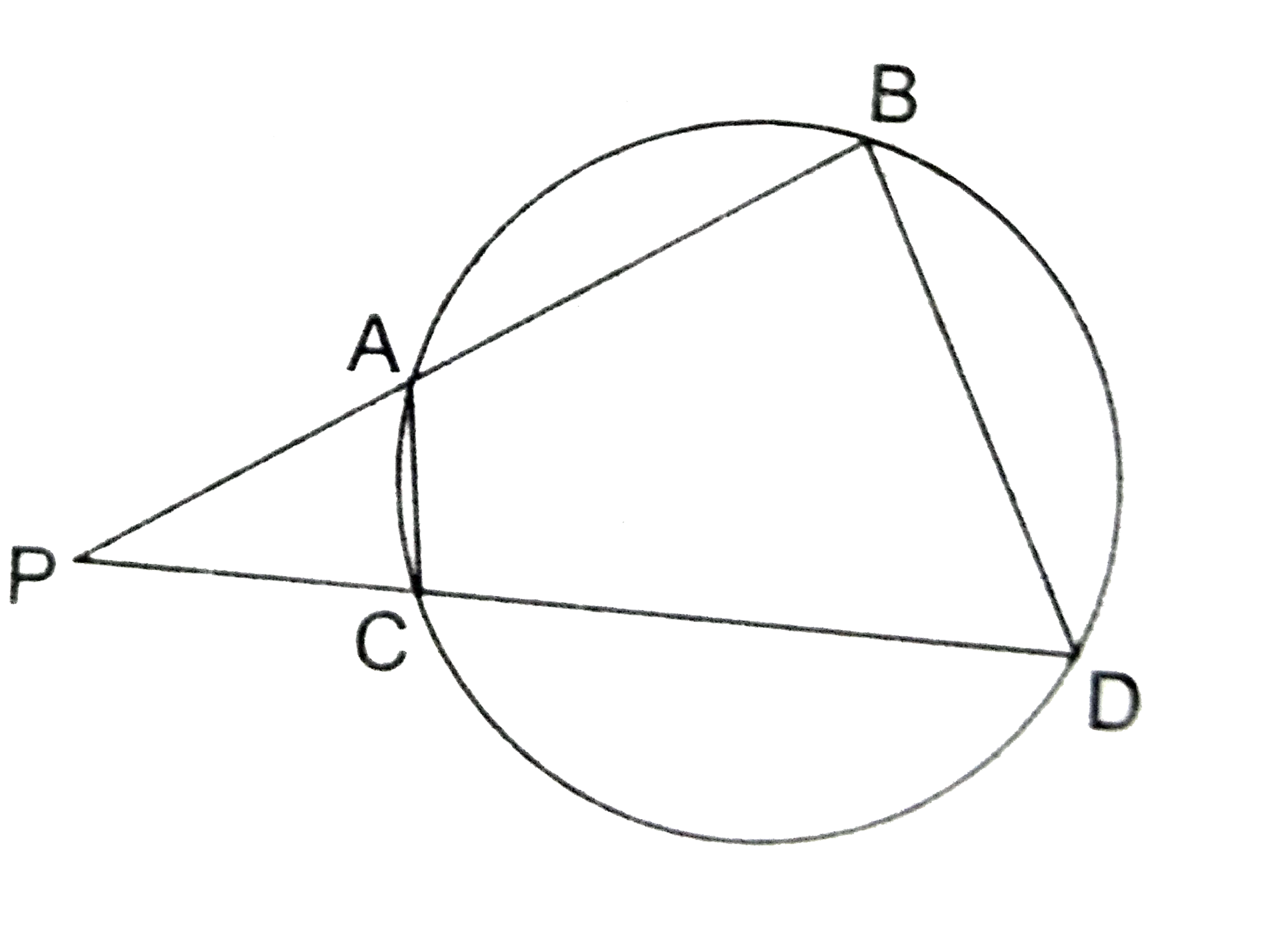
View SolutionTwo chords AB and CD of a circle intersect at a point outside the circle. Prove that
(a)ΔPAC~ΔPDB (b)PA⋅PB=PC⋅PD.
View Solution
