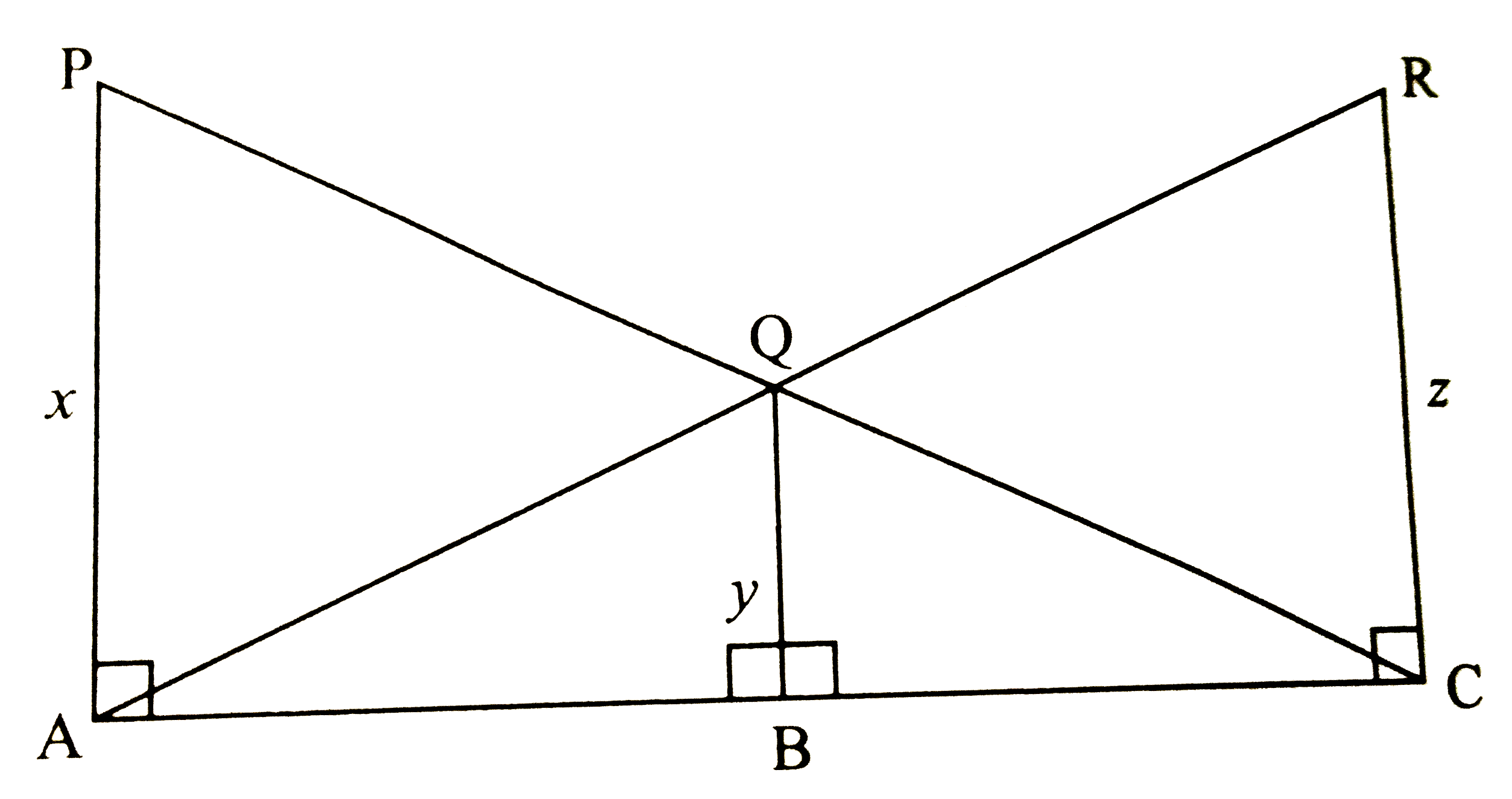
In the above figure, seg PA, seg QB and seg RC are perpendicular to seg AC. From the information given in the figure, prove that:
1x+1z=1y
In ΔPAC and ΔQBC
∠PAC≅∠QBC….(given)
∠PCA≅∠QCB….(Common angle)
∴ΔPAC~ΔQBC …..(By AA test)
∴PAQB=ACBC….(Corresponding sides of similar tirangles are in proportion)
xy=ACBC
yx=BCAC...(I) ...(by invertendo)
Similarly, ΔRCA~ΔQBA..... (By AA test)
RCQB=ACAB...(Corresponding sides of similar triangles are in proportion
∴zy=ACAB
∴yz=ABAC.....(II)....(by invertendo)
∴yx+yz=BCAC+ABAC [Adding (I) and (II)]
∴y(1x+1Z)=BC+ABAC
∴y(1x+1z)=ACAC(A−B−C)
∴(1x+1z)=1
∴1x+1z=1y
Topper's Solved these Questions
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MODEL QUESTION PAPEREXERCISE - SOLVE THE FOLLOWING SUBQUESTIONS: (ANY ONE)2videos
MODEL QUESTION PAPER
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MODEL QUESTION PAPEREXERCISE - SOLVE ANY TWO OF THE FOLLOWING SUBQUESTIONS:4videos
MODEL QUESTION PAPER
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MENSURATIONEXERCISE - Assignment 8.58videos
MENSURATION
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PRACTICE QUESTION BASEDEXERCISE - STATISTICS13videos
PRACTICE QUESTION BASED
Similar Questions
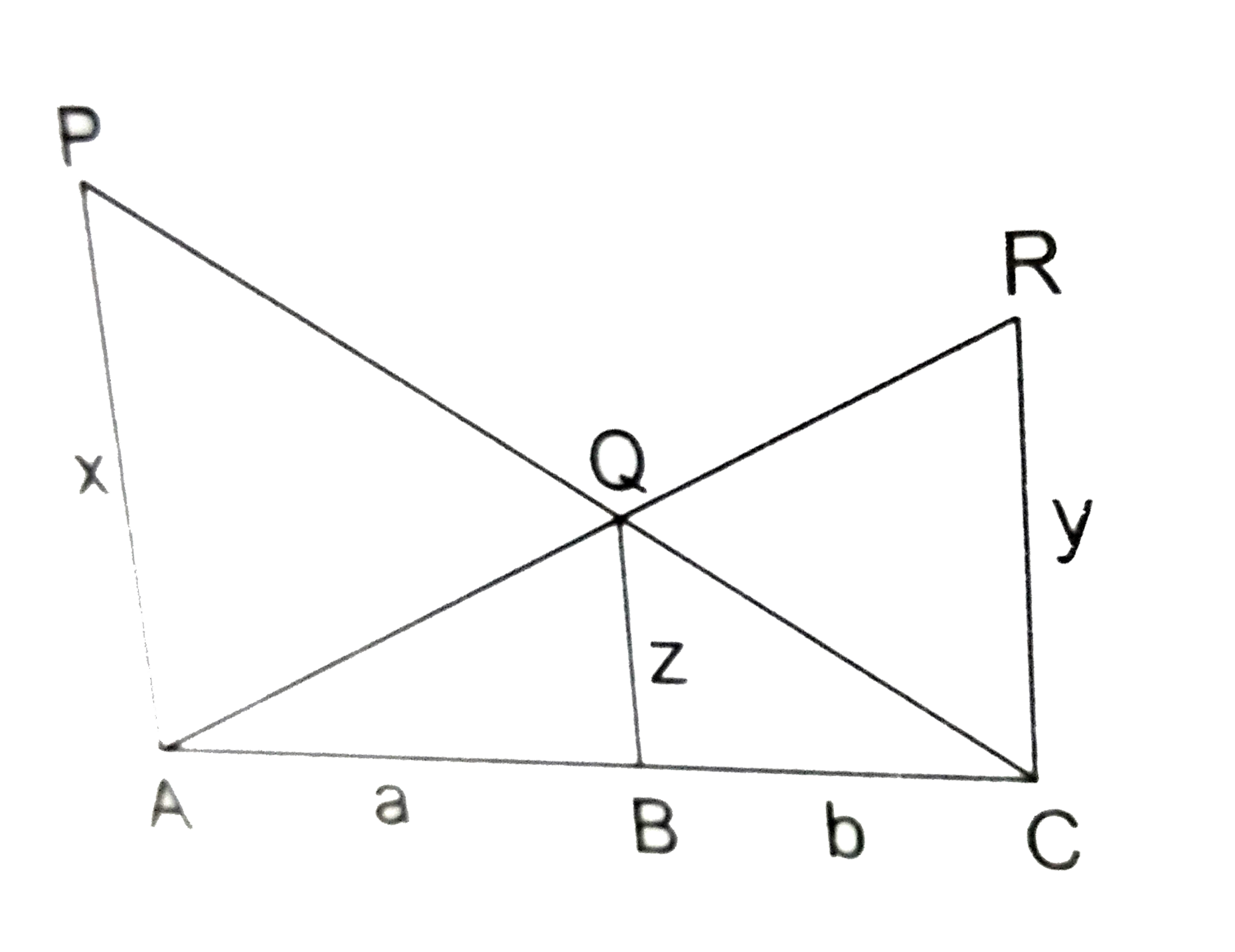
In the given figure, PA, QB and RC each is perpendicular to AC such that PA=x,RC=y,QB=z,AB=a,andBC=b
Prove that 1x+1y=1z
 View Solution
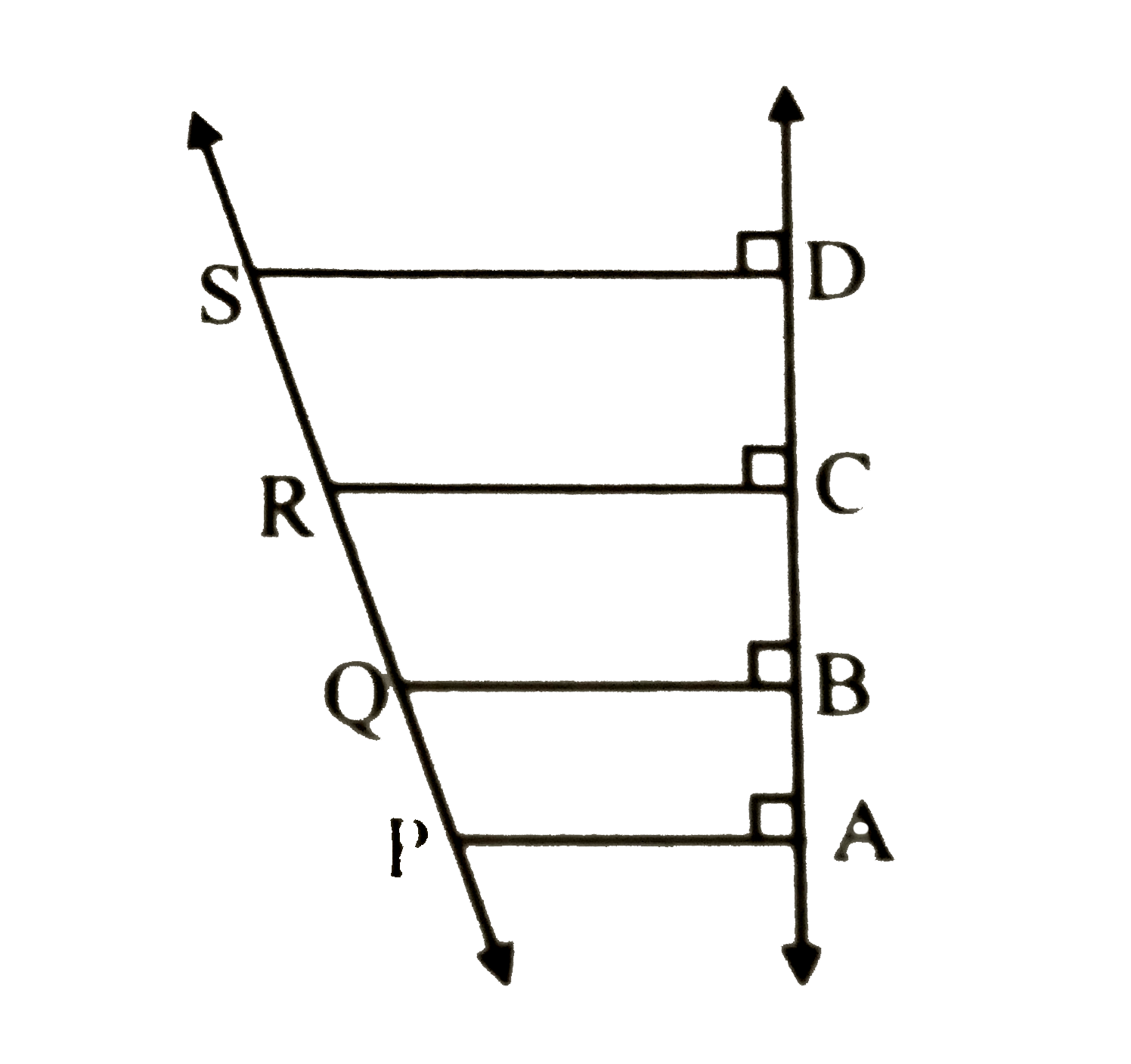
View SolutionIn the adjoining figure, seg PA , seg QB, seg RC and seg SD are perpendicular to line AD. AB = 60, BC = 70, CD = 80, PS = 280, then find PQ, QR and RS.
 View Solution
View SolutionIn the given figure, each of PA, QB and RC is perpendicular to Ac. If AP=x,QB=z,RC=y,AB=aandBC=b, show that 1x+1y=1z
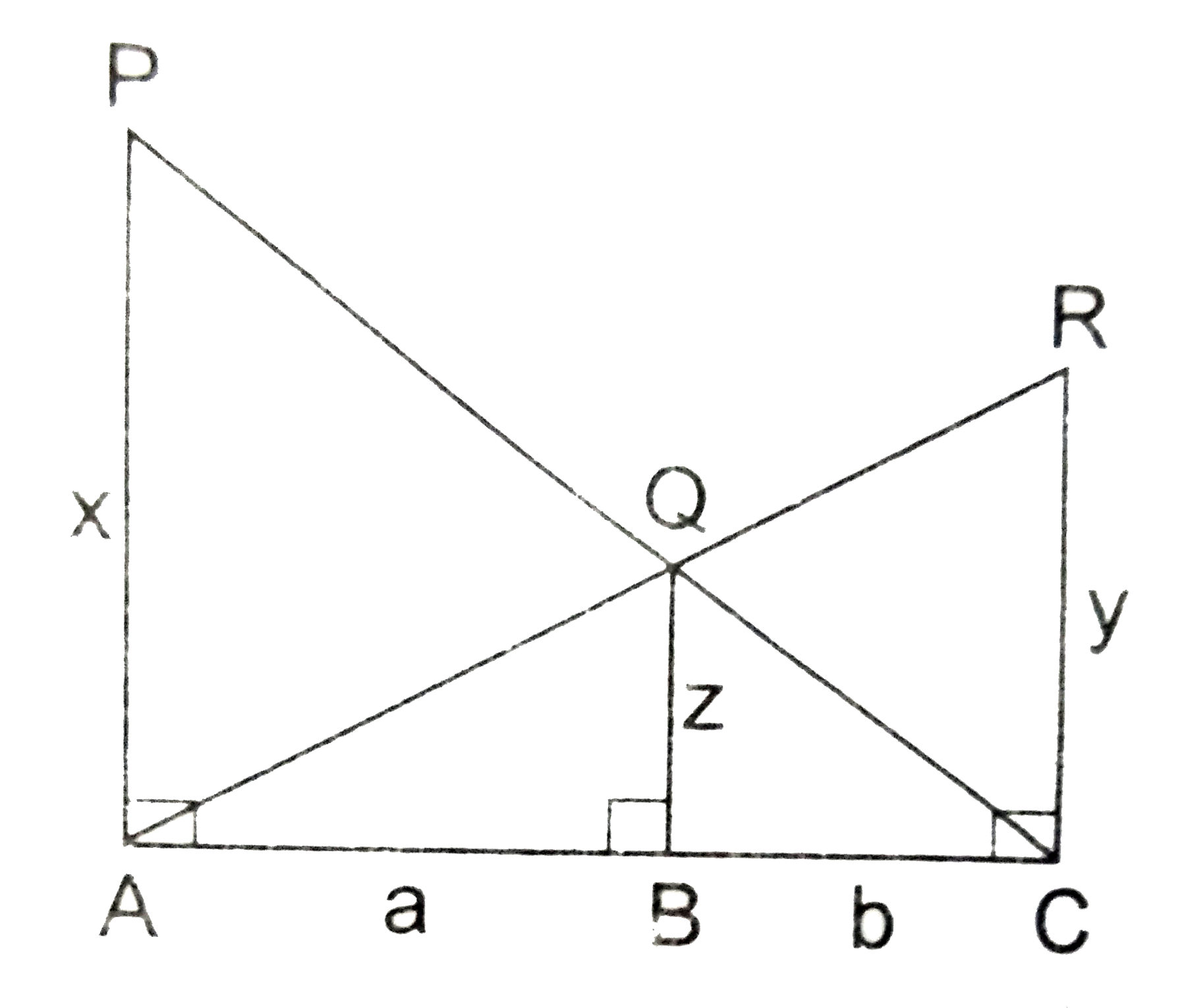 View Solution
View Solution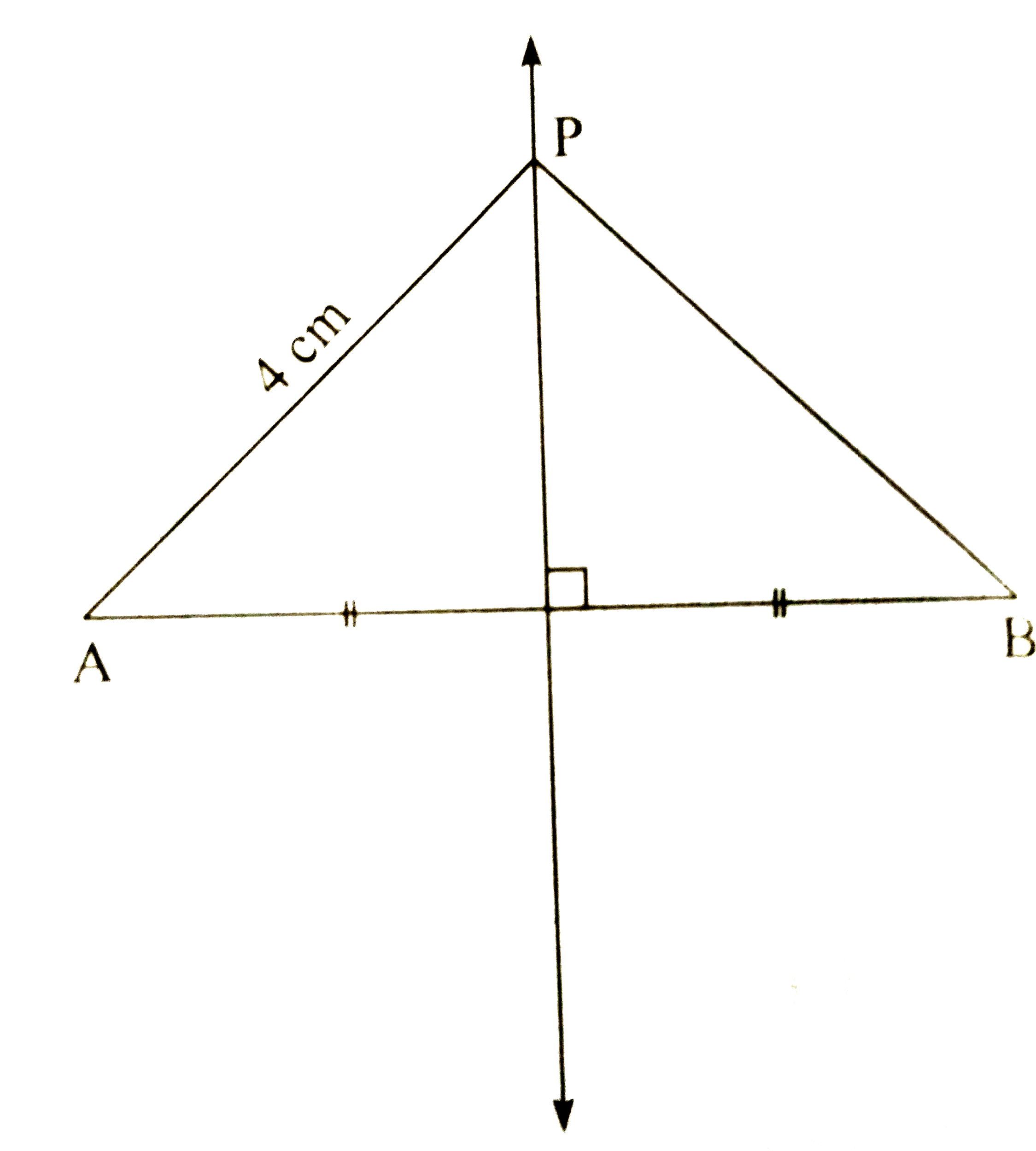
In the figure , point P lies on the perpendicular bisector of seg AB. If PA =4 cm , find PB. State reason .View SolutionIn the figure, seg AB≅ seg AC, ray CE bisects ∠ACB , ray BD bisects ∠ABC. Prove that ray ED∣∣ side BC.
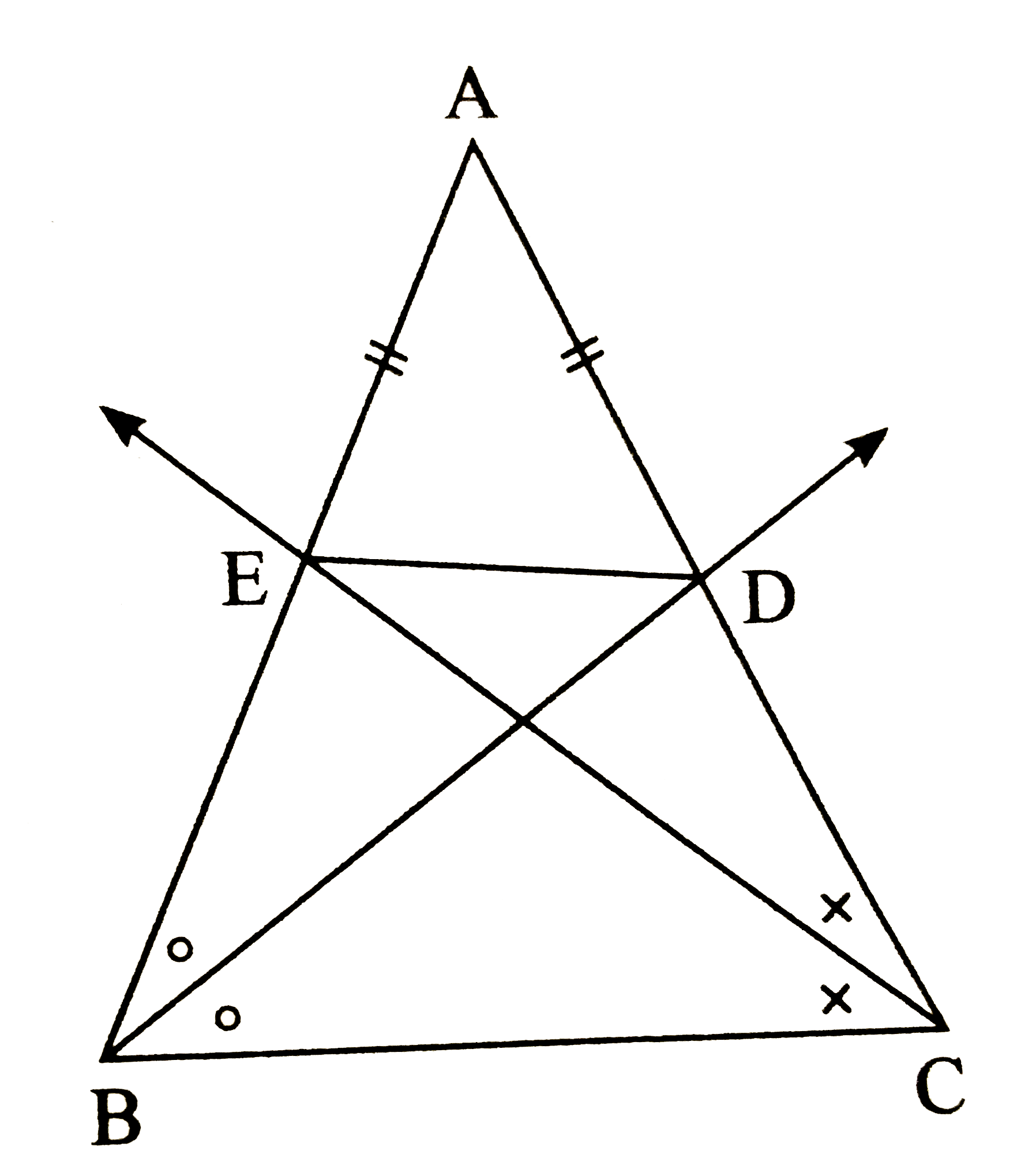 View Solution
View SolutionDraw seg AB of length 5.1 cm. Draw its perpendicular bisector
View SolutionIn the given figure, ∠P≅∠R , seg PQ ≅ seg RQ. Prove that ΔPQT≅ΔRQS .
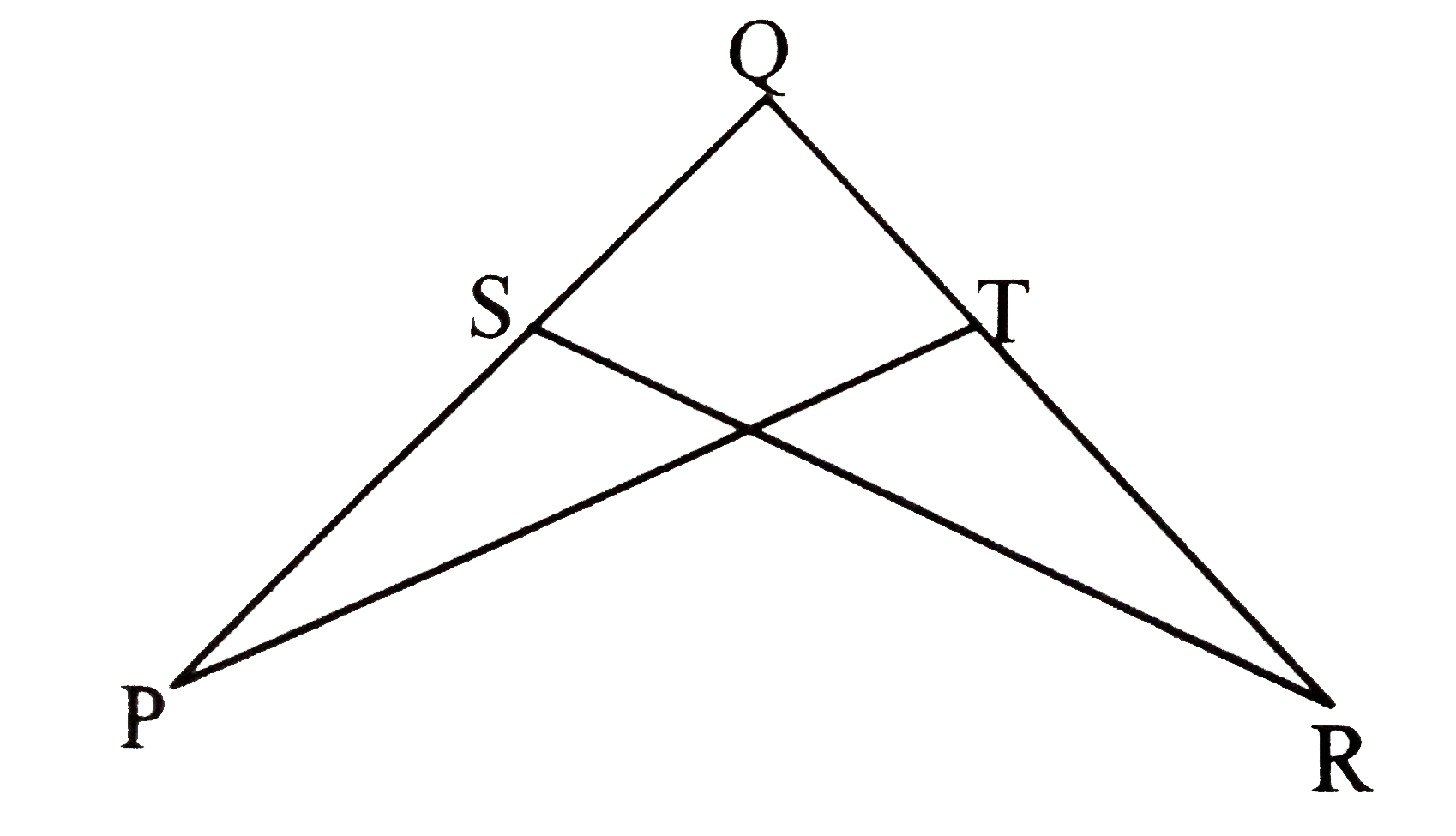 View Solution
View SolutionIn the adjoining figure , seg EF is a diameter and seg DF is a tangent segment. The radius of the circle is r. Prove that, DE×GE=4r2.
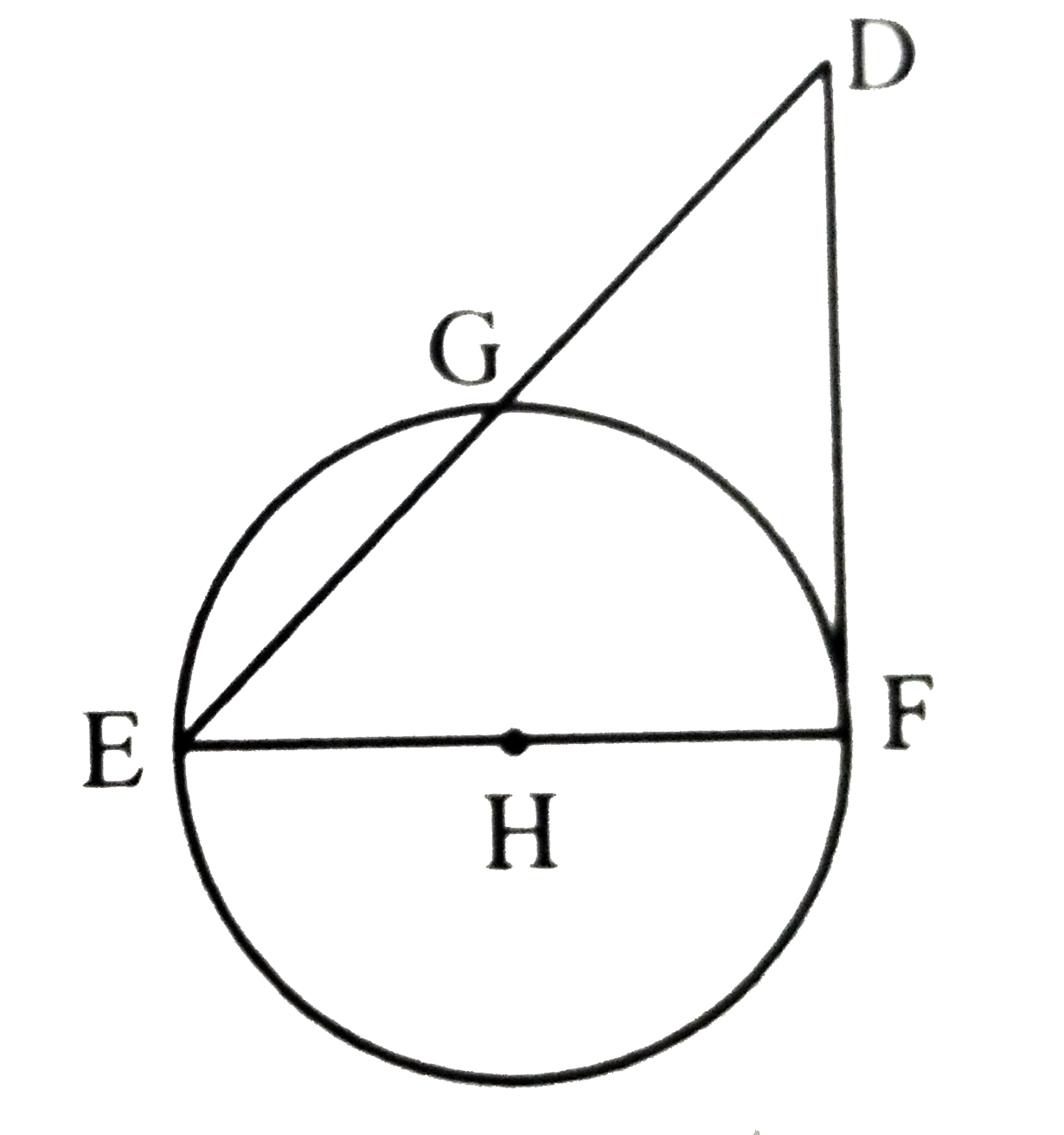 View Solution
View SolutionIn the adjoining figure, seg XY∣∣ seg AC, IF 3AX=2BX and XY=9 then find the length of AC.
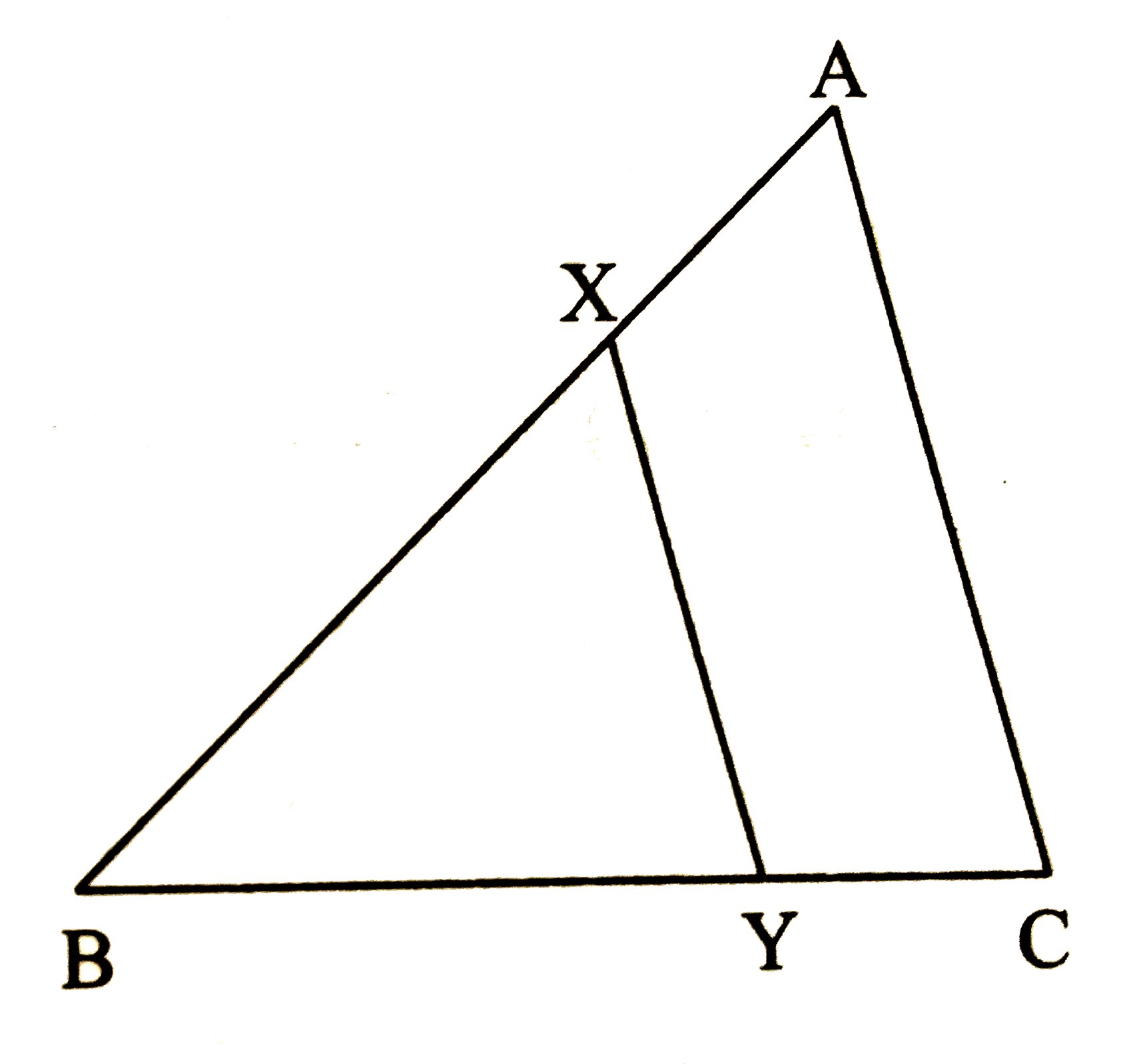 View Solution
View SolutionIn figure, seg EF is a diameter and seg DF is a tangent segment. The radius of the circle is r. Prove that, DE×GE=4r2.
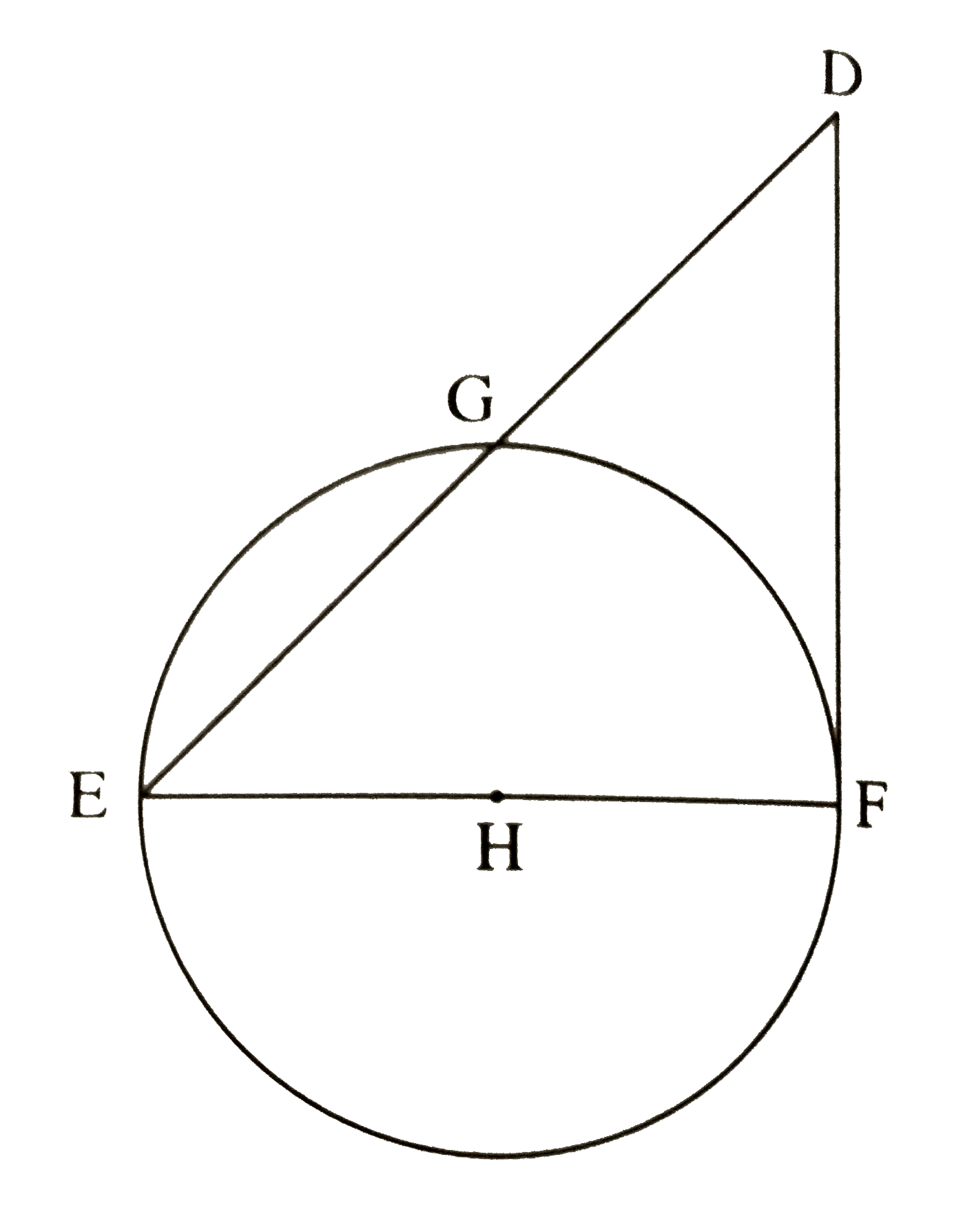 View Solution
View Solution

