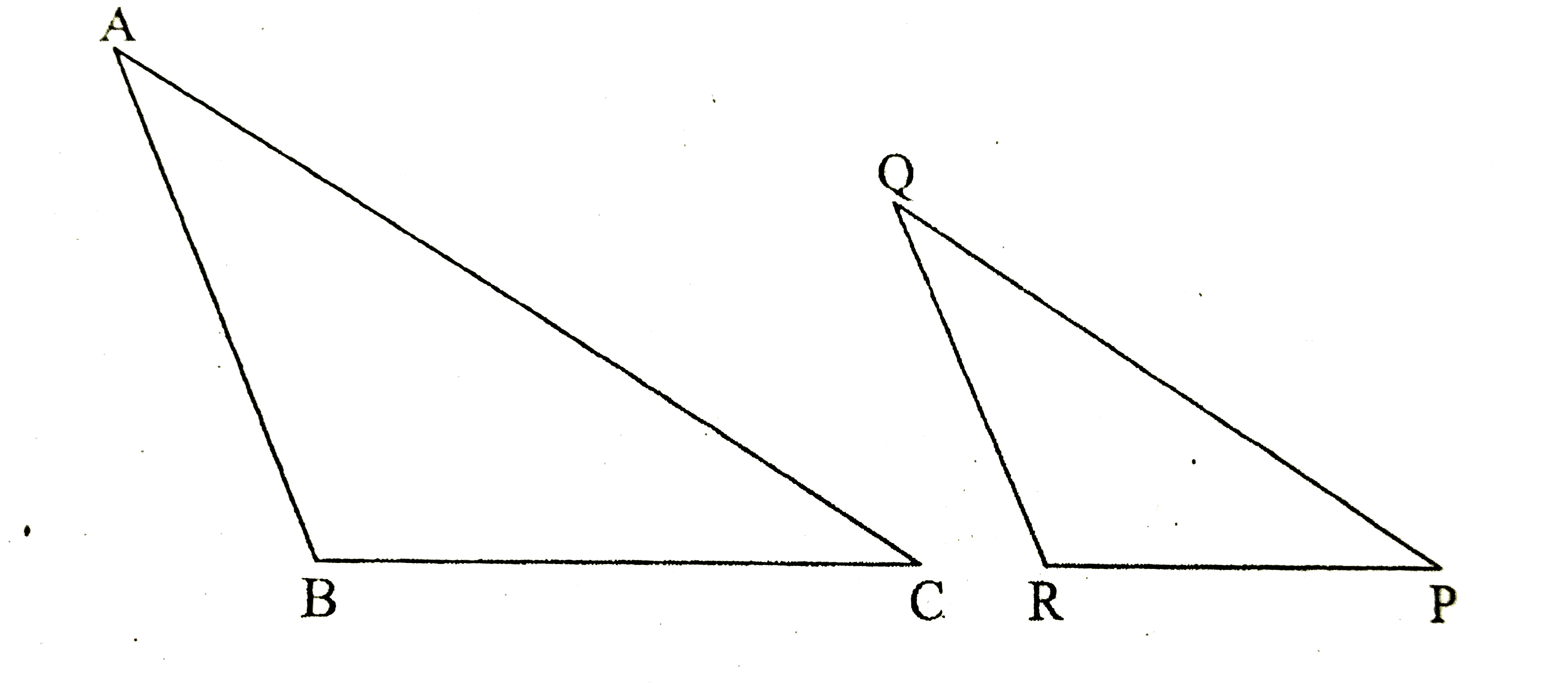
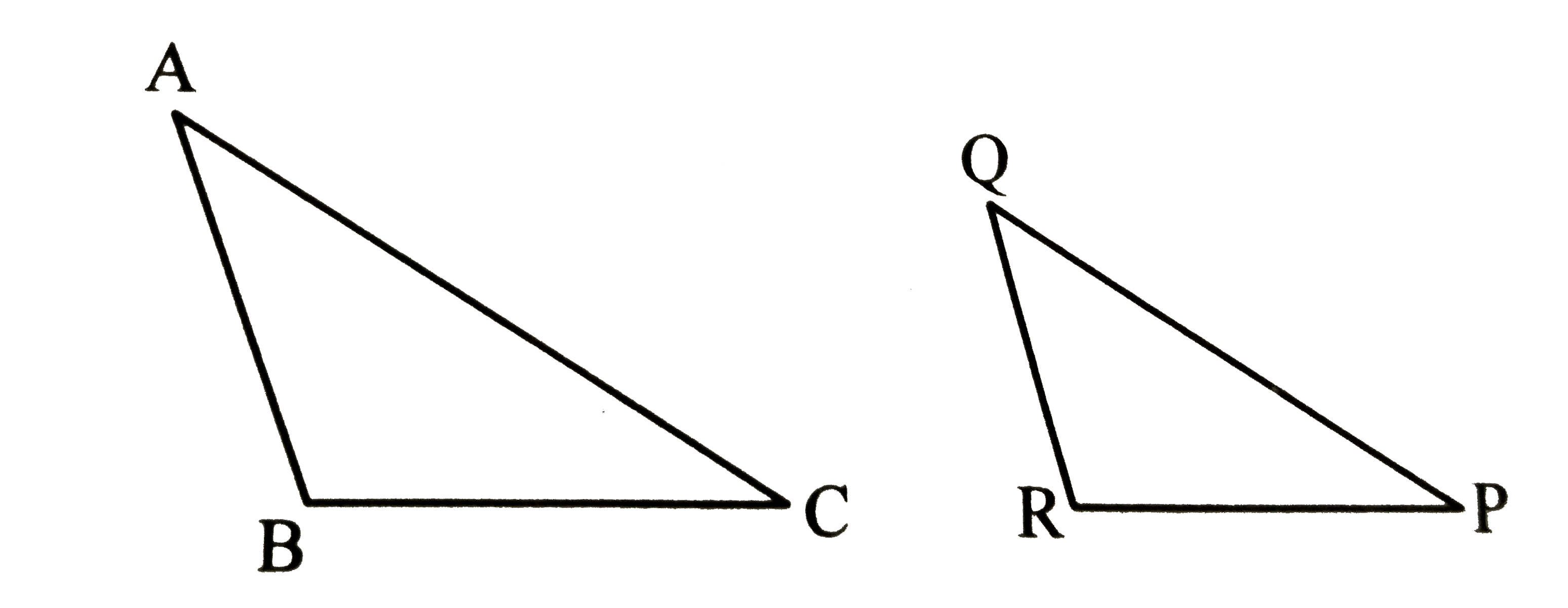
In $Δ A B C$ and $Δ P Q R$ in a one-to-one correspndence $\frac{A B}{Q R} = \frac{B C}{P R} = \frac{C A}{P Q}$ then

A

$Δ P Q R ~ Δ A B C$

B

$Δ P Q R ~ Δ C A B$

C

$Δ C B A ~ Δ P Q R$

D

$Δ B C A ~ Δ P Q R$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

|

Answer

Step by step video & image solution for In DeltaABC and DeltaPQR in a one-to-one correspndence (AB)/(QR)=(BC)/(PR)=(CA)/(PQ) then by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.

Updated on:21/07/2023

Class 10 MATHS TRIANGLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If in two $Δ A B C$ and $Δ P Q R$ , $\frac{A B}{Q R} = \frac{B C}{P R} = \frac{C A}{P Q}$ , then
A $Δ P Q R ~ Δ C A B$
B $Δ P Q R ~ Δ A B C$
C $Δ C B A ~ Δ P Q R$
D $Δ B C A ~ Δ P Q R$
Question 2 - Select One
Choose the correct alternative.
$Δ A B C and Δ P Q R$ are equilateral triangles. If $A (Δ A B C) : A (Δ P Q R)$ = 1 : 16, and AB = 2 cm, then what is the length of PR?
A4 cm
B2 cm
C6 cm
D8cm
Question 3 - Select One
Assertion : In $Δ A B C and Δ P Q R, A B = P Q A C = P R and ∠ B A C = ∠ Q P R$
$∴ Δ A B C ≅ Δ P Q R$
Reason : Both the triangles are congruent by SSS congruence.
AIf both assertion and reason are true and reason is the correct explanation of assertion.
BIf both assertion and reason are true but reason is not the correct explanation of assertion.
CIf assertion is true but reason is false.
DIf assertion is false but reason is true.

NAVNEET PUBLICATION - MAHARASHTRA BOARD-SIMILARITY-EXAMPLE TYPE

Home
Ask Doubt
Profile