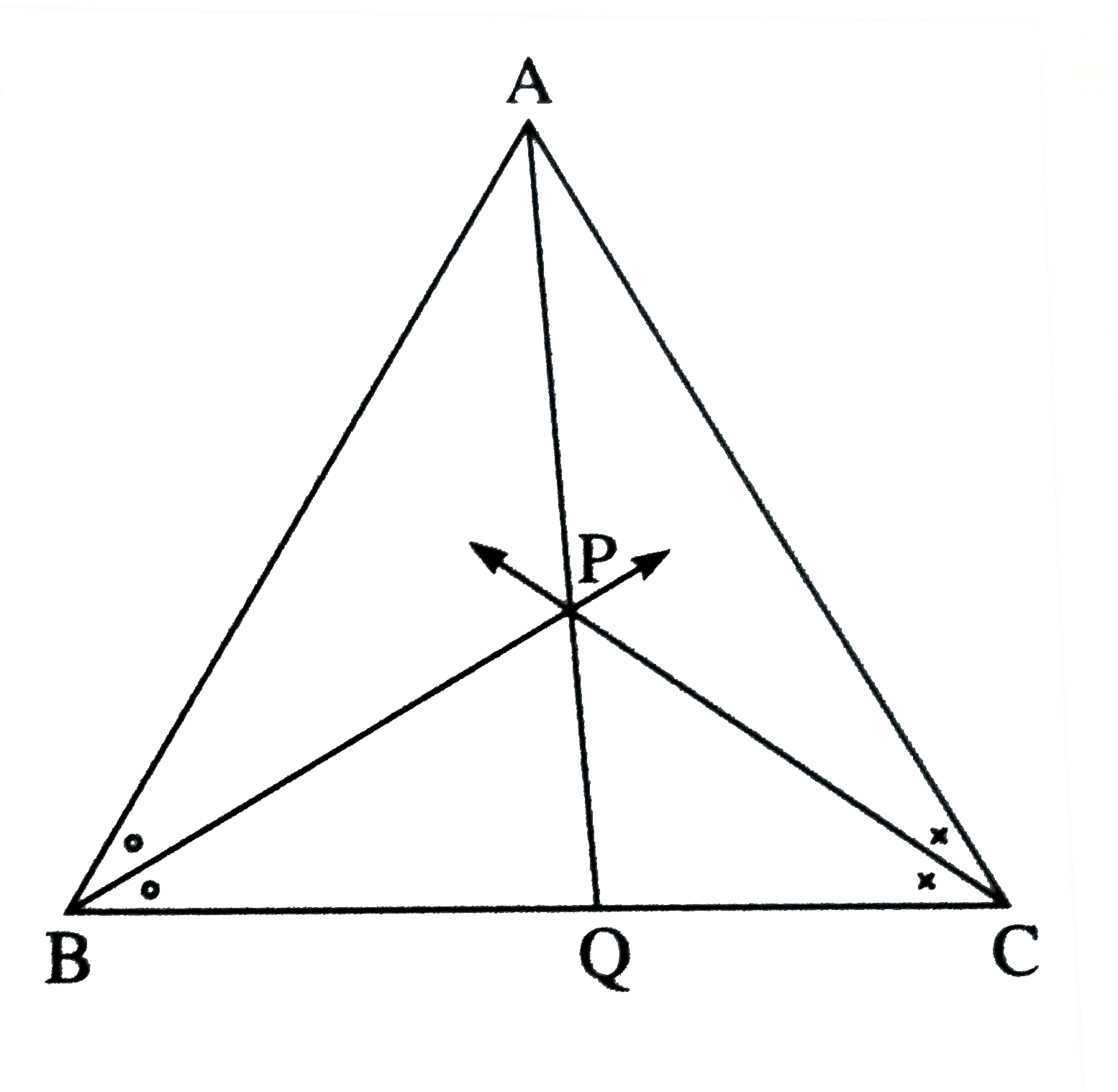
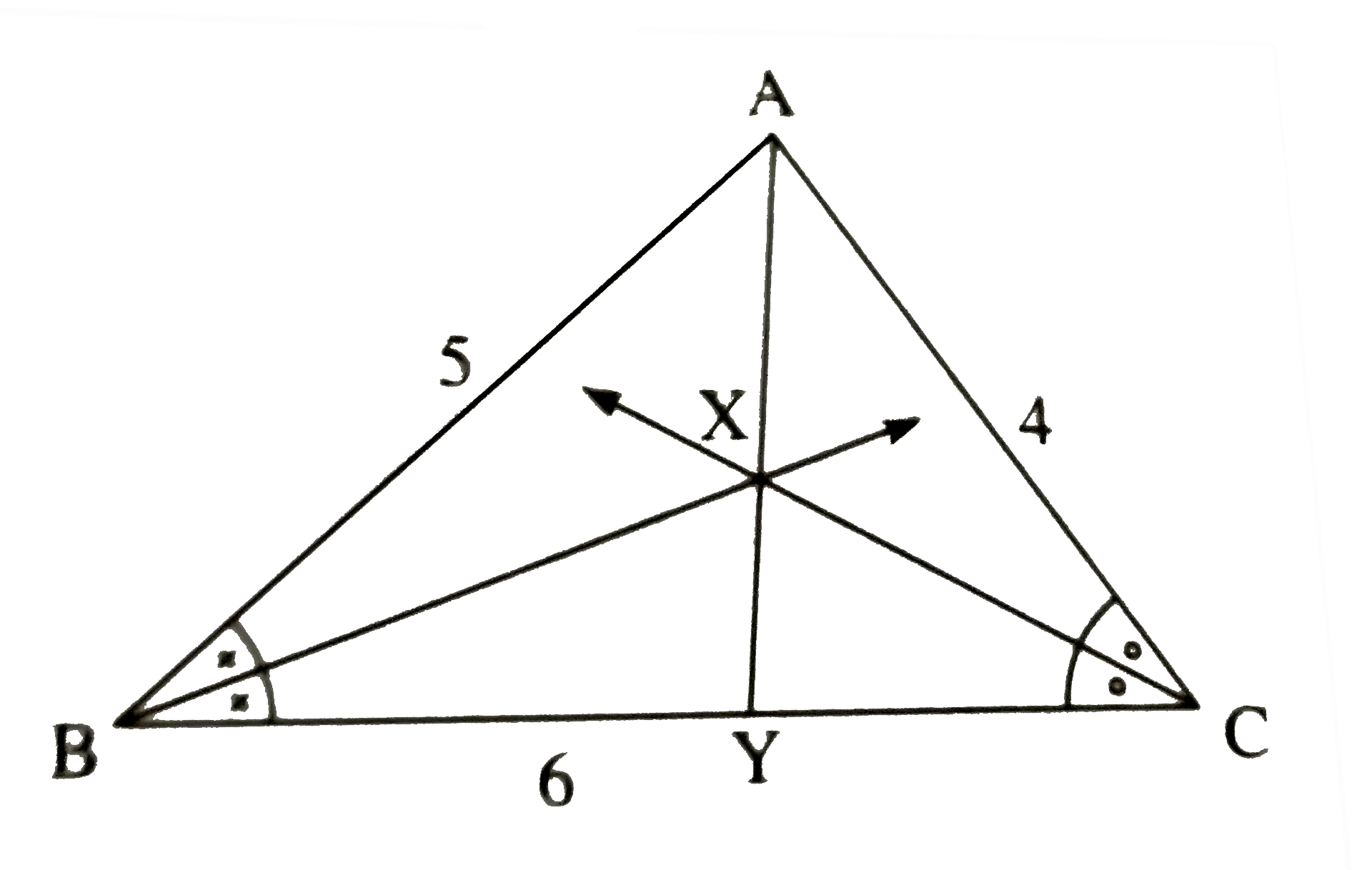
In the figure bisectors of ∠B and ∠C of ΔABC intersect each other in point X. Line AX intersects side BC in pont Y. AB=5,AC=4,BC=6 then find AXXY
Video Solution
Text Solution
In ΔABY,
ray BX bisects ∠ABY ………..(Given)
∴ by the theorem of angle bisector of a triangle,
ABBY=AXXY……………1
In ΔACY
ray CX bisects ∠ACY…..(Given)
∴ by the theorem of angle bisector of a triangle,
ACCY=AXXY ..............2
ABBY=ACCY=AXXY..........[From 1 and 2]
∴5BY=4CY=AXXY
By theorem on equal ratios, ltbr 5+4BY+CY=AXXY
∴9BC=AXXY..........(B−Y−C)
∴96=AXXY
∴AXXY=32
AXXY=32
|
Topper's Solved these Questions
- SIMILARITYBook:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:SIMILARITYExercise:CHALLENGING QUESTIONS
Explore 8 Videos
- SIMILARITYBook:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:SIMILARITYExercise:MCQ TYPE
Explore 15 Videos
- SIMILARITYBook:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:SIMILARITYExercise:2.5 (4 mark each)
Explore 3 Videos
- QUADRATIC EQUATIONS Book:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:QUADRATIC EQUATIONS Exercise:ASSIGNEMENT 2.4
Explore 8 Videos
- STATISTICSBook:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:STATISTICSExercise:EXAMPLES FOR PRACTICE (MCQs)
Explore 35 Videos
Similar Questions
Knowledge Check
In a ΔABC, the bisectors of ∠Band∠C intersect each other point O. Then∠BOC is ?
A∠BOC=180∘+12∠A.B∠BOC=90∘+12∠A.C∠BOC=180∘−12∠A.D∠BOC=90∘−12∠A.The bisector of the exterior ∠A of ΔABC intersects the side BC produced to D. Here CF is parallel to AD.
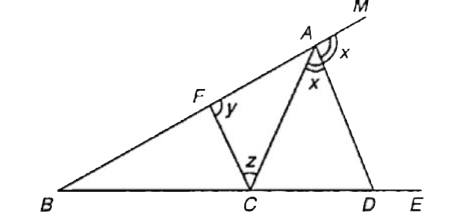 AABAC=BDCDBABAC=CDBDCABAC=BCCDDnone of these
AABAC=BDCDBABAC=CDBDCABAC=BCCDDnone of theseMid-points of the sides AB and AC of a ΔABC are (3, 5) and (-3, -3) respectively, then the length of the side BC is
A10 unitB20 unitC15 unitD30 unit
The bisectors of ∠Band∠C of an isosceles ΔABC with AB=AC intersect each other at point O. Show that the exterior angle adjacent to ∠ABC is equal to ∠BOC.
View SolutionIn ΔABC, bisector of ∠A intersects BC at D. If AB = 4 cm, AC = 3 cm and ∠A=60∘, then find the length of AD-
View SolutionIn ABC , the bisector of ∠A intersects BC in D . If AB=18cm , AC=15cm and BC=22cm , find BD
View SolutionIn ΔABC, D is the midpoint of side AB. Line DE∣∣ side BC. A−E−C. Prove that pont E is the midpoint of side AC.
View SolutionIn the figure AD is the external bisector of ∠EAC, inttersects BC produced to D. If AB=12cmandBC=4cm, find CD.
View Solution