Question

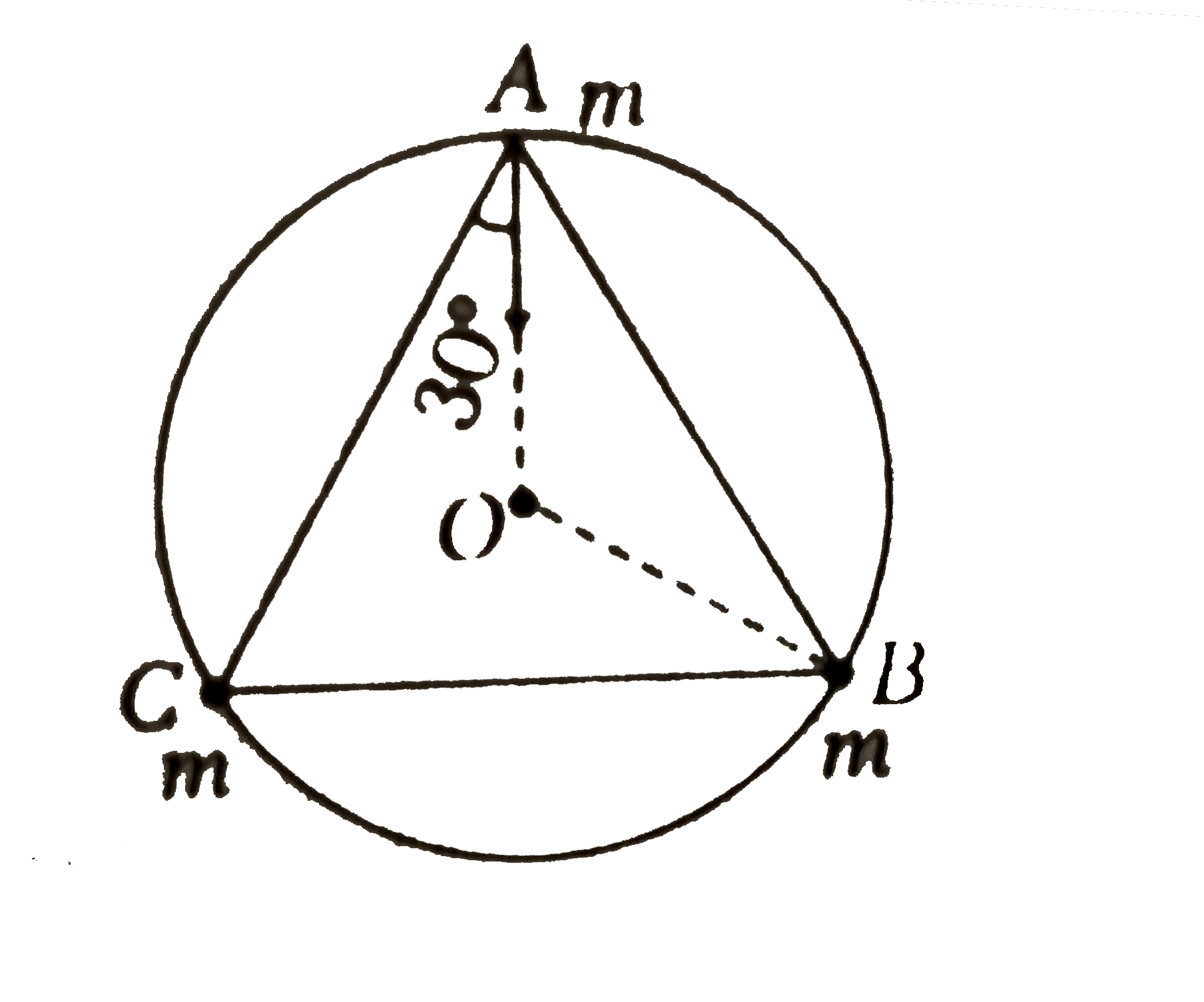
Three indentical bodes of mass M are locatd at the verticles of an equailateral triangle of side L they revolve undr the effect of mutual gravitational force in a circular orbit circumscibing the traingle while preserving the equilateral triangle their orbital velocity is

Options

A. `sqrt(GM)/(L)`
B. `sqrt(3GM)//(2L)`
C. `sqrt(3GM)/(L)`
D. `sqrt(2GM)/(3L)`

Answer

A

Explanation

To find the orbital velocity of three identical bodies of mass $$M$$ located at the vertices of an equilateral triangle of side $$L$$, we can follow these steps:

Step 1: Understand the System
We have three identical masses \( M \) at the vertices of an equilateral triangle with side length \( L \). They are revolving around a common center (the centroid of the triangle) in a circular orbit.

Step 2: Determine the Centripetal Force
Each mass experiences gravitational forces from the other two masses. The net force acting on each mass provides the necessary centripetal force to keep it in circular motion.

Step 3: Calculate the Gravitational Force
The gravitational force \( F \) between any two masses \( M \) separated by distance \( L \) is given by:
\( F = \frac{G M^2}{L^2} \)
where \( G \) is the gravitational constant.

Step 4: Analyze the Forces Acting on One Mass
Each mass experiences gravitational attraction from the other two masses. The angle between the forces due to the two other masses is \( 60^\circ \) (since the triangle is equilateral).

The net force \( F_{\text{net}} \) acting on one mass can be calculated using the formula for the resultant of two forces:
\( F_{\text{net}} = \sqrt{F^2 + F^2 + 2F \cdot F \cdot \cos(60^\circ)} \)
Substituting \( \cos(60^\circ) = \frac{1}{2} \):
\( F_{\text{net}} = \sqrt{F^2 + F^2 + 2F^2 \cdot \frac{1}{2}} = \sqrt{2F^2 + F^2} = \sqrt{3F^2} = \sqrt{3}F \)

Step 5: Relate the Net Force to Centripetal Force
The net gravitational force \( F_{\text{net}} \) acts as the centripetal force required for circular motion:
\( F_{\text{net}} = M \cdot \frac{V^2}{R} \)
where \( R \) is the radius of the circular path. For an equilateral triangle, the distance from the centroid to a vertex is given by:
\( R = \frac{L}{\sqrt{3}} \)

Step 6: Set Up the Equation
Equating the net force to the centripetal force:
\( \sqrt{3}F = M \cdot \frac{V^2}{R} \)
Substituting \( F = \frac{G M^2}{L^2} \):
\( \sqrt{3} \cdot \frac{G M^2}{L^2} = M \cdot \frac{V^2}{\frac{L}{\sqrt{3}}} \)

Step 7: Simplify the Equation
Rearranging gives:
\( \sqrt{3} \cdot \frac{G M^2}{L^2} = M \cdot \frac{V^2 \sqrt{3}}{L} \)
Cancelling \( M \) and \( \sqrt{3} \):
\( \frac{G M}{L^2} = \frac{V^2}{L} \)
Multiplying both sides by \( L \):
\( \frac{G M}{L} = V^2 \)

Step 8: Solve for Velocity
Taking the square root of both sides gives:
\( V = \sqrt{\frac{G M}{L}} \)

Final Answer
The orbital velocity \( V \) of each mass is:
\( V = \sqrt{\frac{G M}{L}} \)