A ball A of mass $m$ is suspended by a thread of length $r = 1.2 m$ . Another ball $B$ of mass $2 m$ is projected from the ground with velocity it $u = 9 m / s$ such that at the highest point of its trajectory it collides head-on elastically with ball $A$ . It is observed that during subsequent motion, tension in the thread at the highest point is equal to $m g$ .

The height of the point of suspension of ball $A$ from the ground is

A

$\frac{81}{40} m$

B

$\frac{129}{40} m$

C

$\frac{81}{20} m$

D

$\frac{101}{40} m$

Text Solution

Verified by Experts

The correct Answer is:B

Let velocity of ball $A$ at highest point be $v$ then first considering its $F B D$ at this point

$m g + T = \frac{m v^{2}}{r}$
where $T = m g$
$\Rightarrow v^{2} = 2 r g$
$\Rightarrow v = \sqrt{2 r g} = 2 \sqrt{6} m / s$
If the velocity of ball $A$ just after the collisin is $v_{1}$ then according to law of conservation of energy its $K E$ at $A = (K E + P E)$ at highest point
$\frac{1}{2} m v_{1}^{2} = \frac{1}{2} m v^{2} + m g (2 r)$
$v_{1} = \sqrt{6 r g}$ or $v_{1} = 6 \sqrt{2} m / s$
If angle of project of ball $B$ is $θ$ , then horizontal component of its velocity will be $9 cos θ$ and it remains constant.

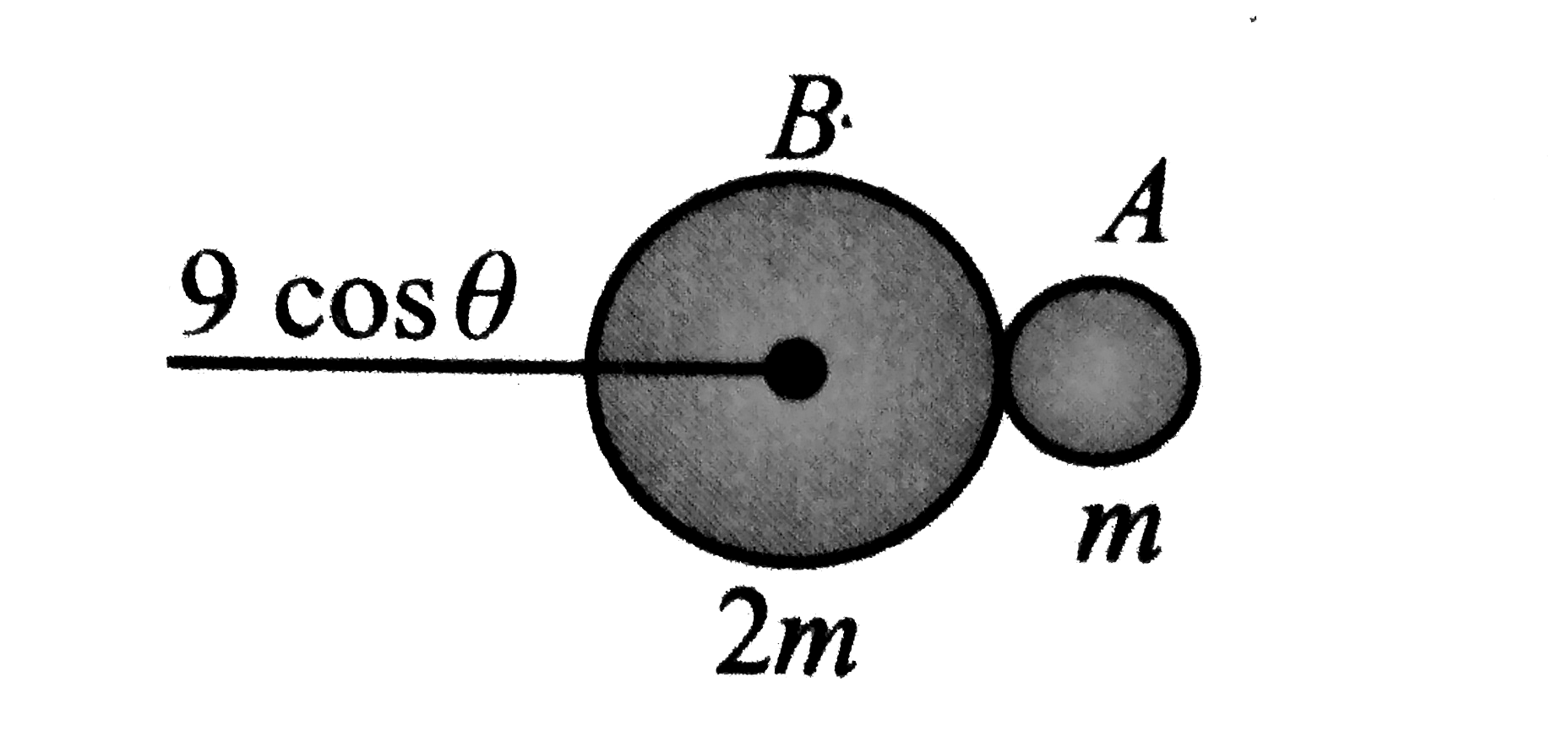
Now considering collision of balls $B$ and $A$ , let velocities of balls $A$ and $B$ , just after the collision, be $v_{1}$ and $v_{2}$ respectively as shown in figure.
Then according to the law of conservation of momentum
$m v_{1} + 2 m v_{2} = 2 m (9 cos θ)$ ...........i
Since collision is elastic, therefore $e = 1$
$\frac{v_{1} - v_{2}}{9 cos θ} = 1$ or $v_{1} - v_{2} = 9 cos θ$ ........ii
Solving eqn i and ii we get
$cos θ = \frac{1}{\sqrt{2}}$ or $θ = 45^{\circ}$
$v_{2} = \frac{3}{\sqrt{2}} m s^{- 1}$
height of initial position of ball $A$ (lowest position) is equal to maximum height ascened by the ball $B$ . It is equal to
$H = \frac{{(9 sin θ)}^{2}}{2 g} = \frac{81}{40} m$
Height of point of suspension from the ground is
$H = r = \frac{129}{40} m$

Updated on:21/07/2023

|

Class 11 PHYSICS CENTRE OF MASS

Topper's Solved these Questions

Similar Questions

CENGAGE PHYSICS-CENTRE OF MASS-Linked Comprehension

Home
Ask Doubt
Profile