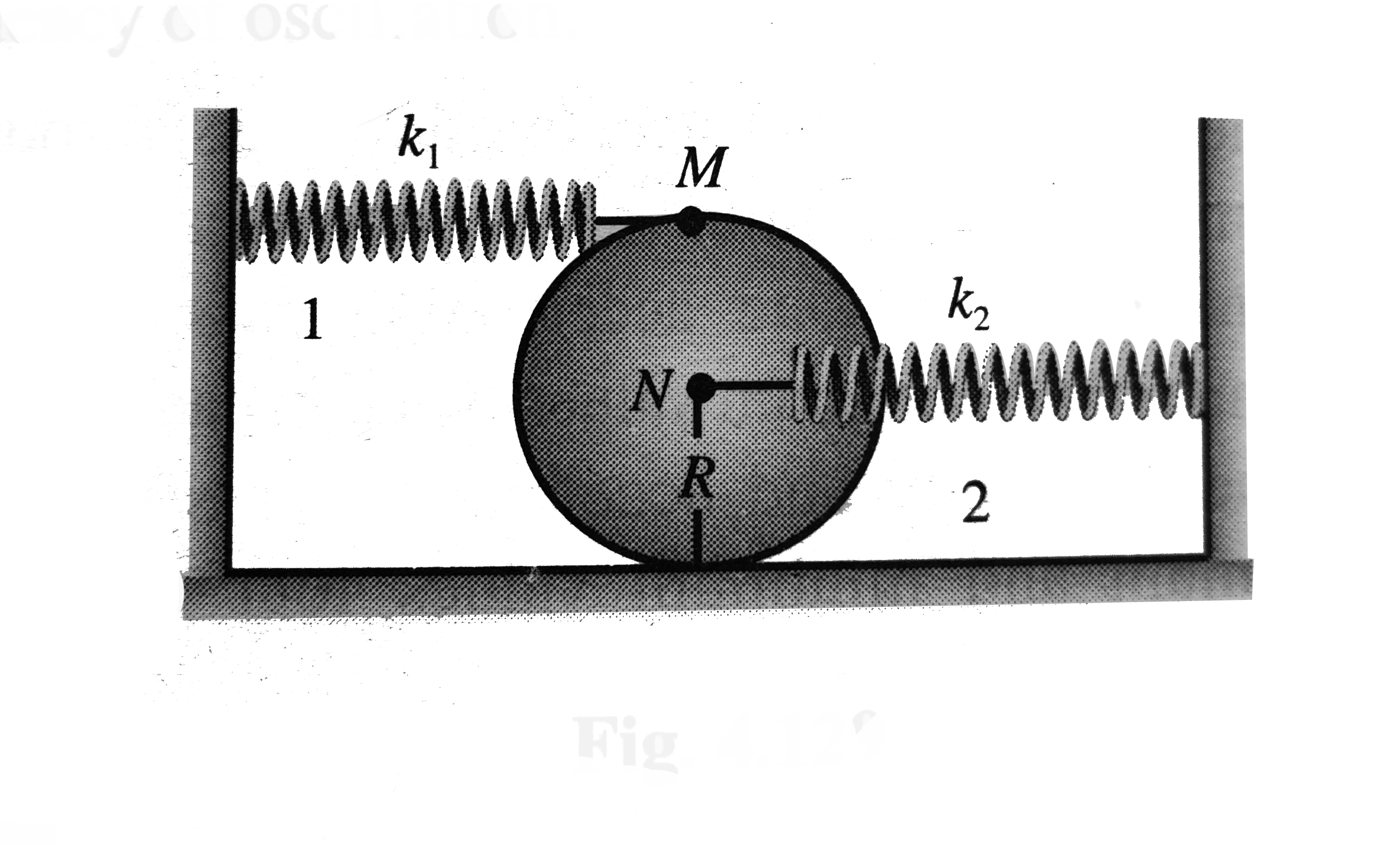
A uniform dise of mass m and radius R is connected with two light springs 1 and 2. The springs are connected at the highest point M and the CM 'N' of the dise. The other ends of the springs are rigidly attached with vertical walls. If we shift the CM in horizontalby a small distance , the discoscillates simple harmonically. Assuming a perfect rolling of the dise on the horizontal surface , find the angular frequency of oscillation.
The correct Answer is:√2(4k1+k2)3m
Let us displace the CM by asmall distance x towards right. As a result the body rolls on the horizontalsurface. Hence spring 1 is elonged by x1=2Rθ and spring 2 is compressed by x1=2Rθ, where θ is the angle of rotation of the rolling body.
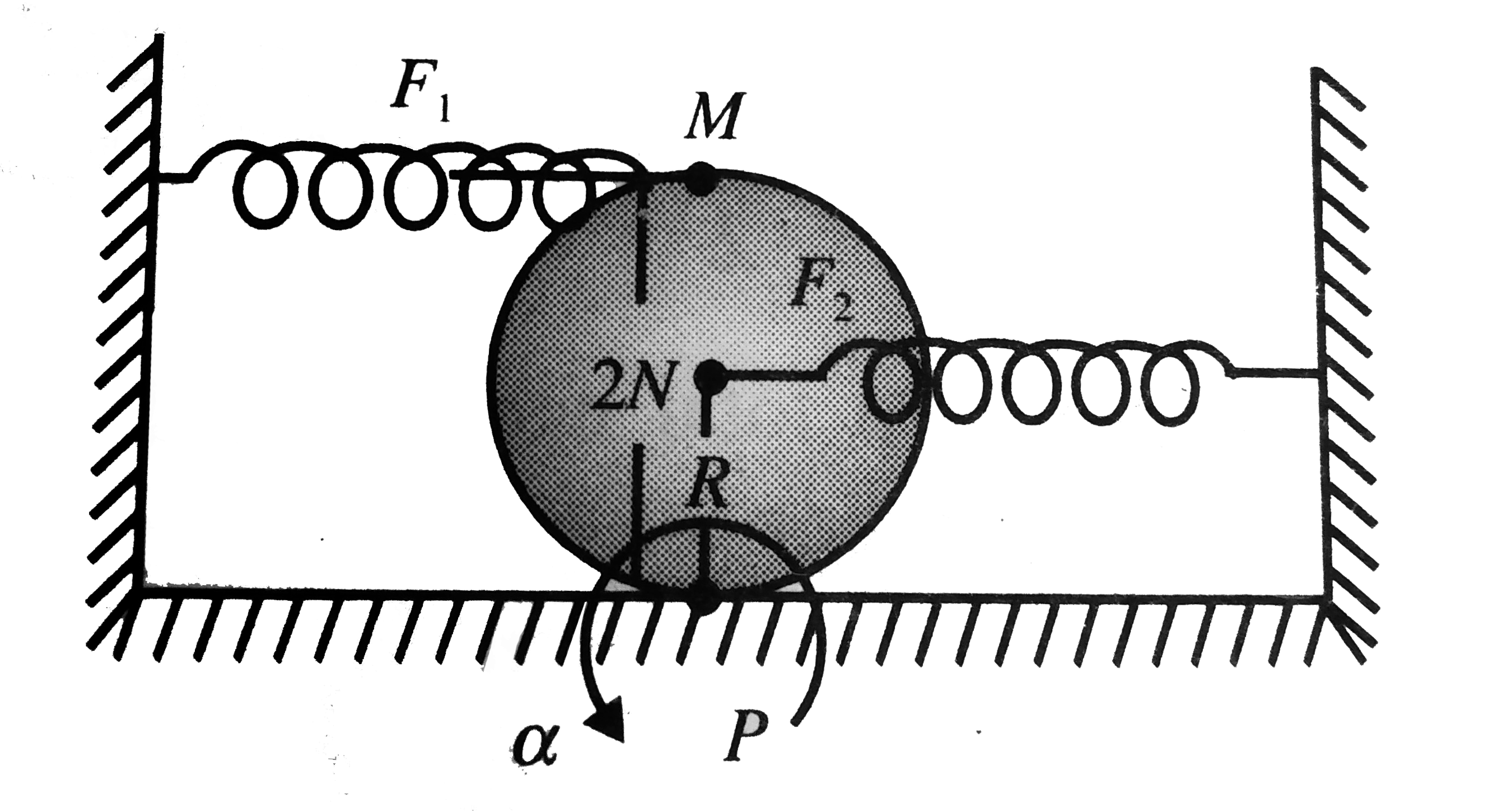
The spring forces F1andF2 produce anticlockwise torques F1(2R)andF2(R), respectively about P. Summing up the spring tarque about P, we have
τ≠t=F1(2R)andF2(R)
where F1=k1x1=k1(2Rθ)
and F2=k2x2=k2(Rθ)
This gives τnet=−(4R2k1+R2k2)θ=−(4k1+k2)R2θ
This tarque produces anticlokwise acceleration α for clocwise displacement θ , which can be given as
α=τ≠tlP=−(4k1+k2)R2θlP
Substituting lP=mR2+lC=mR2+mR22=3mR22
We have α=−2(4k1+k2)3mθ
Comparing the above equation with α=−ω2θ, we have
ω=√2(4k1+k2)3m
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Single Correct107videos
LINEAR AND ANGULAR SIMPLE HARMONIC MOTION
- BOOK - CENGAGE PHYSICSCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Multiple Correct35videos
LINEAR AND ANGULAR SIMPLE HARMONIC MOTION
- BOOK - CENGAGE PHYSICSCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Exercise 4.223videos
LINEAR AND ANGULAR SIMPLE HARMONIC MOTION
- BOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Interger11videos
KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICS
- BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type3videos
MISCELLANEOUS KINEMATICS
Similar Questions
A very light rod of length l pivoted at O is connected with two springs of stiffness k1 & k2 at a distance of a & l from the pivot respectively. A block of mass ma attached with the spring k2 is kept on a smooth horizontal surface. Find the angular frequency of small oscillation of the block m.
_S01_031_Q01.png) View Solution
View SolutionTwo blocks of masses m and m' are connected by a light spring on a horizontal frictionless table . The spring is compressed and then released . Find the ratio of acceleration of masses .
View Solution
Knowledge Check
- Question 1 - Select One
A disc of mass M and radius R has a spring of constant k attached to its center , the other end of the spring being fixed to a vertical wall. If the disk rolls without slipping on a level floor, how far to the right does the centre of mass move , if Initially the spring was unstretched and the angular speed of the disc was ω0
 ARω√(2M/3k)BRω√(3M/2k)CRω√(M/k)DRω√(M/2k)
ARω√(2M/3k)BRω√(3M/2k)CRω√(M/k)DRω√(M/2k) - Question 2 - Select One
In the figure shown a block of masss m is atteched at ends of two spring The other ends of the spring are fixed The mass m is released in the vertical plane when the spring are released The velocity of the block is maximum when
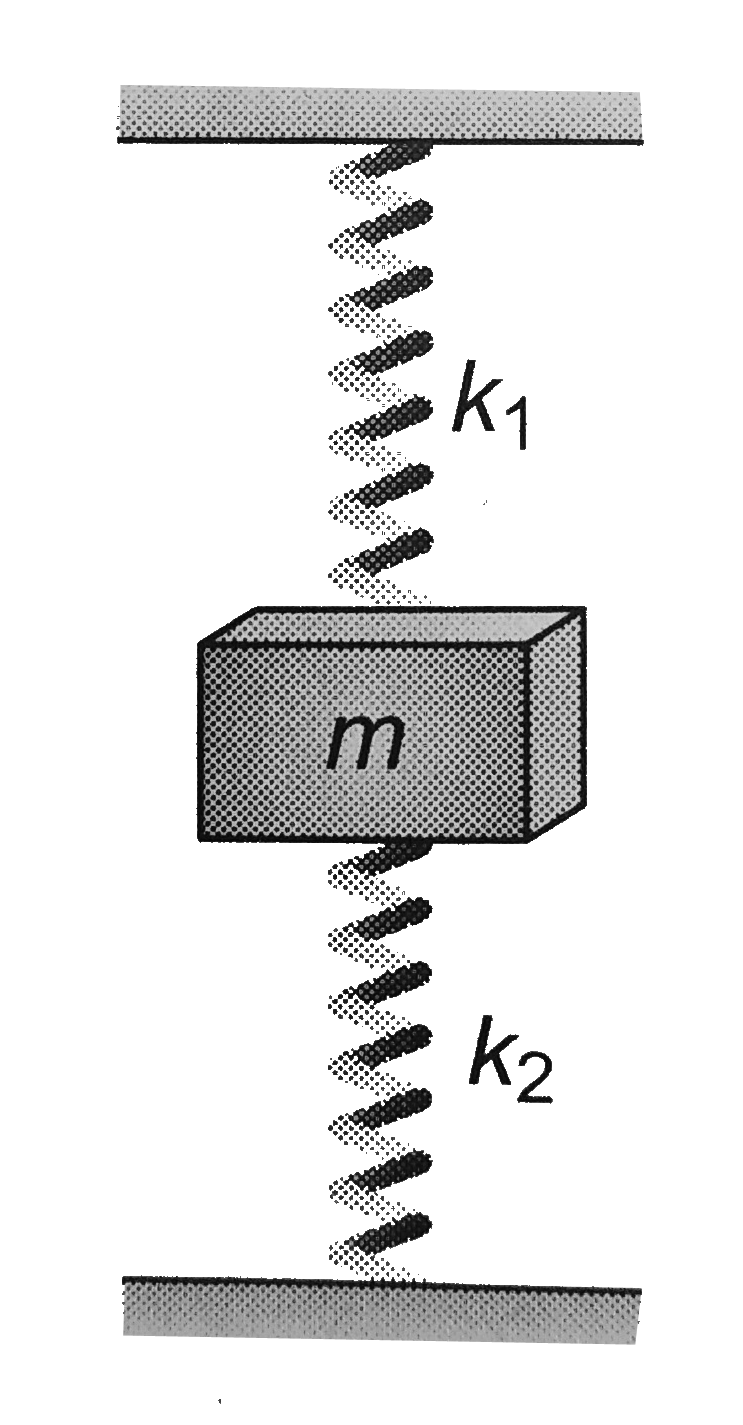 Ak1 is compressed and k2 is elongatedBk1 is elongated and k2 is compressedCk1 and k2 both are compressedDk1 and k2 both are elongated.
Ak1 is compressed and k2 is elongatedBk1 is elongated and k2 is compressedCk1 and k2 both are compressedDk1 and k2 both are elongated. - Question 3 - Select One
On a smooth inclined plane a body of mass M is attached between two springs. The other ends of the springs are fixed to firm supports. If each spring has a force constant k, the period of oscilation of the body is (assuming the spring as massless)
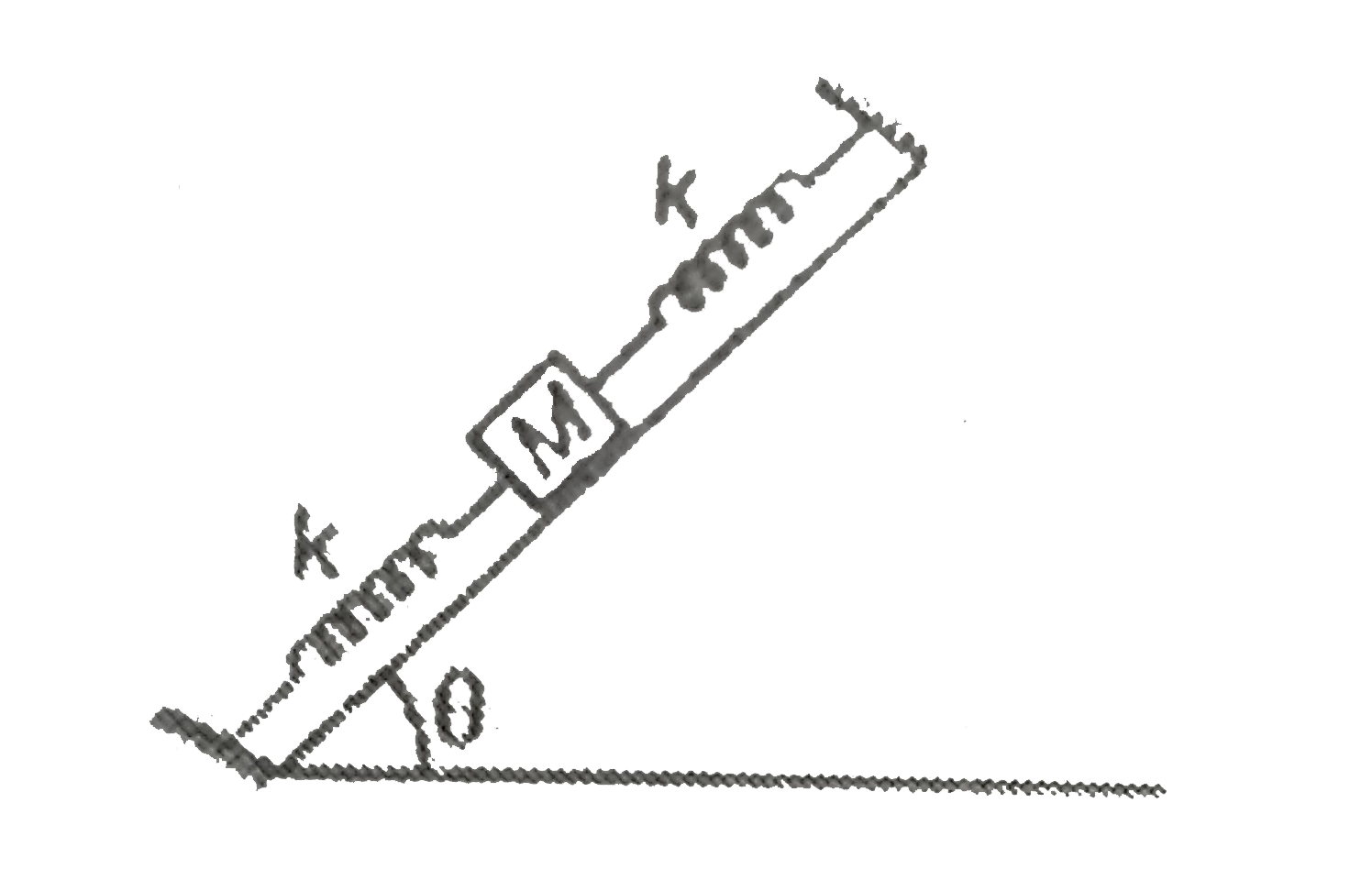 A2π√M2kB2π√2Mk)C2π√Msinθ2kD2π√2Msinθk)
A2π√M2kB2π√2Mk)C2π√Msinθ2kD2π√2Msinθk)
A straight rod of light L and mass m is pivoted freely from a point of the roof. Its lower end is connected to an ideal spring of spring constant K. The other end of the spring is connected to the wall as shown in the figure. Find the frequency of small oscillation of the system.
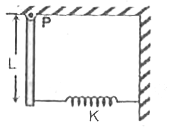 View Solution
View SolutionOn a smooth inclined plane a body of mass M is attached between two springs. The other ends of the spring are fixed to ffirm supports. If each spring has a force constant k, the period of oscillation of the body is: (assuming the spring as massless)
 View Solution
View SolutionOn a smooth inclined plane, a body of mass M is attached between two springs. The other ends of the springs are fixed to firm supports. If each spring has force constant K , the period of oscillation of the body (assuming the springs as massless) is
 View Solution
View SolutionA uniform thin cylindrical disk of mass M and radius R is attaached to two identical massless springs of spring constatn k which are fixed to the wall as shown in the figure. The springs are attached to the axle of the disk symmetrically on either side at a distance d from its centre. The axle is massless and both the springs and the axle are in horizontal plane. the unstretched length of each spring is L. The disk is initially at its equilibrium position with its centre of mass (CM) at a distance L from the wall. The disk rolls without slipping with velocity →V0=vacV0ˆi. The coefficinet of friction is μ.
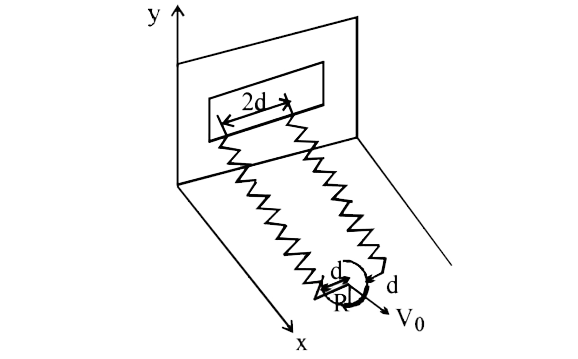
The centre of mass of the disk undergoes simple harmonic motion with angular frequency ω equal to -View SolutionTwo springs are joined and connected to mass m as shown. If the springs separately have force constants K1 and K2 , then frequency of oscillation of mass m is
 View Solution
View Solution

