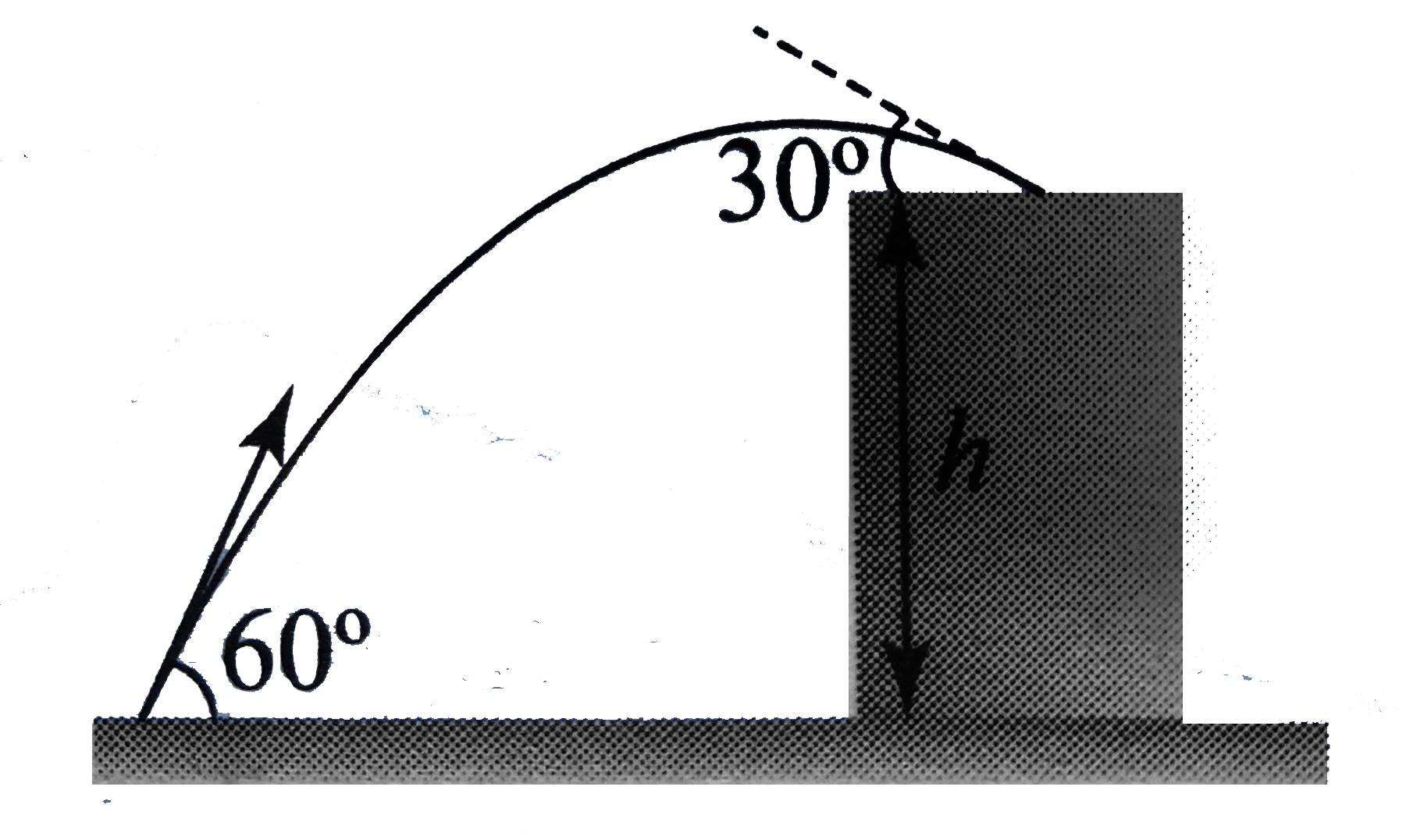
A stone projected at an angle of 60^@ from the ground level strikes at

A particle at a height $h$ from the ground is projected with an angle $30^{\circ}$ from the horizontal, it strikes the ground making angle $45^{\circ}$ with horizontal. It is again projected from the same point with the same speed but with an angle of $60^{\circ}$ with horizontal. Find the angle it makes with the horizontal when it strikes the ground
A ${tan}^{- 1} (4)$
B ${tan}^{- 1} (5)$
C ${tan}^{- 1} (\sqrt{5})$
D ${tan}^{- 1} (\sqrt{3})$
A stone is thrown from the top of a tower at an angle of $30^{\circ}$ above the horizontal level with a velocity of 40 m/s. it strikes the ground after 5 second from the time of projection then the height of the tower is
A20 m
B25 m
C40 m
D55m
A stone projected at angle $θ$ with horizontal from the roof of a tall building falls on the ground after three seconds. Two second after the projection it was again at the level of projection. Then the height of the building
A $5 m$
B25 m
C20m
D15m

A stone projected at an angle of $60^{\circ}$ from the ground level strikes at an angle of $30^{\circ}$ on the roof of a building of height $h$ . Then the speed of projection of the stone is: