A particle of mass 'm' and initially at rest is acted by a force F=a t. Newtons best representation of force-displacement graph is:
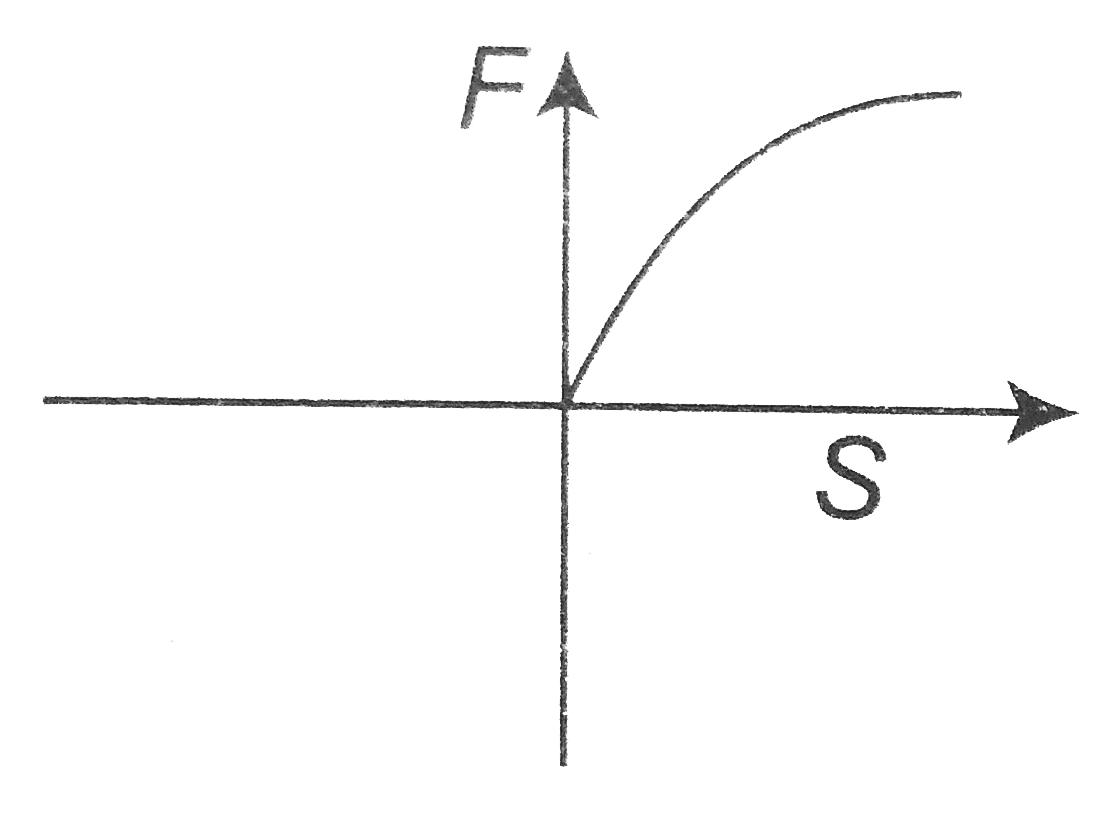
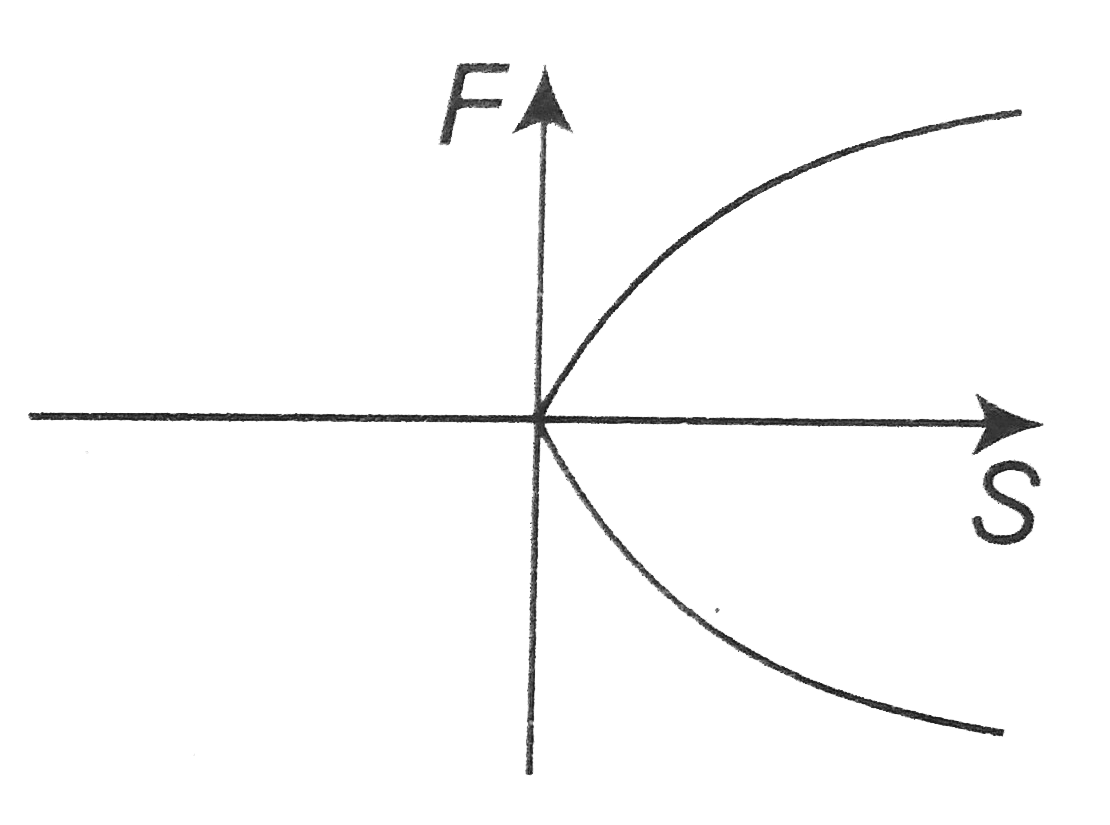
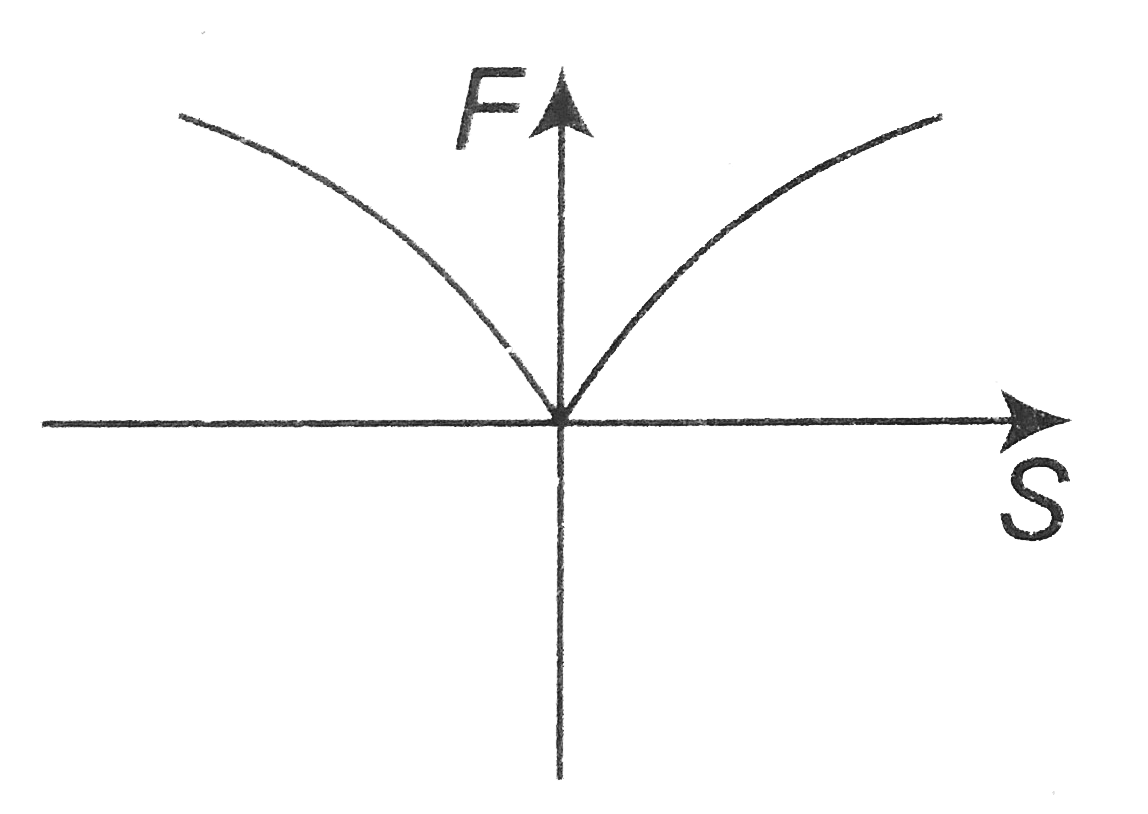
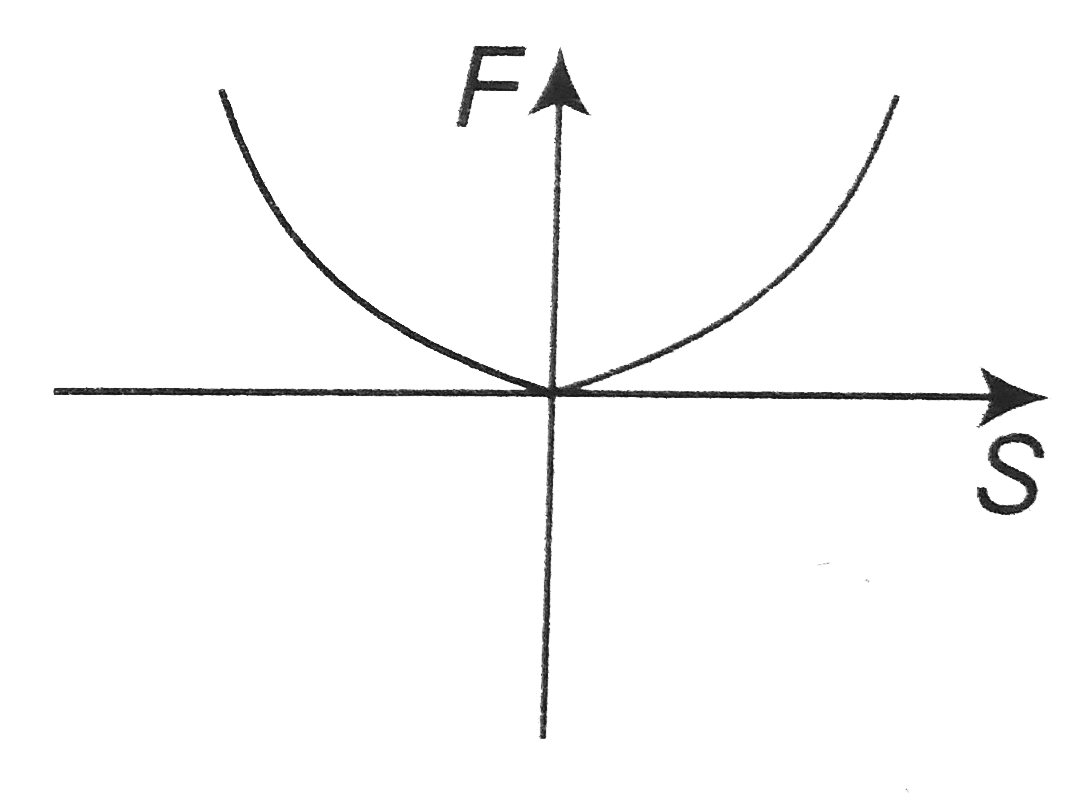
The correct Answer is:A
F=at,mdvdt=at,∫mdv=∫atdt
mv=at22,mdsdt=at22
∫mds=∫at22dt
ms=at36=a6(Fa)3 ,
:. S∝F3 or F∝S1/3 .
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBOOK - A2ZCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Equilibrium Of A Particle
19 Videos
- NEWTONS LAWS OF MOTIONBOOK - A2ZCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Applications Of Newton'S Laws Of Motion
33 Videos
- NEWTONS LAWS OF MOTIONBOOK - A2ZCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Chapter Test
30 Videos
- MOTION IN TWO DIMENSIONBOOK - A2ZCHAPTER - MOTION IN TWO DIMENSIONEXERCISE - Chapter Test
29 Videos
- OSCILLATION AND SIMPLE HARMONIC MOTIONBOOK - A2ZCHAPTER - OSCILLATION AND SIMPLE HARMONIC MOTIONEXERCISE - Chapter Test
29 Videos
Similar Questions
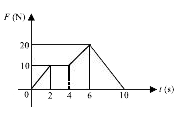
A particle of mass 2 kg is initially at rest. A force , acts on it whose magnitude changes with time. The force-time graph is of shown here.
The velocity of the particle after 10 s is
View SolutionA particle of mass m , initially at rest , is acted upon by a variable force F for a brief interval of time T. It begins to move with a velocity u after the forrce stops acting . F is shown in the graph as a function of time. The curve is a semicircle.

View Solution
Knowledge Check
A block of mass m at rest is acted upon by a force F for a time t. The kinetic energy of block after time t is
A2 FtmB2FtmCFtmDF2t22mA block of mass m at rest is acted upon by a force F for a time t. The kinetic energy of block after time t is
AF2t2mBF2t22mCF2t23mDFt2mA particle of mass m, initially at rest is acted upon by a variable force F for a brief interval of time T. It begins to move with a velocity u after the force stops acting. F is shown in the graph as a function of time. The curve is a semicircle. Then
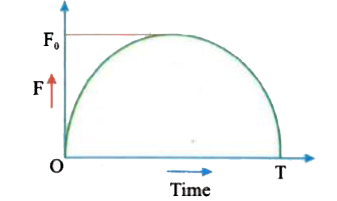 Au=πF202mBu=πT28mCu=πF0T4mDu=πF0T2m
Au=πF202mBu=πT28mCu=πF0T4mDu=πF0T2m
A particle of mass m initially at rest. A variabl force acts on the particle f=kx2 where k is a constant and x is the displacment. Find the work done by the force f when the speed of particles is v.
View SolutionA block of mass m at rest is acted upon by a force F for a time t. The kinetic energy of block after time t is
View SolutionA particle of mass m at rest is acted upon by a force P for a time t. Its kinetic energy after an interval t is
View SolutionA particle of mass m at rest is acted upon by a force P for a time t. Its kinetic energy after an interval t is
View SolutionA particle of mass m initially at rest is acted upon by a unidirectional force F=F∘cos(π2t). The distance travelled by the particle when it stops first in the subsequent motion is ,
View Solution